Transport analysis of the EHL-2 spherical torus in a high-ion-temperature scenario

1.   Introduction

The EHL-2 project is aimed at developing a spherical torus experimental device to address key physics issues associated with p-¹¹B fusion plasmas [1, 2]. The main design parameters for EHL-2 are the major radius R₀ = 1.05 m, plasma current I_p = 3 MA, and toroidal magnetic field B_T = 3 T. The main purpose of this project is to achieve high-performance plasmas, such as plasmas of temperatures above 20 keV and/or densities in the order of 10²⁰ m⁻³, required for proton–boron fusion in a spherical torus configuration. One of the most severe challenges for the EHL-2 project is how to effectively dissipate the huge amount of power crossing the separatrix from the core plasma to the scrape-off layer (SOL) [3], before reaching the plasma facing components (PFCs), especially the divertor targets, to avoid excessive erosion of material and thus to ensure the sustainability and safety of PFCs. For proton–boron fusion, the required ion temperature (T_i) (~ 200 keV) is over ten times higher than that for deuterium–tritium fusion (in the order of 10 keV) [2, 4], making the challenges in divertor power exhausting much more severe. Therefore, it is essential to explore divertor configurations that are superior to those of ITER [5], featuring substantial flux expansion near the strike points. For this purpose, an advanced divertor with an X-point target (XPT) [6] divertor has been designed to handle the heat load effectively and to ensure the feasibility of achieving and maintaining the plasma conditions of high performances.

Similar to the case of ITER [7, 8], detached/semi-detached regimes [9, 10] are required for EHL-2 to meet the condition of acceptable heat loads ($\leqslant5\;\mathrm{M}\mathrm{W}/{\mathrm{m}}^{2}$) [11] on divertor targets. Hence, the goal of the divertor physics design is to achieve divertor detachment at relatively low plasma densities so as to achieve good compatibility with the high plasma confinement. In addition, to avoid excessive erosion of the target material, the peak values of plasma temperatures at the divertor plates should be $< 5-10\;\mathrm{e}\mathrm{V}$ [9], and the greatest threat to the safety of divertors may be posed by the high ion temperature operation mode of EHL-2. The divertor design, considering the above-mentioned physics requirements and engineering constraints, will be presented in the following sections.

This paper is structured as follows. The general objectives and requirements for the divertor physics design in EHL-2 are described in section 2. In section 3, the preliminary design of the divertor geometry via the particle tracking strategy is introduced. In addition, detailed SOLPS-ITER [12, 13] simulations of divertor/SOL plasmas in EHL-2 with the preliminary target plate geometry are presented in section 4. Finally, the conclusions are given in section 5.

2.   Basic concept of divertor physics design

This section describes the basic plasma conditions based on which the divertor design has been performed. In order to maximize the controllability of the divertor heat load, a reference magnetic equilibrium with the XPT divertor configuration was proposed, the main characteristics of which are depicted in figure 1 [14]. The divertor configuration shown in figure 1 has a conventional inner divertor and an XPT outer divertor of a remarkable flux expansion.

Figure  1.  Equilibrium used for EHL-2 divertor physics design.

DownLoad: Full-Size Img PowerPoint

EHL-2 has three primary operation scenarios: the high ion temperature scenario, the high-performance steady-state scenario, and the high triple product scenario, among which the high T_i scenario is expected to pose the greatest challenge for the control of the divertor load [15]. Therefore, a reference discharge in the high T_i scenario is selected for the divertor physics design in EHL-2. This reference discharge has a maximum toroidal field (B_T) of 3 T, a maximum plasma current (I_p) of 3 MA, and a total heating power (P_in) of 20 MW. In addition, the safety factor at the 95% poloidal flux surface q₉₅ is about 6.59. The power decay length ${\lambda }_{\mathrm{q}}$ for EHL-2, a key parameter determining the peak divertor heat load, can be extrapolated from the experimental scaling [16] (${\lambda }_{\mathrm{q}}=0.7{B}_{\mathrm{T}}^{-0.77}{q}_{95}^{1.05}{P}_{\mathrm{S}\mathrm{O}\mathrm{L}}^{0.09}\approx 3\;\mathrm{m}\mathrm{m}$). Based on the design parameters shown above, the peak heat load on the inner target (IT) (${q}_{\mathrm{p}\mathrm{e}\mathrm{a}\mathrm{k}\_\mathrm{i}}$) and that on the outer target (OT) (${q}_{\mathrm{p}\mathrm{e}\mathrm{a}\mathrm{k}\_\mathrm{o}}$) can be estimated by ${q}_{\mathrm{p}\mathrm{e}\mathrm{a}\mathrm{k}\_\mathrm{i},\mathrm{o}}={q}_{\mathrm{p}\mathrm{e}\mathrm{a}\mathrm{k}\_\parallel \_\mathrm{i},\mathrm{o}}\mathrm{s}\mathrm{i}\mathrm{n}\theta$, where ${q}_{\mathrm{p}\mathrm{e}\mathrm{a}\mathrm{k}\_\mathrm{||}\_\mathrm{i}}$ and ${q}_{\mathrm{p}\mathrm{e}\mathrm{a}\mathrm{k}\_\mathrm{||}\_\mathrm{o}}$ are the peaks of the parallel heat flow on the high-field side (HFS) and low-field side (LFS), respectively, which are given by ${q}_{\mathrm{p}\mathrm{e}\mathrm{a}\mathrm{k}\_\mathrm{||}\_\mathrm{i},\mathrm{o}}={P}_{\mathrm{S}\mathrm{O}\mathrm{L}}/{A}_{\mathrm{i},\mathrm{o}}$, and $\theta$ is the pitch angle ($\theta \sim 5 {\text{°}}$), $A\mathrm{_i}$ and $A\mathrm{_o}$ are the plasma wetted area at the inner target plate and that at the outer one, respectively, which are given by ${A}_{\mathrm{i},\mathrm{o}}=2{\text{π}}{R}_{\mathrm{s}\_\mathrm{i},\mathrm{o}}{\lambda }_{\mathrm{q}\_\mathrm{i},\mathrm{o}}$ ($\lambda_{\mathrm{q}_{\mathrm{o}}}\approx\lambda_{\mathrm{q}}\times f_{\mathrm{x}_{\mathrm{o}}}$, $\lambda_{\mathrm{q}_{\mathrm{i}}}= \lambda_{\mathrm{q}_{\mathrm{o}}}\times f_{\mathrm{x}_{\mathrm{i}}}/f_{\mathrm{x}_{\mathrm{o}}}$), where ${f}_{\mathrm{x}\_\mathrm{i}}\approx 9$ and ${f}_{\mathrm{x}\_\mathrm{o}}\approx 23$ are the flux expansion on the HFS and LFS, respectively. ${R}_{\mathrm{s}\_\mathrm{i}}=0.62\;\mathrm{m}$ and ${R}_{\mathrm{s}\_\mathrm{o}}=1.36\;\mathrm{m}$ are the major radii at the inner strike point and outer strike point. Assuming that the radiative power loss is about half of ${P}_{\mathrm{i}\mathrm{n}}$, i.e. ${P}_{\mathrm{S}\mathrm{O}\mathrm{L}}=10\;\mathrm{M}\mathrm{W}$, and further assuming that one-third of the power goes to the HFS, and upper and lower targets receive equal power in double-null (DN) geometry, ${q}_{\mathrm{p}\mathrm{e}\mathrm{a}\mathrm{k}\_\mathrm{i}}$ and ${q}_{\mathrm{p}\mathrm{e}\mathrm{a}\mathrm{k}\_\mathrm{o}}$ are estimated to be 5.6 MW/m² and 0.5 MW/m². This indicates that the inner target plate may face a more serious challenge than the outer one. It is notable that Eich scaling is derived from the inter-edge localized mode (ELM) phase under attached divertor conditions. However, to protect the PFCs of the divertor, EHL-2 is designed to operate in a type-I ELM mitigation scenario with a detached divertor at high power. Experiments and modelling work [17–21] indicate that in the ELM-suppressed and detached phase, the power decay length may broaden, offering a potentially favourable outcome. Moreover, the divertor configuration can also influence the power decay length [22, 23]. While predicting that the exact decay length remains an open challenge, in this work, we have assumed a value that falls within an optimistic yet reasonable range. In addition, the core T_i is anticipated to reach 30 keV and can even exceed 50 keV. The core electron temperature is expected to be around 10–20 keV and the central line-averaged electron density is $6\times {10}^{19}\;{\mathrm{m}}^{-3}$. A lower plasma density at the upstream separatrix is more favourable for achieving high T_i.

In EHL-2, PFCs, including the divertor targets, will be made from carbon-based materials (i.e. CFC). Generally, the heat load limit, i.e. the maximum heat flux onto the target plate that is tolerable for the operation safety, depends on the thermal conductivity of materials. CFC material with a thermal conductivity of 300 W/(m·°C) has been selected as the target material for EHL-2, thereby balancing the performance and cost. Numerous modelling and experimental tests on the performances of the selected CFC material have been performed. It is found that in the test case with a heat flux of 5 MW/m² and continuous water cooling, the surface temperature increases to about 1000 °C; meanwhile, when the heat flux is increased to 7–10 MW/m², the surface temperature of the CFC material can even be increased to above 2000 °C, causing remarkable erosions of the CFC material. Hence, the heat flux limit on the selected CFC material is demonstrated to be ~ 5 MW/m².

Firstly, particle tracking strategies were used to obtain a quick estimation of the heat load on PFCs to facilitate the determination of the divertor-target position and geometry. Then, the SOLPS-ITER code was used for a more detailed divertor physics design, exploring the required plasma conditions for the onset of detachment.

3.   Divertor target geometry

Extensive efforts have been put into the EHL-2 divertor geometry design and the divertor performance evaluation. The preliminary shape and position of the target plate have been determined by considering the accommodation of various equilibrium configurations and by accounting for the structure, pumping and support space of the vacuum chamber. As shown in figure 2 (red lines), the proposed divertor structure can easily adapt to the requirements of adjusting the XPT divertor configuration (see blue and black lines in figure 2). However, as described above, detached operation regimes are required to keep the heat loads on divertor targets at a tolerable level. Therefore, the divertor closure needs to be maximized to facilitate the onset of detachment. To this end, the first step in the divertor design is a rapid (qualitative) estimation of the heat load distribution on the PFCs, such as the divertor targets and baffles, and a heat load proxy model is used for the estimation. In this model, transport parallel to the field lines is treated as anisotropic diffusion, which can be viewed as an extension of the field line tracing process. It can be used for the estimation of heat loads on PFCs in fusion devices in various different magnetic geometries, including tokamaks [24] and stellarators [25]. An important parameter in this model is the isotropic perpendicular diffusion coefficient ${D}_{\perp }$ to the ratio of the anisotropic parallel diffusion coefficient ${D}_{||}$ to (${D}_{\perp }/{D}_{||}$), which is set to 10⁻⁷ following [25].

Figure  2.  Preliminary design of the baffles on LFS and HFS.

DownLoad: Full-Size Img PowerPoint

To avoid excessive erosion of the baffle, the innermost radial position at which it can be placed has been predicted via the above-introduced model for heat load estimation. Figure 3 shows the estimated distribution of heat load on PFCs for the cases denoted as LFS1, LFS2 and LFS3, respectively, where LFS1, LFS2 and LFS3 represent that the baffles on the LFS are located at a short, medium and long radial distance to the separatrix (figures 2 and 3), respectively. For the LFS1 case with a short radial distance to the separatrix, a significant amount of heat power is deposited onto the baffle on the LFS. As the baffle on the LFS moves outward (for the LFS2 case), the radial distance to the last closed flux surface increases, but the divertor closure decreases. However, the heat load on baffles on the LFS decreases significantly in the LFS2 case, compared with that in the LFS1 case. As the outboard baffle moves further outward, there is an almost negligible heat load on the baffle (for the LFS3 case). In summary, for the position of the baffle on the LFS, the LFS2 case is a moderate choice.

Figure  3.  The estimated heat load distribution on PFCs for the cases LFS1, LFS2 and LFS3, respectively. LFS1, LFS2 and LFS3 represent that the baffles at the LFS are located at a short, medium and long radial distance to the separatrix, respectively.

DownLoad: Full-Size Img PowerPoint

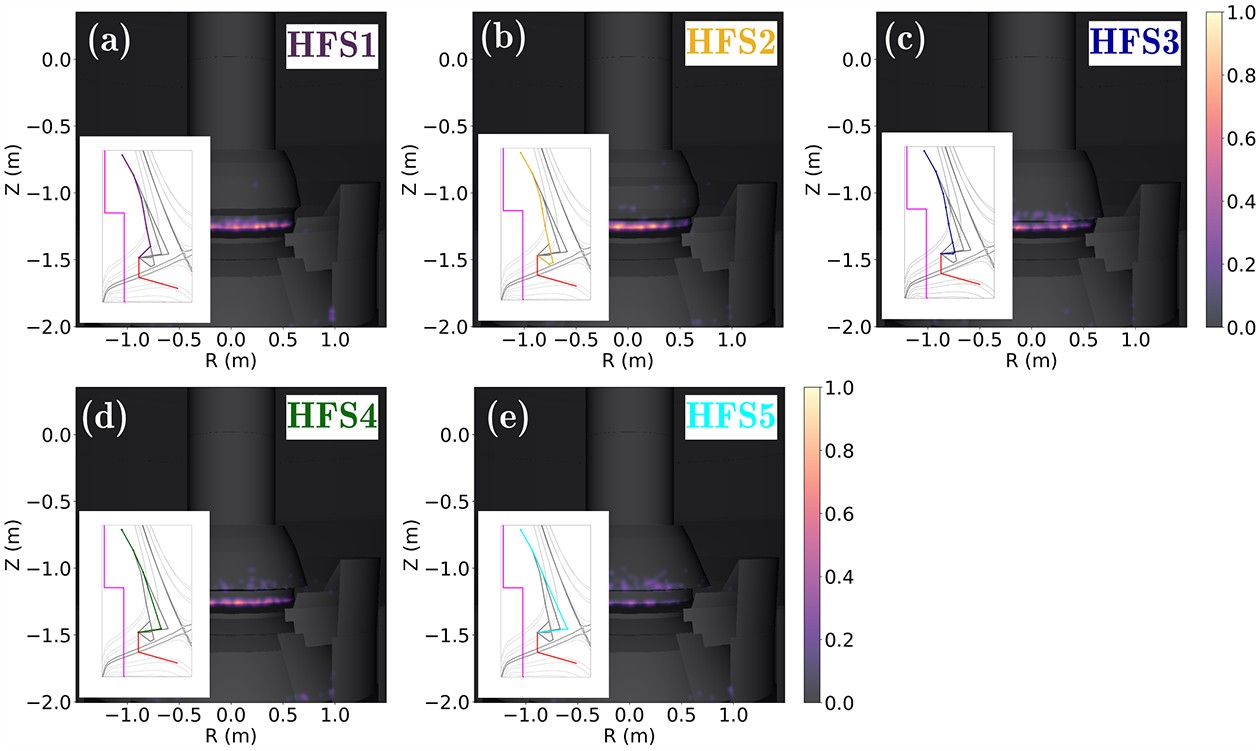
From the estimations via the particle tracing strategy, the divertor target plate on the HFS confronts higher heat loads than that on the LFS. Therefore, much more attention has been paid to the design of the inner divertor. Figure 4 shows the estimated heat load distribution on the PFCs for the cases denoted as HFS1, HFS2, HFS3, HFS4 and HFS5, respectively, where HFS1, HFS2, HFS3, HFS4 and HFS5 present five different shapes of baffles on the HFS (figures 2 and 4). From the comparisons among the HFS1, HFS2 and HFS3 cases, the heat loads on baffles on the HFS in the HFS2 case are acceptable. The baffles will face a greater heat load if the closure is increased further (see the HFS3 case). The baffle in the HFS2 case can be extended inward to some extent (case HFS4), but not too much, and further downward extension of the baffles (as seen in case HFS5) is not feasible. The HFS baffle can ultimately be designed as per that for the HFS4 case.

Figure  4.  The estimated heat load distribution on PFCs for the cases HFS1, HFS2, HFS3, HFS4 and HFS5, respectively. HFS1, HFS2, HFS3, HFS4 and HFS5 denote five different shapes of the baffles on the HFS highlighted in the panels (a), (b), (c), (d) and (e), respectively (see also figure 2).

DownLoad: Full-Size Img PowerPoint

Based on the quick estimation of heat load distribution on the PFCs via the proxy model, we determined the innermost flux surfaces at which the LFS/HFS baffles can be located. Moreover, to ensure compatibility with various plasma equilibria, a divertor geometry as shown in figure 5 was proposed for EHL-2. The divertor configuration shown in figure 5 exhibits good closure on both the LFS and HFS. However, the inner side of the target plate on the HFS is limited by engineering constraints, preventing further inward movement. For better accommodation of the secondary X-point on the LFS, the outer divertor target is designed as the combination of a vertical plate and a horizontal one. Additionally, the horizontal plate for the OT is connected to baffles in the private flux region (PFR) and the vertical one is connected to the passive plate.

Figure  5.  Preliminary design of divertor targets (red lines).

DownLoad: Full-Size Img PowerPoint

4.   SOLPS-ITER simulation results

4.1   Divertor detachment

The SOLPS-ITER code [8, 9], which couples the three-dimensional (3D) kinetic model EIRENE [13] for tackling neutral behaviours (e.g. ionization, recombination and charge-exchange, etc.) and the 2D multi-fluid plasma solver B2.5 [26] for handling behaviours of ions and electrons, was used to simulate the divertor/SOL plasmas in EHL-2 for evaluating the steady-state divertor heat loads, focusing on the semi-detached/detached operation regimes. According to [27], to mitigate the issue of power exhaust in spherical tokamaks with tight aspect ratios, a DN configuration is advantageous over a single-null (SN) one. Hence, this work focuses on divertor performances in plasmas under the connected double-null (CDN) configuration. However, practical control of the plasma vertical stability will likely lead to an oscillation around the symmetry point, which may lead to transient loading of the divertors. Therefore, the impact of the degree of disconnection on in–out/up–down power sharing will be addressed in the next step but not included in this manuscript. Figure 6 shows the grid (96 poloidal × 36 radial meshes) for SOLPS-ITER calculations, which has been generated based on a CDN magnetic equilibrium with an XPT divertor configuration (figure 2).

Figure  6.  Grid for B2.5 in SOLPS-ITER calculations. Shown is the divertor geometry considered in the simulations.

DownLoad: Full-Size Img PowerPoint

The hydrogen plasma species (H⁰, H⁺), boron species (B⁰, B⁺, B²⁺, B³⁺, B⁴⁺, B⁵⁺) and carbon impurity species (C⁰, C⁺, C²⁺, C³⁺, C⁴⁺, C⁵⁺, C⁶⁺) are considered in the simulations. The total power crossing the core–edge interface (CEI) from the core to the calculation region is specified to be P_CEI = 20.0 MW. In addition, P_CEI is assumed to be equally shared between ions and electrons, which is a commonly used assumption in fluid boundary plasma modelling using SOLPS-like (e.g. UEDGE [28], EDGE2D [29], SONIC [30, 31], etc.) code. The parallel plasma transport is assumed to be classical [3] and the cross-field (radial) transport coefficients are set as spatial constants for all species: the anomalous particle diffusivity ${D}_{\perp }$ = 0.3 m²‧s⁻¹ and the anomalous ion and electron thermal diffusivity ${\chi}_{\perp,{\mathrm{i}} }$ = ${\chi}_{\perp,{\mathrm{e}} }$ = 1.0 m²‧s⁻¹. Consequently, in all the simulation cases, the outboard mid-plane (OMP) radial gradient scale lengths for density (${\lambda }_{\mathrm{n}}$) and temperature (${\lambda }_{\mathrm{T}}$) are ${\mathrm{\lambda }}_{\mathrm{n}}\sim 1.6-2.5\;\mathrm{c}\mathrm{m}$ and ${\mathrm{\lambda }}_{\mathrm{T}}\sim 2.4-4.1\;\mathrm{m}\mathrm{m}$ (gradient scale lengths in the detached regimes are found to be larger than those in attached regimes), respectively, which are similar to those for current tokamaks [32]. The hydrogen molecule (D₂) and boron atom (B) are mixed in the same proportion and are puffed from one wall segment located at the OMP. According to [33], the optimal conditions for achieving thermal nuclear reactions occur at n_boron/n_ion $\cong$ 14%, where n_boron/n_ion is the ratio of the boron density to the total ion density. As the concentration of boron increases, the rate of growth in the beam thermal reaction gradually declines. Hence, in our simulation cases, n_boron/n_ion in the core has been kept at $\lesssim 14\mathrm{\%}$. Carbon impurities were introduced in the simulations due to the fact that the divertor targets will be made from carbon-based material. The carbon sputtering rate is fixed at 4%. The designated surface for extracting neutral particles has a recycling coefficient of 0.9. Drift effects are not included in the simulations presented in section 4.1, but will be addressed in section 4.2. Since both the divertor geometry and magnetic configuration are up–down symmetrically shaped on both sides of the mid-plane, no up–down asymmetry in the plasma profiles has been observed for all the simulations presented in section 4.1.

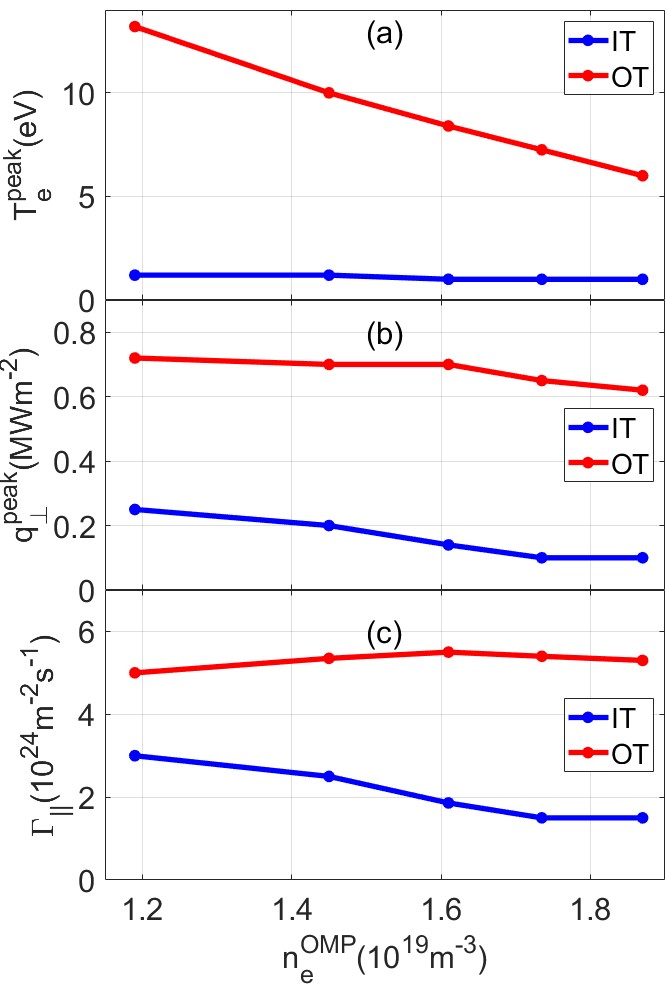
To quantitatively describe the onset of divertor detachment, a systematic scan of the separatrix plasma density at the OMP (${n}_{\mathrm{e}}^{\mathrm{O}\mathrm{M}\mathrm{P}}$) has been carried out. Figure 7 illustrates the peak values of the electron temperature (T_e), perpendicular heat load (${q}_{\perp }$) and parallel particle flux density (${\mathrm{\Gamma }}_{\parallel }$) at the inner and outer targets as a function of ${n}_{\mathrm{e}}^{\mathrm{O}\mathrm{M}\mathrm{P}}$. It can be seen that when ${n}_{\mathrm{e}}^{\mathrm{O}\mathrm{M}\mathrm{P}}$ increases to $1.62\times10^{19}\mathrm{\ m}^{-3}$, the peak values of T_e on both the IT and OT can be reduced to below 10 eV, and the peak values of ${q}_{\perp }$ on both targets can be reduced to below 1 MW/m². At the same time, ${\mathrm{\Gamma }}_{\parallel }$ begins to decrease, and the plasma enters the divertor detachment regime. Hence, the results of SOLPS-ITER calculations indicate that the designed divertor, shown in figure 6, has good closure and can achieve a detached operation regime at a relatively low upstream plasma density. The ease access to divertor detachment in EHL-2 predicted by SOLPS-ITER also suggests that the XPT divertor configuration with a remarkable flux expansion has a great advantage in power handling.

Figure  7.  Peak values of the electron temperature ${T}_{\mathrm{e}}^{\mathrm{p}\mathrm{e}\mathrm{a}\mathrm{k}}$ (a), perpendicular heat flux ${q}_{\perp }^{\mathrm{p}\mathrm{e}\mathrm{a}\mathrm{k}}$ (b), and parallel particle flux density ${\mathrm{\Gamma }}_{\parallel }$ (c) at the inner and outer targets, as a function of the separatrix plasma density at the outboard mid-plane (${n}_{\mathrm{e}}^{\mathrm{O}\mathrm{M}\mathrm{P}}$).

DownLoad: Full-Size Img PowerPoint

As mentioned above, the safe operation of the EHL-2 device requires that the divertor heat flux (q_t) should be controlled below 5 MW·m⁻² and the peak values of T_e at the target be controlled at < 5–10 eV. As shown in figure 7, both q_t and T_e at the IT are well below the operational safety threshold. The q_t at the OT can also be controlled below 5 MW·m⁻², but the peak value of T_e is close to the operational safety threshold. For plasmas with low upstream plasma density, how to effectively reduce the plasma temperature at the OT will become the focus of future research. In the following section, the main characteristics of plasmas profiles for the simulation case with $n_{\mathrm{e}}^{\mathrm{O}\mathrm{M}\mathrm{P}}= 1.62\times10^{19}\ \mathrm{m}^{-3}$ will be described.

Figure 8 shows the radial profiles of electron density (n_e), T_e and T_i at the inner mid-plane (IMP) and OMP for the simulation case with ${n}_{\mathrm{e}}^{\mathrm{O}\mathrm{M}\mathrm{P}}$ increased to be $1.62\times10^{19}\mathrm{\ m}^{-3}$. The simulated T_i is much higher than T_e due to the relatively low collisionality between the electrons and ions.

Figure  8.  Radial profiles of n_e, T_e and T_i at the IMP and OMP for the simulation case with ${n}_{\mathrm{e}}^{\mathrm{O}\mathrm{M}\mathrm{P}}$ increased to $1.62\times {10}^{19}\;{\mathrm{m}}^{-3}$.

DownLoad: Full-Size Img PowerPoint

Figure 9 shows the radial profiles of T_e at the IT and the OT. Since the strike point on the LFS is located near the outer divertor corner featuring a good closure for neutrals, the radiative power loss in the outer divertor region near the strike point is much higher than that in the far SOL. Consequently, the peak of T_e target profiles at the outer side is not located near the strike point but at the position of ~ 30 cm along-target distance to the strike point, as shown in figure 9. Although the peak value of T_e in the far SOL is relatively high, the peak divertor heat load on the OT remains low (figure 7).

Figure  9.  The radial profiles of electron temperature at the IT (a) and the OT (b).

DownLoad: Full-Size Img PowerPoint

Figure 10 shows the radial profiles of the heat flux on the IT and the OT. The peak of the target heat flux profile at the inner side appears in the divertor region near the strike point, while that at the outer side is located at the position of ~ 10 cm along-target distance to the strike point.

Figure  10.  The radial profiles of heat flux (${q}_{\mathrm{t}\mathrm{a}\mathrm{r}\mathrm{g}\mathrm{e}\mathrm{t}}$) onto the IT (a) and the OT (b).

DownLoad: Full-Size Img PowerPoint

Figure 11 gives the radial profiles of n_e at the IT and the OT. The peak of the radial n_e target profile at the inner side appears near the strike point, and it is the same case as that at the outer side.

Figure  11.  The radial profiles of n_e at the IT (a) and the OT (b).

DownLoad: Full-Size Img PowerPoint

Figure 12 shows the 2D distributions of total radiation power, total neutral (H⁰, H₂, B⁰ and C⁰) density, total carbon (sum of C⁰ to C⁺⁶) density and total boron (sum of B⁰ to B⁺⁵) density. It is clear that many neutrals are trapped in the divertor region, facilitating the onset of divertor detachment at relatively low upstream plasma densities. In the simulation case with both divertors in detached regimes (i.e. with ${n}_{\mathrm{e}}^{\mathrm{O}\mathrm{M}\mathrm{P}}$ increased to 1.62×10¹⁹ m⁻³), the total radiative power loss is 12.5 MW. Carbon, boron and hydrogen contribute 10.9 MW, 0.04 MW and 1.56 MW to the total radiation power, respectively. In addition, the carbon density in the divertor region is much higher than that in the core, and thus the radiative power loss in the core plasma is relatively low, which may greatly benefit the achievement of high-performance plasmas in EHL-2.

Figure  12.  The 2D distributions of (a) total radiation power, (b) total neutral density, (c) total carbon impurity density and (d) total boron density.

DownLoad: Full-Size Img PowerPoint

The above-introduced SOLPS-ITER simulation results demonstrate that the divertor geometry shown in figure 6 exhibits good closure and can maintain a relatively high neutral particle pressure in the divertor region, facilitating efficient particle removal through pumping. Moreover, this divertor can greatly help with the dissipation of power heat at relatively low plasma densities, and thus facilitate access to divertor plasma regimes with high radiative power losses to reduce heat loads on the divertor plates. Additionally, it helps maintain the upstream neutral density at relatively low levels, which in turn decreases sputtering in the main chamber and may improve core plasma confinement.

4.2   Impact of drifts

Drifts [34, 35], such as the diamagnetic and ${\boldsymbol{E}}\times \boldsymbol{B}$ drifts, can significantly influence the heat flux distribution on tokamak divertors by causing asymmetry between the inner and outer targets as well as between the upper and lower ones, enhancing heat flux near strike points and affecting divertor detachment. They also impact plasma–wall interactions [36] and impurity transport [37], which in turn influences the heat load on the target plates. A profound understanding of drift effects on the transport properties of divertor/SOL plasmas is essential for optimizing the divertor performance and prolonging the lifetime of divertor components in the high T_i mode of EHL-2.

Figure 13 shows the typical drift-flow patterns of plasma ions in EHL-2 with the CDN configuration, which is the plasma configuration selected in our simulations. From figure 13, typically, plasma ions can be driven from the lower outer (LO) divertor region to the lower inner (LI) region through the PFR by both ${\boldsymbol{E}}_{r}\times \boldsymbol{B}$ and ${\boldsymbol{E}}_{\theta }\times \boldsymbol{B}$ drifts, causing in–out asymmetry in the divertor heat flux, but plasma ions in the LI divertor region can be moved towards the upper inner (UI) one by the ${\boldsymbol{E}}_{r}\times \boldsymbol{B}$ drift flow through the inner SOL. Similarly, plasma ions can be driven from the UI divertor region to the upper outer (UO) region through the PFR by both ${\boldsymbol{E}}_{r}\times \boldsymbol{B}$ and ${\boldsymbol{E}}_{\theta }\times \boldsymbol{B}$ drifts, but plasma ions in the UO divertor region can be moved towards the LO one by the ${\boldsymbol{E}}_{r}\times \boldsymbol{B}$ drift flow through the outer SOL. That is, plasma parameters (e.g., n_e, T_e, q_t) at the target plates may be greatly affected by drift-driven plasma flows in both the divertor and SOL regions. It is notable that the actual drift-flow patterns in the tokamak edge may be much more complicated than those shown in figure 13. Hence, to quantitatively describe the drift-driven processes in EHL-2, detailed modelling and analysis using SOLPS-ITER [12, 38] are performed and described in the following section. The main setup for SOLPS-ITER calculations is similar to those shown in section 4.1, but, here, the effects of drifts and currents are self-consistently considered.

Figure  13.  Typical drift flow pattern of plasma ions in EHL-2 with CDN configuration.

DownLoad: Full-Size Img PowerPoint

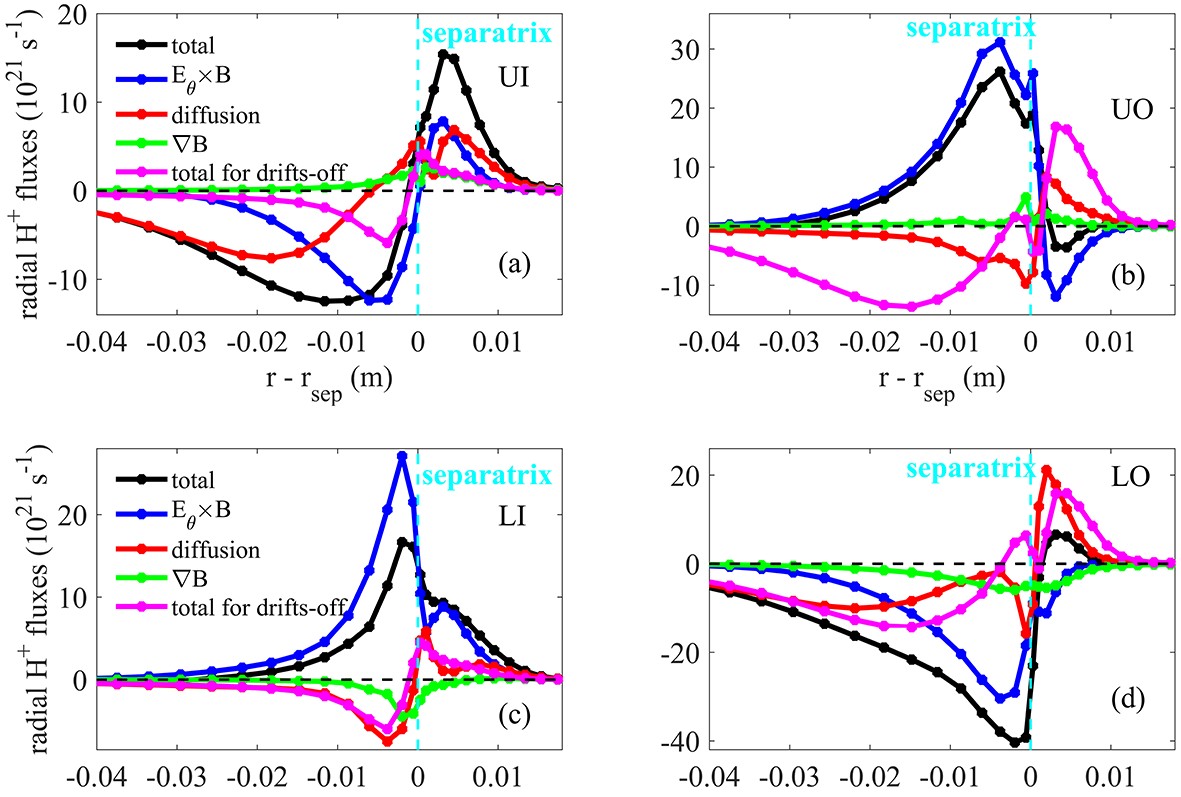
Different components of the radial H⁺ ion flow in the LI, UI, UO and LO divertor regions are poloidally summed over the flux surfaces and shown in figure 14, to separate the role of diffusion, ${\boldsymbol{E}}_{\theta }\times \boldsymbol{B}$ and $\nabla B$ drift in radial particle transport. For a better comparison, the total of surface-integrated radial H⁺ ion flows in different divertor regions for the drift-on and -off cases are also shown in figure 14. The data shown in figure 14 demonstrate that the inclusion of drifts and currents in the simulations will greatly affect the radial particle transport in all the LI, UI, UO and LO divertor regions and that, in the drift-on case, ${\boldsymbol{E}}_{\theta }\times \boldsymbol{B}$ contributes the most to radial H⁺ flows. In addition, from figure 14, ${\boldsymbol{E}}_{\theta }\times \boldsymbol{B}$ drift tends to drive H⁺ ions radially from the lower-outer (upper-inner) divertor region to the lower-inner (upper-outer) one through the PFR (i.e. the region with negative abscissa values in figure 14), just as figure 13 depicts. In the common flux region of LI and UI divertors, ${\boldsymbol{E}}_{\theta }\times \boldsymbol{B}$ drift tends to drive H⁺ ions radially from the region near the separatrix to the far SOL, while in the common flux region of UO and LO divertors, ${\boldsymbol{E}}_{\theta }\times \boldsymbol{B}$ drift tends to drive H⁺ ions radially from the far SOL to the near SOL. That is, the direction of radial ${\boldsymbol{E}}\times \boldsymbol{B}$ drift H⁺ flow in the LI (UO) divertor region is found to be opposite to that in the LO (UI) one. In addition, in the divertor region, the magnitude of the radial H⁺ flux driven by $\nabla B$ drift is found to be much smaller than that by ${\boldsymbol{E}}_{\theta }\times \boldsymbol{B}$ drift and that by diffusion, and thus the $\nabla B$ drift is found to play a less important part in the radial particle transport in the divertor region.

Figure  14.  Different components, including those driven by diffusion, ${\boldsymbol{E}}_{\theta}\times{\boldsymbol{B}}$ and $\nabla B$ drift, together with the total of the radial H⁺ ion flow, poloidally integrated along the flux surfaces in the upper inner (UI) (a), upper outer (UO) (b), lower inner (LI) (c) and lower outer (LO) (d) divertor regions, for the drift-on and drift-off cases. Positive ordinate values in these panels represent that the radial fluxes are directed from the PFR to the common flux region (CFR/SOL), while negative ordinate values represent that the radial fluxes are directed from the CFR (SOL) to the PFR. Positive abscissa values correspond to CFR (SOL), while negative abscissa values correspond to PFR.

DownLoad: Full-Size Img PowerPoint

Figure 15 depicts the radially integrated poloidal H⁺ fluxes ${\mathrm{\Gamma }}_{{\mathrm{H}}^{+}}^{\mathrm{p}\mathrm{o}\mathrm{l}}$ in the lower private flux region (PFRL) and that in the upper PFR (PFRU), together with ${\mathrm{\Gamma }}_{{\mathrm{H}}^{+}}^{\mathrm{p}\mathrm{o}\mathrm{l}}$ in the inner and outer SOL, for the drifts-on case. From figure 15, in most of the PFRL, ${\mathrm{\Gamma }}_{{\mathrm{H}}^{+}}^{\mathrm{p}\mathrm{o}\mathrm{l}}$ is (negative) directed towards the lower inner divertor target (LIT), while in most of the PFRU, ${\mathrm{\Gamma }}_{{\mathrm{H}}^{+}}^{\mathrm{p}\mathrm{o}\mathrm{l}}$ is (positive) directed towards the upper outer divertor target (UOT). As for ${\mathrm{\Gamma }}_{{\mathrm{H}}^{+}}^{\mathrm{p}\mathrm{o}\mathrm{l}}$ in the SOL, in most of the inner SOL, ${\mathrm{\Gamma }}_{{\mathrm{H}}^{+}}^{\mathrm{p}\mathrm{o}\mathrm{l}}$ is (positive) directed towards the upper inner target (UIT), while in most of the outer SOL, ${\mathrm{\Gamma }}_{{\mathrm{H}}^{+}}^{\mathrm{p}\mathrm{o}\mathrm{l}}$ is (negative) directed towards the lower outer divertor target (LOT). Consequently, the in–out asymmetry caused by ${\boldsymbol{E}}\times \boldsymbol{B}$ drift-driven radial/poloidal particle flux through the PFR can be decreased by the ${\boldsymbol{E}}\times \boldsymbol{B}$ drift-driven poloidal flow through the SOL. In addition, ${\mathrm{\Gamma }}_{{\mathrm{H}}^{+}}^{\mathrm{p}\mathrm{o}\mathrm{l}}$ in the PFRL is found to be larger than that in the PFRU. As shown in [39], the divertor asymmetry caused by classical drifts may become invisible as the degree of detachment increases, but the drift effects on divertor detachment are non-ignorable. Hence, to highlight the effects of drifts on the target T_e and q_t, a simulation case is selected, in which the divertor plasma is only partially detached in the region near the strike point, while it is attached in most of the rest of the divertor region, and the corresponding target profiles are shown in figures 16–18.

Figure  15.  (a) Radially integrated poloidal H⁺ fluxes through the lower private flux region (PFRL) and the upper private flux region (PFRU), and (b) radially integrated poloidal H⁺ fluxes through the inner SOL and outer SOL, for the drift-on simulation case. LIT, UIT, UOT and LOT represent the lower inner, upper inner, upper outer and lower outer target plates, respectively. The positive values in these panels represent that the poloidal H⁺ fluxes are in the clockwise direction, while the negative values represent that the poloidal H⁺ fluxes are in the anti-clockwise direction.

DownLoad: Full-Size Img PowerPoint

Figure  17.  T_e profiles along the LO, UI, UO and LO divertor target plates for the drift-on and -off cases.

DownLoad: Full-Size Img PowerPoint

Figure 16 compares the n_e target profiles for the drift-off and drift-on cases. As described above, although plasma ions can be moved from the LO divertor region to the LI one by ${\boldsymbol{E}}\times \boldsymbol{B}$ drift-driven flow through the PFR, a large part of the plasma ions in the LI divertor region can be moved towards the UI one by the poloidal ${\boldsymbol{E}}\times \boldsymbol{B}$-drift through the SOL. Consequently, there is not a huge increase of n_e at the LIT in the drift-on case compared with that in the drift-off case. Similarly, the combined effects of drift-driven plasma flow through the PFRU and that through the inner SOL lead to a small decrease of n_e at the UIT in the drift-on case compared with that in the drift-off case. Drift effects on n_e at the LOT and UOT seem to be larger than those at the LIT and UIT.

Figure  16.  Plasma density profiles along the LI, UI, UO and LO divertor target plates for the drift-on and -off cases.

DownLoad: Full-Size Img PowerPoint

From figure 17, which shows the T_e target profiles for the drift-on and -off cases, the T_e values at the divertor targets can also be greatly affected by drifts. At the LIT and UOT, drifts will lead to the decrease of T_e, especially in the near SOL, while at the UIT and LOT, drift effects will lead to an increase of T_e in the near SOL. In the PFR and far SOL, the influence of drifts on the T_e target value is almost negligible. It is notable that, due to the drift effects, the plasma temperature T_e at the LO target plate can increase to higher than 20 eV, which significantly exceeds the design goal. Hence, in the future optimization of divertors in EHL-2, drift effects will be further focused on.

Figure  18.  Heat flux profiles along the LI, UI, UO and LO divertor target plates for the drift-on and -off cases.

DownLoad: Full-Size Img PowerPoint

Due to the drift effects on particle and heat transport in the divertor region and/or SOL, both the deposition pattern and peak values of divertor heat fluxes can be affected by drifts, as shown in figure 18. From figure 18, drifts can decrease the peak value of heat flux (q_t) on the LIT, UIT and UOT but increase the peak heat flux at the LOT. Hence, drifts may pose a great challenge on the lifetime of LOT in the future high-power operation of EHL-2. In both the drift-on and -off cases, the peak values of T_e and q_t at the LO and UO target plates are found to be larger than those at the LI and UI target plates.

To investigate the influence of drifts on plasma detachment in the LO and UO divertor region, the gas puffing rate has been scanned in both the drift-on and -off cases, and the peak values of T_e and q_t at the target plates are shown in figure 19. From figure 19, in the drift-off cases, with the boron (B) seeding rate increased to be 5×10²¹ s⁻¹, the peak value of T_e at the UO target plate and that at the LO target plate can be decreased to below 5 eV and then these two divertors enter into the detached operation regime. Meanwhile, in the drift-on case, to make both the UO and LO target plates detach from the divertor plasma, the boron seeding rate should be increased to as high as 1.0×10²² s⁻¹. That is, the drift effects may make the LO divertor much more difficult to become detached in the future high ion temperature operation mode.

Figure  19.  The peak values of T_e ((a) and (c)) and q_t ((b) and (d)) at the LO ((c) and (d)) and UO ((a) and (b)) target plates for the drift-on and -off cases plotted against the boron seeding rate.

DownLoad: Full-Size Img PowerPoint

4.3   Impact of gas puff location

To investigate the impact of the gas puffing location on target T_e and q_t, simulations with the gas puffing slots located at both the upper and lower domes (up–down symmetrically distributed) and simulations with gas puffing from a wall segment at the OMP have been performed. Figure 20 compares the T_e and q_t target profiles for the simulation case with gas puffing from the domes against those for the simulation case with gas puffing from upstream. From figure 20, the values of T_e and q_t at the inner target plate for the simulation case with gas puffing from the domes are lower than those for the simulation case with gas puffing from the upstream, but, with gas puffing from the dome, T_e in the far SOL of the outer divertor region is much more difficult to be decreased. To avoid a serious erosion of components in the far SOL of the LO divertor, the puffing slot at the dome should be located much closer to the outer divertor region than to the inner one. With gas puffing from the upstream, the plasma in the outer divertor region can be effectively cooled, promoting the detachment of plasma here. However, the plasma density in the core, which should be at the lowest possible level to achieve the highest possible p-¹¹B fusion reaction rate, can be more easily increased in the simulation case with gas puffing from the upstream (figure 21). Hence, from this point of view, puffing fuel and/or impurity gas from the upstream should be carefully used in the future high ion temperature operation mode of EHL-2.

Figure  20.  Target profiles of q_t ((a) and (b)) and T_e ((c) and (d)) for the simulation case with gas puffing from dome and for the simulation case from upstream.

DownLoad: Full-Size Img PowerPoint

Figure  21.  Radial profiles of n_e and T_e at the OMP for the simulation case with gases puffing from the domes and those for the simulation case with gases puffing from the upstream.

DownLoad: Full-Size Img PowerPoint

5.   Conclusions

The design of the divertor target for the EHL-2 device aimed to address the challenges of power exhaust in proton–boron plasmas. The design work includes a preliminary evaluation of heat load distribution on PFCs by particle tracking strategies and a more detailed divertor physics design via SOLPS-ITER code focusing on the onset and operation of semi-detached/detached regimes. The finally designed divertor target features a biased configuration and is compatible with various magnetic equilibria. The configuration of the baffles was optimized using a particle diffusion model to simulate the heat load distribution on different PFCs to make sure that both the engineering constraints and physical requirements were met. The results from SOLPS-ITER simulations demonstrated that the designed divertor target could effectively handle the power heat load and particle recycling in the divertor region, and thus this divertor is suitable for use in future high-power plasma operations of the EHL-2 device.

SOLPS-ITER calculations, self-consistently taking into consideration the ${\boldsymbol{E}}\times \boldsymbol{B}$ drift, diamagnetic drift, viscosity currents and electric potential, have been performed to address the drift effects on particle and heat transport in the divertor/SOL of EHL-2 as well as on the divertor heat load and detachment. The results demonstrated that the drifts can greatly impact both the radial and poloidal particle/heat transport in the divertor/SOL region, and thus affect the in–out/up–down asymmetry in the target plasma profiles. Particularly, the ${\boldsymbol{E}}\times \boldsymbol{B}$ drift rather than diffusion plays a dominant role in the divertor radial particle transport. The target n_e, T_e and q_t profiles are determined by drift-driven plasma flows through both the PFR and SOL. With drifts switched on, the peak value of T_e at the LI/UO target plate decreases, while that at the UI/LO target plate increases. For both the drift-on and -off cases, the LO and UO divertor regions are found to be hotter than the LI and UI ones. Drifts will lead to a decrease in peak heat flux (q_t,peak) at the LI, UI and UO target plates, but an increase of q_t,peak at the LO one, making the plasma in the LO divertor region much more difficult to detach from the target plate than plasma in other divertor regions. As the divertor plasma enters into the detached operation regime, the drift effects become less remarkable.

In addition, the SOLPS-ITER simulations also show that the gas puffing location can also greatly affect the divertor/SOL plasma behaviour in EHL-2. When the fuel (and/or) impurity gases are puffed from the dome, to effectively decrease the divertor heat load at the LFS, the puffing slot should be located much closer to the outer divertor region than the inner one. Puffing from the upstream leads to an easy increase in upstream plasma density, and thus should be carefully used in the high ion temperature operation mode in future.

Presently, resonant magnetic perturbation (RMP) coils are also being designed and considered for use in EHL-2 for the alleviation/suppression of ELMs. The impact of RMPs on the divertor particle/heat flux pattern will be addressed in the further optimization of the divertor geometry to promote the controllability of RMP-affected divertor heat load. Moreover, for the divertor and equilibrium presented in this paper, the secondary X-point of the XPT divertor configuration is located outside the divertor region. Calculations for configurations where the secondary X-point is inside the divertor region will also be conducted in the future.