| Citation: | Bo ZHU, Ximu HAN, He SU, Xiangjie MA, Guoyan WU. Study on discharge characteristics of low-temperature sub-atmospheric pressure under steep change rate voltage[J]. Plasma Science and Technology, 2025, 27(1): 015401. DOI: 10.1088/2058-6272/ad85bc |
Aiming at the gas discharge problem in electric aircraft, this work studies the gas discharge characteristics at low-temperature sub-atmospheric pressure. A gas discharge shooting platform was built, and the discharge process was photographed by intensified charge-coupled device (ICCD). A two-dimensional axisymmetric model of needle-plate electrode gas discharge was established, and three sets of Helmholtz equations were used to solve the photoionization. The results show that under the same voltage, the electric field intensity in the discharge process increases first, then decreases and finally increases again. The discharge speed increases with the increase of altitude, and the electron density in the streamer decreases with the increase of altitude. The development speed of the streamer in the middle stage is higher than that in the early stage, and the speed increases more obviously with the increase of altitude. The development speed of the streamer in the later stage is lower than that in the middle stage, but with the increase of altitude, the development speed of the streamer in the later stage is higher than that in the middle stage.
With the emphasis on energy conservation and emission reduction, electric aircraft have become an important development trend in the aviation industry. However, electric aircraft require higher power density and voltage than traditional aircraft. Therefore, electric aircraft face more severe insulation problems, and it is of great significance to study the discharge characteristics of electric aircraft in working environments [1]. China’s medium-sized civil aviation aircraft operate above the normal operation altitude of 7000–11000 m. At this height, the working pressure and temperature are in the ranges of 170–308 Torr and 222–246 K, respectively, which belongs to the category of low-temperature sub-atmospheric pressure, and the insulation medium is air [2, 3]. A non-uniform electric field exists inside the electric aircraft, and the needle-plate electrode is a typical non-uniform electric field distribution. The needle-plate streamer discharge is also a typical air discharge form. Therefore, this work studies needle-plate discharge under low-temperature, sub-atmospheric pressure.
For the study of gas discharge characteristics, the macroscopic characteristics such as the shape and trend of gas discharge can be studied experimentally. Zhao et al [4] measured and studied the time-varying electric field of atmospheric pressure pulsed streamer discharge, and used ICCD to observe whether there was an obvious luminescence phenomenon so as to determine the repeatability of time and space of a single streamer channel. Hao et al [5] studied the conversion of pattern discharge and glow discharge in atmospheric pressure helium, and used ICCD to photograph the discharge images on the side and bottom of the electrode, thus discovering the evolution process of discharge. Nijdam et al [6] studied the influence of the ratio of oxygen and nitrogen on the photoionization parameters. An ICCD was used to capture the image of the streamer propagation to measure the propagation velocity of the streamer, so as to study the discharge of different oxygen and nitrogen ratios. Chen et al [7] studied the diameter of atmospheric streamer discharge under lightning impulse voltage, and observed the change of streamer diameter with time through four consecutive single-pulse streamer discharge photos taken by ICCD.
With the development of computer technology and the progress of mathematical algorithms, gas discharge can not only be studied through experiments, but also simulated to obtain the microscopic parameters of discharge and analyze the microscopic mechanism of discharge. Researchers have explored the numerical algorithm of gas discharge in depth. Photoionization plays an important role in the development of gas discharge, and can be calculated using the photoionization integral model proposed by Zheleznyak et al [8]. This model can accurately calculate the photoionization rate, but the integral model has the disadvantages of a large amount of calculation and a long calculation cycle. In order to improve the computational efficiency, Bourdon et al [9] transformed the integral model of photoionization into a set of Helmholtz differential equations, which greatly improved the speed of photoionization calculations. Cai et al [10] proposed three sets of Helmholtz differential equations and verified the accuracy of the model by comparing it with the Zheleznyak integral model. Therefore, the three sets of Helmholtz differential equations not only ensure the accuracy of the calculation, but also improve the computational efficiency. In this study, three sets of Helmholtz differential equations are used to calculate the photoionization.
An accurate simulation method is the basis of microscopic analysis of gas discharge. Herrmann et al [11] introduced the development and application of a two-dimensional fluid model, simulated the discharge triggered by pulsed nanosecond high voltage at the air–water interface, and studied the propagation mechanism of streamer discharge. Vass et al [12] simulated the density distribution of atomic oxygen in a radio frequency plasma jet in a He/O2 mixture using a fluid-dynamics simulation method. Simonchik et al [13] used COMSOL simulation to study the effect of cathode surface temperature on the parameters of the cathode falling layer. Niu et al [14] and Ai et al [15] used finite element simulation software to simulate the positive corona discharge characteristics of SF6 gas and air, respectively. In order to study the development process of partial discharge under high-frequency stress, Wang et al [16] established a two-dimensional plasma simulation model of partial discharge of needle-plate electrode structure, and obtained the spatial and temporal distribution characteristics of microscopic parameters such as electron density, electron temperature, and surface charge. Zhao et al [17] studied the discharge characteristics of square wave voltage under plate-to-plate electrodes. A two-dimensional simulation model was established, and the distribution characteristics of electron and positive ion density under square wave voltage were obtained. You et al [18] established a two-dimensional plasma model to simulate the breakdown voltage of helium in a uniform field. Based on the fluid dynamics model, Leng et al [19] conducted numerical simulation calculations on discharge models at different altitudes. The variation of physical quantities such as current waveform, electron density, and average electron energy with altitude is studied.
At present, most of the research on gas discharge is carried out at normal temperature and pressure, but the research on low-temperature sub-atmospheric pressure is relatively lacking. The applied voltage is mostly DC and AC voltage, and the discharge phenomenon under steep change rate voltage is less studied. In this study, a low-temperature sub-atmospheric air discharge experimental platform is built, and the discharge image is taken by ICCD. The discharge characteristics of the air gap with a needle-plate gap distance of 1 cm are studied by COMSOL simulation software. A two-dimensional axisymmetric model of gas discharge is established. Three sets of Helmholtz equations are used to solve the photoionization of gas discharge. The electric field intensity, electron density, and streamer development speed of needle-plate electrode discharge under low-temperature sub-atmospheric pressure are analyzed. The discharge characteristics under sub-atmospheric pressure steep change rate voltage at low-temperature are studied through experiment and simulation.
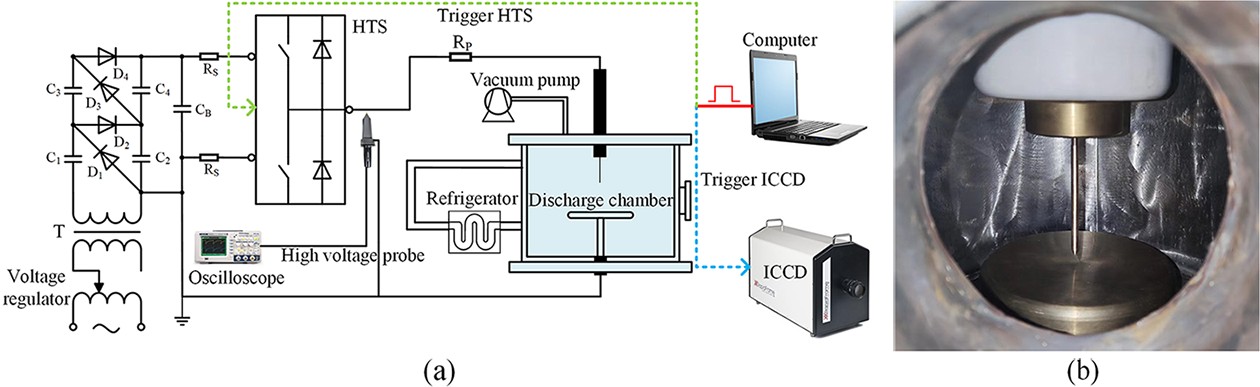
In this paper, the gas discharge shooting platform is shown in figure 1(a). It mainly includes test power supply, fast high voltage transistor switches (HTS), oscilloscope, high voltage probe, vacuum pump, refrigerator, discharge chamber, ICCD, and computer. HTS is a square wave voltage generator switch composed of high voltage transistor switches produced by BEHLKE in Germany. It can be triggered by a pulse signal sent by a computer, and the voltage with a steep change rate can be obtained by adjusting the rising (falling) edge of the output square wave through RS and CB. The discharge chamber adopts a double-layer closed design, and the refrigerator and vacuum pump provide the required temperature and pressure for the discharge. ICCD is the framing camera XXRapidFrame (Stanford Optical Company, USA). The camera has a minimum exposure time of 1.2 ns and a minimum inter-frame time of 0.1 ns. It can capture up to six times of discharge images at one time, and can completely capture the discharge process. Because the light intensity generated by the discharge is strong, a clear image can be captured using the shortest exposure time. Therefore, the exposure time of 1.2 ns is used in this study. The needle-plate electrode discharge chamber used in the experiment is shown in figure 1(b).
In this study, the electron continuity equation and the electron energy density equation are used to describe the behavior of electrons. The equation is:
| \frac{\partial n_{\text{e}}}{\partial t}+\nabla\cdot\boldsymbol{\Gamma}_{\text{e}}=R_{\text{e}}, | (1) |
| \boldsymbol{\Gamma}_{\text{e}}=-(\mu_{\text{e}}\cdot\boldsymbol{E})n_{\text{e}}-D_{\text{e}}\cdot\nabla n_{\text{e}}, | (2) |
| \frac{\partial n_{\varepsilon}}{\partial t}+\nabla\cdot\mathbf{\Gamma}_{\varepsilon}+\boldsymbol{E}\cdot\mathbf{\Gamma}_{\text{e}}=S_{\text{en}}, | (3) |
| \boldsymbol{\Gamma}_{\varepsilon}=-(\mu_{\varepsilon}\cdot\boldsymbol{E})n_{\varepsilon}-D_{\varepsilon}\cdot\nabla n_{\varepsilon}, | (4) |
| D_{\text{e}}=\mu_{\text{e}}T_{\text{e}},\ \mu_{\varepsilon}=\frac{5}{3}\mu_{\text{e}},\ D_{\varepsilon}=\mu_{\varepsilon}T_{\text{e}}, | (5) |
where {n_{\text{e}}} is the electron density, m−3; \boldsymbol{\Gamma}_{\text{e}} is the electron flux, m−2·s−1; {R_{\mathrm{e}}} is the electron source term, m−3·s−1, which mainly includes ionization, attachment, excitation, recombination and photoionization reactions; {\mu _{\text{e}}} is the electron mobility, m2·V−1·s−1; \boldsymbol{E} is the electric field intensity, V·m−1; {D_{\text{e}}} is the electron diffusion coefficient, m2·s−1; n_{\varepsilon} is the electron energy density, eV·m−3; \boldsymbol{\Gamma}_{\varepsilon} is the electron energy flux, eV·m−2·s−1; {S_{{\text{en}}}} is the energy change caused by inelastic collision, eV·m−3·s−1; \mu_{\varepsilon} is the electron energy mobility, m2·V−1·s−1; D_{\varepsilon} is the electron energy diffusion coefficient, m2·s−1.
For the convenience of analysis, this paper defines the reduced electron mobility, that is, {\mu _{\text{e}}} \cdot N , V−1·m−1·s−1, N is the density of neutral particles in air. At the same time, the reduced electron diffusion coefficient, reduced electron energy mobility, and reduced electron energy diffusion coefficient are defined in units of m−1·s−1, V−1·m−1·s−1, and m−1·s−1, respectively. The relationship between the four transport coefficients can be expressed by the Einstein relationship of equation (5).
The electric field intensity is calculated by Poisson equation:
| \boldsymbol{E}=-\nabla V, | (6) |
| \nabla\cdot(\varepsilon_0\varepsilon_{\text{r}}\boldsymbol{E})=\rho_{\text{q}}, | (7) |
where V is the potential, V, {\varepsilon _0} is the vacuum dielectric constant, {\varepsilon _{\text{r}}} is the relative dielectric constant and {\rho _{\text{q}}} is the space charge density, C·m−3.
For other particles except electrons, the following heavy-matter transport equation is used for calculation:
| \rho\frac{\partial}{\partial t}(w_k)+\rho(\boldsymbol{u}\cdot\nabla)w_k=\nabla\cdot\boldsymbol{j}_k+R_k, | (8) |
| \boldsymbol{j}_k=\rho w_kv_k, | (9) |
where \rho is the mixture density, kg·m−3; {w_k} is the mass fraction of the k-th substance in the whole space; u is the fluid velocity of the material in the vector case, m·s−1; {R_k} is the source term of material k, kg·m−3·s−1; {v_k} is the multi-component diffusion rate of substance k.
The photoionization integral model proposed by Zheleznyak is highly accurate. Zheleznyak believes that photoionization is caused by the absorption of the emitted photons by oxygen molecules when the excited state N2 transitions to the ground state. In space, two micro-elements with a distance of R = \left| {\vec r - \vec r'} \right|, the photons emitted from one micro-element dV1 are absorbed by oxygen molecules, and the photoionization is
| {S_{{\text{ph}}}}(\vec r,t) = \int\limits_{{V_1}} {\frac{{I(\vec r,t)g(R)}}{{4{\text{π}}{R^2}}}} {\text{d}}{V_1} , | (10) |
where I(\vec r,t) is the number of photons released by dV1, which can be expressed as
| I(\vec r,t) = \xi \frac{{{p_{\text{q}}}}}{{p + {p_{\text{q}}}}}\frac{{{v_{\text{u}}}}}{{{v_{\text{i}}}}}{v_{\text{i}}}{n_{\text{e}}}(\vec r,t) , | (11) |
where \xi is the average photoionization efficiency; p and {p_{\text{q}}} are air pressure and quenching pressure, respectively, for air {p_{\text{q}}} = 30 Torr; {v_{\text{u}}} and {v_{\text{i}}} are excitation frequency and ionization frequency, respectively; {n_{\text{e}}}(\vec r,t) is the electron density at time t at the space point \vec r [20].
The absorption function g(R) can be expressed as:
| \frac{{g(R)}}{{{p_{{{\text{o}}_{\text{2}}}}}}} = \frac{{\exp ( - {\chi _{\min }}{p_{{{\text{o}}_{\text{2}}}}}R) - \exp ( - {\chi _{\max }}{p_{{{\text{o}}_{\text{2}}}}}R)}}{{{p_{{{\text{o}}_{\text{2}}}}}R\ln ({\chi _{\max }}/{\chi _{\min }})}} , | (12) |
where {p_{{{\text{o}}_{\text{2}}}}} is the partial pressure of O2; {\chi _{\min }} and {\chi _{\max }} are the minimum and maximum absorption cross sections of O2, respectively.
Although the photoionization integral model proposed by Zheleznyak is more accurate, it is computationally inefficient and takes a lot of computational time. In order to improve the computational efficiency, this study uses the Helmholtz equation to solve the photoionization. The basic idea is to express the absorption function as a series sum, so that the photoionization is transformed into the sum of multiple sets of Helmholtz equations. The equation is expressed as:
| {S_{{\text{ph}}}}(\vec r,t) = \sum\limits_j {S_{{\text{ph}}}^j(\vec r,t)} , | (13) |
| {\nabla ^2}S_{{\text{ph}}}^j(\vec r,t) - {({\lambda _j}{p_{{{\text{o}}_{\text{2}}}}})^2}S_{{\text{ph}}}^j(\vec r,t) = - {A_j}p_{{{\text{o}}_{\text{2}}}}^{\text{2}}{v_{\text{i}}}{n_{\text{e}}}(\vec r,t) , | (14) |
where {\lambda _j} is the oxygen absorption cross section. The parameters {\lambda _j} and {A_j} are solved by the global optimization method, so that the error between the objective function and the absorption function is minimized.
The boundary of the calculation region (except the symmetry axis) satisfies the far-field radiation boundary, that is, the Sommerfeld radiation boundary:
| \frac{\partial S_{\text{ph}}^j(\vec{r},t)}{\partial\boldsymbol{n}}+(\lambda_jp_{\text{o}_{\text{2}}})S_{\text{ph}}^j(\vec{r},t)=0, | (15) |
where \boldsymbol{n} is the unit normal vector.
The calculation result of photoionization is added to equation (1) as a source term to simulate its influence on the discharge process.
In this study, a two-dimensional axisymmetric structure of the needle-plate electrode is established to simulate the discharge phenomenon of the needle-plate gap. The geometric model is shown in figure 2. In order to trigger the streamer discharge faster, the initial electron clusters are set near the electrode. The position of the initial electron cluster has a certain distance from the electrode position to improve the convergence speed [21]. Therefore, the initial electron density is set to:
| \begin{split} &\sum\limits_j {n_j^ - (r,z)} {|_{t = 0}} + {n_{\text{e}}}(r,z){|_{t = 0}} = \sum\limits_i {n_i^ - (r,z)} {|_{t = 0}} \\&\qquad={n_1} + {n_0}\exp \left[ - {\left(\frac{r}{{{\sigma _{{r}}}}}\right)^2} - {\left(\frac{{z - {z_0}}}{{{\sigma _{{z}}}}}\right)^2}\right] , \end{split} | (16) |
where {n_1} = {10^7} cm−3, {n_0} = {10^{14}} cm−3, {\sigma _{{r}}}=0.01 cm, {\sigma _{{z}}}= 0.005 cm, {z_0} = 0.975 cm. {n_{\text{e}}}(r,z), n_i^ + (r,z), n_j^ - (r,z) are electron density, positive ion density, and negative ion density, respectively. {\sigma _{{r}}} is the radial electron cluster size, and {\sigma _{{z}}} is the axial electron cluster size.
An accurate boundary condition is the prerequisite for the correct solution of the gas discharge model. In this study, the zero-charge boundary conditions are used for the open boundary:
| -\boldsymbol{n}\cdot\boldsymbol{D}=0, | (17) |
where \boldsymbol{D} is the electric displacement vector, C·m−2.
In this study, the electronic behaviors such as electron reflection, electron thermal motion, and secondary electron emission on the electrode are considered. The boundary conditions of electron flux and electron energy flux are as follows:
| \boldsymbol{n}\cdot\boldsymbol{\Gamma}_{\text{e}}=\frac{1-r}{1+r}\left(\frac{1}{2}v_{\text{e,th}}n_{\text{e}}+n_{\text{e}}(\mu_{\text{e}}\cdot\boldsymbol{E})\cdot\boldsymbol{n}\right)-\sum_{ }^{ }\gamma_i(\boldsymbol{\Gamma}_i\cdot\boldsymbol{n}), | (18) |
| \boldsymbol{n}\cdot\boldsymbol{\Gamma}_{\varepsilon}=\frac{1-r}{1+r}\left(\frac{5}{6}v_{\text{e,th}}n_{\varepsilon}+n_{\varepsilon}(\mu_{\varepsilon}\cdot\boldsymbol{E})\cdot\boldsymbol{n}\right)-\sum_{ }^{ }\gamma_i\varepsilon_i(\boldsymbol{\Gamma}_i\cdot\boldsymbol{n}), | (19) |
where r is the reflection coefficient, {\gamma _i} is the secondary electron emission coefficient, \sum_{ }^{ }\gamma_i(\boldsymbol{\Gamma}_i\cdot\boldsymbol{n}) is the secondary emission flow term, {\varepsilon _i} is the secondary electron average energy, \sum_{ }^{ }\gamma_i\varepsilon_i(\boldsymbol{\Gamma}_i\cdot\boldsymbol{n}) is the secondary emission energy flow term, and {v_{{\text{e,th}}}} is the thermal velocity of the electron:
| {v_{{\text{e,th}}}} = \sqrt {\left(\frac{{8{k_{\text{B}}}{T_{\text{e}}}}}{{ {\text{π}} {m_{\text{e}}}}}\right)} , | (20) |
where {T_{\text{e}}} is the electron temperature, {m_{\text{e}}} is the electronic quality, and {k_{\text{B}}} is the Boltzmann constant.
For heavy matter and ions, which will interact with the wall for surface reactions and are affected by the electric field, the boundary conditions are:
| \boldsymbol{n}\cdot\boldsymbol{j}_k=M_kc_k\mu_kZ_k(\boldsymbol{n}\cdot\boldsymbol{E})+M_kR_{\text{surf,}k},\ \ Z_k\left(\boldsymbol{n}\cdot\boldsymbol{E}\right) > 0, | (21) |
where {M_k} is the molar mass of substance k, {Z_k} is the molar fraction of substance at the volume level, {c_k} is the concentration of substance k, and {\mu _k} is the average mobility of substance k in the mixture.
Appropriate mesh generation is the basis for reasonable calculation of the model. Mesh generation not only should ensure that the discharge phenomenon can be accurately captured, but also should ensure computational efficiency. Therefore, this study divides the streamer discharge area and non-streamer discharge area differently. In the streamer discharge area, a finer mesh is used to accurately analyze the discharge phenomenon. In the non-streamer discharge area, a thicker mesh is used to ensure that the grid is not too much, leading to an increase in the amount of calculation. The mesh generation is shown in figure 3.
The mesh parameters are shown in table 1, where the unit mass is an important parameter to measure the goodness of the mesh. It can be seen from table 1 that the minimum unit mass of this model is 0.5624 and the average unit mass is 0.8891. In many applications, it is considered that the quality of units with unit mass less than 0.1 is worse.
| Parameter type | Numerical value |
| Unit numbers | 20634 |
| Edge unit numbers | 558 |
| Minimum unit mass | 0.5624 |
| Average unit mass | 0.8891 |
| Unit area ratio | 3.549×10−4 |
| Mesh area (cm2) | 1.807 |
In the process of gas discharge, there will be related chemical reactions which mainly include five types of electron-collision reactions: ionization, elastic collision, excitation, attachment, and interparticle reactions. In this study, 30 reactions are selected to simulate the chemical reaction process of gas discharge, as shown in table 2 [21–23].
| Number | Reaction equation | Reaction rate coefficient |
| 1 | \text{e + }\text{O}_{\text{2}}\to\text{e + e + O + }\text{O}^+ | Collision cross section calculation |
| 2 | {\text{e + }}{{\text{N}}_{\text{2}}} \to {\text{e + }}{{\text{N}}_{\text{2}}} | Collision cross section calculation |
| 3 | {\text{e + }}{{\text{N}}_{\text{2}}} \to {\text{e + e + }}{\text{N}}_{\text{2}}^{\text{+ }} | Collision cross section calculation |
| 4 | {\text{e + }}{{\text{O}}_{\text{2}}} \to {{\text{O}}^{{ - }}}{\text{ + O}} | Collision cross section calculation |
| 5 | {\text{e + }}{{\text{O}}_{\text{2}}} \to {\text{e + }}{{\text{O}}_{\text{2}}} | Collision cross section calculation |
| 6 | {\text{e + }}{{\text{O}}_{\text{2}}} \to {\text{e + Os + Os}} | Collision cross section calculation |
| 7 | {\text{e + }}{{\text{O}}_{\text{2}}} \to {\text{e + e + }}{\text{O}}_{\text{2}}^{\text{+ }} | Collision cross section calculation |
| 8 | {\text{e + }}{{\text{N}}_{\text{2}}} \to {\text{e + }}{{\text{N}}_{\text{2}}}{\text{s}} | Collision cross section calculation |
| 9 | {\text{e + }}{\text{O}}_{\text{2}}^{\text{+ }} \to {\text{O + O}} | 2×10−13(300/Te) |
| 10 | {\text{e + }}{\text{N}}_{\text{2}}^{\text{+ }} \to {\text{N + N}} | 2.8×10−13(300/Te)0.5 |
| 11 | {\text{O}}_{\text{2}}^{{- }}{\text{ + }}{\text{O}}_{\text{2}}^{\text{+ }} \to {\text{2}}{{\text{O}}_{\text{2}}} | 2×10−37 |
| 12 | {{\text{O}}_{\text{2}}}{\text{ + }}{\text{N}}_{\text{2}}^{\text{+ }} \to {\text{O}}_{\text{2}}^{\text{+ }}{\text{ + }}{{\text{N}}_{\text{2}}} | 6×10−17 |
| 13 | {\text{O}}_{\text{2}}^{{ - }}{\text{ + }}{\text{O}}_{\text{2}}^{\text{+ }}{\text{ + }}{{\text{N}}_{\text{2}}} \to {\text{2}}{{\text{O}}_{\text{2}}}{\text{ + }}{{\text{N}}_{\text{2}}} | 2.4×10−42 |
| 14 | {\text{e + 2}}{{\text{O}}_{\text{2}}} \to {\text{O}}_{\text{2}}^{{ - }}{\text{ + }}{{\text{O}}_{\text{2}}} | 2×10−41(300/Te) |
| 15 | {\text{O + }}{\text{O}}_{\text{2}}^{\text{+ }} \to {{\text{O}}^{\text{ + }}}{\text{ + }}{{\text{O}}_{\text{2}}} | 2×10−37 |
| 16 | \text{N}^{\text{+}}\text{ + N + }\text{N}_{\text{2}}\to\text{N}_{\text{2}}^{\text{+ }}\text{ + }\text{N}_{\text{2}} | 5×10−41 |
| 17 | {\text{O}}_{\text{2}}^{\text{+ }}{\text{ + }}{{\text{O}}_{\text{2}}}{\text{ + }}{{\text{O}}_{\text{2}}} \to {\text{O}}_{\text{4}}^{\text{+ }}{\text{ + }}{{\text{O}}_{\text{2}}} | 2.4×10−42 |
| 18 | {\text{O}}_{\text{2}}^{\text{+ }}{\text{ + }}{{\text{O}}_{\text{2}}}{\text{ + }}{{\text{N}}_{\text{2}}} \to {\text{O}}_{\text{4}}^{\text{+ }}{\text{ + }}{{\text{N}}_{\text{2}}} | 2.4×10−42 |
| 19 | {\text{N}}_{\text{4}}^{\text{+ }}{\text{ + }}{{\text{O}}_{\text{2}}} \to {\text{O}}_{\text{2}}^{\text{+ }}{\text{ + 2}}{{\text{N}}_{\text{2}}} | 2.5×10−16 |
| 20 | {\text{N}}_{\text{2}}^{\text{+ }}{\text{ + }}{{\text{O}}_{\text{2}}} \to {\text{O}}_{\text{2}}^{\text{+ }}{\text{ + }}{{\text{N}}_{\text{2}}} | 6×10−17 |
| 21 | {\text{N}}_{\text{2}}^{\text{+ }}{\text{ + }}{{\text{O}}_{\text{2}}}{\text{ + }}{{\text{N}}_{\text{2}}} \to {{\text{O}}_{\text{2}}}{\text{ + }}{\text{N}}_{\text{4}}^{\text{+ }} | 5×10−41 |
| 22 | {\text{N}}_{\text{2}}^{\text{+ }}{\text{ + }}{{\text{N}}_{\text{2}}}{\text{ + }}{{\text{N}}_{\text{2}}} \to {{\text{N}}_{\text{2}}}{\text{ + }}{\text{N}}_{\text{4}}^{\text{+ }} | 5×10−41 |
| 23 | {\text{e + }}{{\text{N}}_{\text{2}}}{\text{ + }}{\text{N}}_{\text{2}}^{\text{+ }} \to {\text{2}}{{\text{N}}_{\text{2}}} | 6.07×10−34 |
| 24 | {\text{2e + }}{\text{N}}_{\text{2}}^{\text{+ }} \to {\text{e + }}{{\text{N}}_{\text{2}}} | 2.5×10−46Te0.8 |
| 25 | {{\text{O}}^{{ - }}}{\text{ + O}} \to {\text{e + }}{{\text{O}}_{\text{2}}} | 5×10−16 |
| 26 | {\text{e + }}{{\text{O}}_{\text{2}}}{\text{ + O}} \to {\text{O}}_{\text{2}}^{{ - }}{\text{ + O}} | 1×10−37 |
| 27 | {\text{O}}_{\text{2}}^{{ - }}{\text{ + O}} \to {{\text{O}}^{{ - }}}{\text{ + }}{{\text{O}}_{\text{2}}} | 3.3×10−16 |
| 28 | {\text{O}}_{\text{2}}^{{ - }}{\text{ + }}{\text{N}}_{\text{2}}^{\text{+ }} \to {{\text{N}}_{\text{2}}}{\text{ + }}{{\text{O}}_{\text{2}}} | 1.6×10−13 |
| 29 | {{\text{O}}^{{ - }}}{\text{ + }}{\text{O}}_{\text{2}}^{\text{+ }} \to {\text{O + O + O}} | 1×10−13 |
| 30 | {{\text{O}}^{{ - }}}{\text{ + }}{\text{O}}_{\text{4}}^{\text{+ }} \to {\text{O + }}{{\text{O}}_{\text{2}}}{\text{ + }}{{\text{O}}_{\text{2}}} | 1×10−13 |
{\text{Os}} and \text{N}_{\text{2}}\mathrm{s}\mathrm{ } in table 2 represent the excited states of {\text{O}} and {{\text{N}}_{\text{2}}}, respectively. Reactions 1–8 in table 2 are electron-collision reactions, and their cross-sectional data were imported into COMSOL so that the reaction rates could be calculated. At the same time, the transport parameters related to the model can also be calculated from the cross-sectional data using the BOLSIG+ software. Reactions 9–30 are interparticle reactions, and the reaction rates are given in table 2, including two- and three-body reactions in m3·s−1 and m6·s−1, respectively. Ions and metastable particles impacting the wall will undergo surface reactions and become stable neutral particles. The surface reactions considered in this study are {\text{N}}_2^ + \to {{\text{N}}_2} , {\text{O}} \to 0.5{{\text{O}}_2} , {\text{O}}_2^ + \to {{\text{O}}_2} , {\text{O}}_2^ - \to {{\text{O}}_2} , {{\text{O}}^ - } \to 0.5{{\text{O}}_2} , {{\text{O}}^ + } \to 0.5{{\text{O}}_2} , {\text{N}}_4^ + \to 2{{\text{N}}_2} , and {\text{O}}_4^ + \to 2{{\text{O}}_2} .
Temperature and air pressure are two important factors affecting discharge. Aircraft fly at different altitudes, corresponding to different temperatures and air pressures. Therefore, this work studies the gas discharge phenomenon at altitudes of 7000–11000 m, as shown in table 3. A voltage V with a steep change rate is applied to the needle electrode, and the time to reach the peak voltage is 2.5 ns, as shown in figure 4. Figure 4(a) is the applied voltage of the needle electrode during simulation, and figure 4(b) is the applied voltage of the needle electrode measured experimentally. The plate electrode is grounded.
| Altitude (m) | Temperature (K) | Pressure (Torr) |
| 7000 | 246 | 308 |
| 8000 | 240 | 267 |
| 9000 | 234 | 230 |
| 10000 | 228 | 198 |
| 11000 | 222 | 170 |
In this study, the experiment and simulation model are used to explore the needle-plate discharge under steep change rate voltage in a low-temperature sub-atmospheric pressure environment. The same steep change rate voltage is applied, as shown in figure 4. The electric field intensity, electron density, and streamer development speed of needle-plate electrode discharge at low temperature and sub-atmospheric pressure are explored.
After applying the voltage in figure 4(a) to the needle electrode, the electric field intensity distribution during the streamer discharge process at different altitudes is obtained, as shown in figure 5. The five groups of figures in figure 5 are the distributions of electric field intensity at each altitude. The three figures in each group are the distributions of electric field when the electric field intensity is the highest at this altitude, the lowest, and when breakdown occurs, respectively. From figure 5, we can see:
(1) The development of electric field intensity has gone through three stages. In the first stage, the electric field intensity rises to the highest electric field intensity, corresponding to the first figure of each group. In the second stage, the electric field intensity decreases from the highest to the lowest, corresponding to the second figure of each group. In the third stage, the electric field intensity continues to rise until the breakdown occurs, corresponding to the third figure of each group.
Taking the altitude of 7000 m as an example, the three stages of the development of electric field intensity are discussed. The comparison of electron density and electric field intensity at the initial moment and the highest moment of electric field intensity is shown in figure 6. It can be seen from figure 6 that the initial electron cluster has a certain distance from the needle electrode. In the initial stage of discharge development, these electrons first develop to the needle electrode due to the attraction of positive and negative polarity, thus strengthening the electric field intensity between the needle electrode and the initial electron cluster. In the first stage, the strong field region develops downward from the needle electrode with a smaller radius of curvature, and lies between the needle electrode and the initial electron cluster. As the electron moves to the needle electrode and the amplitude of the applied voltage increases, the electric field between the needle electrode and the initial electron cluster also increases. In the second stage, the strong field region continues to develop downward. The development after 1.1 ns is that the strong field region is wrapped outside the streamer. At this time, the strong field region is continuously away from the needle electrode, the high-particle-concentration distribution area of the streamer head increases, and the electric field distortion caused by the streamer head is weakened, so that the value of the electric field intensity is continuously reduced. In the third stage, after the strong field region develops to the plate electrode, the positive ions of the streamer head interact with the ground plate electrode, which strengthens the electric field intensity in the strong field region, and then breakdown occurs.
(2) With the increase of altitude, the time for each group to reach the highest electric field intensity and breakdown gradually decreases. This is because the breakdown voltage decreases with the increase of altitude, and the breakdown time will decrease when the same voltage is applied.
After applying the voltage in figure 4(a) to the needle electrode, the electron density distributions at different altitudes are obtained, as shown in figure 7. The electron density distributions in figure 7 correspond to the electric field intensity distributions in figure 5. In order to better determine the size of the electron density by the color depth of the streamer, the corresponding stages at different altitudes in figure 7 use the same legend.
It is worth noting that when the streamer develops to the position of the first figure at each altitude, the electron density has a sudden drop, as shown in the blank electron density in figure 8, and then develops downward at a higher density. Taking the altitude of 7000 m as an example, the plasmoids set at the initial time shielding the internal electric field, as shown at the time t = 0 ns in figure 6, so that the ionization reaction of electrons here is weakened. Subsequently, it develops downwards at a higher density because the strong field region reaches a maximum after crossing the plasmoids, as shown at the time t = 1.1 ns in figure 6.
By comparing the five groups of pictures in figure 7, it can be found that as the altitude increases, the color of the streamer gradually becomes lighter, that is, the electron density gradually decreases. On one hand, this is because as the altitude increases, the pressure decreases, the formed plasma is thinner, and the electron density is smaller. On the other hand, with the increase of altitude, the breakdown time decreases continuously, and there is not enough time to generate more electrons. In addition, it should be pointed out that this work only studies the discharge phenomenon before breakdown. This period of time is very short, and the streamer has not yet spread to both sides. Therefore, there is no phenomenon whereby the pressure decreases and the plasma volume increases.
After applying the voltage in figure 4(a) to the needle electrode, taking 7000, 9000, and 11000 m as examples, the streamer is divided into three time periods, namely the early, middle, and late stages, to illustrate its development speed law. In order to observe the position of streamer development more clearly, the axial electron density distribution of the three time points selected at each altitude are drawn, as shown in figure 9.
According to the results in figure 9, the streamer development speed and the growth rate or decline rate of the speed in each period are calculated. The calculation results are shown in table 4. It can be seen from table 4:
| Altitude | Early | Middle | Late | ||
| Velocity (106 m·s−1) | 7000 | 0.742 | 1.290 | 1.100 | |
| 9000 | 0.769 | 1.577 | 1.520 | ||
| 11000 | 0.818 | 1.909 | 1.925 | ||
| Velocity variation rate | 7000 | Up 73.9% | Down 14.7% | ||
| 9000 | Up 105.0% | Down 3.6% | |||
| 11000 | Up 133.4% | Down 1.8% | |||
(1) When the altitude is 7000 m, the streamer development speed in the middle stage (3.1–6.2 ns) is significantly higher than that in the early stage (0–3.1 ns), and the streamer development speed in the late stage (6.2–9.2 ns) is lower than that in the middle stage. This is because the strong field region of the early streamer head is small and the region of electron collision ionization is small, so the streamer development speed is relatively slow. In the middle stage of streamer development, the range of the strong field region expands, the impact ionization of electrons intensifies, and the streamer accelerates. In the later stage, the streamer is in a thinner state, and the electron free path increases. Although it is beneficial to the impact ionization of electrons, the applied voltage in the later stage has decreased to a certain extent, so that the electric field intensity in the strong field area has decreased and the range of the strong field area after reaching the plate electrode has narrowed, so the development speed of the streamer in the later stage has decreased.
(2) With the increase of altitude to 7000 and 11000 m, the increase of streamer development speed in the middle stage (2.6–5.2 and 2.2–4.4 ns) is more obvious. On one hand, this is because with the increase of altitude, the pressure decreases, the electron free path increases compared with 7000 m, and the electron accumulates enough energy to impact ionization. On the other hand, the middle stage voltage corresponding to 7000 and 11000 m is at a higher level. In addition, with the increase of altitude to 11000 m, the development speed of the late stage (4.4–6.4 ns) streamer began to appear slightly larger than that of the middle stage streamer development speed. This is because when the altitude is 11000 m, the breakdown time is short and the applied voltage is still at a certain height in the late stage, so the decrease of electric field intensity in the strong field area is limited, the plasma in the late stage is thinner than that in the middle stage, and the electron free path increases, which is conducive to impact ionization, so the streamer development speed is slightly higher than that in the middle stage.
The needle electrode is applied with the same rate of change of voltage. The discharge processes at altitudes of 7000, 9000, and 11000 m are photographed by ICCD. Through a large number of experiments, it is found that the discharge will occur randomly on the left and right sides of the needle electrode, which is caused by the random distribution of particles in the air during discharge. In order to discuss the gas discharge phenomenon more conveniently and accurately, we choose the discharge on the right side of the needle electrode for discussion. Three pictures are selected at each altitude to represent the initiation, development, and end of the discharge process. The discharge process is shown in figure 10. It can be seen from figure 10 that the length of discharge channel is different at different altitudes. This is due to the rough surface of the needle electrode, which easily causes surface discharge. As the altitude increases, the pressure decreases, the electron free path increases, and the gas discharge becomes easier, resulting in surface discharge on the rough electrode surface, and the gas discharge channel becomes longer, which has little effect on the main discharge.
By comparing the three sets of pictures in figure 10, it is found that with the increase of altitude, the time required for the discharge from the beginning to the end gradually decreases, that is, with the increase of altitude, the speed of discharge development becomes faster. This is consistent with the simulation law of figure 5, but the breakdown time obtained by simulation is slightly lower than the breakdown time obtained by experiment. This is because the main reactions in the discharge process are only considered in this study, and many reactions in the actual discharge process are not taken into account, resulting in a slight difference in breakdown time. In addition, by observing the discharge channels at different altitudes, it can be found that with the increase of altitude, the discharge channels gradually darkened, that is, the electron density during the discharge process decreased with the increase of altitude. This is consistent with the variation of electron density with altitude in figure 7.
In this study, a shooting platform for gas discharge is built, and a two-dimensional axisymmetric simulation model is established. The discharge phenomenon of low-temperature sub-atmospheric pressure under steep change rate voltage is explored in both experiment and simulation. Through the analysis of the results, the following conclusions are obtained:
(1) Under the same steep change rate voltage, the maximum electric field intensity of the streamer head increases first, then decreases and finally increases during the discharge process.
(2) The discharge speed increases with the increase of altitude, and the electron density in the streamer decreases with the increase of altitude.
(3) From the beginning of streamer development to the final breakdown, the development speed of the streamer in the middle stage is higher than that in the early stage, and the speed increases more obviously with the increase of altitude. The velocity of the late stage of the streamer is first smaller than that of the middle stage, but with the increase of altitude, the development speed of the late stage of the streamer is higher than that of the middle stage.
| [1] |
Sayed E et al 2021 IEEE Trans. Transp. Electrif. 7 2976 doi: 10.1109/tte.2021.3089605
|
| [2] |
Zhao Z H et al 2022 IEEE Trans. Plasma Sci. 50 2333 doi: 10.1109/tps.2022.3186808
|
| [3] |
Zhao Z H et al 2023 Proc. CSEE 43 4034 doi: 10.13334/j.0258-8013.pcsee.213012
|
| [4] |
Zhao Z et al 2023 Proc. CSEE 44 3347 doi: 10.13334/j.0258-8013.pcsee.223049
|
| [5] |
Hao Y P, Zheng B and Liu Y G 2012 High Voltage Eng. 38 1568 (in Chinese) doi: 10.3969/j.issn.1003-6520.2012.07.005
|
| [6] |
Nijdam S et al 2010 J. Phys. D: Appl. Phys. 43 145204 doi: 10.1088/0022-3727/43/14/145204
|
| [7] |
Chen S, Zeng R and Zhuang C J 2013 J. Phys. D: Appl. Phys. 46 375203 doi: 10.1088/0022-3727/46/37/375203
|
| [8] |
Zheleznyak M B, Mnatsakanyan A K and Sizykh S V 1982 Teplofiz. Vys. Temp. 20 423
|
| [9] |
Bourdon A et al 2007 Plasma Sources Sci. Technol. 16 656 doi: 10.1088/0963-0252/16/3/026
|
| [10] |
Cai X J et al 2015 Proc. CSEE 35 240 doi: 10.13334/j.0258-8013.pcsee.2015.01.029
|
| [11] |
Herrmann A, Margot J and Hamdan A 2024 Plasma Sources Sci. Technol. 33 025022 doi: 10.1088/1361-6595/ad286f
|
| [12] |
Vass M et al 2024 Plasma Sources Sci. Technol. 33 015012 doi: 10.1088/1361-6595/ad1f37
|
| [13] |
Simonchik L et al 2024 Plasma Sources Sci. Technol. 33 025014 doi: 10.1088/1361-6595/ad2580
|
| [14] |
Niu H Q et al 2021 High Voltage Eng. 47 4063 doi: 10.13336/j.1003-6520.hve.20200661
|
| [15] |
Ai J W et al 2021 High Voltage Eng. 47 4377 doi: 10.13336/j.1003-6520.hve.20210060
|
| [16] |
Wang J et al 2022 High Volt. 7 439 doi: 10.1049/hve2.12156
|
| [17] |
Zhao Z H et al 2022 IEEE Trans. Plasma Sci. 50 2786 doi: 10.1109/tps.2022.3188756
|
| [18] |
You Q et al 2020 Ann. Nucl. Energy. 141 107351 doi: 10.1016/j.anucene.2020.107351
|
| [19] |
Leng X J et al 2025 J. At. Mol. Phys. 42 100 doi: 10.19855/j.1000-0364.2025.033001
|
| [20] |
Luque A et al 2007 Appl. Phys. Lett. 90 081501 doi: 10.1063/1.2435934
|
| [21] |
Zhao Z H et al 2021 IEEE Access 9 51896 doi: 10.1109/access.2021.3070335
|
| [22] |
Kossyi I A et al 1992 Plasma Sources Sci. Technol. 1 207 doi: 10.1088/0963-0252/1/3/011
|
| [23] |
Liu L P and Becerra M 2017 J. Phys. D: Appl. Phys. 50 345202 doi: 10.1088/1361-6463/aa7c71
|
| Parameter type | Numerical value |
| Unit numbers | 20634 |
| Edge unit numbers | 558 |
| Minimum unit mass | 0.5624 |
| Average unit mass | 0.8891 |
| Unit area ratio | 3.549×10−4 |
| Mesh area (cm2) | 1.807 |
| Number | Reaction equation | Reaction rate coefficient |
| 1 | \text{e + }\text{O}_{\text{2}}\to\text{e + e + O + }\text{O}^+ | Collision cross section calculation |
| 2 | {\text{e + }}{{\text{N}}_{\text{2}}} \to {\text{e + }}{{\text{N}}_{\text{2}}} | Collision cross section calculation |
| 3 | {\text{e + }}{{\text{N}}_{\text{2}}} \to {\text{e + e + }}{\text{N}}_{\text{2}}^{\text{+ }} | Collision cross section calculation |
| 4 | {\text{e + }}{{\text{O}}_{\text{2}}} \to {{\text{O}}^{{ - }}}{\text{ + O}} | Collision cross section calculation |
| 5 | {\text{e + }}{{\text{O}}_{\text{2}}} \to {\text{e + }}{{\text{O}}_{\text{2}}} | Collision cross section calculation |
| 6 | {\text{e + }}{{\text{O}}_{\text{2}}} \to {\text{e + Os + Os}} | Collision cross section calculation |
| 7 | {\text{e + }}{{\text{O}}_{\text{2}}} \to {\text{e + e + }}{\text{O}}_{\text{2}}^{\text{+ }} | Collision cross section calculation |
| 8 | {\text{e + }}{{\text{N}}_{\text{2}}} \to {\text{e + }}{{\text{N}}_{\text{2}}}{\text{s}} | Collision cross section calculation |
| 9 | {\text{e + }}{\text{O}}_{\text{2}}^{\text{+ }} \to {\text{O + O}} | 2×10−13(300/Te) |
| 10 | {\text{e + }}{\text{N}}_{\text{2}}^{\text{+ }} \to {\text{N + N}} | 2.8×10−13(300/Te)0.5 |
| 11 | {\text{O}}_{\text{2}}^{{- }}{\text{ + }}{\text{O}}_{\text{2}}^{\text{+ }} \to {\text{2}}{{\text{O}}_{\text{2}}} | 2×10−37 |
| 12 | {{\text{O}}_{\text{2}}}{\text{ + }}{\text{N}}_{\text{2}}^{\text{+ }} \to {\text{O}}_{\text{2}}^{\text{+ }}{\text{ + }}{{\text{N}}_{\text{2}}} | 6×10−17 |
| 13 | {\text{O}}_{\text{2}}^{{ - }}{\text{ + }}{\text{O}}_{\text{2}}^{\text{+ }}{\text{ + }}{{\text{N}}_{\text{2}}} \to {\text{2}}{{\text{O}}_{\text{2}}}{\text{ + }}{{\text{N}}_{\text{2}}} | 2.4×10−42 |
| 14 | {\text{e + 2}}{{\text{O}}_{\text{2}}} \to {\text{O}}_{\text{2}}^{{ - }}{\text{ + }}{{\text{O}}_{\text{2}}} | 2×10−41(300/Te) |
| 15 | {\text{O + }}{\text{O}}_{\text{2}}^{\text{+ }} \to {{\text{O}}^{\text{ + }}}{\text{ + }}{{\text{O}}_{\text{2}}} | 2×10−37 |
| 16 | \text{N}^{\text{+}}\text{ + N + }\text{N}_{\text{2}}\to\text{N}_{\text{2}}^{\text{+ }}\text{ + }\text{N}_{\text{2}} | 5×10−41 |
| 17 | {\text{O}}_{\text{2}}^{\text{+ }}{\text{ + }}{{\text{O}}_{\text{2}}}{\text{ + }}{{\text{O}}_{\text{2}}} \to {\text{O}}_{\text{4}}^{\text{+ }}{\text{ + }}{{\text{O}}_{\text{2}}} | 2.4×10−42 |
| 18 | {\text{O}}_{\text{2}}^{\text{+ }}{\text{ + }}{{\text{O}}_{\text{2}}}{\text{ + }}{{\text{N}}_{\text{2}}} \to {\text{O}}_{\text{4}}^{\text{+ }}{\text{ + }}{{\text{N}}_{\text{2}}} | 2.4×10−42 |
| 19 | {\text{N}}_{\text{4}}^{\text{+ }}{\text{ + }}{{\text{O}}_{\text{2}}} \to {\text{O}}_{\text{2}}^{\text{+ }}{\text{ + 2}}{{\text{N}}_{\text{2}}} | 2.5×10−16 |
| 20 | {\text{N}}_{\text{2}}^{\text{+ }}{\text{ + }}{{\text{O}}_{\text{2}}} \to {\text{O}}_{\text{2}}^{\text{+ }}{\text{ + }}{{\text{N}}_{\text{2}}} | 6×10−17 |
| 21 | {\text{N}}_{\text{2}}^{\text{+ }}{\text{ + }}{{\text{O}}_{\text{2}}}{\text{ + }}{{\text{N}}_{\text{2}}} \to {{\text{O}}_{\text{2}}}{\text{ + }}{\text{N}}_{\text{4}}^{\text{+ }} | 5×10−41 |
| 22 | {\text{N}}_{\text{2}}^{\text{+ }}{\text{ + }}{{\text{N}}_{\text{2}}}{\text{ + }}{{\text{N}}_{\text{2}}} \to {{\text{N}}_{\text{2}}}{\text{ + }}{\text{N}}_{\text{4}}^{\text{+ }} | 5×10−41 |
| 23 | {\text{e + }}{{\text{N}}_{\text{2}}}{\text{ + }}{\text{N}}_{\text{2}}^{\text{+ }} \to {\text{2}}{{\text{N}}_{\text{2}}} | 6.07×10−34 |
| 24 | {\text{2e + }}{\text{N}}_{\text{2}}^{\text{+ }} \to {\text{e + }}{{\text{N}}_{\text{2}}} | 2.5×10−46Te0.8 |
| 25 | {{\text{O}}^{{ - }}}{\text{ + O}} \to {\text{e + }}{{\text{O}}_{\text{2}}} | 5×10−16 |
| 26 | {\text{e + }}{{\text{O}}_{\text{2}}}{\text{ + O}} \to {\text{O}}_{\text{2}}^{{ - }}{\text{ + O}} | 1×10−37 |
| 27 | {\text{O}}_{\text{2}}^{{ - }}{\text{ + O}} \to {{\text{O}}^{{ - }}}{\text{ + }}{{\text{O}}_{\text{2}}} | 3.3×10−16 |
| 28 | {\text{O}}_{\text{2}}^{{ - }}{\text{ + }}{\text{N}}_{\text{2}}^{\text{+ }} \to {{\text{N}}_{\text{2}}}{\text{ + }}{{\text{O}}_{\text{2}}} | 1.6×10−13 |
| 29 | {{\text{O}}^{{ - }}}{\text{ + }}{\text{O}}_{\text{2}}^{\text{+ }} \to {\text{O + O + O}} | 1×10−13 |
| 30 | {{\text{O}}^{{ - }}}{\text{ + }}{\text{O}}_{\text{4}}^{\text{+ }} \to {\text{O + }}{{\text{O}}_{\text{2}}}{\text{ + }}{{\text{O}}_{\text{2}}} | 1×10−13 |
| Altitude (m) | Temperature (K) | Pressure (Torr) |
| 7000 | 246 | 308 |
| 8000 | 240 | 267 |
| 9000 | 234 | 230 |
| 10000 | 228 | 198 |
| 11000 | 222 | 170 |
| Altitude | Early | Middle | Late | ||
| Velocity (106 m·s−1) | 7000 | 0.742 | 1.290 | 1.100 | |
| 9000 | 0.769 | 1.577 | 1.520 | ||
| 11000 | 0.818 | 1.909 | 1.925 | ||
| Velocity variation rate | 7000 | Up 73.9% | Down 14.7% | ||
| 9000 | Up 105.0% | Down 3.6% | |||
| 11000 | Up 133.4% | Down 1.8% | |||