Nonlinear saturation of reversed shear Alfvén eigenmode via high-frequency quasi-mode generation

1.   Introduction

Compared to electrons and X/$\gamma$-rays, the energy loss of ionizing radiation of ions has a pronounced Bragg peak at the end of their trajectory [1]. This characteristic of ion beams is conceived to be useful in cancer therapy since it would be much easier to concentrate the dose on the tumor while minimizing the toxicity to the surrounding healthy tissues [2, 3]. Therefore, proton therapy has attracted significant attention in the past decades and has become a commercial approach to treat localized cancers [4]. However, the available ion beam therapy (IBT) centers (only about 100 centers worldwide) are all based on the conventional accelerators which are huge and expensive [5, 6]. This hinders the IBT from being a standard treatment that can be afforded by more cancer patients.

Laser-driven ion acceleration offers an extremely large acceleration gradient, several orders of magnitude higher than that of the conventional radio frequency (RF) accelerators [7‒15]. In principle, protons can be accelerated to 200 MeV within submillimeter long. Therefore, it is a promising approach to significantly reduce the accelerator size and then the cost of the therapy. Recently, laser-based IBT has become a highly-active research field [16‒28]. Several international groups are dedicated to making this approach become feasible. The involved laser facilities include the Gemini laser at Oxford [26], ELIMED of ELI Beamlines at Prague [27], Draco PW laser at Dresden [21], BELLA PW laser at Berkeley [23], and CLAPA laser at Beijing [28]. To realize this, there are several huge challenges to be addressed. The first is how to achieve monoenergetic proton beams with peak energy above 200 MeV. This has been widely discussed in both experiments and theory [10‒12, 15, 29], though it is still very challenging. Several acceleration mechanisms have been proposed and verified in experiments, including the well-known target normal sheath acceleration (TNSA) [30‒32], radiation pressure acceleration (RPA) [33‒37], collisionless shockwave acceleration [38‒40], etc. The second one is how to manipulate the ion beam transport with a compact system. It has not received sufficient attention although it is believed to be equally important for demonstrating the commercial advantage of the laser-based IBT [41].

Most discussions about the design of the beam transport systems (BTSs) rely on conventional magnets. In the known BTSs, usually a set of quadrupole magnets is used to collimate the proton beam [21, 25], while dipole magnets are employed to deflect the proton beam and remove high-energy electrons and other species of ions generated along with the protons [26]. However, to control the high-energy protons up to 200 MeV, the required magnetic field is very strong, and the magnets would be very hefty. Then the required gantry would still be huge and heavy [42], seriously compromising the compactness of the laser-based IBT.

In this paper, we put forward a new BTS design for the laser-driven IBT. It contains a helical coil irradiated by a nanosecond laser pulse, a pair of dipole magnets and apertures, as shown in figure 1. We combine three different codes to simulate the whole interaction process from generation of high-energy protons via laser-plasma interactions (particle-in-cell (PIC) code), creation of strong magnetic field in the coil (finite element analysis) to proton beam transport and deposition (Monte-Carlo (MC) code). We demonstrate the effectiveness of this design and show that it enables the generation of a spread-out Bragg peak (SOBP) within a few centimeters and the dose is approximately 16.5 cGy, while the instantaneous dose rate reaches up to $10^9$ Gy/s. For typical proton radiation therapy (RT) treatment [43, 44], a required laser repetition frequency is only 0.067 Hz.

Figure  1.  Schematic of our BTS design for the laser-driven proton RT. High-energy protons are produced from solid targets irradiated by relativistic laser pulses. The proton transport system consists of a solenoid for collimation, a pair of sector dipole magnets for energy selection, and two apertures. The solenoid is made of a wire bent into a helical coil, with each end connected to a pair of parallel metal disc targets. This capacitor disc target is irradiated by a nanosecond laser pulse to drive large currents and then induce strong magnetic fields inside the solenoid. There are two diagnostic layers along the path of the proton beam, marked by black dashed lines. Finally, the modulated proton beam enters the water phantom to mimic the dose deposition in proton therapy.

DownLoad: Full-Size Img PowerPoint

2.   Results

2.1   Laser-driven proton acceleration

In principle, our design is suitable for different laser-driven proton acceleration mechanisms, such as TNSA, RPA and others. One only needs to adjust the coil and its driven laser parameters to match the properties of the laser-accelerated protons. Here, as an example to elucidate its feasibility, we consider the laser-peeler proton acceleration scheme [45, 46] that we proposed recently, where a linearly-polarized laser is incident on a thin edge of a tape target, much simpler than the schemes [47] proposed earlier so electrons can stay in phase for a longer time and be attracted at the rear edge to higher density. In order to treat the deep-seated tumors, such as lung cancer [48], the required proton energy is above 200 MeV [3] and the optimal energy spectrum is characterized by a small spread ($\sim1\%$) and a sharp distal falloff at the high-energy range [49]. We found that the beam qualities of the protons generated from the peeler scheme are close to these requirements.

We utilize the three-dimensional (3D) PIC code epoch [50] to investigate the laser-plasma interaction and proton acceleration processes. A laser pulse with intensity of $I_{\rm L}=5.35 \times 10^{21}\; {\rm W/cm^2}$ (corresponding to a normalized intensity of $a_{\rm L}=50$), pulse duration of $\rm \tau_L=24\; fs$ (full width at half maximum, FWHM) and focal spot size of $r_{\rm L}=7.5\lambda$ (FWHM) is employed. To reduce the simulation scale, a high-$Z$ tape target (assuming gold) with initial charge state of $\rm Au^{51+}$ with electron density $n_{\rm e}=40n_{\rm c}$ and dimensions of $x \times y \times z={\rm 43.75\lambda \times 0.75\lambda \times 33.75\lambda}$ is utilized. Here $n_{\rm{c}}= 1.1\times10^{21}\; \left(\lambda/\mathrm{\mu m}\right)^2\; \rm{c}\mathrm{m}^{-3}$ is the critical density. Protons come from a hydrocarbon (CH) layer attached behind the tape target with a thickness of $0.75\lambda$. Justifications of choosing these parameters and detailed acceleration processes have been discussed in our recent work [49]. Since here we focus on the beam transport system, we only briefly show the key results of the laser-driven proton acceleration in figure 2.

Figure  2.  3D PIC simulation results. (a) Spatial distribution of the final proton energy, where the target is represented by the gray cuboid. (b) The final energy spectrum at $t=90T_0$.

DownLoad: Full-Size Img PowerPoint

Because of the generation of high-flux collimated superponderomotive energy electrons driven by the surface plasma wave, a longitudinal bunching and transverse focusing field is self-established at the rear edge, leading to the generation of high-energy monoenergetic proton beams [45]. The proton charge produced in a single shot is about 1 nC (only protons accelerated away from the target are considered here). Figure 2(a) shows the distribution of the proton energy in 3D. One can see that the highest energy protons are confined in the center with smaller divergences. The final proton energy spectrum is displayed in figure 2(b). The peak energy is about 200 MeV and energy spread is about $3.9\%$ with 2.1$\times10^9$ protons (corresponding to 0.34 nC) contained in the FWHM of the peak energy. To meet the required dose of proton radiotherapy in a treatment process, multiple proton beams should be delivered within 1–3 min so the scheme has to be feasible to be operated at a repetition-rate mode. To realize this, one can employ a conveyor belt design where the tape targets are conveyed to the laser focused point at a uniform speed (synchronized with the laser repetition rate) [45].

2.2   Estimation of the generated magnetic field

To reduce the particle loss and focus protons, a unit containing strong magnetic fields is usually necessary. In reference [21], a pulsed two-solenoid beamline is mounted. Here to minimize the transport system size, we utilize a laser-driven pulsed magnet, i.e., a helical coil irradiated by a laser pulse [51]. The helical coil is only about millimeter long with each end connected to a pair of parallel solid metal disc targets. A laser pulse ejects electrons from the laser-irradiated region, while the ejected electrons can be caught by the other disc, leading to a voltage $U$ between the two disc targets. This potential would cause electrons flowing through the coil, and then induce a loop that generates a magnetic field inside the solenoid region. Depending on the material and size of the coil $R=\rho l/a$, the current, $I=U/R$, varies from kA to tens of kA or even higher. Here $R$ is the total resistance of the solenoid wire, and $\rho$ is the resistivity of the wire material, $l$ is the length of the wire, and $a$ is the cross section. Since here our primary goal is to demonstrate the principle of this BTS, for simplicity, we assume a current of 5.3 kA. The current value is related to both the total escape charge $Q$ caused by laser-target interaction and the solenoid parameters (such as total resistance and inductance). Among them, the escape charge $Q$ is primarily determined by laser parameters. According to the model presented in references [52] and [53], it allows us to estimate the charging current and the total charge $Q$ produced on the target by the escaped hot electrons during the high-power laser-target interaction. For a cuurent of 5.3 kA, the required total escape charge $Q$ is approximately several nC. These can be achieved by using a laser pulse with an intensity of $\rm \sim 10^{15}\; W/cm^2$ and a pulse duration of several nanoseconds.

Then we use the multi-physics simulation software COMSOL [54] to calculate the distribution of the magnetic field inside the solenoid. The coil is made of a silver wire with a diameter of $\rm 100\; \mu m$. The sizes of the internal diameter, pitch, and length of the coil are 2.0, 0.2, and 10.0 mm, respectively. The simulated 3D distribution of the magnetic field is illustrated in figure 3(a). The magnetic field is almost uniform in the center (figures 3(b) and (c)) with a strength of about 30 T.

Figure  3.  Estimation of the magnetic field distribution with the comsol code. (a) The 3D spatial distribution of the magnetic field generated by the solenoid, (b) and (c) the lineouts of the magnetic field along $x$ and $z$ dimensions, respectively.

DownLoad: Full-Size Img PowerPoint

For 200 MeV protons, it takes about $\tau_{\rm p} \simeq 50$ ps for them to pass through the solenoid. We assume that the magnetic field distribution inside the solenoid remains constant within $\tau_{\rm p}$. In GEANT4 [55], the distribution of the magnetic field is initialized via the G4MagneticField module as one of the initial simulation conditions.

2.3   Proton beam transport and dose delivery

To simulate the proton transport and dose deposition processes, we further utilize the MC code geant4 [55] and take the data of the proton momenta ($p_x$, $p_y$, $p_z$) obtained from PIC simulations as the initial input while assuming it is a point source. The BTS consists of a solenoid, a pair of sector bipolar magnets, and two apertures, as illustrated in figure 1. Here the physical list QGSP$\_$BIC is used, and the inelastic scattering and nuclear reaction are self-consistently considered.

The first aperture made of lead is 5 cm thick with a 6 mm inner diameter, ensuring that only protons near the axis can pass through. Behind this aperture is a pair of sector dipole magnets with opposite magnetic field directions to deflect the proton beam, i.e., the first one with magnetic field pointing in the $-z$ direction while the second in $z$ direction. The sector magnets have a radius of $R_{\rm s}=10\; {\rm cm}$, a central angle of $\theta_{\rm s}=45 {\text{°}}$, and a magnetic field strength of $B=10\; {\rm T}$. Protons of different energies have different deflection radii ($r=\gamma_{\rm p} m_{\rm p} v_{\rm p}/eB$) and will be deflected to different positions in $y$ direction. Here $m_{\rm p}$, $v_{\rm p}$ and $\gamma_{\rm p}$ are the proton mass, velocity and relativistic Lorentz factor, respectively, and $e$ is the electron charge. By placing another lead aperture with thickness of 5 cm and 20 mm inner diameter at the exit, large-divergence or low-energy protons ($\rm <170\; MeV$) will be blocked. Then, the selected protons will fly freely until entering the water.

After passing through the beam transport devices, most forward high-energy electrons and X-rays, generated alongside the quasi-monoenergetic proton beam, are deflected or shielded. Besides, one of the advantages using the dipole magnets is that the protons no longer move in the same direction as the electrons and X-rays. Only the remaining deflected high-quality collimated proton beam is delivered into the RT chamber. Due to the similarities between human tissues and water in terms of dose deposition characteristics, conductivity, and composition, we utilize a water phantom to represent the treatment target to facilitate the simulation setup and serve as a reference for treatment planning.

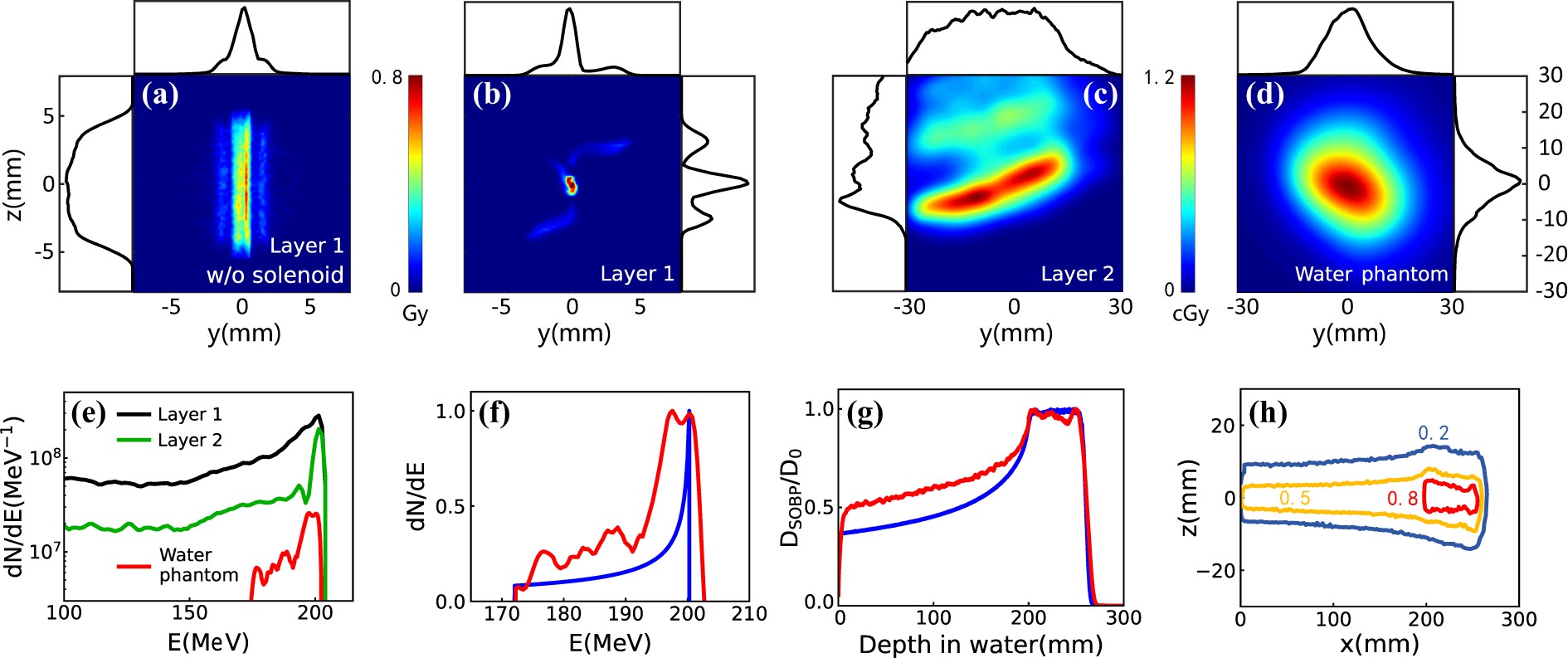
The water phantom is positioned coaxially with the second lead aperture. The deposited dose of proton beams is recorded by a $\rm 40\times40\times40 \; cm^3$ water phantom, which is divided into $4000 \times 4000 \times 4000$ cells. To ensure the consistency of the simulation, we output the information of all the macroprotons with energy higher than 10 MeV obtained from the aforementioned 3D PIC simulation, and then load them into the GEANT4 simulation. In order to observe the energy spectrum and transverse shape of the proton beam at different stages, virtual probe layers are placed behind solenoid and the dipole magnets to record the transverse dose distribution of the protons passed through (see figure 1). The detailed distribution is shown in figures 4(a)–(d), and the energy spectrum is also displayed in figure 4(e), respectively.

Figure  4.  Energy spectra and dose distribution of the proton beams in the transport and deposition stage simulated via the GEANT4 code. (a) and (b) Dose distributions at layer 1 without and with the solenoid, (c) and (d) the dose distributions for the case with the solenoid in the plane $(y,z)$ at diagnostic layer 2 and water phantom, respectively. Note that in (a) and (b), the dose values are normalized to the maximum dose at layer 1, and in (c) and (d) they are normalized to the maximum dose at water phantom. (e) The energy spectra of protons at diagnostic layers 1, 2, and water phantom are depicted by the black, green, and red lines, respectively. (f) Comparison of the energy spectrum of protons after the transport system (red line) and the ideal SOBP proton spectrum (blue line). (g) Lineouts of the effective dose curve (red), and ideal SOBP curve (blue) along $x$ direction. (h) The projection of normalized dose deposition on the $(x,z)$ plane at y = 0 mm.

DownLoad: Full-Size Img PowerPoint

For comparison, the FWHM of the proton energy spectrum and the beam spot diameter (defined as the width where the dose is half of the maximum) at each stage are listed in table 1. One can see that at the diagnostic layer 1, if without the collimation of the solenoid, the proton beam exhibits a dispersed transverse distribution (as shown in figure 4(a)). The elongation along the $z$ direction is due to the fact that the tape target is much longer in the $z$ direction than in the $y$ direction [45]. Conversely, with the solenoid, when propagating through the solenoid, protons will be focused by the longitudinal magnetic field. Thus, we observe a much more compact transverse distribution in figure 4(b), see lineouts in $y$ and $z$ directions shown in the upper and right side of figure 4(b). One can see that in the center, there is a tight pronounced peak which can be treated as a point-like Gaussian distribution. Moreover, we see two rotating-like wings surrounding the central peak. These indicate that protons are focused by the magnetic field.

Table  1.  The FWHM of proton spectrum and beam spot diameter at different stages.

Stage	FWHM of proton spectrum (MeV)	Beam spot diameter (mm)
Layer 1 (w/o solenoid)	14.39	-
Layer 1	14.39	1.0
Layer 2	8.57	35.0
Water phantom	6.83	20.0

 | Show Table

DownLoad: CSV

Then, the protons will propagate through the sector dipole magnets. Since these magnets can only deflect protons without focusing, their spatial distribution will be extended, as shown in figure 4(c). One can see that there is a region with significantly higher dose compared to the surrounding areas. This region corresponds to the area where high-energy protons from the proton spectrum represented by the green line in figure 4(e) pass through. The diameter of the proton beam focal spot is increased to approximately 35 mm. In figure 4(c), one can see that the beam seems to be split into two at diagnostic layer 2. This is because apart from the prominent peak around 200 MeV, there is also a lower energy peak around 100 MeV (see the proton energy spectrum distribution shown in figure 2(b)). As a result, after being deflected by the dipole magnets, this fraction of protons will accumulate at another location, forming the second spike in the transverse distribution characterized by a lower dose. However, due to their larger divergence, they cannot pass through the following slit and will be filtered out.

Subsequently, these protons will be further filtered and reshaped by the second aperture. The remaining protons will enter the water phantom and deposit their energies. The cross section ($y,z$) of the dose distribution is shown in figure 4(d), while the dose distribution in depth is illustrated in figure 4(g). Figure 4(h) displays the projection of the normalized dose distribution at $y=0$ mm. One can see that there is a dose plateau within the range from $\rm 200\; mm$ to $\rm 255\; mm$ in the $x$ direction. About 31.8% of macroprotons can pass through the BTS and reach the water phantom. This conversion efficiency is much higher than that via conventional magnets or pulsed two-solenoid beamline. The corresponding realistic proton number deposited in the water phantom is about $N=1.6\times 10^{9}$. The absolute dose value at the plateau position is $\rm 16.5\; cGy$ (1 Gy = 100 cGy), and the instantaneous dose rate reaches $\sim 10^9$ Gy/s. Usually the dose requirement for RT is 2 Gy per fraction [43], which requires at least 12 proton beams. Considering that a single treatment fraction is usually completed within 1–3 min [44], the laser repetition frequency should be higher than $\rm 0.067\; Hz$. The can be realized with the state-of-the-art petawatt-class laser technology [56] and targetry [57].

Further, to make a quantitative comparison with the ideal SOBP, we evaluate the compliance of the dose plateau. The dose plateau range $w_{\rm SOBP}$ is defined as the distance between the distal and proximal 90% dose equivalent points of the peak dose [58]. Within this range, the deviation of the dose equivalent $\delta$ varies from $-6.70\%$ to $3.59\%$, and the normalized standard deviation of the dose equivalent is $\sigma = D_{\rm STDEV}/D_{\rm mean} = 2.19\%$, where $D_{\rm mean}$ is the average dose and $D_{\rm STDEV}$ is the standard deviation. These results indicate that the obtained dose uniformity meets the requirements of clinical RT. Moreover, the distal falloff width of the dose plateau is $w_{\rm d}=7.0\; {\rm mm}$ (defined as the width of distal dose falloff from the $80\%$ to $20\%$ points of the peak dose [58]). For comparison, the ideal SOBP simulated according to [49] is depicted by the blue line in figure 4(g), with $w_{\rm d}=4.9\; {\rm mm}$. The detailed comparisons are presented in table 2.

Table  2.  Properties of the SOBP dose distribution in figure 4(g)

\$	$w_{\rm SOBP}$ (mm)	$w_{\rm d}$ (mm)	$\delta$	$\sigma$
Ideal SOBP	199.2‒256.3	4.9	$-8.52\%$ to $1.95\%$	$1.76\%$
$n_{\rm p}:n_{\rm C^{6+}}=1:1$	198.8‒254.7	7.0	−6.70% to 3.59%	2.19%

 | Show Table

DownLoad: CSV

3.   Discussion and conclusion

In our scheme, a large number of energetic electrons would be produced together with the proton beam. However, the magnetic field within the coil exceeds 10 T before the proton pulse reaches the helical coil, which would effectively deflect the high-energy electrons. On the other hand, a set of dipole magnets are in place to separate high-energy electrons from the mono-energetic proton beam. Given these factors, the electrons reaching the water phantom could be neglected.

Here for simplicity, we focus on the effect of the magnetic field inside the solenoid and neglect any accompanying electric fields [9]. As discussed in reference [9], there is a self-generated electric field inside the coil that can further accelerate those synchronized protons while decelerate those unsynchronized ones. Therefore, for more precise calculation, one needs to adjust the delay between the femtosecond and the nanosecond laser pulses to ensure the interested protons experience an accelerating and focusing field. However, this is just a minor effect that does not change the principle of our design.

On the other hand, current design is still not an all-optical BTS where a pair of dipole magnets is utilized. Moreover, an additional nanosecond laser pulse is adopted to support the generation of the magnetic field in the coil. However, as discussed in reference [9], this actually can be realized by simply connecting one end of the coil to the proton source target. The question would be if the generated magnetic field is sufficiently strong to focus the high-energy protons and whether the dipole magnets are still necessary.

In conclusion, we proposed a new design for the proton BTS of the laser-based IBT. We conducted combined simulations to investigate the whole process and show that this design is reasonable. Our simulations demonstrate that with this design, proton beams with peak energy above 200 MeV can be manipulated. An SOBP with a dose of 16.5 cGy and a width of several cm at a penetration depth of about 26 cm can be obtained. The instantaneous dose rate is extremely high, reaching $10^9$ Gy/s, which can be used in the FLASH RT studies.