Toroidal torques due to n = 1 magnetic perturbations in ITER baseline scenario

1.   Introduction

In present-day tokamak devices operating in H-mode, occurrence of the edge localized mode (ELM) is a common observation. The so-called type-I ELMs with relatively large-scale structures are characterized by intense bursts, during which a substantial amount of particle and thermal fluxes are released from the plasma boundary regime onto the plasma facing components. Consequently, type-I ELMs can potentially cause the severe erosion of materials surrounding the plasma, especially in the future tokamak devices such as ITER [1].

In view of this, it becomes imperative to tailor large ELMs, through either passive or active methods. Significant progress in this direction has been made through the active feedforward approach, utilizing the resonant magnetic perturbation (RMP) field to successfully suppress or mitigate type-I ELMs, as evidenced in recent experimental endeavors across several present-day fusion devices [2–8]. The experimental results not only highlight the effectiveness of RMPs, but also elevate the technique to a relatively mature status for ELM control, making it the baseline ELM control strategy for ITER.

Building on a solid foundation of theoretical analysis and experimental evidence, it has been demonstrated that the plasma response plays key roles in ELM control, ranging from causing three-dimensional (3D) plasma boundary corrugation [9–11], optimizing the spectrum of the RMP field [12–16], facilitating the 3D resonant field penetration during ELM suppression [4, 17], to the RMP-induced effects on the plasma momentum and particle transport [18–22].

The direct cause of the plasma momentum transport is the toroidal torque acting on the plasma, produced by the applied 3D field perturbation. This macroscopic 3D field induced momentum transport can substantially modify the toroidal rotation profile of the plasma [23], to an extent of causing full rotation braking and even mode locking [24, 25]. The latter should be avoided during the operation of a tokamak device due to the danger of causing plasma disruption. Understanding the toroidal torque distribution inside the plasma, produced by 3D perturbations, is therefore important for safe operation of the device.

Employment of the 3D RMP field, for the purpose of controlling ELMs, inherently produces toroidal torques as a consequence of the plasma response. These torques, typically acting as the momentum sinks, can come out of different physics natures. Important ones include the electromagnetic (‘JXB’) torque, the torque due to neoclassical toroidal viscosity (NTV), and the torque associated with the Reynolds (‘REY’) stress tensor. These torques in turn modify (damp) the toroidal flow of the plasma [26, 27]. Within the magnetohydrodynamic (MHD) description, 3D-field induced torques are all quasi-linear terms, implying that the torque value is proportional to the square of the plasma response field amplitude. We emphasize that the quasi-linearity here refers to the product of two linear perturbations with the same toroidal mode number, while non-linear MHD terms often involve perturbations of different (non-vanishing) toroidal mode numbers.

In this study, we will systematically quantify these quadratic terms for the ITER baseline scenario, with 5.3 T toroidal field, 15 MA plasma current and the fusion gain of $Q= 10$ [28]. This is of particular relevance given a general understanding that the toroidal flow in ITER is likely slower than that in many of the present-day tokamak devices. Given the uncertainty in the toroidal rotation prediction for ITER, we will scan the equilibrium flow speed (along both co- and counter-current directions) while computing the plasma response and the ensuing toroidal torques. The present study will only consider the $n=1$ (n is the toroidal mode number) perturbation field in view of the potential danger for causing mode locking in ITER. While computing toroidal torques in the slow-rotation regime, we find an interesting double-peak structure in the net torque versus the flow speed. Significant attention will therefore be paid to understand the physics mechanism behind this double-peak structure.

We remark that RMP-induced toroidal torques have also been computed for ITER in early work [10, 28]. While reference [10] focused on torques associated with higher-$n$ ($n=3$ and 4) RMP fields (and without scanning the toroidal rotation frequency), a recent study reported in reference [29] only considered the NTV torque in an ITER plasma.

The paper is organized as follows. Section 2 briefly discusses several model aspects, including the mathematical formulation behind the MARS-F code [30] which we utilize for the present study, the ITER equilibrium and the toroidal flow model. Section 3 reports various numerical results on the $n=1$ RMP-induced toroidal torques, with a special emphasis on the double-peak structure. Finally, section 4 summarizes the work.

2.   MARS-F formulation, equilibrium and ELM control coil current configuration

2.1   Formulation

The MARS-F code [30] solves the linearized single-fluid MHD equations in the plasma region, including the plasma resistivity and the toroidal plasma rotation (with frequency $\mathrm{\Omega }$) in full toroidal geometry [31]

where quantities $\boldsymbol{j}$, $\boldsymbol{b}$, $\boldsymbol{v}$, ${\rho }_{1}$ and p denote the linear perturbations for the plasma current, magnetic field, fluid velocity, plasma density and pressure, respectively. The plasma displacement is denoted as $\boldsymbol{\xi }$. The equilibrium quantities for the plasma density, pressure, current, magnetic field, and fluid velocity are represented by $\rho$, $P$, $\boldsymbol{J}$, $\boldsymbol{B}$ and ${\boldsymbol{V}}_{0}$, respectively. The unit vectors along the (geometric) toroidal angle and the vertical direction in the poloidal plane are denoted as $\widehat{\boldsymbol{\phi }}$ and $\widehat{\boldsymbol{Z}}$, respectively. $R$, $\eta$ and $\varGamma =5/3$ are the plasma major radius, the plasma resistivity and the ratio of specific heats, respectively. n represents the toroidal harmonic number, assumed to be 1 in this study as mentioned earlier. The last two terms from equation (3) – the perturbed momentum equation, denote the Coriolis and centrifugal forces.

The MARS-F model assumes a pure vacuum region outside the plasma. The RMP coils are located in the vacuum region, with the coil current density, ${\boldsymbol{j}}_{\mathrm{R}\mathrm{M}\mathrm{P}}$, considered as a source term satisfying $\nabla \times \boldsymbol{b}={\boldsymbol{j}}_{\mathrm{R}\mathrm{M}\mathrm{P}}$. The frequency of the RMP coil current is denoted by ${\mathrm{\Omega }}_{\mathrm{R}\mathrm{M}\mathrm{P}}$. The left-hand side of the above equations shows that the RMP coil current frequency contributes to a finite frequency shift, similar to that associated with the toroidal plasma rotation, although the latter also contributes to the right-hand of the equations.

We note that the perturbed magnetic field $\boldsymbol{b}$ in the MARS-F formulation always refers to the total perturbations produced by all conducting structures (including the plasma, coils etc.). Because the current perturnation is a spatially localized quantity, we have $\nabla \times \boldsymbol{b}={\boldsymbol{j}}_{\mathrm{p}\mathrm{l}\mathrm{a}\mathrm{s}\mathrm{m}\mathrm{a}}$ in the plasma region and $\nabla \times \boldsymbol{b}={\boldsymbol{j}}_{\mathrm{R}\mathrm{M}\mathrm{P}}$ in the region occupied by the RMP coils.

2.2   Equilibrium and 3D coil current configuration

In this study, we consider a baseline D-T scenario designed for ITER, with the full plasma current (15 MA), full magnetic field (5.3 T) and the fusion gain factor of $Q=10$. Figures 1(a)–(d) show radial profiles of the equilibrium safety factor, plasma pressure, (surface-averaged) toroidal plasma current density and the plasma number density. The key equilibrium parameters are ${q}_{0}=1.01$, ${q}_{95}=3.14$, ${\beta }_{\mathrm{N}}=1.97$, with the on-axis safety factor ${q}_{0}$ and the corresponding value at 95% equilibrium poloidal flux ${q}_{95}$. The normalized $\beta$ value is defined as ${\beta }_{\mathrm{N}}\equiv \beta \left(\%\right)a\left(\mathrm{m}\right){B}_{0}\left(\mathrm{T}\right)/ {I}_{\mathrm{p}}\left(\mathrm{M}\mathrm{A}\right)$, where a is the plasma minor radius, ${B}_{0}$ the equilibrium toroidal magnetic field at the plasma major radius ${R}_{0}$, and ${I}_{\mathrm{p}}$ the total plasma current.

Figure  1.  Equilibrium quantities for the ITER baseline scenario with the plasma current 15 MA, magnetic field 5.3 T and fusion gain Q = 10, showing (a)–(e) plot the radial profiles of the safety factor), pressure (normalized by ${B}_{0}^{2}/{\mu }_{0}$), the surface-avearged toroidal current density (normalized to unity on-axis), the plasma density (normalized to unifty on-axis), and the toroidal plasma rotation (normalized by the on-axis toroidal Alfvén frequency), respectively. Here, ${\psi }_{\mathrm{p}}$ represents the normalized equilibrium poloidal magnetic flux. (f) The poloidal cross section of the plasma shape (blue curve), the ELMs control coils (red squared curves) and the double wall structure designed for ITER (black curve).

DownLoad: Full-Size Img PowerPoint

The toroidal plasma rotation profile (figure 1(e)) is self-consistently simulated by the ASTRA transport code [32], assuming toroidal momentum input from the neutral beam injection foreseen for ITER. ASTRA assumes two key physics parameters: the plasma pedestal Prandtl number $\left({P}_{r}\right)$ and the ratio of the toroidal momentum to thermal confinement times $\left({\tau }_{\phi }/{\tau }_{E}\right)$. The profile shown in figure 1(e) corresponds to the assumption of ${P}_{r}=0.3$ and ${\tau }_{\phi }/{\tau }_{E}=1$. In what follows, we will scan the on-axis rotation frequency while self-similarly scale the whole rotation profile, as shown in figure 1(e).

Three toroidal rows of 3D magnetic coils, located at the low-field side, are designed in ITER (figure 1(f)), with nine window-frame coils per row. For the ITER baseline scenario (at least during the D-T phase), each of the 27 coils will be individually powered, allowing flexible choice of the coil phasing – the toroidal phase difference among three rows of coil currents for a given toroidal mode number n – for controlling ELMs. This flexibility has been employed in reference [10], where the optimal coil phasing (for the best ELM control) has been determined for each n-number from 1 to 6 according to certain figures of merit. For the $n=1$ configuration in particular, we found the optimal coil phasing between the upper and the middle rows ${\varPhi }_{\mathrm{U}}-{\varPhi }_{\mathrm{M}}=-{165}{\text{°}}$ and the optimal coil phasing between the lower and the middle rows ${\varPhi }_{\mathrm{L}}-{\varPhi }_{\mathrm{M}}={140}{\text{°}}$, where “U, M, L” denote the upper, middle and lower row. This coil phasing will be assumed in this work, while we perform toque studies due to the $n=1$ RMP in ITER. Furthermore, in view of the linear scaling of the plasma response to the applied coil current (and the quadratic scaling for the toroidal torques), we will assume a unit current, of 1 kAt, in all RMP coils.

We show one example with ${\mathrm{\Omega }}_{0}=2\times {10}^{-4}{\mathrm{\Omega }}_{\mathrm{A}}$ to illustrate the plasma response to the applied magnetic field perturbation, where ${\mathrm{\Omega }}_{0}$ is the on-axis toroidal plasma toroidal frequency, and ${\mathrm{\Omega }}_{\mathrm{A}}$ is Alfvén frequency. Figure 2 compares the poloidal spectra of the applied vacuum field with that of the total perturbation field including the plasma response. These plots show that the assumed n = 1 vacuum field has small resonant components (non-resonant field is adequate for NTV calculations), which are nevertheless further shielded at the corresponding rational surface locations due to the plasma response. Relatively large field outside of the resonant layers facilitates generation of the NTV torque in the plasma core region.

Figure  2.  Poloidal spectra of the perturbed radial field ${b}_{m}^{1}\left({\psi }_{\mathrm{p}}\right)$ for (a) the vacuum field and (b) the total field including the plasma response. The $n=1$ RMP coil current configuration is assumed. The ‘+’ symbols denote the resonant harmonics at the locations of the corresponding rational surfaces (i.e. $q=m/n=2/1,3/1$).

DownLoad: Full-Size Img PowerPoint

3.   MARS-F computed toroidal torques due to n = 1 RMP

In this section, we utilize MARS-F to compute three types of toroidal torques due to the applied RMP field: the NTV torque as well as that associated with the Maxwell and Reynolds stress tensors. We emphasize that all these torques are exclusively generated by the plasma response to the applied 3D field. We are primarily interested in the net torques over the plasma volume V_p.

The Maxwell stress torque is also commonly known as the $\boldsymbol{j}\times \boldsymbol{b}$ torque or the resonant electromagnetic torque. The net torque is computed as

where the notation $*$ indicates complex conjugate, and ${T}_{\boldsymbol{j}\times \boldsymbol{b}}$ is the surface averaged torque density. s here denotes the radial coordinate. Similarly, the net torque associated with the Reynolds stress is computed as

where ${T}_{\mathrm{R}\mathrm{E}\mathrm{Y}}$ is again the surface-averaged torque density.

The NTV torque has been extensively investigated in the context of tokamak physics [33], providing a theoretical framework for understanding the neoclassical effect associated with MHD perturbations such as the resistive wall mode. The first experimental evidence of the importance of the NTV torque on the plasma toroidal momentum confinement was reported in reference [34], where quantitative agreement was established between the theory-calculated NTV torque and that measured in the dedicate NSTX experiments.

In this study, the net NTV torque, ${T}_{\mathrm{N}\mathrm{T}\mathrm{V}}^{\mathrm{n}\mathrm{e}\mathrm{t}}$, is computed by MARS-F following a semi-analytic model from reference [35], where various plasma collisionality and resonance regimes are smoothly connected. This NTV torque model has previously been verified on a full toroidal drift kinetic model implemented in both MARS-K and GPEC [36]. Finally, the total net torque, ${T}_{{\mathrm{tot}}}^{\mathrm{n}\mathrm{e}\mathrm{t}}$, which directly influences the plasma toroidal flow due to the applied 3D field, is calculated as the sum of above three torques

In what follows, we will mainly report the net torques – for the total as well as each individual contribution – while varying the following parameters: the wall resistivity, the on-axis toroidal rotation frequency, the pedestal rotation profile, and the RMP coil current frequency. The focus is on the torque variation versus the plasma flow speed, in view of physics uncertainties associated with predicting the toroidal rotation speed in future devices including ITER.

3.1   Effect of wall resistivity

The $n=1$ wall eddy current decay time for the ITER vacuum vessel, with a double-wall structure, is about 0.4 s [37]. This translates to ${\tau }_{\mathrm{w}}/{\tau }_{\mathrm{A}}=5\times {10}^{5}$ for the baseline scenario plasma, where the resistivity wall time ${\tau }_{\mathrm{w}}$ is normalized by the Alfvén time ${\tau }_{\mathrm{A}}$. As the first study, we will vary the resistivity wall time to find out (i) how this affects the net toroidal torques acting on the plasma column, and (ii) how the results assuming the ITER double-wall compare with that of the no-wall or ideal-wall limit.

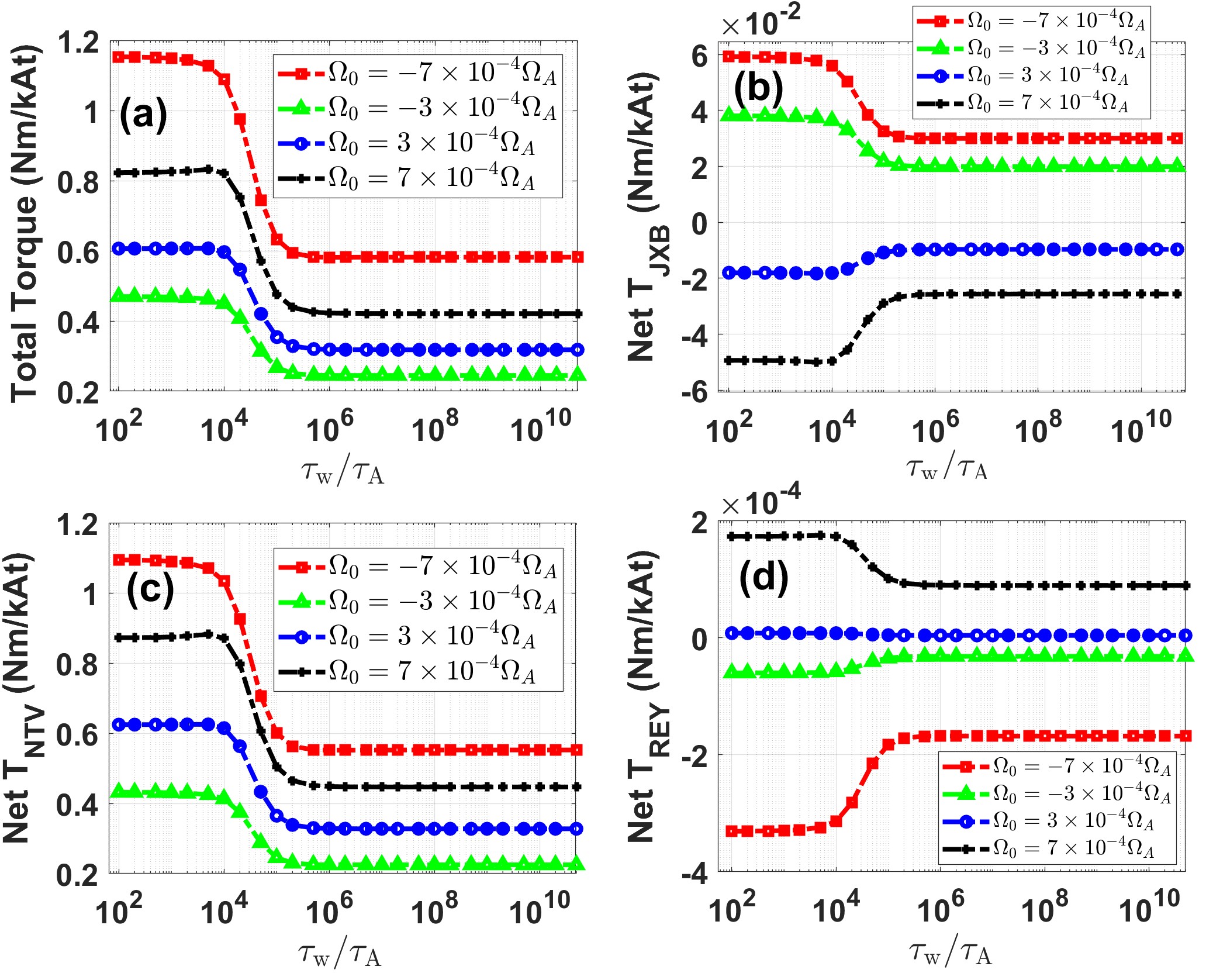
Figure 3 reports the MARS-F computed net torques for 4 cases, with different toroidal rotation frequencies ${\mathrm{\Omega }}_{0}=\pm 3\times {10}^{-4}{\mathrm{\Omega }}_{\mathrm{A}},\mathrm{ }\pm 7\times {10}^{-4}{\mathrm{\Omega }}_{\mathrm{A}}$ at the magnetic axis. Note that we consider cases with slow toroidal flow here, which will be shown later on to be the most interesting regime. It is evident that the amplitude of all three types of the toroidal torques, together with the sum of them, decreases with increasing the wall time in particular in the range of $\tau_{\mathrm{w}}/\tau_{\mathrm{A}}\ \sim\ [10^4,\ 10^6]$. The net torques on the other hand saturate at the no-wall and ideal-wall limits. The fact that the net torques remain nearly the same between the ITER resistive wall (${\tau }_{\mathrm{w}}/{\tau }_{\mathrm{A}}=5\times {10}^{5}$) and the ideal wall limit indicates that the ITER vacuum vessel essentially acts as an ideal conducting wall, as long as the toroidal torque computations are concerned.

Figure  3.  Comparison of (a) the total net torque ${T}_{{\mathrm{tot}}}^{\mathrm{n}\mathrm{e}\mathrm{t}}$ (the sum of all three types of the net torques), (b)–(d) the net electromagnetic torque ${T}_{\mathrm{J}\mathrm{X}\mathrm{B}}^{\mathrm{n}\mathrm{e}\mathrm{t}}$, the net neoclassical toroidal viscous torque ${T}_{\mathrm{N}\mathrm{T}\mathrm{V}}^{\mathrm{n}\mathrm{e}\mathrm{t}}$ and the net toroidal torque associated with the Reynolds stress ${T}_{{\mathrm{REY}}}^{\mathrm{n}\mathrm{e}\mathrm{t}}$, respectively, with scanning different normalized wall time ${\tau }_{\mathrm{w}}/{\tau }_{\mathrm{A}}$, and different toroidal rotation frequencies, ${\mathrm{\Omega }}_{0}=\pm 3\times {10}^{-4}{\mathrm{\Omega }}_{\mathrm{A}},\mathrm{ }\pm 7\times {10}^{-4}{\mathrm{\Omega }}_{\mathrm{A}}$. Here, the RMP field is produced by the $n=1$ coil current configuration with all three rows and optimal coil phasing. The assumed RMP frequency is ${10}^{-4}{\mathrm{\Omega }}_{\mathrm{A}}$.

DownLoad: Full-Size Img PowerPoint

Because of the total momentum conservation, the RMP-induced toroidal torques acting on the plasma have to be balanced by the coil-wall system. In the no-wall limit, the total torque acting on the plasma is balanced by the counter-torque acting on the coil system. The presence of a resistive (or ideal) wall absorbs a portion of the torque. This fact can be used as an intuitive argument explaining the reduced net torques acting on the plasma with increasing the wall time.

An important observation from figure 3, more specific to the ITER plasma under consideration, is the dominant contribution of the NTV torque to the total. This is associated with two important circumstances, i.e. (i) the slow plasma flow and (ii) the high thermal temperatures thus low particle collisionality, both favoring access to the so-called resonant NTV regime where the net torque is substantially enhanced. This important physics element will be illustrated in more detail later on. We remark that a similar result was also obtained for the ITER plasma in the presence of the $n=3$ RMP field [10]. Despite the dominant NTV contribution, we will keep reporting the other two types of torques (the electromagnetic and Reynolds stress torques) as well in view of physics understanding.

We also comment on the sign of the net torques, shown in figure 3, with respect to the assumed plasma flow direction. Negative sign of the net torque implies braking of the plasma co-current toroidal flow within our sign convention. Figure 3 thus shows that the NTV torque acts to enhance the co-current flow and reduces the counter-current flow (which is ultimately related to the so-called off-set rotation of the plasma due to NTV), for reasons to be elaborated later on. On the other hand, the electromagnetic torque always acts to reduce the plasma flow, independent of the flow direction. Interestingly, the Reynolds stress torque acts to speed up the plasma flow (independent of the flow direction), albeit with a minor effect. Figure 3 also shows that the amplitude of all three types of toques is strongly affected by the assumed initial flow speed. This important dependence is investigated in more detail in the following subsection.

3.2   Influence of toroidal flow speed

In this subsection, we focus on the effect of the toroidal flow speed on the computed net torques, in view of (i) the uncertainty in predicting the toroidal plasma rotation in ITER, and (ii) an interesting physics result occurring at slow flow as we will report. We start by showing the MARS-F computed toroidal torque density profiles (figure 4), assuming the ASTRA-predicted toroidal rotation profile (figure 1(b)). The plasma resistivity is evaluated according to the Spitzer model, resulting in the corresponding on-axis Lundquist number of $S=1.03\times {10}^{10}$. Furthermore, we assume an AC RMP field rotating at 0.1 kHz, a plausible option that has been considered for ITER to allow toroidally more uniform heat load onto the plasma facing components and within the engineering constraints on the coils [13, 15].

Figure  4.  Radial distributions of (a) the electromagnetic torque density, (b) the NTV torque density, and (c) the torque density associated with the Reynolds stress, for the ITER baseline scenario with $n=1$ 3D perturbation field and the (on-axis) rotation frequency fixed at $\mathrm{\Omega}_0=1.68\times10^{-2}\Omega_{\mathrm{A}}$. Here, ${\psi }_{\mathrm{p}}$ represents the normalized equilibrium poloidal magnetic flux. Toroidal torques are exerted as the plasma responds to the applied $n=1$ 3D perturbation field, produced by three rows of RMP coils in ITER with 1 kAt current and the optimal coil phasing of $(-165\text{°},\ 0\text{°},\ 140\text{°})$ for three rows. Vertical dashed lines indicate the radial location of the $n=1$ rational surfaces.

DownLoad: Full-Size Img PowerPoint

The net torques, obtained by integrating the torque densities shown in figure 4 over the plasma minor radius, are: $T_{\mathrm{t}\mathrm{o}\mathrm{t}}^{\mathrm{n}\mathrm{e}\mathrm{t}}=-8.51\times10^{-2}\; \mathrm{N}\mathrm{\cdot m}$, $T_{\mathrm{J}\mathrm{X}\mathrm{B}}^{\mathrm{n}\mathrm{e}\mathrm{t}}=-7.31\times10^{-3}\; \mathrm{N\cdot}\mathrm{m}$, $T_{\mathrm{N}\mathrm{T}\mathrm{V}}^{\mathrm{n}\mathrm{e}\mathrm{t}}= -7.79\times10^{-2}\; \mathrm{N\cdot}\mathrm{m}$, and $T_{\mathrm{R}\mathrm{E}\mathrm{Y}}^{\mathrm{n}\mathrm{e}\mathrm{t}}=6.14\times10^{-5}\; \mathrm{N}\mathrm{\cdot m}$, for the total and each of the individual contributions, respectively (recall that 1 kAt coil current is assumed here). The largest contribution to the net torque comes from the NTV, despite the fact that the peak values of the torque densities are larger for the Maxwell and Reynolds stress torques. This is because the latter (i) are much more locally radially distributed, near the perturbation rational surfaces, than the NTV torque, and (ii) vary in signs and are thus subject to a substantial cancellation effect when the net torques are evaluated. We therefore infer that while the NTV torque will likely be more important for the global modification of the plasma toroidal flow in ITER, the electromagnetic torque may play a larger role in local change of the rotation near the perturbation rational surfaces. In what follows, we will focus more on the net torques while scanning the toroidal rotation frequency.

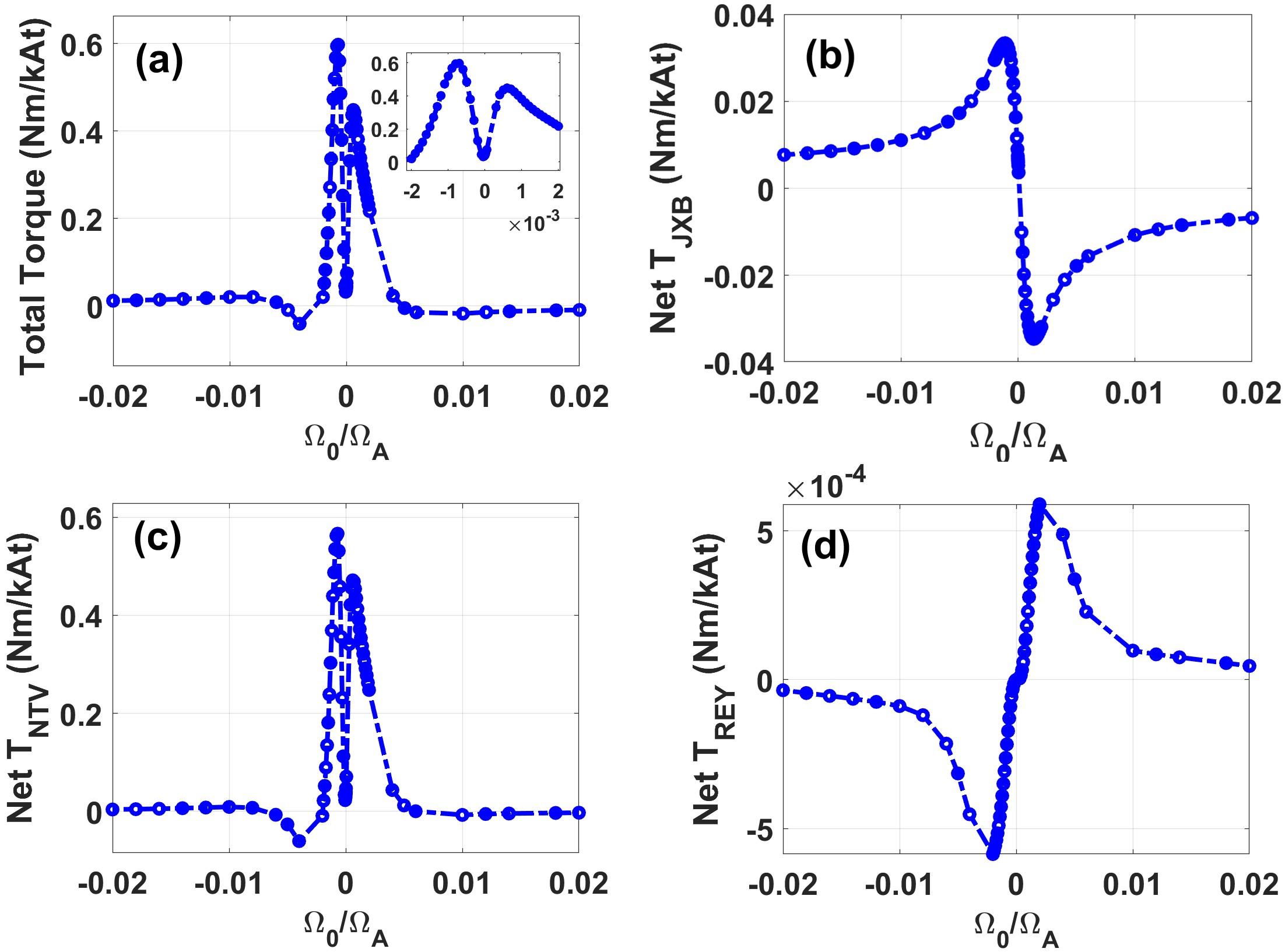
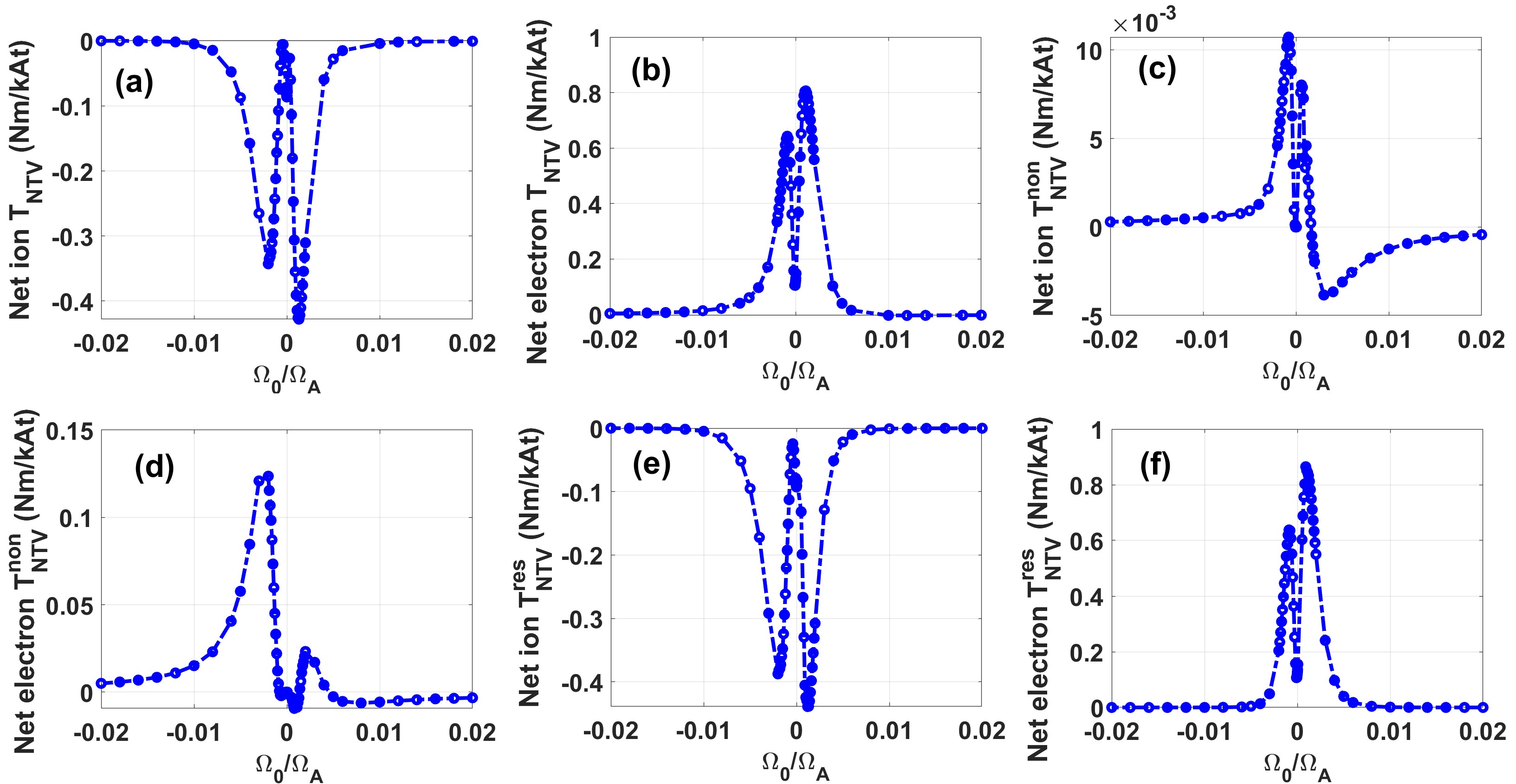
Figure 5 summarizes the computed net torques while scanning the on-axis toroidal rotation frequency in both co- and counter-current directions. Similar to the example reported in figure 4, the total net torque is dominantly contributed by NTV. This is particularly evident at slower plasma flow, where the NTV produces a large torque, with the positive sign independent of the assumed flow direction. On the contrary, both the electromagnetic and the Reynolds stress net torques switch signs together with the on-axis rotation frequency. Because of the dominant contribution by the NTV, the total net torque (figure 5(a)) nearly repeats that of the net NTV torque (figure 5(c)) as we scan the rotation speed.

Figure  5.  The computed net toroidal torques versus the (on-axis) toroidal rotation frequency (normalized by the on-axis toroidal Alfvén frequency ${\mathrm{\Omega }}_{\mathrm{A}}$), for (a) the total net torque ${T}_{\mathrm{t}\mathrm{o}\mathrm{t}}^{\mathrm{n}\mathrm{e}\mathrm{t}}$ including (b) the net electromagnetic torque ${T}_{\mathrm{J}\mathrm{X}\mathrm{B}}^{\mathrm{n}\mathrm{e}\mathrm{t}}$, (c) the net NTV torque ${T}_{\mathrm{N}\mathrm{T}\mathrm{V}}^{\mathrm{n}\mathrm{e}\mathrm{t}}$, and (d) the net torque ${T}_{\mathrm{R}\mathrm{E}\mathrm{Y}}^{\mathrm{n}\mathrm{e}\mathrm{t}}$ associated with the Reynolds stress. Toroidal torques are exerted as the plasma responds to the applied $n=1$ 3D perturbation field. The inset in plot (a) shows details of the double-peak structure at slow plasma rotation.

DownLoad: Full-Size Img PowerPoint

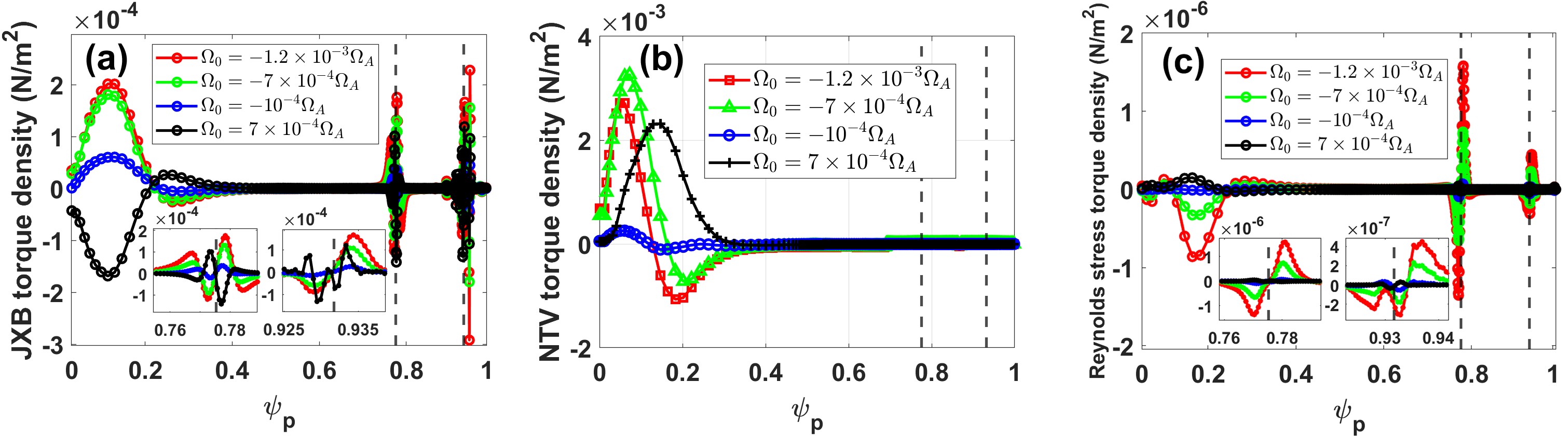
The most interesting observation from figure 5 is perhaps a double-peak structure in the net NTV torque versus rotation (c.f. inset from figure 5(a)), when the latter nearly vanishes. A similar finding was also reported in an early analytic work [38]. To better understand the physics nature of this double peak, we plot and compare in figure 6 the corresponding torque density profiles while assuming small on-axis rotation frequencies of ${\mathrm{\Omega }}_{0}={-1.2\times 10}^{-3}{\mathrm{\Omega }}_{\mathrm{A}}$, ${-7\times 10}^{-4}{\mathrm{\Omega }}_{\mathrm{A}}$, $-{10}^{-4}{\mathrm{\Omega }}_{\mathrm{A}}$ and ${7\times 10}^{-4}{\mathrm{\Omega }}_{\mathrm{A}}$.

Figure  6.  Radial distributions of the computed toroidal torque densities for (a) the electromagnetic torque, (b) the NTV torque, and (c) the torque associated with the Reynolds stress. Here, ${\psi }_{\mathrm{p}}$ represents the normalized equilibrium poloidal magnetic flux. Assumed are different rotation frequencies (${\mathrm{\Omega }}_{0}={-1.2\times 10}^{-3}{\mathrm{\Omega }}_{\mathrm{A}}$, ${-7\times 10}^{-4}{\mathrm{\Omega }}_{\mathrm{A}}$, $-{10}^{-4}{\mathrm{\Omega }}_{\mathrm{A}}$ and ${7\times 10}^{-4}{\mathrm{\Omega }}_{\mathrm{A}}$) at slow toroidal flow regime. Vertical dashed lines indicate the radial location of the $n=1$ rational surfaces for the considered ITER scenario. Insets in plots (a) and (c) show details of the torque density distribution near the perturbation rational surfaces. Toroidal torques are exerted as the plasma responds to the applied $n=1$ 3D perturbation field produced by three rows of RMP coils in ITER with 1 kAt current.

DownLoad: Full-Size Img PowerPoint

This double-peak structure is primarily contributed by the NTV from both thermal ions and electrons. We mention that a similar phenomenon has been experimentally observed and theoretically calculated for the DIII-D plasma [39]. Specifically, reference [39] reported a single large peak in the NTV torque corresponding to a toroidal rotation rate where the radial electric field ${E}_{r}$ is near zero. The difference of our result here, with respect to the previous finding, is the presence of two peaks near but not exactly at the vanishing flow. Nevertheless, observation of similar peaking structures at slow flow underscores the fundamental physics role of the superbanana plateau resonant regime in contributing to the large NTV torque, which appears to be insensitive to the device and RMP configurations (e.g. n = 3 RMP employed in reference [39] versus n = 1 assumed in the present study) .

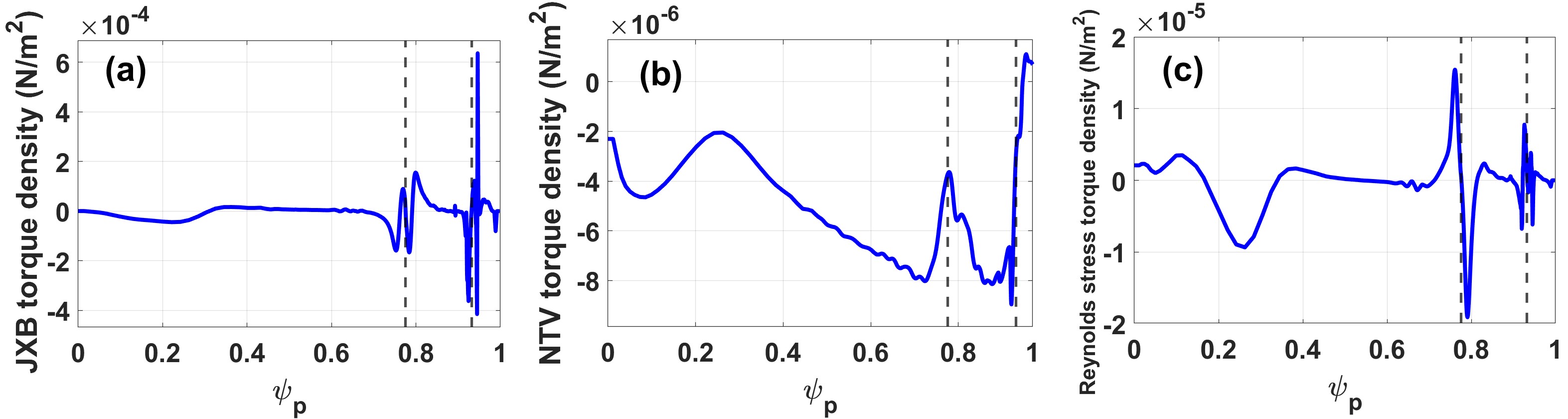
Several interesting observations can be made from figure 6. First, the torque density distribution has a large peak near the magnetic axis at slow flow, for all three types of the toroidal torque (to be explained later on). Note that such a core-localized peak is much less prominent at fast plasma flow for ${T}_{\mathrm{R}\mathrm{E}\mathrm{Y}}$ and ${T}_{\boldsymbol{j}\times \boldsymbol{b}}$ (c.f. figure 4). Next, the ${T}_{\boldsymbol{j}\times \boldsymbol{b}}$ and ${T}_{\mathrm{R}\mathrm{E}\mathrm{Y}}$ torque densities also peak at the $q=2$ and 3 rational surfaces, as expected for the $n=1$ perturbation. The sharp radial variations of the torque densities near rational surfaces are numerically well resolved (insets in figures 6(a) and (c)). Finally, in terms of the overall amplitude of the torque density, the NTV contribution is the largest among all three types. This, in combination with the cancellation effect of the ${T}_{\mathrm{R}\mathrm{E}\mathrm{Y}}$ and ${T}_{\boldsymbol{j}\times \boldsymbol{b}}$ densities across rational surfaces, further promotes the dominant role of the net NTV torque as reported in figure 5, at slow plasma flow.

We note that the core-localized NTV becomes small as the plasma flow speed approaches zero (the blue curve in figure 6(b)). Because of the dominant NTV contribution to the total net torque, we investigate the double-peak structure shown in figure 5(a) by focusing on the NTV torque physics at slow plasma flow. The net NTV torque is contributed by both the thermal ions and electrons. For each particle species, the associated torque comes from the resonant and non-resonant portions. All these individual contributions are illustrated and compared in figure 7.

Figure  7.  The computed net NTV torques versus the on-axis toroidal rotation frequency (normalized by the on-axis toroidal Alfvén frequency ${\mathrm{\Omega }}_{\mathrm{A}}$) for the ITER baseline scenario, contributed by thermal ions (left panels) and electrons (right panels). For each particle species, the ((a) and (b)) net torque also comes from the ((c) and (d)) non-resonant and ((e) and (f)) resonant NTV contributions. The NTV torques are exerted as the plasma responds to the applied $n=1$ 3D perturbation field.

DownLoad: Full-Size Img PowerPoint

The resonant NTV becomes important when the plasma $\boldsymbol{E}\times \boldsymbol{B}$ rotation is slow, thus better matching the (slow) toroidal precession of the banana orbits of trapped thermal particles. In other words, the ${\omega }_{\mathrm{E}}\sim{\omega }_{\mathrm{D}}$ resonance condition is well satisfied at slow plasma flow (where ${\omega }_{\mathrm{E}}$ is assumed to be the $\boldsymbol{E}\times \boldsymbol{B}$ flow in this study, and ${\omega }_{\mathrm{D}}$ is the precessional drift frequency). We make two important notes here. Firstly, the resonance here refers to Landau wave-particle resonance in the plasma frame, where the wave is the plasma perturbation due to the applied 3D field. Because a nearly static perturbation is applied (as shown in figures 5–7), the corresponding wave frequency is effectively the plasma toroidal rotation frequency in the plasma frame. Secondly, the Landau resonance can only be prominent in the low-collision regime, which is the case in the plasma core in ITER. Figure 7 shows that the large net torque, at slow plasma flow, mainly comes from the resonant NTV contribution. This is even more so for thermal ions which are nearly collisionless in ITER. Nevertheless, MARS-F modeling finds an even larger resonant NTV contribution from thermal electrons at slow plasma flow, resulting in the so-called electron root for the NTV torque.

The non-resonant NTV contributions, occurring when the resonance conditions are not well satisfied (i.e. ${\omega }_{\mathrm{E}} > {\omega }_{\mathrm{D}}$ and/or finite particle collisionality), are relatively small in ITER. We note that the aforementioned wave-particle resonance occurs in the local particle phase space. Therefore, for a given configuration space and a given plasma flow, there are always particles that do not satisfy the resonance conditions, resulting in (small but finite) non-resonant NTV. Figure 7 shows that the double-peak structure observed in figure 5(a) is predominantly associated with the resonant NTV contribution.

We also comment on the signs of the net torques. In the rotation range within the double-peak structure, the resonant NTV due to thermal ions is counter-current. The non-resonant contribution is co-current but very small, resulting in the counter-current NTV for ions. Both the resonant and non-resonant net NTV torques due to thermal electrons are co-current and larger in amplitude than that of the ion contributions. Consequently, partial cancellation occurs between the ion and electron contributions for the net NTV torque, resulting in a smaller but positive total net NTV as reported in figure 5(c).

The resonant versus non-resonant NTV torques in the configuration space are compared in figure 8, where we compute the torque density contributions assuming various (fast versus slow) toroidal rotations. The sum of the thermal ion and electron contributions is taken here when showing the torque densities. The fast-flow cases (figures 8(a) and (b)) refer the toroidal rotation frequencies well outside the range of the double-peak structure shown in figure 5(a). Because of the symmetry of the structure, only cases with negative rotation frequencies are included in figure 8.

Figure  8.  Radial distributions of the computed toroidal NTV torque densities for the non-resonant (left panels) versus resonant (right panels) components, assuming several cases of ((a) and (b)) fast flow regime ($\mathrm{\Omega}_0=-1.8\times10^{-2}\mathrm{\Omega}_{\mathrm{A}}$, $-1.6\times10^{-2}\mathrm{\Omega}_{\mathrm{A}}$ and $-1.4\times10^{-2}\mathrm{\Omega}_{\mathrm{A}}$) and ((c) and (d)) slow flow regime ($\mathrm{\Omega}_0=-3\times10^{-3}\mathrm{\Omega}_{\mathrm{A}}$, $-1.7\times10^{-3}\mathrm{\Omega}_{\mathrm{A}}$ and $-1.3\times10^{-3}\mathrm{\Omega}_{\mathrm{A}}$) for the considered ITER scenario. Here, ${\psi }_{\mathrm{p}}$ represents the normalized equilibrium poloidal magnetic flux. Vertical dashed lines indicate the radial location of rational surfaces associated with the $n=1$ 3D perturbation field, produced by three rows of RMP coils with 1 kAt coil current.

DownLoad: Full-Size Img PowerPoint

With a higher toroidal plasma rotation as shown in figures 8(a) and (b), the maximum non-resonant component of the NTV torque density is larger than the resonant component. At slower toroidal flow (figures 8(c) and (d)), the overall magnitude of the resonant NTV is much larger than that of the non-resonant component. More importantly, figure 8 shows that NTV torque predominantly comes from the plasma core in the ITER scenario considered, independent of the assumed toroidal flow speed. Since the resonant contribution is dominant, we search for an explanation in terms of the precessional drift frequency of trapped particles in the particle phase space.

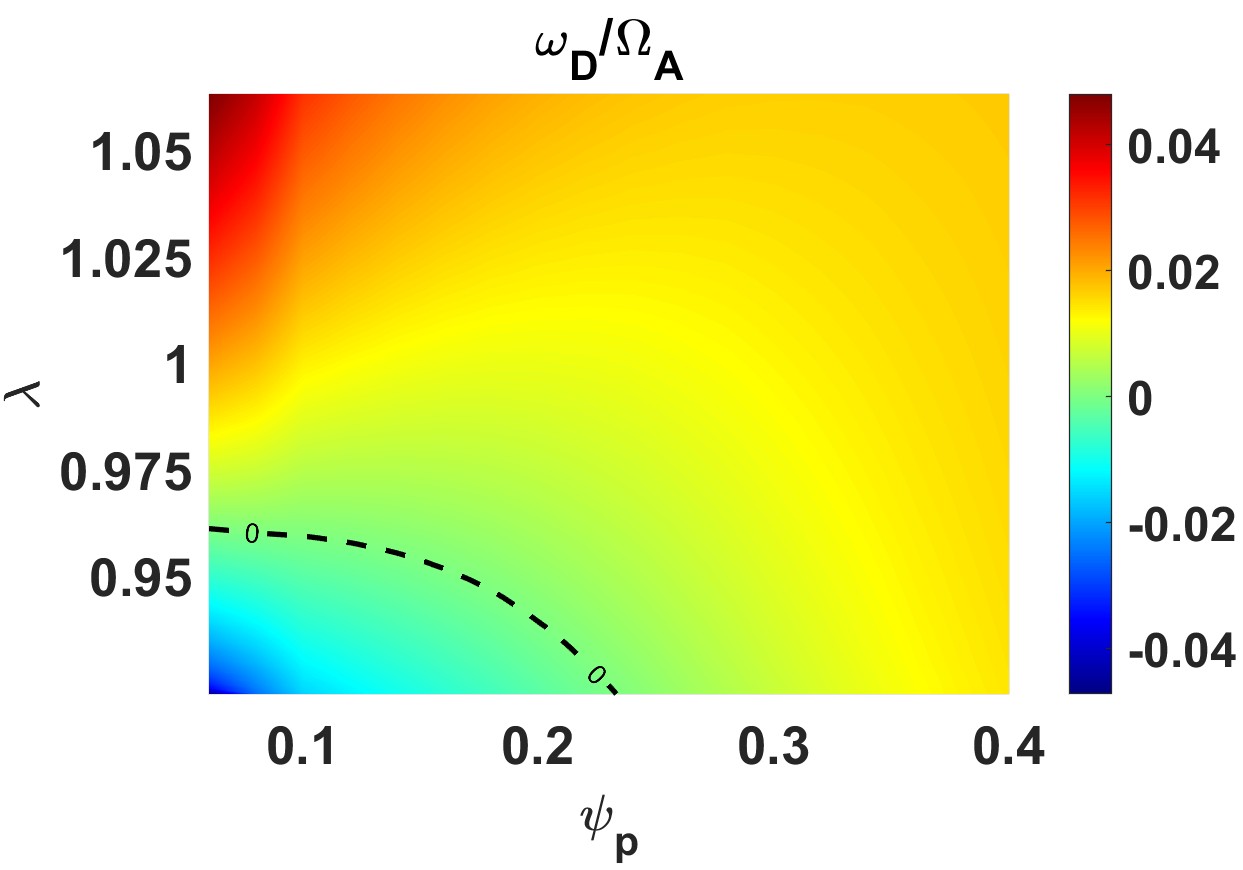
In the local phase space, the toroidal precession frequency ${\omega }_{\mathrm{D}}$ scales linearly with the particle energy but non-linearly with the particle pitch $\lambda$ (defined here as the ratio of the particle magnetic momentum to the particle kinetic energy). In particular, the diamagnetic effect produces the so-called precession reversal effect [40]. Consequently, ${\omega }_{\mathrm{D}}$ can vanish at certain particle pitch at a given plasma radial coordinate, as is indeed the case for the ITER plasma under consideration (figure 9). Even more importantly, it is the slope of the dependence ${\omega }_{\mathrm{D}}\left(\lambda \right)$ at the reversal point that ultimately dictates the resonance contribution of trapped particles at slow (or vanishing) plasma flow [40]. Small slope (i.e. small $|\partial {\omega }_{\mathrm{D}}/\partial \lambda |$), as shown in figure 9, leads to a large resonant contribution. This is the reason for the computed large resonant NTV torque density in the plasma core region, as shown in figure 8(d). Note that this is despite the fact that the particle trapping fraction is relatively small in the plasma core. The resonant NTV contribution of course vanishes at the magnetic axis, where the trapped particle fraction also vanishes. We also point out the good alignment of the radial domain (${\psi }_{\mathrm{p}} < 0.25$ in figure 8(d)) showing a large resonant NTV torque with that covered by the toroidal precession reversal effect (figure 9), further confirming the above physics explanation.

Figure  9.  The MARS-K computed toroidal procession drift frequency ${\omega }_{\mathrm{D}}$ (normalized by the toroidal Alfvén frequency ${\mathrm{\Omega }}_{\mathrm{A}}$) in the 2D $\left({\psi }_{\mathrm{p}},\lambda \right)$ space for trapped particles [both thermal ions and electrons], in the presence of the equilibrium magnetic field. Here $\lambda ={\left({v}_{\parallel }/v\right)}^{2}\left({B}_{0}/B\right)$ denotes the particle pitch. The particle energy is fixed at a value corresponding to the thermal temperature. The dashed line indicates the precession reversal point where ${\omega }_{\mathrm{D}}$ vanishes.

DownLoad: Full-Size Img PowerPoint

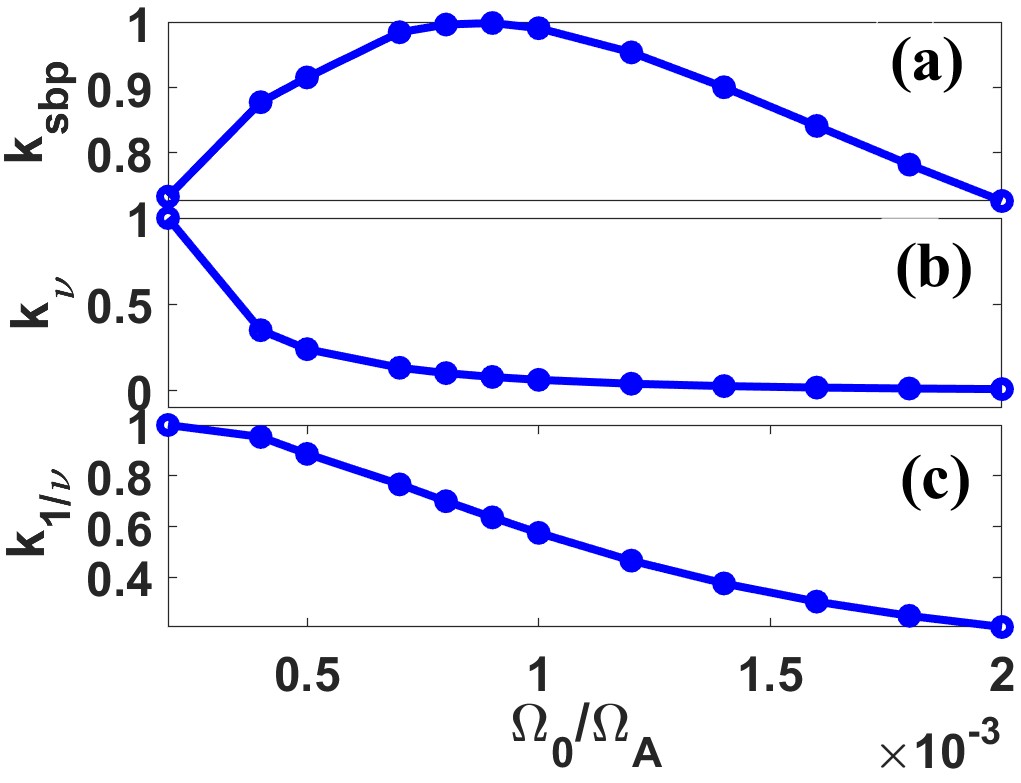
Figure 9 reveals the origin of the resonant NTV contributions, but still does not explain the double-peak structure of the net NTV torque versus plasma rotation shown in figure 5(c). A careful examination of all factors associated with the NTV torque points to the kernels obtained after the particle pitch-angle integration, as shown in figure 10. These kernels, corresponding to the asymptotic limits of the NTV transport fluxes [33], are evaluated at approximately the radial location of the peak NTV torque density and for the resonant super-plateau regime (figure 10(a)) versus the two non-resonant regimes (figures 10(b) and (c)). We point out that the values of ${k}_{\mathrm{s}\mathrm{b}\mathrm{p}}$ factor peak for both positive and negative rotations, while figure 10 only shows the one with positive rotation.

Figure  10.  Comparison of the asymptotic limits of the NTV transport fluxes versus the on-axis toroidal rotation frequency, showing kernels from the particle pitch-angle integration for (a) the superbanana plateau regime ${k}_{\mathrm{s}\mathrm{b}\mathrm{p}}$, (b) the $\nu$-regime and (c) the $1/\nu$-regime. The kernels are evaluated at ${\psi }_{\mathrm{p}}=0.1$ and for the particle energy corresponding to the thermal temperature.

DownLoad: Full-Size Img PowerPoint

Figure 10(a) shows a non-monotonic behavior of the resonant NTV kernel at slow rotation. This appears to be the ultimate reason of the double-peak structure reported in figure 5(c) for ITER. Note that the pitch-angle integral shown in figure 10(a) also partially propagates into evaluation of the non-resonant portion of the NTV torque via the particle energy integration, causing also the double-peak structure in the non-resonant NTV torque in ITER, as shown in figure 7. Other regimes (the so-called $\nu$-regime and $1/\nu$-regime) exhibit a monotonic behavior.

We should mention that the pitch-angle integrals versus the plasma rotation, compared in figure 10, are normalized by the peak value, because we are mostly interested in the overall behavior of the curves. We also mention that other factors, e.g. the response amplitude for the Lagrangian perturbation of the magnetic field while varying rotation, as well as the favorable average curvature-induced plasma screening (at slow flow) [25], have also been examined but no trends are identified that are consistent with the double-peak structure appearing in the net NTV torque for this scenario.

We emphasize that in the single fluid formulation, what matters is the plasma fluid flow. In the drift-kinetic theory (which is what the NTV calculation is based upon), the ultimate role is played by the $\boldsymbol{E}\times \boldsymbol{B}$ flow in combination with the diamagnetic flow, but the roles of these two flows are separated. Our assumption of using the plasma flow as proxy of the $\boldsymbol{E}\times \boldsymbol{B}$ flow is generally valid only in the case of sufficiently fast plasma flow (faster than the diamagnetic flow), or in the plasma core region where the diamagnetic flow is slow. The latter applies more to our situation since the computed NTV torque peaks near the plasma core.

3.3   Effect of pedestal rotation on net torques

We now turn to a sensitivity study of the RMP-induced net torque against uncertainty in predicting the pedestal toroidal rotation in ITER. Based on the model assumptions, the ASTRA transport code predicts vastly different pedestal rotations [10]. Examples of two extreme rotation profiles (dubbed Rotation A and Rotation B) are shown in figure 11(a) for the ITER scenario considered here. The assumed transport models in the ASTRA simulation are ${P}_{r}=1$ (for the Prandtle nmber) and ${\tau }_{\phi }/{\tau }_{E}=1$ (Rotation A) and ${P}_{r}=0.3$ and ${\tau }_{\phi }/{\tau }_{E}=1$ (Rotation B), respectively.

Figure  11.  Comparison of the computed net torques for the ITER plasma with different rotation models, where (a) plots the radial profiles of the toroidal rotation with model A (${P}_{r}=1$ and $\tau_{\phi}/\tau_E=1$) and model B (${P}_{r}=0.3$ and $\tau_{\phi}/\tau_E=1$), here ${\psi }_{\mathrm{p}}$ represents the normalized equilibrium poloidal magnetic flux, and compare (b) the net electromagnetic torque, ${T}_{\mathrm{J}\mathrm{X}\mathrm{B}}^{\mathrm{n}\mathrm{e}\mathrm{t}}$, (c) the NTV torque, ${T}_{\mathrm{N}\mathrm{T}\mathrm{V}}^{\mathrm{n}\mathrm{e}\mathrm{t}}$, and (d) the toroidal torque associated with the Reynolds stress, ${T}_{\mathrm{R}\mathrm{E}\mathrm{Y}}^{\mathrm{n}\mathrm{e}\mathrm{t}}$, versus the (on-axis) toroidal rotation frequency (normalized by the on-axis toroidal Alfvén frequency ${\mathrm{\Omega }}_{\mathrm{A}}$). The toroidal torques are exerted due to the plasma response to the applied $n=1$ 3D perturbation field.

DownLoad: Full-Size Img PowerPoint

Figures 11(b)–(d) show the MARS-F computed net torques versus the on-axis rotation frequency, while fixing the overall shape of the radial profiles for these two extreme cases shown in figure 11(a). As the key observation, the computed net torque is generally robust against variation of the pedestal rotation. The only exception is the toroidal torque associated with the Reynolds stress (figure 11(d)), which starts to significantly differ at fast co-current rotation. We have also scanned the rotation profiles between the two extreme cases shown in figure 11(a). The resulted net torques fall between the blue and red curves from figures 11(b)–(d), as expected.

3.4   Effect of frequency of n = 1 3D perturbation field on net torque

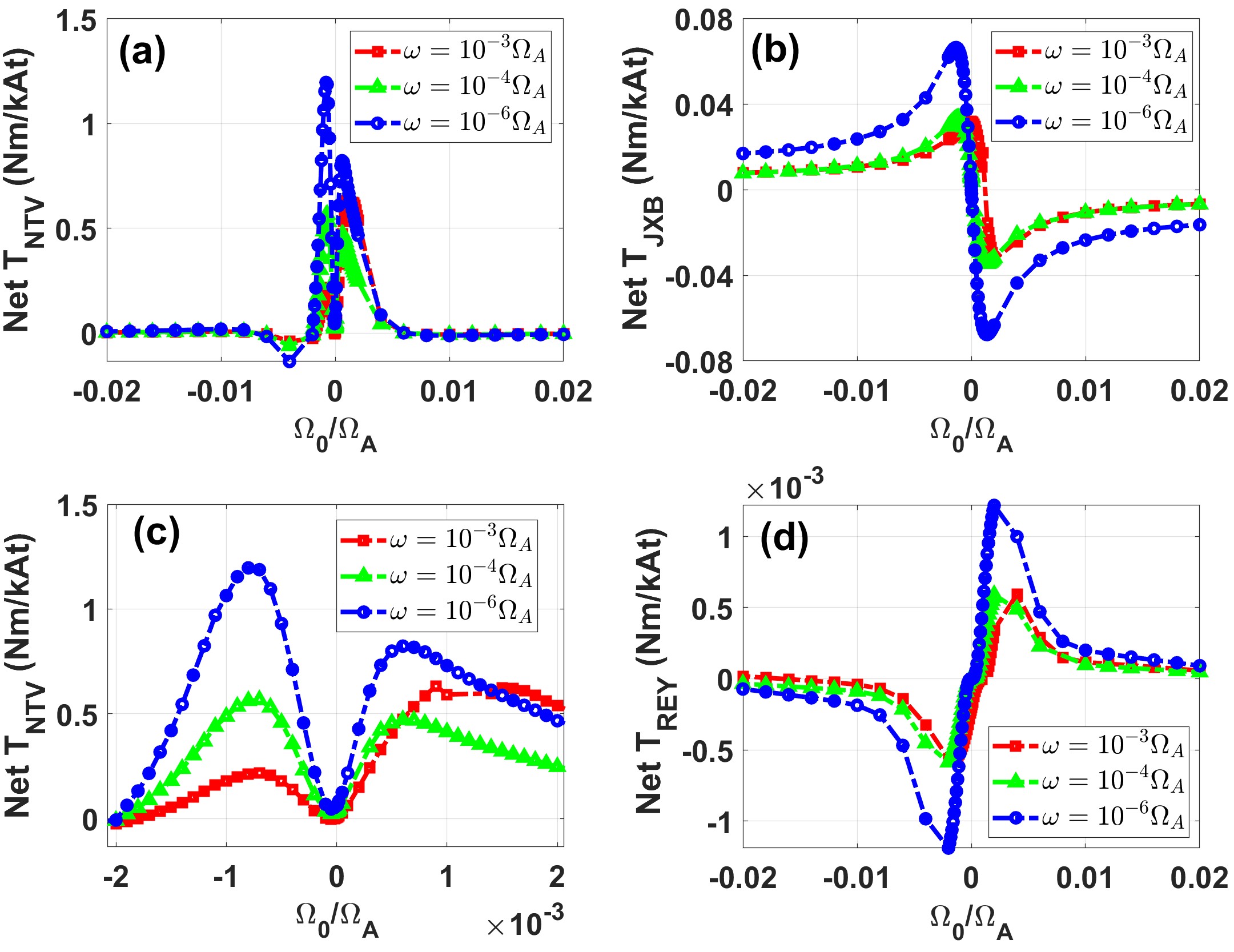
Out of concerns on the RMP-induced divertor heat loads, a slowly rotating RMP field (at several Hz) is also considered for ITER. This prompts us to perform a sensitivity analysis of the computed net torques against the coil current frequency, as the final part of the present investigation. The results are summarized in figure 12, where we assume three frequencies for the applied RMP: $\omega=10^{-3}\mathrm{\Omega}_{\mathrm{A}},\ 10^{-4}\mathrm{\Omega}_{\mathrm{A}}$ and $10^{-6}\mathrm{\Omega}_{\mathrm{A}}$, corresponding to about 1 kHz, 0.1 kHz and 1 Hz.

Figure  12.  Comparison of (a) the NTV torque, ${T}_{\mathrm{N}\mathrm{T}\mathrm{V}}^{\mathrm{n}\mathrm{e}\mathrm{t}}$, (b) the net electromagnetic torque, ${T}_{\mathrm{N}\mathrm{T}\mathrm{V}}^{\mathrm{n}\mathrm{e}\mathrm{t}}$, and (d) the toroidal torque associated with the Reynolds stress , ${T}_{\mathrm{R}\mathrm{E}\mathrm{Y}}^{\mathrm{n}\mathrm{e}\mathrm{t}}$, versus the (on-axis) toroidal rotation frequency (normalized by the on-axis toroidal Alfvén frequency ${\mathrm{\Omega }}_{\mathrm{A}}$), with different frequencies of the 3D perturbation field, $\omega=10^{-3}\mathrm{\Omega}_{\mathrm{A}},\ 10^{-4}\mathrm{\Omega}_{\mathrm{A}},\ 10^{-6}\mathrm{\Omega}_{\mathrm{A}}$, where (c) shows the zoomed-in ${T}_{\mathrm{N}\mathrm{T}\mathrm{V}}^{\mathrm{n}\mathrm{e}\mathrm{t}}$. The toroidal torques are exerted due to the plasma response to the applied $n=1$ 3D perturbation field.

DownLoad: Full-Size Img PowerPoint

Figures 12(b) and (d) show that a finite-frequency RMP field tends to produce less Maxwell and Reynolds stress torques, in a wide range of toroidal plasma rotation. The largest net torque contribution, however, still comes from the NTV torque (figure 12(a)). The effect of finite RMP frequency here is more localized, i.e. strongly reducing the amplitude of the double-peak structure at the slow-flow regime (figure 12(c)). Figure 12 thus shows that application of the RMP field with a small finite frequency not only helps the heat load distribution on the divertor plates, but also changes the net toroidal torques acting on the plasma.

On the physics side, we point out a subtle difference between the plasma toroidal rotation and the RMP field rotation. While the former contributes to inertial forces on the plasma – the centrifugal and Coriolis forces, the latter only introduces a finite frequency shift in the left-hand side of equations (1)–(5), for computing the plasma response.

4.   Disscussion and conclusion

Utilizing the MARS-F (and partly MARS-K) code, we have systematically investigated the toroidal torques generated by the $n=1$ RMP field and acting on the plasma column in ITER. Careful evaluation has been carried out for the slow-flow regime which is relevant to burning plasma scenarios. Both co- and counter-current plasma rotations are considered to exploit the symmetry property of the computed torques. Furthermore, sensitivity studies have also been performed against variation of several important plasma-wall-coil parameters (wall resistivity, plasma pedestal rotation, and the RMP coil current frequency). Our modelling revealed two key findings.

First, the neoclassical toroidal viscosity, in particular the resonant portion, is found to provide the dominant contribution to the total toroidal torque under the slow plasma flow regime in ITER. The other two types of torques that we examined, associated with the Maxwell and Reynolds stress tensors, respectively, are small in amplitude as compared to the NTV torque. As a physics-wise interesting observation though, the net electromagnetic torque switches sign from the co- to counter-current flow, thus always opposing the plasma rotation. The Reynolds stress torque on the other hand always enhances the plasma flow, albeit with negligibly small effect.

The second significant finding is the double-peak structure for the computed net torque in ITER, as we scan the toroidal rotation frequency near zero. This net torque, again primarily contributed by NTV from both thermal ions and electrons, remains positive independent of the plasma flow direction. In other words, in the very slow plasma rotation regime, the NTV torque accelerates the co-current flow but decelerates the counter-current flow. Since the double-peak structure occurs only at nearly vanishing toroidal rotation, this finding is of primarily physics interest. Among various possible factors, the resonant NTV contribution in the superbanana plateau regime, in particular the non-monotonic behavior of the wave-particle resonance integral (over the particle pitch angle), is identified as the ultimate reason for the appearance of the double-peak structure.

The above findings remain robust against variations of a range of factors. The dominance of the NTV torque with varying wall resistivity highlights the practical importance of controlling the former for tailoring the plasma rotation (and rotation shear) in ITER. The computed net torque, versus the whole rotation scan, is found insensitive to the pedestal rotation. The latter can be vastly different with different assumptions on the momentum transport in ITER. Finally, imposing a finite frequency on the applied RMP field reduces the overall amplitude of the net NTV torque, but without changing the characteristic double-peak structure. This suggests that a rotating RMP field, at Hz-level, may also affect the plasma flow (at very slow flow regime) via NTV, on top of helping reduce the peak heat load on the divertor surface.

These findings are important to ITER. In particular, the double-peak structure that we report in this work implies more complicated flow damping dynamics in the slow-flow regime. This also means that the RMP field penetration and error-field induced mode locking in ITER may be more complicated when the NTV torque is dominant. Double peaking of the NTV torque can introduce different mode locking bevavior than that predicted by conventional theory where the resonant Maxwell stress torque plays the dominant role.

We make two remarks here. Firstly, the NTV net torque studied in this work is comparable to the NBI torque in ITER and thus can significantly affect the plasma flow. The NBI system operating at 33 MW in ITER privides about 35 N·m net torque according to the modeling [41]. This net torque can be smaller than the RMP induced torques in ITER, as shown in previous work [10, 42]. The torque density distribution is however often different between the NBI and the 3D field-induced torques [43]. Secondly, the smallness of the Maxwell and Reynolds stresses, as compared to the NTV torque, is valid in specific situations considered in this work (i.e. resulting from plasma response to applied 3D perturbations at slow plasma flow). In other circumstances, these stresses can be large and play important roles, e.g. in driving intrinsic flows [44]. Depending on the situation, these stresses can also be important even in MHD settings.