| Citation: | Wei YAN, Guinan ZOU, Zhongyong CHEN, You LI, Jiangang FANG, Zhifang LIN, Zhonghe JIANG, Nengchao WANG, Bo RAO, Yangbo LI, Zhengkang REN, Chuanxu ZHAO, Yu ZHONG, Fanxi LIU, Yinlong YU, Zisen NIE, Xun ZHOU, Yuan SHENG, Yuwei SUN, Song ZHOU, Xiaoqing ZHANG, Zhoujun YANG, Zhipeng CHEN, Yonghua DING, the J-TEXT Team. Influence of 3D helical magnetic perturbations on runaway electron generation in J-TEXT tokamak[J]. Plasma Science and Technology, 2025, 27(3): 035104. DOI: 10.1088/2058-6272/ada5c6 |
A large number of runaway electrons (REs) generated during disruption can cause significant damage to next-generation large-scale tokamaks. The influence of three-dimensional (3D) helical magnetic perturbations on the suppression of RE generation was explored using a set of 3D helical coils in J-TEXT tokamak, which can excite m/n = −2/2 helical magnetic perturbations. Experimental evidence shows that the −2/2 magnetic perturbations caused by the opposite coil current direct plasma toward the high-field side, simultaneously enhancing the magnetic fluctuations, which would enhance the radial loss of REs and even prevent RE generation. On the other hand, −2/2 magnetic perturbations can also reduce the cooling time during the disruption phase and generate a population of high-energy REs, which can interact with high-frequency magnetic fluctuations and in turn suppress RE generation. The critical helical coil current was found to correlate with electron density, requiring higher coil currents at higher densities. According to the statistical analysis of RE generation at different electron densities, the applied −2/2 magnetic perturbations can increase the magnetic fluctuations to the same level at lower electron densities, which can decrease the threshold electron density for RE suppression. This will be beneficial for RE mitigation in future large tokamak devices.
Major disruption is one of the crucial issues for tokamak operations, especially for large devices with a high plasma current. The runaway electrons (REs) generated during the disruption damage the plasma-facing components of a tokamak [1]. For ITER with 15 MA plasma current operation, a major disruption will lead to the formation of a potentially huge runaway current, up to 10 MA [2, 3]. Therefore, effectively avoiding and mitigating REs is one of the key issues for future large tokamaks.
In general, a sufficiently high plasma density can suppress the avalanche amplification of REs through Coulomb collisions, which have been investigated through massive gas injection (MGI) [4, 5] and shattered pellet injection [6]. Owing to the insufficient assimilation efficiency of injected impurities, the required Rosenbluth density is very difficult to achieve [7, 8], and the dissipation efficiency becomes saturated when the injected impurity quantity exceeds a certain amount [9]. However, the injected impurity concentrations influence the generation of REs; even an increase in the RE beam current density is observed at low pressures of helium and other gases [10, 11].
Another way to decouple REs is to enhance runaway transport loss by external magnetic perturbations, which has been extensively studied in many devices. The experimental results for JT-60U showed that the confinement of REs was improved in the case of micromagnetic fluctuations and degraded in macroscale magnetic fluctuations [12]. RE suppression has been found at magnetic fluctuations larger than a certain threshold, which is identified at the start of the current quench (CQ) in TEXTOR tokamak [13]. In the DIII-D tokamak, n = 3 and n = 1 resonant magnetic perturbations (RMPs) were applied before thermal quench (TQ), where n is the number of toroidal modes. The results showed that REs were successfully suppressed, perhaps by altering the nature of the magnetic perturbations [14]. In the ASDEX Upgrade experiment, the application of three-dimensional (3D) fields affected the electron temperature profile and seemingly changed the dynamics of the disruption, resulting in a significantly reduced current and lifetime of the generated RE beam [15]. A systematic study of RE mitigation by magnetic perturbations was conducted using the J-TEXT tokamak. During the intended disruption by the massive argon injection, magnetic fluctuations were observed at the beginning of the CQ, and the generated runaway current was inversely proportional to the level of natural magnetic fluctuations [16]. It was also observed that the runaway current can be fully avoided by applying m/n = 2/1 mode RMP before TQ, where m is the number of poloidal modes. The efficiency of RE suppression is related to the trigger time of RMP, which enlarges the magnetic islands to form stronger stochasticity in the whole plasma cross section during disruption [17, 18]. External RMP coils producing magnetic perturbations may be the simplest and most effective method; however, another way to produce magnetic perturbations has been explored in J-TEXT. Experimental results demonstrate that hydrogen injected by supersonic molecular beam injection during the plasma current flattop phase can lead to magnetic perturbations, which rapidly increase RE loss [19]. At the same time, many theoretical investigations and simulations that focused on RE mitigation have been conducted. The pre-existing m/n = 2/1 magnetic islands have been used in the 3D magnetohydrodynamic (MHD) code called NIMROD to simulate the J-TEXT disruption experiment. The results show that the ratio of the remaining REs is not monotonically dependent on the width of pre-existing 2/1 magnetic islands, and the duration of magnetic fluctuations δB/Bt (exceeding (4–6)×10−3) is key for RE loss [20]. Simulations have also been conducted for a 3D coil in SPARC and suggested that it can prevent runaways [21]. The MARS-F code was used to simulate the mitigation of REs by 3D magnetic perturbations for the ITER 15 MA baseline deuterium–tritium scenario. It was found that the magnetic perturbations applied to the pre-disruption plasma were moderately effective in mitigating the RE seeds in ITER when considering the vacuum field model and related to the eigenmode structure of magnetic fluctuations [22]. The use of magnetic perturbations to mitigate REs has yielded promising results for the existing tokamaks. Future tokamaks that are earlier in the design can incorporate appropriate coils to suppress the RE generation.
Recently, a set of 3D helical coils was designed and installed in the J-TEXT tokamak to optimize tokamak configuration and improve plasma stability. There is an opportunity to investigate the suppression of RE generation in the optimized plasma configuration using 3D helical coils, which may provide a new idea for RE mitigation during major disruptions.
The remainder of this paper is organized as follows. An introduction to the experimental setup is described in section 2. The dependence of the RE generation on the 3D helical field is presented in section 3 and the influence of the 3D helical field on the electron density threshold for RE suppression is also presented in section 3. Finally, section 4 presents the discussion and conclusion.
The J-TEXT tokamak is a conventional iron core tokamak, operated at a major radius R0 = 1.05 m, and minor radius a = 0.25–0.29 m with a movable titanium-carbide-coated graphite limiter. The typical J-TEXT discharge in the limiter configuration was obtained with a toroidal field Bt of ~ 2.0 T, a plasma current Ip of ~ 200 kA, a pulse length of 800 ms, and plasma densities <ne> of (1–7)×1019 m−3. The main studies on J-TEXT focused on the impact of 3D magnetic perturbation fields on magnetic topology, plasma disruptions, and magnetic fluctuations [23, 24].
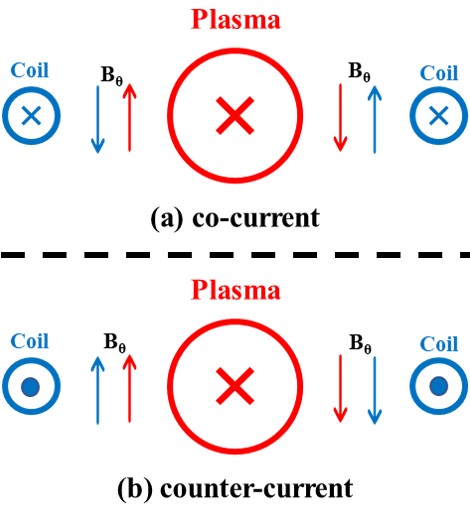
To combine the advantages of the tokamak and the stellarator, a 3D helical coil system was designed and installed in the J-TEXT tokamak to generate an additional rotational transform instead of a partial plasma current to optimize the tokamak configuration and improve plasma stability. The 3D helical coils are called external rotational transform (ERT) coils. The ERT coil system consisted of two closed helical coils, and the helical major radius and minor radius were Rcoil = 1.05 m and rcoil = 0.305 m, respectively. The ERT coil system was chosen as two rings to approximate a helical magnetic field (l = 2 and m = 2, where l and m represent the number of turns in the poloidal and toroidal directions of the ERT coil, respectively) [25]. The designed dominant mode of the ERT coils is m/n = −2/2 nonresonant perturbations, which were obtained from Fourier decomposition in cylindrical coordinates [26, 27]. As shown in figure 1, when the coil current is in the same direction as the plasma current, the magnetic perturbations induced by ERT are called co-magnetic perturbations (co-MPs). Otherwise, magnetic perturbations are called counter-magnetic perturbations (counter-MPs).
In these experiments, disruptions with runaway current were deliberately triggered by the injection of large amounts of argon (Ar) using a fast MGI valve [28]. A set of toroidal and poloidal arrays of Mirnov coils were installed on J-TEXT to measure the magnetic fluctuations and determine the poloidal and toroidal mode numbers [29]. The electron cyclotron emission (ECE) diagnostic at port 9 indicates the onset of disruption by a drop in electron temperature and provides information about the duration of the TQ [30]. Hard X-ray radiation (HXR) from RE-induced thick target bremsstrahlung was measured using NaI and LaBr3 detectors [31]. A three-wave far-infrared laser polarimeter–interferometer system [32] was installed to measure the electron density and the Faraday angle. Soft X-ray (SXR) diagnostics at port 3 with a 1.2 cm spatial resolution were used to observe the cooling process [33].
A systematic study of disruption-generated REs was performed in the J-TEXT tokamak and found that REs can be suppressed when magnetic fluctuations are larger than a certain threshold at the beginning of the CQ [16]. Owing to the 3D helical coils installed in the vacuum chamber, the minor radius of the plasma was reduced to 0.22 m, and the plasma current was adjusted to 70 kA. In this section, first, the relationship between the generated runaway current and magnetic fluctuations without 3D helical magnetic perturbations is given in the parameters. The dependence of RE suppression on 3D helical magnetic perturbations is presented later.
As mentioned, the plasma cross section changed owing to the 3D helical coils. To confirm that magnetic fluctuations can suppress REs under the current discharge conditions, experiments without 3D helical magnetic perturbations were performed as a reference. Two discharges at different toroidal magnetic fields were compared, as shown in figure 2. The toroidal magnetic fields in Nos. 1090840 (blue line) and 1090862 (red dotted–dashed line) are 1.7 and 1.9 T, respectively. The electron density was 2.4×1019 m−3 and larger than the threshold, which REs could not generate during the disruption at 1.7 T. The trigger time of Ar MGI was 0.45 s, and the flight time was approximately 2 ms; therefore, the trigger time of disruption was approximately 0.452 s. When the toroidal magnetic field was 1.9 T, the formed runaway current was approximately 50 kA. The REs have better confinement in the core with a higher toroidal magnetic field, and radial diffusion is a rapidly decreasing function of the toroidal magnetic field [34]. The MHD signals (dB/dt) during 0.451–0.454 s at the low-field side (LFS) and high-field side (HFS) are shown in figures 1(b) and (c), respectively. The amplitudes of the MHD signal at LFS and HFS were slightly larger in No. 1090840 than those in No. 1090862 during the TQ and CQ phases. The magnetic fluctuations (δB) were calculated by filtering the MHD signal with a high-pass filter (> 2 kHz) and integrating it from 0.451 to 0.454 s, which were normalized with Bt in figures 1(d) and (e). It can be seen that the magnetic fluctuations in No. 1090840 at LFS and HFS are larger than those in No. 1090862 during the disruption phases. The same phenomenon can also be seen in the wide distribution of the magnetic fluctuation frequency of the spectrum of the MHD, which is obtained by Fourier transform, as shown in figure 3. The magnetic fluctuations above 30 kHz were more obvious and longer in No. 1090840 than those in No. 1090862, indicating that the enhanced magnetic fluctuations were mainly in the high-frequency band.
Because TQ is too fast, it is difficult to accurately judge from the diagnostic signal. Therefore, the cooling time that included the pre-TQ and TQ phases was used for the analysis. The cooling time was estimated from the beginning of ECE and SXR to a minimum value, which usually occurs when the injected gas reaches the plasma boundary. The SXR and ECE signals were used to indicate the temperature evolution, as shown in figures 4(a) and (b), respectively. The area between the dotted lines in figure 4 represents the cooling phase, during which the temperature began to decrease to a minimum value after Ar was injected. It can be seen that the cooling time was shorter for No. 1090862 than for No. 1090840. During this phase, the peaks of the loop voltages (figure 4(c)) are almost the same, whereas the HXR signal is larger in No. 1090840 than that in No. 1090862. These phenomena may indicate that larger magnetic fluctuations can enhance the REs lost to the wall. The production of Dreicer-generated REs is predominantly determined by the toroidal electric field, which follows a high electric field (loop voltage) and low electron density. The shorter cooling time leads to a decrease in the plasma temperature from kiloelectron volt to approximately several electron volts, and the electrons cool down mainly because of collisions with the plasma. If the collision time is longer than the duration of TQ during the disruption, these energetic electrons form a large number of high-energy REs in the tail of the Maxwell distribution [16, 35]. The shorter cooling time and almost the same loop voltage indicate that the reason for RE generation is the hot-tail mechanism, not the Dreicer mechanism.
The relationship between the magnetic fluctuations and REs with different toroidal magnetic fields is shown in figure 5. With an increase in the toroidal magnetic field, the magnetic fluctuations decreased, whereas the runaway current increased. This trend is consistent with previous results [1, 16], and the validity of the following results was ensured.
The 3D helical magnetic perturbations (m/n = −2/2) induced by ERT coils can optimize the tokamak configuration and improve plasma stability. The influence of the 3D helical magnetic perturbations on RE generation was also explored. As shown in figure 6, three discharges (No. 1090028 (black line), No. 1090836 (blue dotted–dashed line), and No. 1090006 (red dotted–dashed line)) were compared. The plasma parameters were almost the same, the plasma current was 70 kA, the electron densities were in the range of (2–2.2)×1019 m−3, and the toroidal magnetic field was 1.7 T. No. 1090028 was the reference discharge without 3D helical magnetic perturbations. The 3D helical magnetic perturbations induced by the coil current IERT = −5 kA and IERT = +5 kA were applied before the disruption triggered in Nos. 1090836 and 1090006, respectively. The positive sign ‘+’ indicates that the coil current is the same as the plasma current, and the negative sign ‘−’ indicates the opposite direction as the plasma current.
As shown in figure 6, when the disruption was triggered by Ar MGI, a runaway current of approximately 40 kA was formed without 3D helical magnetic perturbations. The runaway current showed little difference when co-MPs were applied, whereas the runaway current disappeared when counter-MPs were applied. Compared with No. 1090028 during 0.451–0.454 s, the levels of MHD signals at LFS (figure 6(b1)) and HFS (figure 6(c1)) are larger in No. 1090836 during the TQ and CQ phases, and the level of magnetic fluctuations is larger at HFS (figure 6(e1)) and has little difference at LFS (figure 6(d1)). The levels of the MHD signal and magnetic fluctuations in No. 1090006 are almost the same as those in No. 1090028 at LHS (figures 6(b2) and (d2)), and even lower at HFS (figures 6(c2) and (e2)). From the frequency spectrum of the MHD signals (figure 7), it can be seen that the spectrum frequency below 30 kHz is more obvious and longer in Nos. 1090028 and 1090006 than that in No. 1090836. When the frequency was above 30 kHz, the level of the frequency spectrum in No. 1090836 became more obvious, whereas in No. 1090006, it became weaker. This may be because counter-MPs can enhance high-frequency magnetic fluctuations, whereas co-MPs have the opposite effect. It seems that high-frequency magnetic fluctuations can enhance RE loss and avoid the formation of runaway current, whereas the effect of low-frequency magnetic fluctuations on RE loss is not significant.
The 3D helical magnetic perturbations influence other parameters during the cooling phase. As shown in figure 8, the cooling time was also obtained from SXR and ECE. When counter-MPs were applied, the cooling process became faster, but the maximum loop voltage was almost the same as that of the reference discharge. When the co-MPs were applied, the cooling process slowed, and the loop voltage had a smaller peak value. This shows that co- and counter-MPs have opposite effects on the plasma parameters during the disruption phase. The change in HXR was more obvious after the cooling phase in No. 1090836, which indicates that the REs were lost to the wall. The similar loop voltage and electron density show that the Dreicer mechanism is not the loss mechanism with counter-MPs, but that a shorter cooling time would enhance the number of high-energy electron tails, which suggests the mechanism of hot-tail RE generation.
The statistical relationship between the runaway current and 3D magnetic perturbations is shown in figure 9, and the levels of the 3D magnetic perturbations are expressed by the coil current (IERT). When the counter-MPs were applied, the runaway current decreased with increasing field strength. It is specifically stated here that one point was very large with IERT = −5 kA, the electron density of this discharge was 1.7×1019 m−3, and those of other discharges were above 2×1019 m−3. REs are more likely to be generated at lower electron densities. It can also be seen that counter-MPs had little effect on the suppression of RE generation.
As mentioned previously, under the same electron density, the larger the toroidal magnetic field, the easier it is to generate REs. The influence of 3D helical magnetic perturbations on the suppression of RE generation with different toroidal magnetic fields was also investigated. As shown in figure 10, the black rectangles represent the reference data without 3D helical magnetic perturbations, and they increase with an increase in the toroidal magnetic field. To better compare the results with and without 3D helical magnetic perturbations, the range of electron densities was wide, as shown in figure 10. The electron densities at 1.7 T are close to 1.7×1019 m−3, which are lower than those shown in figure 5 and need larger magnetic fluctuations to suppress RE generation. When co-MPs were applied, the REs were completely suppressed under 1.7 and 1.9 T, except for low-electron-density discharges. The runaway current did not completely disappear under 2.1 T, but it was also smaller than the reference discharge.
Although there is some uncertainty about the relationship between REs and 3D helical magnetic perturbations owing to the limitation of data quantity, the existing data also show that the effect is indeed credible to a certain extent.
Based on previous studies, complete suppression of REs is required to achieve Rosenbluth electron density [7, 8], which is difficult to reach by MGI. The enhancement of magnetic fluctuations induced by the application of RMP increases the REs loss during disruptions, leading to robust runaway suppression in discharges with a relatively low electron density [36]. According to the above results, counter-MPs can also enhance the magnetic fluctuations to suppress REs, especially high-frequency magnetic fluctuations. The influence of counter-MPs on the electron density threshold for RE suppression was investigated.
Typical results are shown in figure 11, where the plasma current is 70 kA, and the toroidal magnetic field is 2.1 T. The electron densities in Nos. 1090868 (black line) and 1090876 (red dotted–dashed line) are approximately 2.3×1019 m−3. Counter-MPs with IERT = −5 kA were applied before disruption in No. 1090876, and No. 1090868 was the reference discharge. The runaway current was approximately 50 kA in No. 1090868 and disappeared with counter-MPs applied in No. 1090876. This trend is consistent with the 1.7 T result above. No. 1090864 discharge was used to compare with the above discharges, the electron density was increased to 2.7×1019 m−3, and the runaway current disappeared, which shows that the electron density is larger than the threshold of RE suppression. By comparing the magnetic fluctuations from the MHD signals, the magnetic fluctuations in Nos. 1090864 and 1090876 at LFS and HFS are all larger than those in No. 1090868. It is shown that increasing the electron density can enhance the magnetic fluctuations [34], and the applied counter-MPs can increase the magnetic fluctuations to the same amplitude as a higher electron density and suppress RE generation.
The relationships between the electron density and runaway with different counter-MPs at Bt = 1.7 and 2.1 T are shown in figures 12(a) and (b), respectively. The black rectangles represent the discharges without counter-MPs, the blue dots and red positive triangles are applied counter-MPs with IERT = −1 and −5 kA at 1.7 T (figure 12(a)), and the red dots are applied counter-MPs with IERT = −5 kA at 2.1 T (figure 12(b)). In figure 12(a), the electron density threshold of RE suppression without counter-MPs was approximately 2.4×1019 m−3. When counter-MPs with IERT = −1 kA were applied, the electron density threshold was approximately 2×1019 m−3. The discharges with IERT = −5 kA were too few to obtain what the electron density threshold was, but it should be somewhere between 1.8×1019 and 2.2×1019 m−3, which is lower than 2.4×1019 m−3. From figure 12(b) at Bt = 2.1 T, the electron density thresholds were approximately 2.7×1019 and 2.6×1019 m−3 without and with counter-MPs, respectively. Because the level of magnetic fluctuations is a decreasing function of toroidal magnetic fields, the effect of magnetic perturbations on the suppression of RE generation is more significant under a lower toroidal magnetic field. The results show that counter-MPs can decrease the electron density thresholds of RE suppression, which could reduce the challenge of complete suppression of RE generation by increasing the electron density in future large tokamaks.
The ERT can generate an additional rotational transform to optimize the tokamak configuration and improve the plasma stability on J-TEXT. To understand the process of RE generation, the profiles of SXR and ECE are shown in figure 13. There are 14 ports at the midplane on J-TEXT, which are evenly distributed in the toroidal direction. Port 1 was defined as 0°; therefore, the SXR and ECE diagnostics were located at 77° (port 3) and 231° (port 9) in the toroidal direction, respectively. The black line is the reference without 3D helical magnetic perturbations, whereas the blue and red lines represent the applied 3D helical magnetic perturbations with IERT = −5 and +5 kA, respectively. When the co-MPs were applied, the profiles shifted toward the LFS, and the changes in ECE were more obvious in the LFS, whereas the shift toward the HFS when counter-MPs were applied. This indicates that there are some changes in the plasma configuration, and the experimental effect of the rotational transform needs to be confirmed by the results of more toroidal diagnostics.
The magnetic fluctuations during the cooling phase at different poloidal positions are shown in figure 14, where 0° and −180° represent the midplanes of LFS and HFS, respectively. When co-MPs were applied, the magnetic fluctuations slightly increased at LFS and showed no obvious change at HFS. When counter-MPs were applied, the magnetic fluctuations were more obvious at HFS, and there was no significant change at LFS, suggesting that the difference was probably not caused by geometric effects. Both co-MPs and counter-MPs changed the plasma configuration in J-TEXT and enhanced the magnetic fluctuations. However, only counter-MPs significantly suppressed RE generation.
From the synchrotron radiation results of the infrared camera in TEXTOR [37], Alcator C-Mod [38], and J-TEXT [39], REs always appeared on the HFS and were confined to the plasma core region. As shown in figure 13, the plasma profile shifted toward the HFS when counter-MPs were applied, suggesting that REs also moved toward HFS. The magnetic fluctuations were also enhanced at HFS (figure 14), which is more capable of enhancing the loss of REs and suppressing their generation.
From figure 8, we know that REs were suppressed when the cooling time was shorter, but this was different from the hot-tail RE generation without 3D helical magnetic perturbations. The small difference in loop voltage also suggests that it may not be the Dreicer mechanism. From the comparison of the frequency spectra, the counter-MPs can enhance the higher-frequency magnetic fluctuations, and this is dependent on RE suppression. If the population of high-energy REs is sufficiently enhanced, they can interact with high-frequency magnetic fluctuations that have a wide distribution and enhance RE scattering, resulting in the suppression of RE generation [40].
In conclusion, REs generated during disruption cause significant damage to the tokamak and need to be mitigated. A set of 3D helical coils was designed to provide ERT in the J-TEXT tokamak. The designed dominant mode of the 3D helical magnetic perturbations is m/n = −2/2 when two helical coils carry a current in the co- or counter-direction as the toroidal plasma current, which is expected to optimize the plasma configuration and improve stability. To explore the influence of 3D helical magnetic perturbations on the suppression of RE generation, 3D helical magnetic perturbations with co- and counter-currents were applied before disruption, and the disruption was triggered by Ar MGI to obtain a stable runaway current. The results showed that 3D helical magnetic perturbations can suppress RE generation. Experimental evidence indicates that counter-MPs can change the plasma configuration closer to the HFS and enhance the magnetic fluctuations, which enhances the radial loss of RE. At the same time, the cooling time during the disruption phase was decreased, and the population of high-energy REs was enhanced in a shorter cooling time. When the number of REs is sufficiently large, REs can interact with the enhanced frequency magnetic fluctuations, leading to RE suppression, whereas the co-MPs have little influence on the suppression of RE generation.
According to the statistical analysis of RE generation at different electron densities, counter-MPs can decrease the electron density threshold that REs cannot generate. This means that even if the Rosenbluth electron density is not reached, the generation of REs can be suppressed at a lower electron density. This may help with the control of REs on tokamaks.
This study was supported by the National Magnetic Confinement Fusion Energy R&D Program of China (Nos. 2018YFE0309103 and 2019YFE03010004), National Natural Science Foundation of China (Nos. 12475222, 12205122, and 51821005), and Hubei International Science and Technology Cooperation Projects (No. 2022EHB003).
| [1] |
Lehnen M et al 2009 J. Nucl. Mater. 390–391 740 doi: 10.1016/j.jnucmat.2009.01.200
|
| [2] |
Hender T C et al 2007 Nucl. Fusion 47 S128 doi: 10.1088/0029-5515/47/6/S03
|
| [3] |
Boozer A H 2017 Nucl. Fusion 57 056018 doi: 10.1088/1741-4326/aa6355
|
| [4] |
Reux C et al 2010 Nucl. Fusion 50 095006 doi: 10.1088/0029-5515/50/9/095006
|
| [5] |
Lehnen M et al 2011 Nucl. Fusion 51 123010 doi: 10.1088/0029-5515/51/12/123010
|
| [6] |
Commaux N et al 2016 Nucl. Fusion 56 046007 doi: 10.1088/0029-5515/56/4/046007
|
| [7] |
Hollmann E M et al 2015 Phys. Plasmas 22 021802 doi: 10.1063/1.4901251
|
| [8] |
Hollmann E M et al 2019 Phys. Rev. Lett. 122 065001 doi: 10.1103/PhysRevLett.122.065001
|
| [9] |
Wei Y N et al 2020 Plasma Phys. Control. Fusion 62 025002 doi: 10.1088/1361-6587/ab52c8
|
| [10] |
Kozyrev A and Tarasenko V 2024 Plasma 7 201 doi: 10.3390/plasma7010013
|
| [11] |
Baksht E K et al 2006 Tech. Phys. Lett. 32 948 doi: 10.1134/S1063785006110125
|
| [12] |
Yoshino R and Tokuda S 2000 Nucl. Fusion 40 1293 doi: 10.1088/0029-5515/40/7/302
|
| [13] |
Zeng L et al 2013 Phys. Rev. Lett. 110 235003 doi: 10.1103/PhysRevLett.110.235003
|
| [14] |
Commaux N et al 2011 Nucl. Fusion 51 103001 doi: 10.1088/0029-5515/51/10/103001
|
| [15] |
Gobbin M et al 2018 Plasma Phys. Control. Fusion 60 014036 doi: 10.1088/1361-6587/aa90c4
|
| [16] |
Zeng L et al 2017 Nucl. Fusion 57 046001 doi: 10.1088/1741-4326/aa57d9
|
| [17] |
Chen Z Y et al 2018 Nucl. Fusion 58 082002 doi: 10.1088/1741-4326/aab2fc
|
| [18] |
Lin Z F et al 2019 Plasma Phys. Control. Fusion 61 024005 doi: 10.1088/1361-6587/aaf691
|
| [19] |
Huang D W et al 2017 Plasma Phys. Control. Fusion 59 085002 doi: 10.1088/1361-6587/aa717c
|
| [20] |
Li C H et al 2020 Plasma Phys. Control. Fusion 62 095010 doi: 10.1088/1361-6587/aba365
|
| [21] |
Tinguely R A et al 2021 Nucl. Fusion 61 124003 doi: 10.1088/1741-4326/ac31d7
|
| [22] |
Liu Y Q et al 2022 Nucl. Fusion 62 066026 doi: 10.1088/1741-4326/ac5d62
|
| [23] |
Liang Y et al 2019 Nucl. Fusion 59 112016 doi: 10.1088/1741-4326/ab1a72
|
| [24] |
Wang N C et al 2022 Nucl. Fusion 62 042016 doi: 10.1088/1741-4326/ac3aff
|
| [25] |
Li Y B et al 2024 Fusion Eng. Des. 206 114591 doi: 10.1016/j.fusengdes.2024.114591
|
| [26] |
Jiang Z H et al 2022 Plasma Sci. Technol. 24 124014 doi: 10.1088/2058-6272/aca18d
|
| [27] |
Huang J, Suzuki Y and J-TEXT Team 2021 Plasma Fusion Res. 16 2403047 doi: 10.1585/pfr.16.2403047
|
| [28] |
Luo Y H et al 2014 Rev. Sci. Instrum. 85 083504 doi: 10.1063/1.4891864
|
| [29] |
Han D L et al 2021 Plasma Sci. Technol. 23 055104 doi: 10.1088/2058-6272/abeeda
|
| [30] |
Yang Z J et al 2012 Rev. Sci. Instrum. 83 10E313 doi: 10.1063/1.4732069
|
| [31] |
Chen Z Y et al 2012 Rev. Sci. Instrum. 83 056108 doi: 10.1063/1.4721659
|
| [32] |
Chen J et al 2014 Rev. Sci. Instrum. 85 11D303 doi: 10.1063/1.4891603
|
| [33] |
Li J C et al 2014 Rev. Sci. Instrum. 85 11E414 doi: 10.1063/1.4886432
|
| [34] |
Kudyakov T et al 2012 Nucl. Fusion 52 023025 doi: 10.1088/0029-5515/52/2/023025
|
| [35] |
Harvey R W et al 2000 Phys. Plasmas 7 4590 doi: 10.1063/1.1312816
|
| [36] |
Lin Z F et al 2020 Plasma Phys. Control. Fusion 62 025025 doi: 10.1088/1361-6587/ab5b34
|
| [37] |
Finken K H et al 1990 Nucl. Fusion 30 859 doi: 10.1088/0029-5515/30/5/005
|
| [38] |
Hoppe M et al 2018 Nucl. Fusion 58 026032 doi: 10.1088/1741-4326/aa9abb
|
| [39] |
Tong R H et al 2016 Rev. Sci. Instrum. 87 11E113 doi: 10.1063/1.4960311
|
| [40] |
Lvovskiy A et al 2018 Plasma Phys. Control. Fusion 60 124003 doi: 10.1088/1361-6587/aae95a
|
| [1] | Bowen RUAN (阮博文), Zhoujun YANG (杨州军), Xiaoming PAN (潘晓明), Hao ZHOU (周豪), Fengqi CHANG (常风岐), Jing ZHOU (周静). Estimation of magnetic island width by the fluctuations of electron cyclotron emission radiometer on J-TEXT[J]. Plasma Science and Technology, 2019, 21(1): 15102-015102. DOI: 10.1088/2058-6272/aae382 |
| [2] | Yonghua DING (丁永华), Zhongyong CHEN (陈忠勇), Zhipeng CHEN (陈志鹏), Zhoujun YANG (杨州军), Nengchao WANG (王能超), Qiming HU (胡启明), Bo RAO (饶波), Jie CHEN (陈杰), Zhifeng CHENG (程芝峰), Li GAO (高丽), Zhonghe JIANG (江中和), Lu WANG (王璐), Zhijiang WANG (王之江), Xiaoqing ZHANG (张晓卿), Wei ZHENG (郑玮), Ming ZHANG (张明), Ge ZHUANG (庄革), Qingquan YU (虞清泉), Yunfeng LIANG (梁云峰), Kexun YU (于克训), Xiwei HU (胡希伟), Yuan PAN (潘垣), Kenneth William GENTLE, the J-TEXT Team. Overview of the J-TEXT progress on RMP and disruption physics[J]. Plasma Science and Technology, 2018, 20(12): 125101. DOI: 10.1088/2058-6272/aadcfd |
| [3] | Guo XU (徐国), Bo RAO (饶波), Yonghua DING (丁永华), Mao LI (李茂), Da LI (李达), Ruo JIA (贾若), Minxiong YAN (严民雄), Xinke JI (吉新科), Nengchao WANG (王能超), Zhuo HUANG (黄卓), Daojing GUO (郭道靖), Lai PENG (彭莱). Power supply for generating frequency-variable resonant magnetic perturbations on the J-TEXT tokamak[J]. Plasma Science and Technology, 2018, 20(8): 85601-085601. DOI: 10.1088/2058-6272/aabd2f |
| [4] | Donghui XIA (夏冬辉), Fangtai CUI (崔芳泰), Changhai LIU (刘昌海), Zhenxiong YU (余振雄), Yikun JIN (金易坤), Zhijiang WANG (王之江), J-TEXT team. The anode power supply for the ECRH system on the J-TEXT tokamak[J]. Plasma Science and Technology, 2018, 20(1): 14018-014018. DOI: 10.1088/2058-6272/aa936d |
| [5] | Hailong GAO (高海龙), Tao XU (徐涛), Zhongyong CHEN (陈忠勇), Ge ZHUANG (庄革). Plasma equilibrium calculation in J-TEXT tokamak[J]. Plasma Science and Technology, 2017, 19(11): 115101. DOI: 10.1088/2058-6272/aa7f26 |
| [6] | Zhenling ZHAO (赵朕领), Yilun ZHU (朱逸伦), Li TONG (仝丽), Jinlin XIE (谢锦林), Wandong LIU (刘万东), Changxuan YU (俞昌旋), Zhoujun YANG (杨州军), Ge ZHUANG (庄革), N C LUHMANN JR, C W DOMIER. Quasi-3D electron cyclotron emission imaging on J-TEXT[J]. Plasma Science and Technology, 2017, 19(9): 94001-094001. DOI: 10.1088/2058-6272/aa750d |
| [7] | KE Xin (柯新), CHEN Zhipeng (陈志鹏), BA Weigang (巴为刚), SHU Shuangbao (舒双宝), GAO Li (高丽), ZHANG Ming (张明), ZHUANG Ge (庄革). The Construction of Plasma Density Feedback Control System on J-TEXT Tokamak[J]. Plasma Science and Technology, 2016, 18(2): 211-216. DOI: 10.1088/1009-0630/18/2/20 |
| [8] | ZHANG Zepin(张泽品), CHENG Zhifeng(程芝峰), LUO Jian(罗剑), WANG Zhijiang(王之江), ZHANG Xiaolong(张晓龙), HOU Saiying(侯赛英), CHENG Cheng(成诚). Implementation of Automatic Process of Edge Rotation Diagnostic System on J-TEXT Tokamak[J]. Plasma Science and Technology, 2014, 16(8): 789-793. DOI: 10.1088/1009-0630/16/8/10 |
| [9] | A. C. ENGLAND, Z. Y. CHEN, D. C. SEO, J. CHUNG, Y. S. LEEV, J. W. YOO, W. C. KIM, Y. S. BAE, Y. M. JEONV, J. G. KWAK, M. KWON, the KSTAR Tea. Runaway Electron Suppression by ECRH and RMP in KSTAR[J]. Plasma Science and Technology, 2013, 15(2): 119-122. DOI: 10.1088/1009-0630/15/2/08 |
| [10] | HAO Changduana(郝长端), ZHANG Minga(张明), DING Yonghua(丁永华), RAO Boa(饶波), CEN Yishuna(岑义顺), ZHUANG Ge(庄革). Stress and Thermal Analysis of the In-Vessel Resonant Magnetic Perturbation Coils on the J-TEXT Tokamak[J]. Plasma Science and Technology, 2012, 14(1): 83-88. DOI: 10.1088/1009-0630/14/1/18 |