| Citation: | Xiang GU, Gang YIN, Yuejiang SHI, Lili DONG, Yu WANG, Hong ZANG, Yuanming YANG, Huasheng XIE, Jiaqi DONG, Yueng-Kay Martin PENG, Baoshan YUAN, Qingwei YANG, Yunfeng LIANG, Xianming SONG, Minsheng LIU, the EHL-2 Team. Poloidal field system and advanced divertor equilibrium configuration design of the EHL-2 spherical torus[J]. Plasma Science and Technology, 2025, 27(2): 024011. DOI: 10.1088/2058-6272/adae72 |
The EHL-2 (ENN He-Long 2) spherical torus (ST) project focuses on advancing spherical torus technology to address the unique challenges of p-11B fusion, which demands significantly higher ion temperature and heat flux to the divertor plate compared to traditional deuterium-tritium fusion. With a major radius of 1.05 m and a plasma current of 3 MA, the project aims to evaluate and optimize advanced divertor configurations, specifically the Super-X and X-point target (XPT) divertors. The design incorporates an up-down double-null configuration featuring a conventional inner divertor and an XPT outer divertor to effectively reduce the heat flux. The poloidal field (PF) coil system is meticulously optimized to balance engineering constraints with the flexibility in equilibrium configurations. This design is expected to provide a reference equilibrium configuration for other physics design issues and offer critical insight into heat load management.
The objective of the EHL-2 project is to build a spherical torus experimental device with major radius R0 = 1.05 m, plasma current Ip = 3 MA and toroidal field BT = 3 T to address the key physics issues of proton-boron plasmas at ion temperature above 20 keV and density of the order of plasma density 1020 m−3 in the spherical torus [1, 2]. Heat exhaust will be one of the main challenges in the design to control power load and particle recycling.
For p-11B fusion, the required ion temperature (~ 200 keV) is more than an order of magnitude higher than that for deuterium-tritium fusion (~ 20 keV), resulting in more severe heat load issues. In spherical torus devices, the smaller major radius of the divertor strike points further complicates heat load control. Conventional divertor solutions are unlikely to be suitable for commercial proton-boron ST fusion reactors. Therefore, it is necessary to explore divertor configurations that go beyond ITER [3], with significant flux expansion near the strike points. Future proton-boron fusion will be required to operate in the high ion temperature mode, with divertors operating in a fully detached regime at low upstream separatrix densities, utilizing advanced configurations with complex magnetic geometries. Given that p-11B fusion eliminates concerns related to fuel retention, carbon-based materials are proposed as suitable candidates for plasma-facing components, and the use of carbon-based materials supports the achievement of higher performance parameters. However, the heat load tolerance of carbon-based materials is lower than that of tungsten. Given these challenges, it is crucial to begin advanced divertor research with EHL-2 to support more demanding future applications.
The selection of divertor configurations must consider various factors such as physical requirements and engineering constraints. Both the Super-X divertor (SXD) [4] and XPT divertor [5] have significant flux expansion potential. Experiments at MAST-U have already demonstrated that the Super-X configuration can reduce the heat load to one-tenth of that in conventional configurations [6]. SPARC will test the XPT configuration to address key physics questions about advanced divertor geometries and provide important data for the design of advanced divertors for future fusion reactors [7]. STEP optimized the inner-X divertor (IXD) and outer SXD, believing that this combination can significantly enhance the divertor’s heat exhaust capabilities [8–10]. Taking account of the engineering feasibility of the PF coil and vacuum vessel (VV), an advanced XPT configuration has been designed.
Under the preliminary operating parameters and engineering requirements, a set of PF systems and multiple equilibrium configurations have been proposed. EHL-2 will utilize an up-down double-null configuration, featuring a conventional inner divertor and an advanced XPT configuration for the outer divertor. The reference XPT configuration will form two X-points; a main X-point and a secondary X-point near the outer divertor plate. This high-elongation configuration necessitates precise vertical displacement event (VDE) control. The PF system enables flexible equilibrium configurations, allowing for effective heat load management through configuration adjustments and other techniques.
A significant amount of heat flux will enter the outer divertor, with a portion also directed towards the inner divertor. The outer divertor leg employs an advanced XPT configuration, which greatly extends the leg length and increases magnetic flux expansion, aiding heat load management. Although the inner divertor leg receives less heat flux, the smaller radius and drift effects still place considerable pressure on it. Therefore, it is essential to consider methods, such as radiative divertors, to reduce the heat load and achieve fully detached operation under low upstream separatrix density conditions.
The following sections will present the design results considering physical requirements and engineering constraints. This includes a feasible PF system, a reference advanced divertor configuration, and other viable divertor options. Section 2 will introduce the integration between PF and equilibrium, along with the associated physical requirements and engineering constraints. Section 3 will then describe the PF system. Section 4 will describe the reference XPT configuration and other feasible divertor configurations. Section 5 will discuss the remaining issues of the current study and propose future research directions. Finally, we summarize the key results of this study.
The design of the PF system and equilibrium configuration is a complex, multi-disciplinary and multi-solution problem that must balance physical, engineering and control requirements, and limitations. Figure 1 shows the iteration flowchart between physics and engineering, which can also be applied to the design of future devices.
The design of EHL-2 commenced with a system code [11] that provided preliminary target physics parameters, including key geometric aspects of the plasma [1]. Based on parameters, such as plasma current and toroidal field, the designs for the toroidal field (TF) and central solenoid (CS) coils were established. With this foundation as the basis, the equilibrium configuration and PF system were designed, setting the stage for the design of other components such as the VV and divertor. The size of the TF coils is determined by the major radius, aspect ratio, toroidal field, discharge pulse length and TF material limitations. The CS coils are determined by the volt-second requirement (~ 5 Vs) for the plasma current. The concept of the integrated center stack including the CS coil and center part of the TF coils was proposed by Peng et al [12] to resolve the problem of installing the TF and CS conductor in a small space. After the successful application in the first ST device, START [13], the integrated center stack has become the basic engineering configuration applied in a lot of ST devices such as NSTX/NSTX-U [14, 15], MAST/MAST-U [16, 17], ST-40 [18], Globus-M2 [19], etc. EHL-2 is no exception and adopts this configuration.
Placing the PF coils inside the TF coils, being as close to the VV as possible, allows for more flexible control of the plasma shaping and divertor configuration with moderate PF current. The TF coils on EHL-2 are not to be made of superconducting material. While the high-field side central column limits the TF coils, the low-field side TF coils can be expanded at a lower cost. All the PF coils are placed inside the TF coils. For future designs with superconducting TF coils, a combination of internal and external PF coils may be necessary to meet the requirements of advanced divertor configurations. For p-11B plasma, there is no need to allocate space for a blanket, enabling a more compact device design.
Given the established TF and CS coils, the position and size of the PF coil system should be optimized. The size of individual PF coils will directly depend on the required ampere-turns and maximum current density. The goal for the PF system is to use the fewest possible coil combinations and the smallest coil currents to achieve the desired divertor configuration. Specifically, the PF coils must safely carry the required current without exceeding the limits of the copper conductors, and the temperature rise during a single discharge should be minimal. In addition, considerations for cost, space, power supply and inductance are crucial. We determine a conservative size based on the ampere-turns needed to achieve the highest plasma current (3 MA) and an advanced XPT divertor configuration, ensuring flexibility to adjust for different internal inductances. Mechanical loads on the coils must also be considered to keep the PF coils’ mechanical stress within acceptable limits.
In addition to these factors, the PF system needs to consider the following factors. The ports must accommodate diagnostic, heating, and other systems. The electromagnetic force on the coils must be within stress limits. Engineering heat dissipation is managed by limiting the winding length to avoid extended cooling paths. Gaps are left radially between PF1 and PF3, PF2 and PF4 coils for connectivity, and the outer PF coil’s length-to-width ratio is optimized.
In this study, the EHL-2 device features an up-down symmetric plasma with an aspect ratio (A) of less than 1.9, a major radius (RLCFS) of less than 1.1 m, high elongation (κ) greater than 2.2 and triangularity (δ) greater than 0.3. For a spherical torus, the lower the aspect ratio, the higher the achievable βN. The major radius is determined by the toroidal field and aspect ratio, with both the toro idal field and the aspect ratio being inversely proportional to the major radius. As the elongation and triangularity increase, the shape parameter increases, which contributes to achieving a higher βN [20, 21]. To achieve a higher toroidal magnetic field (BT), the TF coil current needs to be maximized. To achieve a smaller aspect ratio, the central column must be as small as possible. The smaller the central column, the less space is available for the TF coils. At a given major radius and aspect ratio, increasing the elongation ratio requires raising the plasma height, which also necessitates taller TF coils. However, with a smaller TF cross-section in the central column, taller TF coils face greater challenges regarding construction, support and temperature rise during experimental operation. Therefore, the TF coils cannot be excessively tall, which limits the achievable plasma elongation ratio. Plasma must be confined, shaped and controlled by magnetic fields produced by currents in the surrounding PF coils to achieve the prescribed XPT configuration.
The equilibrium design for EHL-2 adopts the SE (Shape Editor) code [22], which integrates multiple functions including boundary editing, free boundary equilibrium, fixed boundary equilibrium and discharge waveform design. SE reads key parameter information from Excel, facilitating iterative adjustments of the equilibrium shape with the PF coils, VV and other systems. SE allows for easy boundary editing and adjustment based on critical geometric parameters. The adjusted boundary can then be used for fixing boundary equilibrium, yielding plasma current distributions and PF current ratios that closely match the designed boundary. The free-boundary equilibrium can provide plasma current distributions based on the given total plasma current and PF current combinations, facilitating the exploration of equilibrium configuration under various PF currents. We carry out this study based on the SE program integrated with free-boundary equilibrium, which can be used for integrated optimization of the plasma configuration while providing feasible divertor solutions and compatible PF coil sets. These will lay the foundation for subsequent efforts to define the machine geometry and finalize its 2D layout, and will further include components such as the VV and TF coils, the first wall, divertor structures, power supplies and control systems.
The design primarily focuses on optimizing the equilibrium configuration and the PF system while allowing space for other components. First, we define zones where the PF coils can be placed, ensuring that they meet engineering constraints. Through continuous optimization iterations, the suitable PF coil set and equilibrium configuration were ultimately determined.
Through numerous iterations, the PF system and multiple equilibrium configurations were ultimately finalized. The system consists of 12 PF coils arranged symmetrically (see figure 2).
The PF1&PF2 coils are located at the ends of the CS and are used to form the main X-point of the divertor configuration. These coils have a trapezoidal structure to ensure smooth, non-recessed closed magnetic surfaces on the inner side.
PF3&PF4 are located inside the main X-point to form the divertor, enhancing elongation and triangularity. PF5&PF6 are positioned inside the second X-point, primarily to form the second X-point. These coils can also be omitted, allowing the second X-point to move inside the divertor, ensuring the flexibility of the divertor configuration. PF7&PF8 are located outside the second X-point to form the second X-point. PF5, PF6, PF7 and PF8 should be positioned as far from the main plasma as possible to flexibly control the outer divertor while avoiding impact on the main plasma. However, due to TF coil space constraints, they cannot be placed too far away.
PF9&PF10 are located outside the main X-point to form the main X-point, enhancing elongation and triangularity. They should not be placed too far from the main X-point since this would hinder large triangularity and require larger currents, making plasma control more difficult. They should not be too close either, as this could cause the last closed-flux surface (LCFS) to become recessed. It is strongly preferred that they should be placed outside the VV, even if it sacrifices the structural integrity of the VV.
PF11&PF12 are positioned radially outwards, like PF9&PF10, and should not be placed too close or too far. These coils primarily generate the vertical field and control the radial position of the plasma. A closer distance between PF11&PF12 is more conducive to forming a highly elongated configuration, but other system requirements for window size must also be considered to avoid placing them too close.
Table 1 outlines the parameters of the PF system, including the positions of coil center position (R&Z), sizes (W&H), turns (Nr in R direction, Nz in Z direction and turn for all) and maximum current of single-turn. Since we wanted to design PF1&PF2 with a trapezoidal structure, we set the Nr to 3.6. The maximum current for single-turn coils and other specifications are based on the coil materials provided by engineering. Minor adjustments will be made as needed for manufacturing.
| R (m) | Z (m) | W (m) | H (m) | Nr | Nz | Turn | Imax (kA) | |
| CS | 0.36 | 0 | 0.08 | 4 | 2 | 158 | 305 | 70 |
| PF1 | 0.456 | 1.6 | 0.072 | 0.8 | 3.6 | 40 | 144 | 12 |
| PF2 | 0.456 | −1.6 | 0.072 | 0.8 | 3.6 | 40 | 144 | 12 |
| PF3 | 0.7 | 2.05 | 0.12 | 0.16 | 6 | 8 | 48 | 12 |
| PF4 | 0.7 | −2.05 | 0.12 | 0.16 | 6 | 8 | 48 | 12 |
| PF5 | 0.85 | 2.35 | 0.12 | 0.12 | 6 | 6 | 36 | 12 |
| PF6 | 0.85 | −2.35 | 0.12 | 0.12 | 6 | 6 | 36 | 12 |
| PF7 | 1.85 | 2.35 | 0.12 | 0.12 | 6 | 6 | 36 | 12 |
| PF8 | 1.85 | −2.35 | 0.12 | 0.12 | 6 | 6 | 36 | 12 |
| PF9 | 1.85 | 1.6 | 0.12 | 0.12 | 6 | 6 | 36 | 12 |
| PF10 | 1.85 | −1.6 | 0.12 | 0.12 | 6 | 6 | 36 | 12 |
| PF11 | 2.5 | 0.5 | 0.2 | 0.2 | 10 | 10 | 100 | 12 |
| PF12 | 2.5 | −0.5 | 0.2 | 0.2 | 10 | 10 | 100 | 12 |
Figure 3 shows the zero-field area formed by the PF and CS coils, which indicates that this set of the PF system can provide a large null-field region, which can be used for future plasma discharges.
In an axisymmetric spherical torus, equilibrium also refers to the balance between the plasma’s thermal pressure and magnetic pressure. Fixed boundary mode involves solving for the plasma current and required PF currents given a specific boundary. Free-boundary equilibrium, on the other hand, involves solving for the plasma current distribution given the known plasma current and PF currents.
SE’s free-boundary equilibrium, based on the monotonic polynomial’s [23] current profile model, is used to iteratively refine the equilibrium shape with the PF system.
The free-boundary equilibrium requires that the PF system should be determined first. We explored potential coil position combinations, each of which must meet specific constraints. The primary objective of the divertor configuration is to form a secondary null in the outer divertor, where enhanced power radiation can significantly reduce the heat load by puffing near this secondary null. To meet the heat load requirements of the inner limiter, the inner scrape-off-layer width must exceed 30 mm. In addition, we aim for the configuration to meet the key plasma geometric parameters: plasma major radius between 1.05 m and 1.1 m, plasma elongation greater than 2.2, plasma triangularity greater than 0.3, aspect ratio less than 1.9.
For highly elongated configurations, particular attention must be given to VDE. During the equilibrium and PF system design phase, we do not consider controlling VDE with a passive stabilizing plate (PSP) or in-vessel vertical displacement control coils (IVVDCC), which will be discussed in another paper. The VDE control of high-parameter (Ip = 3 MA) and high-elongation configuration is a challenge. The controllability of VDE has been preliminarily analyzed in the configuration design, but further optimization is needed to simulate the power supply requirements for VDE feedback control with PSP and IVVDCC. A preliminary qualitative assessment of a high-ion-temperature operation scenario of the optimal position and engineering width of PSP has been reported [24]. Instead, we focus on the passive stabilization capabilities of the PF system and the VV. Typically, the vertical stability parameter f=−F′s/F′d > 1.3 and plasma vertical instability growth rate γ=1τpassive1f−1 are used to evaluate the controllability of VDE [25].
The current in a single-turn coil should not be too large (> 12 kA) since excessively high currents can lead to significant temperature increases, extending the interval between discharges. Conversely, the current should not be too small since very low currents pose greater challenges for the power supply and require a larger physical space.
Based on the above method, we have identified a PF system and a range of double-null divertor equilibrium configurations: the reference XPT configuration and the controllability of the XPT configuration; Super-X configurations; conventional configurations. The final achieved divertor configurations are illustrated in figures 4, 8, 9 and 10. Figure 4 shows the reference XPT divertor configuration, figure 8 displays two Super-X configurations where the outer divertor X-point is disconnected, and figure 9 presents the controllability of the XPT configuration that excludes the use of the PF5&PF6 coils and negative current of the PF5&PF6 coils, allowing the outer X-point to enter the divertor region. In this setup, the currents in the PF3&PF4, as well as the PF7&PF8 coils, are nearly at their maximum limits. Figure 10 presents a series of conventional configurations. Due to the limited number of coils, the inner divertor can only form a conventional configuration. To manage the heat load on the inner divertor, special techniques need to be implemented. The positions of the subsequent PF systems can still undergo fine-tuning, with minimal anticipated changes to the reference configuration.
Figure 4 shows the reference equilibrium configuration of EHL-2. This configuration demonstrates extensive magnetic flux expansion and forms a secondary X-point, indicating substantial potential for efficient heat flux control. We opted to extend the outer leg of the divertor upwards instead of outwards. This approach ensures a smooth outermost closed magnetic surface and a longer outer leg. In addition, since the PF5, PF6, PF7 and PF8 coils are situated on either side of the VV, this design does not necessitate an increase in the VV height. All key geometric parameters meet the requirements listed above. Although the elongation is 2.27, the VDE stability parameter fVDE = 1.98 > 1.3 indicates that stability control can be achieved even without PSP. This high-stability parameter is strongly related to the pre-designed VV structure. A VV closer to the plasma is more effective for VDE control. For more stable VDE control, PSP and IVVDCC are still necessary.
Figure 5 presents the plasma boundary from SE benchmarked against EFIT [26]. The inverse mode of EFIT, with the target plasma boundary from SE, finds a similar plasma boundary to the reference. Figure 6 compares the needed PF currents for SE and EFIT, showing negligible deviations. Preliminary electromagnetic force analysis shown in figure 7 indicates that the forces on the coils are within a reasonable range.
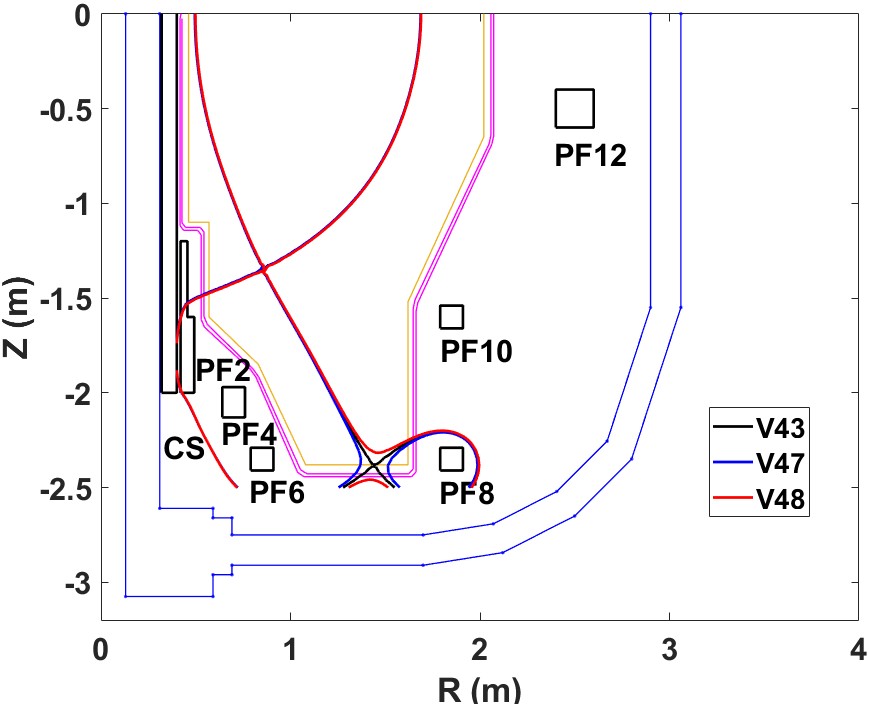
The experiment does not maintain a strictly double-null configuration, and the configuration shown in figure 8 is not strictly realized either; it serves primarily as a control target. When there are deviations in control, the secondary X-point can split into two scenarios, one where the strike point of the outer leg reaches the vertical target plate (red line), and another where the outer leg strikes the horizontal target plate (blue line).
In the reference configuration, the secondary X-point is located on the VV, and considering the space required for the divertor, the secondary X-point will be outside the divertor chamber. To move the secondary X-point inside the divertor, we can either exclude PF5&PF6 or design PF5&PF6 to carry a negative current. Figure 9 shows two configurations without using PF5&PF6 and with negative current of PF5&PF6, resulting in the secondary X-point being within the VV and divertor. In future experiments, the radiation from the secondary X-point can be utilized to significantly reduce the heat load. This configuration places higher current demands on PF3&PF4, as well as PF7&PF8.
Figure 10 shows the conventional divertor configurations, which require lower currents in PF7–10. As we continuously reduce the current in PF7–10 while maintaining the scrape-off-layer width greater than 30 mm, the strike point of the divertor’s outer leg will keep moving inwards, and the plasma major radius will keep increasing. However, this configuration poses significant challenges for controlling the heat load on the outer target plate.
| Ip (MA) | A | a (m) | RLCFS (m) | κ | δ | Rmag (m) | ΔRSOL (mm) | S (m2) | V (m3) | q95 | fVDE | γ (1/s) | W (MJ) | |
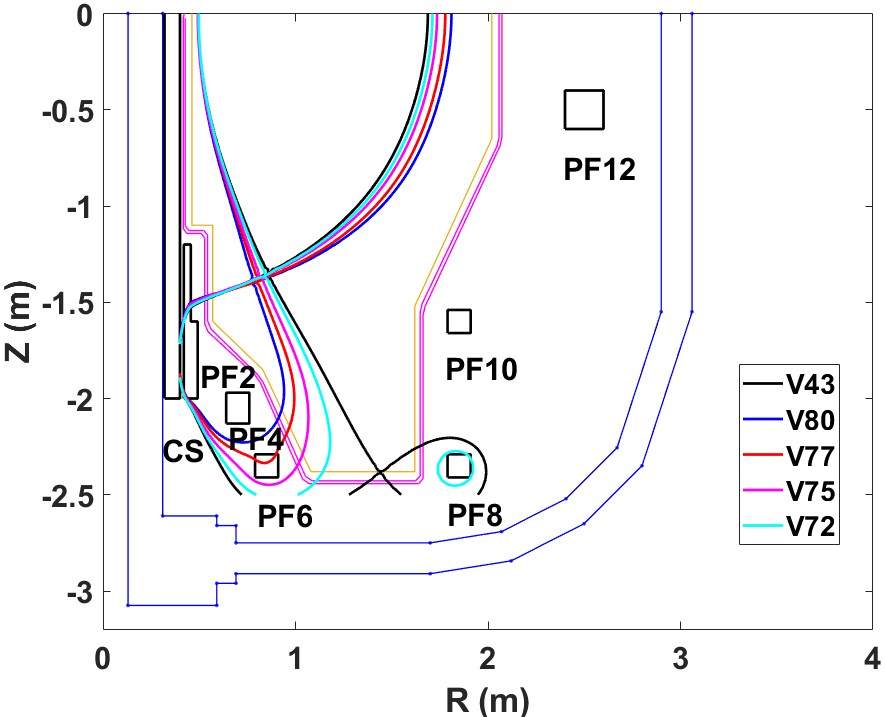
| V43 | 3 | 1.83 | 0.6 | 1.09 | 2.26 | 0.4 | 1.24 | 35 | 2.25 | 15 | 6.59 | 1.98 | 160 | 3.27 |
| V45 | 3 | 1.84 | 0.59 | 1.08 | 2.28 | 0.39 | 1.23 | 32 | 2.23 | 14.8 | 6.57 | 1.87 | 184 | 3.23 |
| V47 | 3 | 1.83 | 0.6 | 1.09 | 2.26 | 0.4 | 1.24 | 33 | 2.25 | 15 | 6.6 | 1.98 | 160 | 3.27 |
| V48 | 3 | 1.84 | 0.6 | 1.09 | 2.26 | 0.39 | 1.24 | 36 | 2.25 | 15 | 6.6 | 1.99 | 159 | 3.28 |
| V64 | 3 | 1.88 | 0.57 | 1.07 | 2.35 | 0.36 | 1.21 | 41 | 2.15 | 14.2 | 6.46 | 1.59 | 285 | 3.14 |
| V72 | 3 | 1.82 | 0.61 | 1.1 | 2.23 | 0.43 | 1.26 | 35 | 2.32 | 15.7 | 6.65 | 2.19 | 127 | 3.35 |
| V75 | 3 | 1.79 | 0.62 | 1.11b | 2.2 | 0.47 | 1.27 | 30 | 2.41 | 16.4 | 7.01 | 2.42 | 102 | 3.38 |
| V77 | 3 | 1.77 | 0.64 | 1.14b | 2.16b | 0.52 | 1.3 | 34 | 2.54 | 17.6 | 7.23 | 2.78 | 75 | 3.48 |
| V80 | 3 | 1.75 | 0.66 | 1.15b | 2.12b | 0.56 | 1.32 | 31 | 2.65 | 18.5 | 7.54 | 3.11 | 59 | 3.54 |
The parameters of the above configurations all meet the requirements of geometric parameters, and the parameters of the main plasma do not differ much. The number with a superscript “b” indicates that the limit value has been exceeded.
Figure 11 shows that the elongation ranges from 2.12 to 2.35, the aspect ratio from 1.75 to 1.88 and the triangularity from 0.36 to 0.56, with the major radius spanning 1.07 to 1.15. This trend suggests that increasing both the elongation and aspect ratios leads to a decrease in the major radius and triangularity.
We have designed the PF system and multiple equilibrium configurations considering physical requirements and engineering limitations. These configurations are designed for plasma current flat-top phase, with PF currents satisfying the demands. However, further optimization and assessment are required to ensure that the PF currents remain adequate throughout the entire plasma current ramp-up phase. Currently, there is no specific plan for achieving the XPT configuration. However, we can refer to the iso-flux control combined with feedforward strategies employed in MAST-U [27]. In the future, if we develop capabilities in AI-based magnetic control [28], we may also explore its application.
Obviously, it is difficult to achieve a plasma current of 3 MA in EHL-2 by CS alone. Non-inductive current drive is one of the key physics issues for EHL-2. The strategy and physics design for current drive are reported in [29].
Detailed heat exhaust capability of this advanced divertor configuration is reported in the divertor physics design [30]. The equilibrium configuration will be iterated based on the results obtained.
All the equilibria mentioned above are based on the monotonic polynomial’s current profile model. Current profiles from heating and current drive [29] and transport simulation [31] will be employed for future iteration. These profiles are expected to facilitate more accurate MHD analyses, leading to more realistic results. Consequently, the physics design of EHL-2 will evolve iteratively, informed by the effects and constraints from heating and current drive, transport, MHD and equilibrium, and aim at achieving the specific physics objectives of EHL-2.
Finally, it is necessary to note that the monotonic polynomial’s current profile model is yet to be verified by the plasma profiles observed on EXL-50U [32]. Improvements to the plasma equilibrium model will continue in the next phase of research.
The EHL-2 project aims to advance spherical torus technology for proton-boron fusion and explore advanced divertor configurations. Key focusing areas include the Super-X and XPT configurations, with the former already showing promise in reducing heat load and the latter to be tested for future applications. Our design incorporates an up-down double-null configuration with a conventional inner divertor and an advanced XPT outer divertor, allowing for effective heat load management. The PF system is carefully optimized to meet engineering constraints while providing flexibility in equilibrium configurations. The EHL-2 device is expected to provide valuable insight into managing high-heat fluxes and the development of future fusion reactors.
This study was supported by the ENN Group and the ENN Energy Research Institute. The authors gratefully acknowledge the invaluable contributions of the ENN fusion team and collaborators in making this project possible. We also thank Xiao Song for the validation work done with EFIT, and Dr Qingquan Yu for helpful discussion.
| [1] |
Liu M S et al 2024 Phys. Plasmas 31 062507 doi: 10.1063/5.0199112
|
| [2] |
Liang Y F et al 2024 Plasma Sci. Technol. in press (https://doi.org/10.1088/2058-6272/ad981a
|
| [3] |
Aymar R, Barabaschi P and Shimomura Y 2002 Plasma Phys. Control. Fusion 44 519 doi: 10.1088/0741-3335/44/5/304
|
| [4] |
Valanju P M et al 2009 Phys. Plasmas 16 056110 doi: 10.1063/1.3110984
|
| [5] |
LaBombard B et al 2015 Nucl. Fusion 55 053020 doi: 10.1088/0029-5515/55/5/053020
|
| [6] |
Fil A et al 2022 Nucl. Fusion 62 096026 doi: 10.1088/1741-4326/ac81d8
|
| [7] |
Kuang A Q et al 2020 J. Plasma Phys. 86 865860505 doi: 10.1017/S0022377820001117
|
| [8] |
Hudoba A et al 2023 Nucl. Mater. Energy 35 101410 doi: 10.1016/j.nme.2023.101410
|
| [9] |
Hudoba A et al 2024 Nucl. Fusion 64 086055 doi: 10.1088/1741-4326/ad53e2
|
| [10] |
Hudoba A et al 2023 Fusion Eng. Des. 191 113704 doi: 10.1016/j.fusengdes.2023.113704
|
| [11] |
Cai J Q et al 2022 Fusion Sci. Technol. 78 149 doi: 10.1080/15361055.2021.1964309
|
| [12] |
Peng Y K M et al 1990 In: Proceedings of the 16th Symposium on Fusion Technology 1990 https://www.osti.gov/biblio/1022679
|
| [13] |
Sykes A et al 1992 Nucl. Fusion 32 694 doi: 10.1088/0029-5515/32/4/I16
|
| [14] |
Peng Y K M and Strickler D J 1986 Nucl. Fusion 26 769 doi: 10.1088/0029-5515/26/6/005
|
| [15] |
Menard J E et al 2012 Nucl. Fusion 52 083015 doi: 10.1088/0029-5515/52/8/083015
|
| [16] |
Darke A C et al 1995 MAST: a Mega Amp Spherical Tokamak Netherlands
|
| [17] |
Harrison J R et al 2019 Nucl. Fusion 59 112011 doi: 10.1088/1741-4326/ab121c
|
| [18] |
Gryaznevich M P and Tokamak Energy Team 2019 AIP Conf. Proc. 2179 020008 doi: 10.1063/1.5135481
|
| [19] |
Panek R et al 2017 Fusion Eng. Des. 123 11 doi: 10.1016/j.fusengdes.2017.03.002
|
| [20] |
Wade M R et al 2001 Phys. Plasmas 8 2208 doi: 10.1063/1.1355980
|
| [21] |
Matsukawa M et al 2006 IEEE Trans. Appl. Supercond. 16 914 doi: 10.1109/TASC.2006.873273
|
| [22] |
Song X M et al 2019 Fusion Eng. Des. 147 111254 doi: 10.1016/j.fusengdes.2019.111254
|
| [23] |
Song X et al 2024 Plasma 7(4) 842 doi: 10.3390/plasma7040045
|
| [24] |
Dong L L et al 2024 Plasma Sci. Technol. in press (https://doi.org/10.1088/2058-6272/ada421
|
| [25] |
Leuer J A 1989 Fusion Technol. 15 489 doi: 10.13182/FST89-A39747
|
| [26] |
Lao L L et al 1985 Nucl. Fusion 25 1611 doi: 10.1088/0029-5515/25/11/007
|
| [27] |
Anand H et al 2024 Nucl. Fusion 64 086051 doi: 10.1088/1741-4326/ad5c80
|
| [28] |
Degrave J et al 2022 Nature 602 414 doi: 10.1038/s41586-021-04301-9
|
| [29] |
Jiang X C et al 2025 Plasma Sci. Technol. in press (https://doi.org/10.1088/2058-6272/adae71
|
| [30] |
Wang F Q et al 2025 Plasma Sci. Technol. in press (https://doi.org/10.1088/2058-6272/adadb8
|
| [31] |
Wang X Y et al 2025 Plasma Sci. Technol. in press (https://doi.org/10.1088/2058-6272/ada9c3
|
| [32] |
Shi Y J et al 2024 Plasma Sci. Technol. in press (https://doi.org/10.1088/2058-6272/ad9e8f
|
| [1] | Xinhao JIANG, Youwen SUN. FEQ: a new flux coordinates based equilibrium solver including both magnetic axis and separatrix[J]. Plasma Science and Technology, 2022, 24(1): 015105. DOI: 10.1088/2058-6272/ac363e |
| [2] | S PUROHIT, Y SUZUKI, S OHDACHI, S YAMAMOTO. Soft x-ray tomographic reconstruction of Heliotron J plasma for the study of magnetohydrodynamic equilibrium and stability[J]. Plasma Science and Technology, 2019, 21(6): 65102-065102. DOI: 10.1088/2058-6272/ab0846 |
| [3] | Qi WANG (汪启), Qingquan YANG (杨清泉), Zhengmao SHENG (盛正卯), Guosheng XU (徐国盛), Jinping QIAN (钱金平), Ning YAN (颜宁), Yifeng WANG (王一丰), Heng ZHANG (张恒). Dependence of the internal inductance on the radial distance between the primary and secondary X-point surfaces in the EAST tokamak[J]. Plasma Science and Technology, 2018, 20(10): 105101. DOI: 10.1088/2058-6272/aad326 |
| [4] | Hailong GAO (高海龙), Tao XU (徐涛), Zhongyong CHEN (陈忠勇), Ge ZHUANG (庄革). Plasma equilibrium calculation in J-TEXT tokamak[J]. Plasma Science and Technology, 2017, 19(11): 115101. DOI: 10.1088/2058-6272/aa7f26 |
| [5] | Yong LU (卢勇), Lijun CAI (蔡立君), Yuxiang LIU (刘雨祥), Jian LIU (刘健), Yinglong YUAN (袁应龙), Guoyao ZHENG (郑国尧), Dequan LIU (刘德权). Thermal-hydraulic analysis of the HL-2M divertor using an homogeneous equilibrium model[J]. Plasma Science and Technology, 2017, 19(9): 95601-095601. DOI: 10.1088/2058-6272/aa7628 |
| [6] | ZHANG Fan (张帆), XU Tao (徐涛), SUN Xinfeng (孙新锋), ZHANG Xiaoqing (张晓卿), ZHUANG Ge (庄革), JIAN Xiang (简翔). Equilibrium Reconstruction and Its Integration in J-TEXT[J]. Plasma Science and Technology, 2016, 18(7): 786-790. DOI: 10.1088/1009-0630/18/7/15 |
| [7] | LUO Bing (罗兵), LUO Zhengping (罗正平), XIAO Bingjia (肖炳甲), YOU Wei (尤玮), TAN Mingsheng (谭名昇), GUO Yong (郭勇), BAI Wei (白伟), MAO Wenzhe (毛文哲), LI Hong (李弘), LIU Adi (刘阿娣), LAN Tao (兰涛), XIE Jinlin (谢锦林), LIU Wandong (刘万东). Capability Assessment of the Equilibrium Field System in KTX[J]. Plasma Science and Technology, 2016, 18(1): 90-96. DOI: 10.1088/1009-0630/18/1/16 |
| [8] | WANG Fumin (王福敏), GAN Kaifu (甘开福), GONG Xianzu (龚先祖), EAST team. Temperature Distribution and Heat Flux on the EAST Divertor Targets in H-Mode[J]. Plasma Science and Technology, 2013, 15(3): 225-229. DOI: 10.1088/1009-0630/15/3/07 |
| [9] | LI Li(李莉), LIU Yue (刘悦), XU Xinyang(许欣洋), XIA Xinnian(夏新念). The Effect of Equilibrium Current Profiles on MHD Instabilities in Tokamaks[J]. Plasma Science and Technology, 2012, 14(1): 14-19. DOI: 10.1088/1009-0630/14/1/04 |
| [10] | HE Zhixiong, DONG Jiaqi, HE Hongda, JIANG Haibin, GAO Zhe, ZHANG Jinhua. MHD Equilibrium Configuration Reconstructions for HL-2A Tokamak[J]. Plasma Science and Technology, 2011, 13(4): 424-430. |
| 1. | Sun, T., Jiang, X., Li, Z. et al. Characterization of fast ion loss in the EHL-2 spherical torus. Plasma Science and Technology, 2025, 27(2): 024002. DOI:10.1088/2058-6272/ad8dfb |
| 2. | Wang, F., Gu, X., Hua, J. et al. Divertor heat flux challenge and mitigation in the EHL-2 spherical torus. Plasma Science and Technology, 2025, 27(2): 024009. DOI:10.1088/2058-6272/adadb8 |
| 3. | Jiang, X., Shi, Y., Song, S. et al. Physics design of current drive and strategy of heating system for EHL-2 spherical torus. Plasma Science and Technology, 2025, 27(2): 024012. DOI:10.1088/2058-6272/adae71 |
| 4. | Wang, Y., Li, K., Huang, Z. et al. Predictions of H-mode access and edge pedestal instability in the EHL-2 spherical torus. Plasma Science and Technology, 2025, 27(2): 024005. DOI:10.1088/2058-6272/ad9f27 |
| 5. | Shi, Y., Song, X., Guo, D. et al. Strategy and experimental progress of the EXL-50U spherical torus in support of the EHL-2 project. Plasma Science and Technology, 2025, 27(2): 024003. DOI:10.1088/2058-6272/ad9e8f |
| 6. | Li, Z., Sun, T., Liu, B. et al. Evaluation of thermal and beam-thermal p-11B fusion reactions in the EHL-2 spherical torus. Plasma Science and Technology, 2025, 27(2): 024004. DOI:10.1088/2058-6272/ad9da2 |
| 7. | Dong, L., Li, L., Liu, W. et al. Instabilities of ideal magnetohydrodynamics mode and neoclassical tearing mode stabilization by electron cyclotron current drive for EHL-2 spherical torus. Plasma Science and Technology, 2025, 27(2): 024006. DOI:10.1088/2058-6272/ada421 |
| R (m) | Z (m) | W (m) | H (m) | Nr | Nz | Turn | Imax (kA) | |
| CS | 0.36 | 0 | 0.08 | 4 | 2 | 158 | 305 | 70 |
| PF1 | 0.456 | 1.6 | 0.072 | 0.8 | 3.6 | 40 | 144 | 12 |
| PF2 | 0.456 | −1.6 | 0.072 | 0.8 | 3.6 | 40 | 144 | 12 |
| PF3 | 0.7 | 2.05 | 0.12 | 0.16 | 6 | 8 | 48 | 12 |
| PF4 | 0.7 | −2.05 | 0.12 | 0.16 | 6 | 8 | 48 | 12 |
| PF5 | 0.85 | 2.35 | 0.12 | 0.12 | 6 | 6 | 36 | 12 |
| PF6 | 0.85 | −2.35 | 0.12 | 0.12 | 6 | 6 | 36 | 12 |
| PF7 | 1.85 | 2.35 | 0.12 | 0.12 | 6 | 6 | 36 | 12 |
| PF8 | 1.85 | −2.35 | 0.12 | 0.12 | 6 | 6 | 36 | 12 |
| PF9 | 1.85 | 1.6 | 0.12 | 0.12 | 6 | 6 | 36 | 12 |
| PF10 | 1.85 | −1.6 | 0.12 | 0.12 | 6 | 6 | 36 | 12 |
| PF11 | 2.5 | 0.5 | 0.2 | 0.2 | 10 | 10 | 100 | 12 |
| PF12 | 2.5 | −0.5 | 0.2 | 0.2 | 10 | 10 | 100 | 12 |
| Ip (MA) | A | a (m) | RLCFS (m) | κ | δ | Rmag (m) | ΔRSOL (mm) | S (m2) | V (m3) | q95 | fVDE | γ (1/s) | W (MJ) | |
| V43 | 3 | 1.83 | 0.6 | 1.09 | 2.26 | 0.4 | 1.24 | 35 | 2.25 | 15 | 6.59 | 1.98 | 160 | 3.27 |
| V45 | 3 | 1.84 | 0.59 | 1.08 | 2.28 | 0.39 | 1.23 | 32 | 2.23 | 14.8 | 6.57 | 1.87 | 184 | 3.23 |
| V47 | 3 | 1.83 | 0.6 | 1.09 | 2.26 | 0.4 | 1.24 | 33 | 2.25 | 15 | 6.6 | 1.98 | 160 | 3.27 |
| V48 | 3 | 1.84 | 0.6 | 1.09 | 2.26 | 0.39 | 1.24 | 36 | 2.25 | 15 | 6.6 | 1.99 | 159 | 3.28 |
| V64 | 3 | 1.88 | 0.57 | 1.07 | 2.35 | 0.36 | 1.21 | 41 | 2.15 | 14.2 | 6.46 | 1.59 | 285 | 3.14 |
| V72 | 3 | 1.82 | 0.61 | 1.1 | 2.23 | 0.43 | 1.26 | 35 | 2.32 | 15.7 | 6.65 | 2.19 | 127 | 3.35 |
| V75 | 3 | 1.79 | 0.62 | 1.11b | 2.2 | 0.47 | 1.27 | 30 | 2.41 | 16.4 | 7.01 | 2.42 | 102 | 3.38 |
| V77 | 3 | 1.77 | 0.64 | 1.14b | 2.16b | 0.52 | 1.3 | 34 | 2.54 | 17.6 | 7.23 | 2.78 | 75 | 3.48 |
| V80 | 3 | 1.75 | 0.66 | 1.15b | 2.12b | 0.56 | 1.32 | 31 | 2.65 | 18.5 | 7.54 | 3.11 | 59 | 3.54 |