High energy electron beam generation during interaction of a laser accelerated proton beam with a gas-discharge plasma

1.   Introduction

The proton beams have a broad range of prospective applications such as high energy density physics (HEDP) [1], proton photography [2], radiotherapy for cancer [3] and fast ignition of inertial confinement fusion [4, 5]. An intense ion beam can provide isochoric-heating of samples and produce large-scale and uniform high energy density matter, which provides a new way for the study of HEDP. For HEDP applications, the interactions between ion beams and plasmas are important, in which the beam energy deposition [6], focusing [7] and instability excitation [8, 9] are the main issues.

A high energy and density proton beam propagating through plasmas can excite ultra-high collective field [10] with a magnitude of GV/m to TV/m. The beam energy can be transferred to the plasma through this field, leading to plasma heating [1] and electron acceleration. In addition, plasma can effectively neutralize the space charge of the ion beam, which is often used to solve the divergence problem in the process of beam transport. In both beam energy deposition and focusing, the beam-plasma interactions are accompanied by many instabilities [11], among which the two-stream instability is the fastest one. Research work showed that the two-stream instability has important impacts on beam transport and may lead to beam defocusing [12]. The generation of high-energy electrons during the interaction of a low-energy ion beam with plasma had been studied with a one-dimensional (1D) electrostatic kinetic simulation [13]. Recently, the collective energy-spectrum broadening of a proton beam at 270 keV due to the two-stream instability excitation was observed experimentally [14].

There are generally two ways for proton beam generation, i.e. conventional rf accelerators and laser-plasma interactions. Over the past decades, laser-driven ion acceleration received wide attention for the extremely high acceleration gradient [15, 16], and the produced proton beams are shown to have exceptional properties, such as high intensity, low emittance, and short pulse duration [17, 18]. Many efficient approaches had been proposed, such as target normal sheath acceleration (TNSA) [19, 20] and radiation pressure acceleration (RPA) [21]. Among them, TNSA can produce proton beams with energy up to 85 MeV [22] or even 100 MeV [23] in the experiment. For the RPA scheme, it is theoretically possible to obtain protons with relatively high energy by light pressure, and the proton beam with an energy of tens of GeV can be obtained by this scheme as indicated in the simulations [24–26].

In recent years, with the rapid development of laser technology, the interaction between petawatt-level laser and plasmas can produce high-energy γ rays and proton beams [27–29]. Compared to the beams produced from conventional accelerators, the laser-driven proton beams have higher energy and beam density, but shorter pulse duration, which is very suitable for the investigations of collective beam-plasma interactions. In the work by Ren et al [10], the energy deposition of a laser-accelerated intense proton beam in a high-density plasma was measured, and the strong collective beam stopping due to plasma return current was observed. Up to the present, little work was performed to investigate the interactions between the laser-accelerated proton beams and low-density gas-discharge plasmas. It should be noted that the properties of gas-discharge plasmas are stable and controllable compared to the plasma produced by laser-solid interactions. In addition, a gas-discharge plasma can be provided as a focusing element for the laser-driven proton beams before the interaction target. In this work, we investigate the propagation of a laser-accelerated proton beam in a low-density gas-discharge plasma, with special attention paid to the generation of high-energy plasma electrons. It is shown that after the saturation stage of the two-stream instability between beam protons and plasma electrons, significant high-energy electrons are formed with maximum energy approaching 2 MeV. These high-energy electrons can obtain velocities higher than that of beam protons and travel to the front of the beam, leading to a new instability between the high-energy electrons and background electrons. The paper is organized as follows: in section 2 the simulation setup is described, and the results are analyzed in section 3. Finally, we give a summary in section 4.

2.   Simulation setup

We perform here 1D collisionless particle-in-cell (PIC) simulations using the electromagnetic kinetic code EPOCH [30]. The beam propagates along the x-axis, which is the direction of the simulation regions. With 1D simulations, the beam focusing effects and the transverse current filamentation can be eliminated, with attention paid to the two-stream instability. The length of the moving simulation regions is 1mm, with a cell size of 0.1 μm and 100 particles per cell for each species. For the parameters in our 1D simulation, the Debye length λ_D = 0.033 μm, the skin depth δ = 11.89 μm and the plasma wavelength λ = 2πv_b/ω_pe = 37.36 μm. The simulation grid size can resolve the plasma wavelength and skin depth, but cannot resolve the Debye length. In the simulations, a moving window approach is adopted and the new fresh plasma ions and electrons are injected continuously into the simulation regions. Therefore, the problem of plasma numerical heating caused by unresolved Debye length can be ignored. A high-voltage gas-discharge hydrogen plasma is considered [31, 32], with ion mass m_i = 1836m_e and charge Z_i = e, where m_e is the electron mass. The plasma temperature and density are set to be T_e = 4 eV and n_e0 = 2.0 × 10²³ m^-3, respectively. The proton beam used in the simulation can be generated by the laser acceleration mechanism. The initial beam energy is set to be E_b0 = 145.3 MeV, and the beam density profile is Gaussian distribution with a peak density n_b0 = 0.25n_e0 and a duration of 0.83 ps (FWHM).

3.   Results

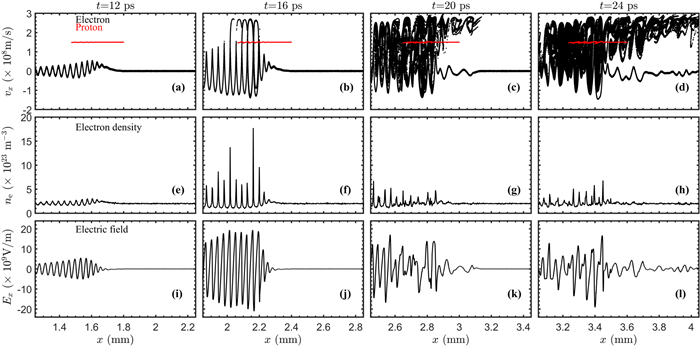
Figure 1 shows the time evolutions of (a)–(d) phase space x - v_x distributions of beam protons (red dots) and plasma electrons (black dots), (e)–(h) plasma electron density n_e and (i)–(l) longitudinal electric field E_x, indicating the growing process of the two-stream instability. The unstable wave produced by two-stream instability can grow and eventually reach saturation by trapping either plasma electrons or beam protons [33]. For a collisionless plasma and monoenergetic beam in 1D simulation, the initial growth rate [8, 14] of the instability is $\gamma {/\omega }_{\mathrm{pe}}=0.7{\left({\displaystyle \frac{n_{\mathrm{b}}}{n_{\mathrm{e}}}}·{\displaystyle \frac{m_{\mathrm{e}}}{m_{\mathrm{b}}}}\right)}^{1/3}\approx 4.5\times {10}^{-2}$ (n_b, n_e, ω_pe being the proton beam density, plasma electrons density and plasma frequency) in this work. When the plasma length reaches the plasma characteristic length L_c = v_bγ^-1 ≈ 133 μm, the two-stream instability can develop [8]. With a propagation distance of 1.6 mm, the instability is expected to increase significantly and the magnitude of longitudinal wakefield reaches 5 × 10⁹ V m^-1, as indicated in figure 1(i) for the travel time t = 12 ps. In this work, we have ${\left[(m_{\mathrm{b}}/m_{\mathrm{e}}){\left(n_{\mathrm{b}}/n_{\mathrm{e}}\right)}^2\right]}^{1/3}\approx 8$ and the saturation of the two-stream instability is caused by plasma electron trapping [33] (see figure 1(b) for the travel time t = 16 ps), in which the electron velocity modulation exceeds levels of proton beam velocity. The magnitude of the longitudinal electric field further increases to 2 × 10¹⁰ V m^-1 and the density of plasma electrons is strongly modulated, with the maximum density approaching 1.8 × 10²⁴ m^-3, 9 times higher than the initial value.

Figure  1.  Time evolutions of (a)–(d) phase space x–v_x distributions of beam protons (red dots) and plasma electrons (black dots), (e)–(h) plasma electron density n_e, and (i)–(l) longitudinal electric field E_x.

DownLoad: Full-Size Img PowerPoint

The formation of high-energy electrons can be observed at t ≈ 20 ps (figure 1(c)), and becomes significant at t ≈ 24 ps (figure 1(d) and x > 3.5 mm). The high-energy electrons can obtain velocities higher than that of beam protons and travel to the front of the beam. Due to the relative streaming between the energetic beam electrons and background electrons, a new two-stream instability occurs. In figures 1(d) and (l) with t = 24 ps, one can see the oscillations of the electron density n_e and longitudinal E_x at the beam front regions (i.e. x ≥ 3.6 mm), indicating the new two-stream instability excitation. The growth rate of this new two-stream instability is ${\gamma }_{\mathrm{s}}/{\omega }_{\mathrm{pe}}\approx \sqrt[3]{n_{\mathrm{s}}/n_{\mathrm{e}}}$ (n_s being the high energy electrons density), this instability (due to electron–electron interactions) develops much faster than the old one.

In order to show the generation process of high-energy electrons clearly, we use the EPOCH program to track the trajectories of plasma electrons, with three typical ones (marked as e1, e2, and e3) shown in figures 2 and 3. In both figures, the position of the beam head is set to be x – v_bt = 0 for clarity. The temporal-spatial distributions of the longitudinal electric field E_x are also displayed in figure 2 for illustration. In the beam frame, ξ = x - v_bt, e1 and e2 move to the left side of the simulation region and are trapped by the wakefield for a few cycles, as indicated in figure 2 at the time interval 16 ps < t < 18 ps and the oscillations in figure 3 at the beam tail regions. With the wave breaking at time t > 18 ps, the electric field is strongly modulated and becomes incoherent, the two electrons escape to the front of the proton beam. It should be noted that e1 oscillates many times at different positions in the front of the proton beam, which is obviously due to the new two-stream instability. In this stage, the electron e1 is decelerated and loses significant energy. The electron e3 moves into the beam regions after the wave breaking and is purely reflected by the wakefield. After accelerated ahead of the proton beam, this electron is accelerated during the stage of the new two-stream instability, as indicated clearly in figure 3 for e3 at x - v_bt > 0.

Figure  2.  Trajectories of three plasma electrons marked as e1, e2 and e3. The background plot is the spatial and temporal distribution of the electric field E_x. In the figure, the position of the beam head is set to be x - v_bt = 0 for clarity.

DownLoad: Full-Size Img PowerPoint

Figure  3.  Trajectories of three plasma electrons marked as e1, e2 and e3 in phase space x - p_x. In the figure, the position of the beam head is set to be x - v_bt = 0 for clarity.

DownLoad: Full-Size Img PowerPoint

The high energy electrons generation can also be identified from the plasma electron momentum distribution function (MDF) and the energy distribution function of high energy electrons (EDF), as shown in figures 4(a) and (b). As the beam travel distance increases, the formation of high-energy beam electrons can be observed from the asymmetric distribution of MDF. According to the previous analysis, the two-stream instability becomes saturated after t ≈ 16 ps. From electron MDF, the high-energy tails (high energy electrons) increase significantly after the instability saturation. The significant difference in the high-energy tails of MDF between the time t = 8 ps and 24 ps can be observed from the figure. The number of the high energy electron beam increases until the time t = 32 ps, with a maximum beam electron energy approaching 2 MeV (figure 4(b)). After that, the number and energy of high-energy electrons decrease gradually. From the density distributions (not shown here), the high energy electrons are produced not continuously and have a pulsed density profile, which can also be observed in figures 1(c) and (d).

Figure  4.  Time evolutions of (a) plasma electron momentum distribution function (MDF) and (b) energy distribution function (EDF) of high energy electrons. The formation of high-energy beam electrons can be clearly identified from the asymmetric distribution of MDF and EDF.

DownLoad: Full-Size Img PowerPoint

The influences of proton beam density on the high energy electron beam generation are also considered here. Figure 5 shows the time evolutions of the number of high-energy electrons (normalized to the number of background plasma electrons) for three beam density cases. From the figure, one can see that as the initial beam density increases, the number of high energy electrons increases and the time (i.e. the plasma length) needed for high energy electron beam generation becomes shorter. Theoretically, a higher beam density leads to a higher growth rate of the instability and a shorter time scale for the instability saturation. With an initial beam density of n_b = 0.4n_e the maximum number of high-energy electrons is about 12% of background plasma electrons in the simulation regions. This value should depend on the size and dimensions of the simulation regions. Two- and three-dimensional PIC simulations will be performed in the future to show the effects of beam focusing and transverse instabilities on the generation of high-energy electrons. It is interesting to note here that with a beam density of n_b = 0.4n_e two peaks can be observed in the time evolution profile (i.e. black line in figure 5), indicating the strong nonlinear beam-plasma interactions.

Figure  5.  Time evolutions of the number of high energy electrons (normalized to the number of background plasma electrons in the simulation regions) for the proton beams with density n_b = 0.2n_e, 0.3n_e and 0.4n_e.

DownLoad: Full-Size Img PowerPoint

4.   Conclusion

In the last few decades, laser-driven proton acceleration has been widely explored because of its important potential applications. The beams produced by this technique often have unique properties, such as high intensity, short pulse duration and low emittance, which are very suitable for the investigations of collective beam-plasma interactions. In this work, the interaction of a laser-accelerated proton beam with a well-controlled gas-discharge plasma is studied in detail with 1D PIC simulations, especially the generation process of high-energy plasma electrons. After the saturation of the two-stream instability between the beam protons and plasma electrons, parts of plasma electrons can escape from the wakefield and are accelerated towards the beam head. The maximum energy of the escaped plasma electrons reaches 2 MeV and a new two-stream instability occurs between the high energy escaped electrons and background electrons. As the proton beam density increases, the plasma length needed for high energy electron generation becomes shorter and the number of produced high energy electrons increases due to a stronger beam-plasma interaction.