| Citation: | Lingke MOU, Zhongyong CHEN, Wei YAN, You LI, Weikang ZHANG, Jiangang FANG, Yingzhou JIANG, Xiaobo ZHANG, Yu ZHONG, Feng LI, Guinan ZOU, Fanxi LIU, Zhipeng CHEN, Zhoujun YANG, Nengchao WANG, Yonghua DING, Yuan PAN, J-TEXT Team. Suppression of runaway current by electrode biasing and limiter biasing on J-TEXT[J]. Plasma Science and Technology, 2022, 24(12): 124001. DOI: 10.1088/2058-6272/ac85a2 |
The avoidance of runaway electrons (REs) generated during plasma disruption is of great concern for the safe operation of tokamak devices. Experimental study on the suppression of runaway current by electrode biasing (EB) and limiter biasing (LB) has been performed on the J-TEXT tokamak, which could be an alternative way to suppress the runaway current. The experimental results show that the higher the voltage value, the smaller the runaway current in both EB and LB experiments. The runaway current can be completely suppressed at an electrode biased voltage of +450 V and a limiter biased voltage of +300 V. The comparison of the energy spectra during the runaway plateau phase shows that the maximum energy max (ERE) and radiation temperature THXR of hard x-rays (HXRs) are significantly reduced after the application of +200 V limiter biased voltage. The electric field generated by the biased voltage may be the key factor to suppress the runaway current, and the measured radial electric field increases obviously after the voltage is applied. This may result in an increase in the loss of REs to realize the suppression of runaway current.
Plasma disruption is of great concern for tokamak operation. It mainly refers to the phenomenon that the plasma loses the confinement of energy, leading to the termination of discharge and even results in damage to the device. When disruption occurs, high-energy runaway electrons (REs) hit the plasma-facing component, which poses a great challenge to the avoidance and mitigation of disruption. The first wall will melt if it is hit by the REs, and the REs also shorten the life of the device. For ITER, up to 70% of the pre-disruption plasma is converted into runaway current, and several tens of mega-electron volt (MeV) energy can be generated during disruption [1, 2]. The generation mechanisms of REs include the Dreicer mechanism [3] (primary generation mechanism), avalanche mechanism [4, 5] (secondary generation mechanism), hot tail mechanism, etc [6].
Over the years, much effort has been devoted to exploring various ways to avoid disruption effectively, and some methods have been found in experiments to mitigate the disruption. Nowadays, the most popular method to suppress the runaway current is injecting a large number of impurities, such as massive gas injection (MGI) [7]. Suppression of REs by MGI on C-Mod, DIII-D, JET and TEXTOR have been extensively studied, and the suppression of REs has been successfully achieved on these tokamaks [8–10]. This is done by increasing the relativistic electron collision frequency νrel, which can be expressed as follows: νrel = nee4 ln Λ/(4πε02me2c3), ln Λ means the Coulombian logarithm, me is the electron mass, ε0 is the vacuum permittivity and ε0 = 8.85×10-12 F m-1. When νrel increases, it can effectively decelerate some high-energy electrons. However, the achievement of the complete suppression of REs must be based on increasing the plasma density to the Rosenbluth density [11]. It is difficult to meet the requirements by MGI on these tokamaks, thus causing a bottleneck. Furthermore, the application of resonant magnetic perturbations (RMPs) has a significant suppression effect on runaway current on ASDEX Upgrade and COMPASS [12, 13]. It is also found on J-TEXT that RMP can excite large magnetic islands, which have the ability to enhance runaway loss during disruption [14]. In contrast, the RMPs have failed to suppress the runway electrons for the large device JET [15]. Therefore, the suppression of RMP on runaway current is inconsistent on different tokamaks.
Some novel methods are being proposed to suppress the runaway current. Electrode biasing (EB) and limiter biasing (LB) are traditional auxiliary systems and they change the floating potential voltage directly, and some experiments with EB and LB have been carried out in recent years [16, 17]. On the IR-T1 tokamak [18] in Iran, the results show that the application of biased voltage during a major disruption can suppress the generation of REs. They also found that the EB applied in edge plasma can modify the radial electric field
In this paper, we discuss the influence of both EB and LB applied to positive bias on runaway current on J-TEXT. The suppression of runaway current has been clearly observed, and it is found that as the voltage increases, the runaway current decreases, and the potential of plasma has been found to be sensitive to the biased voltage. It is also believed that the biased voltage can suppress the runaway current by affecting the radial transport of REs. The paper is arranged as follows. The experimental setup is presented in section 2. In section 3, the influence of positive biased voltage by both electrode and limiter on runaway current is introduced. A discussion is presented in section 4. A summary is given in section 5.
The experiments reported in this paper are carried out on J-TEXT, which has a circle plasma with an iron core, and some of its basic parameters are as follows: major radius R0 = 1.05 m, minor radius a = 0.25‒0.29 m with a movable graphite limiter [20]. It usually operates in the following parameters: the center line-averaged electron density ne is in the range of (1‒7) × 1019 m-3, with toroidal magnetic field Bt of ~2.0 T, pre-disruption plasma current Ip = 120‒220 kA in typical discharges, and the edge safety parameter qa changes with the change in minor radius, toroidal magnetic field and the plasma current.
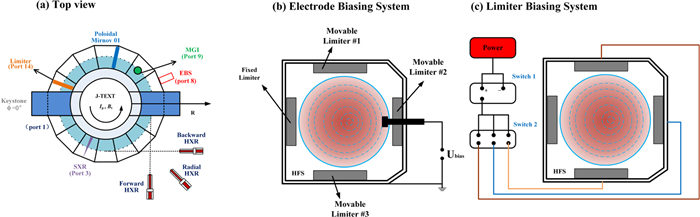
The MGI system has been used to trigger disruption with argon and obtain stable runaway current, which is located at the bottom of port 9 [21]. The Langmuir–Mach probe (LMP) array, which is located at the top of port 13, measures the edge-plasma parameters [22]. There is one array of 24 Mirnov coils for the detection of MHD activities [23]. Three hard x-ray (HXR) detectors are distributed in the forward, radial and backward REs movement directions for measuring thick target bremsstrahlung radiation when the REs hit the first wall or the plasma. The layout of these systems is shown in figure 1(a), and the direction of the plasma current and toroidal magnetic field is counter-clockwise from the top view. In order to measure the energy spectrum of REs, a multi-channel analyzer (MCA) with 0.5 ms time resolution [24] and 1024 channel energy resolution is used.
The EB system [17, 25], shown in figure 1(b), is installed at the mid-plane of port 8, and is equipped with a disc (4 cm in diameter and 1.3 cm in thickness) graphite head [26] and contains a pneumatic driving part. The electrode can reciprocate in the range of 23.5‒25.5 cm in a single discharge [16]. DC voltage and modulation voltage can be selected in the experiment. The LB system in figure 1(c) shares the same power system with the EB system. Three movable limiters are located at the top, bottom and low-field side of the vacuum, respectively. Voltages can be applied to all three limiters at the same time, or only one of them.
The corresponding UEB–IEB of the EB system and the ULB–ILB characteristic of the LB system are shown in figure 2, represented by dots and diamonds, respectively. The data in figure 2 represent the change in currents with voltages on the electrode or the limiter of the low-field side with undisruptive discharges. It is shown that both the EB current IEB and the low-field-side limiter current ILB are approximately proportional to the biased voltage under positive voltages, whereas IEB tends to saturate at about -300 V with a value of -20 A.
The basic plasma parameters in this experiment are as follows: Ip = 160 kA, Bt = 1.8 T, ne = (1‒2) × 1019 m-3 and qa ≈3.5. MGI injects argon at 0.4 s to trigger disruption, leading to a stable runaway current platform. The voltage is applied to the electrode during a time of 0.35‒0.42 s at a position of 25 cm, just 5 mm inside the last closed flux surface (r~25.5 cm). The injection of argon causes the temperature quench (TQ) phase. The sharp drop in the plasma temperature then results in an enhancement of the plasma resistivity, and it further leads to the decay of the plasma current in what is called the current quench (CQ) phase. A strong toroidal electric field is induced in the vacuum during CQ, accelerating the runaway seeds to a higher speed, and a stable runaway current is eventually formed.
In the experiment, different positive voltages have been applied to the electrode. The discharge results at +100, +270 and +450 V biased voltage are shown in figure 3. When the positive voltages are applied to the electrode, the runaway current is suppressed. The higher the voltage value, the smaller the runaway current, and the effect of complete suppression is achieved at +450 V. Figure 3(c) gives the details of the electrode current IEB. A spike appears in the current, which is caused by the MGI valve injection of a large amount of argon impurity causing the TQ and CQ processes, and a strong loop voltage, as shown in figure 3(d), will be generated in the vacuum, resulting in a strong current spike on the electrode.
In the J-TEXT tokamak, hydrogen is usually used for discharge, and most of the atoms and ions at the plasma boundary are in the ground state. When they collide with the electrons, the energy level of the ground-state particles changes, and the radiation generated in this process is represented by Hα emission. It can also be used to characterize the plasma confinement, since when the Hα increases, more particles are transported to the low-field side. During the runaway plateau phase, due to the deterioration of particle confinement, the particles are diffused and lost continuously on the electrode, the current shows a slight increase, as shown in figure 3(e), and the Hα emission also keeps rising. During the final runaway CQ phase, REs are rapidly lost, leading to a rise in Hα density and electrode current again. For discharge #1070545, no runaway current is generated and the electrode is accompanied by higher current. The electrode current even reaches saturation, and the Hα emission is maintained at a high level after disruption for several milliseconds during CQ, then decreases slowly at 0.406 s.
The influence of LB on the runaway current has also been explored. The parameters are as follows: plasma current Ip = 180 kA, toroidal magnetic field Bt = 2.2 T, electron density ne~(1‒1.5) × 1019 m-3. Since there are different vacuum conditions in each experiment, runaway current is hard to generate at some plasma parameters, so the basic plasma parameters of Ip and Bt are not the same in any two experiments, but this will not affect the qualitative results in both experiments. The voltage is applied to the low-field-side limiter only and the radial position of the limiter is fixed at 255 mm. Different voltages are applied to the limiter during a time of 0.35‒0.42 s. Typical experimental results are shown in figure 4.
It can be seen from figure 4(c) that there is still a large limiter current in the TQ and CQ processes, for discharge #1076005, where the Hα emission and ILB also increase with time during the runway plateau phase. However, in discharge with +300 V biased voltage, the Hα radiation remains for a long time during the CQ process, which is similar to the phenomenon observed in the discharge #1070545 in the EB experiment.
Figure 5 shows the runaway current as a function of the electrode current or the limiter current. This completely reveals that the runaway current is negatively correlated with the biased current, either with the EB or LB system. Due to the higher plasma parameters in the LB experiment, the runaway current of the reference discharge is higher, but the limiter requires a smaller threshold for the complete suppression of runaway current. The size of the limiter is 25 cm in length, 10 cm in width and 5 cm in thickness, and the electrode is 4 cm in diameter and 1.3 cm in thickness. Compared to the limiter, the electrode is small in size and has a smaller contact surface with the plasma. If a simple electric power calculation is performed, when the REs can be completely suppressed, the electric power required by the electrode and the limiter is 53.1 and 30.4 kW, respectively. It can be seen from figure 2 that the biased current difference between the electrode and the limiter is not particularly large under the same positive biased voltage. Due to the larger size of the limiter, the effective area for interaction with the plasma is also larger. Therefore, the suppression efficiency of the runaway current is higher, and the REs can be completely suppressed at a lower voltage. The slope of the straight line in figure 5 is assumed to represent the 'suppression efficiency' of the two systems on the runaway current, and it can be seen that the LB is more efficient on the runaway current.
The HXR bremsstrahlung spectra of the REs reflect the relationship between the number of REs and their energy. It is an important way to analyze the behavior of the REs, and it also reflects information about the maximum energy max (ERE) and radiation temperature THXR of the REs. Figure 6 shows the energy spectra during the runaway plateau phase.
The maximum energy max (ERE) is defined as the intersection of the linear fit of the high-energy tail of the spectrum with
The HXR spectra for the reference discharge #1075997 and the discharge with +200 V bias in #1076005 are shown in figure 6(a). The maximum energy of the HXR spectrum in the discharge with +200 V bias is lower than that of the reference discharge. This indicates that the +200 V bias enhances the radial transport loss of REs, which leads to a decrease in both the runaway confinement and the runaway energy. This is beneficial for the suppression of runaway current. The runaway energy is also lower when the +200 V bias is applied to a similar runaway current plateau (62 kA), as shown in figure 6(b). This illustrates that the positive bias has the effect of reducing the runaway confinement and the runaway energy by enhancement of the runaway radial transport loss.
The loss of REs includes collisional transport, electrostatic perturbation and magnetic perturbation. Due to the low collision frequency of REs, neoclassical transport due to collisions is generally not considered. Therefore, the enhancement of the radial transport of REs is also one of the means for runaway loss, and the runaway current is greatly affected by the number of runaway seeds. The suppression of runaway current can also be achieved by increasing the radial transport of runaway seeds. In the experiment of LB, a Langmuir probe has been used. Figure 7 gives information about the radial electric field during the time when the limiter is applied to +250 voltage. By measuring the radial distribution of the floating potential Vf(r), the radial electric field can be obtained from the gradient of the potential Er = -dVf/dr. As can be seen from figure 7, the value of the radial electric field is about 2 kV m-1 without LB. When +250 V limiter biased voltage is applied, the radial electric field increases significantly in the positive direction, the direction of the radial electric field measured is the component along the minor radius in the horizontal direction, and the maximum value of Er can reach about 7 kV m-1. In addition, the average radial electric field values vary with different voltages, which shows that as the voltage increases, Er also increases linearly.
The velocity disturbance caused by the electric field disturbance in the direction perpendicular to the magnetic field is
| (1) |
Generally,
In the experiment, the effect of biased voltage on the plasma parameters at the boundary has also been studied. Figure 8 mainly describes the changes in electron density ne, C-Ⅲ emission and Hα radiation at the plasma boundary, and HXR radiation with +450 V electrode biased voltage and +300 V limiter biased voltage, with the aim of observing the loss of REs at the boundary.
Figures 8(c)‒(h) show the evolution of the electron density ne, C-Ⅲ and Hα emission at the plasma boundary, and all three signals display a significant increase after 0.35 s application of biased voltage. Since the electrode is equipped with a graphite head, some of impurities will occur during the discharge process, resulting in a stronger C-Ⅲ signal in discharge #1070545 than in discharge #1076014 after 0.35 s. Figures 8(i) and (j) give the evolution of HXR radiation. During the discharge of the biased electrode, the HXR radiation keeps rising after 0.35 s, reaches its peak when disruption occurs, and then decreases rapidly. However, during the discharge of the biased limiter #1076014, the HXR signal peaks at about 0.37 s, followed by a slow decline in radiation intensity. Since no runaway current is formed in both discharges, the HXR intensity is lower after disruption. This reveals that the biased voltage significantly enhances the RE losses, which means that a large number of REs are expelled from the plasma due to the biased voltage and the radial transport of the REs increases.
The effect of the magnetic turbulence and accelerating electric field on REs is also studied. Figure 9 shows the relationship between the ratio of IRE/Ip and MHD integrated intensity, and IRE/Ip as a function of Eacceleration/Ecritical in the two experiments of the biased electrode and biased limiter, respectively. Eacceleration is calculated by the mean value of Vloop during the CQ process, and the MHD integrated intensity is measured by Mirnov coils in both the TQ and CQ processes. The blue diamonds and red circles represent the data for the biased electrode and biased limiter, respectively.
It can be inferred from figure 9(a) that larger runaway currents exist when the magnetic fluctuation is lower. This trend is well illustrated by the fitted line in figure 9(a), both with biased electrode and the biased limiter. It is worth mentioning that the loss of REs can be enhanced by magnetic fluctuation, and the runaway beam themselves may also have a stabilizing effect on MHD [31]. Figure 9(b) gives the ratio of IRE/Ip as a function of Eacceleration/Ecritical. Lower Eacceleration/Ecritical results in smaller IRE/Ip. It is worth noting that the direction of the arrow in the figure represents the trend of increasing biased voltages. It seems that when the biased voltage is larger, the MHD behavior during the CQ process is stronger, which on the one hand enhances the loss of runaway seeds, and on the other hand, the corresponding decrease in the ratio of Eacceleration/Ecritical also affects the formation of REs.
The suppression of REs is a serious issue for large-scale tokamak devices. The alternative runaway suppression by EB and LB has been carried out on the J-TEXT tokamak. Positive voltages of different amplitudes are applied to the electrode and limiter; 0–500 and 0–350 V are applied, respectively. Parameters, such as plasma current Ip, loop voltage Vloop, Hα radiation and HXR radiation, are measured. It is found that the runaway current decreases during the increase in the biased voltage in both experiments. Moreover, the runaway current can be completely suppressed at +450 V by EB and +300 V by LB.
By comparing the energy spectra of the REs during the runaway plateau phase, it is found that the number of REs decreases after the application of +200 V limiter biased voltage. Both the radiation temperature THXR and the maximum energy max (ERE) of the REs decrease significantly, indicating that both the number and energy of the REs are reduced, and the suppression of runaway current is achieved by reducing the generation of runaway seeds.
The radial electric field Er generated by the bias may be the main factor suppressing the runaway current, and it is calculated using the gradient from the floating potential, including the component of potential disturbance. In the limiter biased experiment, the electric field measured by the Langmuir probe rises significantly at about 0.35 s. The average values of the radial electric field vary with different voltages, and Er increases linearly as the voltage increases. The diffusion coefficient of the REs is enhanced by electrostatic disturbance, which accelerates the loss of the runaway seeds, leading to a lower runaway plateau. The electron density ne, Hα signal and C-Ⅲ radiation at the boundary are significantly improved after the biased voltage is applied. In addition, there is a significant enhancement in HXR intensity. As a consequence of all the above factors, the number of electrons at the plasma boundary increase, and the interaction between the electrons and the first wall is enhanced, resulting from the improvement in runaway loss.
The suppression of runaway current by bias is a reliable method in some small and medium-size tokamaks, and the effect of radial electric field on runaway confinement and maximum runaway energy is demonstrated. It is found that a positive radial electric field can reduce both the runaway current plateau and the maximum runaway energy. The electrode cannot be used on large-scale devices due to high edge-plasma temperature. This method of suppressing runaway current can be used for the protection of the first wall during disruption in large-scale devices by increasing the radial electric field. The radial electric field can be modulated by the limiter bias or active plasma rotation control.
This work is supported by National MCF Energy R & D Program of China (No. 2019YFE03010004), National Key R & D Program of China (No. 2018YFE0309100), National Natural Science Foundation of China (Nos. 11775089 and 51821005) and National Magnetic Confinement Fusion Science Program of China (Nos. 2015GB111002 and 2015GB104000).
| [1] |
Granetz R S et al 2014 Phys. Plasmas 21 072506 doi: 10.1063/1.4886802
|
| [2] |
Martín-Solís J R, Loarte A and Lehnen M 2017 Nucl. Fusion 57 066025 doi: 10.1088/1741-4326/aa6939
|
| [3] |
Dreicer H 1959 Phys. Rev. 115 238 doi: 10.1103/PhysRev.115.238
|
| [4] |
Rosenbluth M N and Putvinski S V 1997 Nucl. Fusion 37 1355 doi: 10.1088/0029-5515/37/10/I03
|
| [5] |
Besedin N T and Pankratov I M 1986 Nucl. Fusion 26 807 doi: 10.1088/0029-5515/26/6/009
|
| [6] |
Smith H M et al 2009 Plasma Phys. Control. Fusion 51 124008 doi: 10.1088/0741-3335/51/12/124008
|
| [7] |
Wei Y N et al 2019 Plasma Phys. Control. Fusion 61 084003 doi: 10.1088/1361-6587/ab210b
|
| [8] |
Hollmann E M et al 2010 Phys. Plasmas 17 056117 doi: 10.1063/1.3309426
|
| [9] |
Loarte A et al 2011 Nucl. Fusion 51 073004 doi: 10.1088/0029-5515/51/7/073004
|
| [10] |
Bozhenkov S A et al 2008 Plasma Phys. Control. Fusion 50 105007 doi: 10.1088/0741-3335/50/10/105007
|
| [11] |
Granetz R S et al 2007 Nucl. Fusion 47 1086 doi: 10.1088/0029-5515/47/9/003
|
| [12] |
Gobbin M et al 2018 Plasma Phys. Control. Fusion 60 014036 doi: 10.1088/1361-6587/aa90c4
|
| [13] |
Mlynar J et al 2019 Plasma Phys. Control. Fusion 61 014010 doi: 10.1088/1361-6587/aae04a
|
| [14] |
Lin Z F 2019 Plasma Phys. Control. Fusion 61 024005 doi: 10.1088/1361-6587/aaf691
|
| [15] |
Riccardo V et al 2010 Plasma Phys. Control. Fusion 52 124018 doi: 10.1088/0741-3335/52/12/124018
|
| [16] |
Castaldo C et al 2016 Nucl. Fusion 57 016003 doi: 10.1088/0029-5515/56/1/016003
|
| [17] |
Sun Y et al 2014 Plasma Phys. Control. Fusion 56 015001 doi: 10.1088/0741-3335/56/1/015001
|
| [18] |
Ghanbari M R et al 2011 Phys. Scr. 83 055501 doi: 10.1088/0031-8949/83/05/055501
|
| [19] |
Silva C et al 2004 Nucl. Fusion 44 799 doi: 10.1088/0029-5515/44/7/012
|
| [20] |
Liang Y et al 2019 Nucl. Fusion 59 112016 doi: 10.1088/1741-4326/ab1a72
|
| [21] |
Ding Y H et al 2018 Plasma Sci. Technol. 20 125101 doi: 10.1088/2058-6272/aadcfd
|
| [22] |
Liu H et al 2018 Plasma Phys. Control. Fusion 60 115012 doi: 10.1088/1361-6587/aae297
|
| [23] |
Zhuang G et al 2011 Nucl. Fusion 51 094020 doi: 10.1088/0029-5515/51/9/094020
|
| [24] |
Hu J et al 2020 Plasma Sci. Technol. 22 115102 doi: 10.1088/2058-6272/aba681
|
| [25] |
Zhu T Z et al 2014 Rev. Sci. Instrum. 85 053504 doi: 10.1063/1.4873320
|
| [26] |
Sun Y et al 2016 Nucl. Fusion 56 046006 doi: 10.1088/0029-5515/56/4/046006
|
| [27] |
Lvovskiy A et al 2018 Plasma Phys. Control. Fusion 60 124003 doi: 10.1088/1361-6587/aae95a
|
| [28] |
Younis J et al 2009 Plasma Phys. Control. Fusion 51 075017 doi: 10.1088/0741-3335/51/7/075017
|
| [29] |
Younis J et al 2008 Plasma Sci. Technol. 10 529 doi: 10.1088/1009-0630/10/5/02
|
| [30] |
Jaspers R E 1995 Relativistic runaway electrons in tokamak plasmas PhD thesis Technische University Eindhoven (Eindhoven, Netherlands)
|
| [31] |
Reux C et al 2015 Nucl. Fusion 55 129501 doi: 10.1088/0029-5515/55/12/129501
|
| [1] | Ruirui MA, Chen ZHAO, Yao ZHOU, Chang LIU. Numerical study of runaway current impact on sawtooth oscillations in tokamaks[J]. Plasma Science and Technology, 2025, 27(3): 035101. DOI: 10.1088/2058-6272/ad91e7 |
| [2] | Zhongyong CHEN, Zhifang LIN, Wei YAN, Duwei HUANG, Yunong WEI, You LI, Nianheng CAI, Jie HU, Yonghua DING, Yunfeng LIANG, Zhonghe JIANG, J-TEXT Team. Overview of runaway current suppression and dissipation on J-TEXT tokamak[J]. Plasma Science and Technology, 2022, 24(12): 124009. DOI: 10.1088/2058-6272/aca272 |
| [3] | Jie HU (胡捷), Wei YAN (严伟), Ruihai TONG (佟瑞海), Wei LI (李维), Zhongyong CHEN (陈忠勇), Anjue DAI (戴岸珏), Duwei HUANG (黄都伟), Yunong WEI (魏禹农), Tiankui MA (马天逵), Yuan HUANG (黄缘), Yang LI (李阳), Wei ZHENG (郑玮), Zhifang LIN (林志芳), You LI (李由), Huaiyu YANG (杨怀玉), Duoqin WANG (王铎钦), Wei BAI (白炜), Yu ZHONG (钟昱), Jiangang FANG (方建港), J-TEXT Team. Soft landing of runaway currents by ohmic field in J-TEXT tokamak[J]. Plasma Science and Technology, 2020, 22(11): 115102. DOI: 10.1088/2058-6272/aba681 |
| [4] | Huaxiang ZHANG (张华祥), Dehui LI (李德徽), Yunfeng LIANG (梁云峰). Toward modeling of the scrape-off layer currents induced by electrode biasing using 2D particle-in-cell simulations[J]. Plasma Science and Technology, 2020, 22(10): 105102. |
| [5] | Yonghua DING (丁永华), Zhongyong CHEN (陈忠勇), Zhipeng CHEN (陈志鹏), Zhoujun YANG (杨州军), Nengchao WANG (王能超), Qiming HU (胡启明), Bo RAO (饶波), Jie CHEN (陈杰), Zhifeng CHENG (程芝峰), Li GAO (高丽), Zhonghe JIANG (江中和), Lu WANG (王璐), Zhijiang WANG (王之江), Xiaoqing ZHANG (张晓卿), Wei ZHENG (郑玮), Ming ZHANG (张明), Ge ZHUANG (庄革), Qingquan YU (虞清泉), Yunfeng LIANG (梁云峰), Kexun YU (于克训), Xiwei HU (胡希伟), Yuan PAN (潘垣), Kenneth William GENTLE, the J-TEXT Team. Overview of the J-TEXT progress on RMP and disruption physics[J]. Plasma Science and Technology, 2018, 20(12): 125101. DOI: 10.1088/2058-6272/aadcfd |
| [6] | Manman XU (徐曼曼), Yuntao SONG (宋云涛), Gen CHEN (陈根), Yanping ZHAO (赵燕平), Yuzhou MAO (毛玉周), Guang LIU (刘广), Zhen PENG (彭振). Tunable biasing magnetic field design of ferrite tuner for ICRF heating system in EAST[J]. Plasma Science and Technology, 2017, 19(11): 115601. DOI: 10.1088/2058-6272/aa8167 |
| [7] | JU Xingbao (琚兴宝), SUN Haishun (孙海顺), YANG Zhuo (杨倬), ZHANG Junmin (张俊民). Investigation on the Arc Ignition Characteristics and Energy Absorption of Liquid Metal Current Limiter Based on Self-Pinch Effect[J]. Plasma Science and Technology, 2016, 18(5): 531-537. DOI: 10.1088/1009-0630/18/5/15 |
| [8] | LIU Yiying (刘懿莹), WU Yi (吴翊), RONG Mingzhe (荣命哲), HE Hailong (何海龙). Simulation of the Effect of a Metal Vapor Arc on Electrode Erosion in Liquid Metal Current Limiting Device[J]. Plasma Science and Technology, 2013, 15(10): 1006-1011. DOI: 10.1088/1009-0630/15/10/09 |
| [9] | A. C. ENGLAND, Z. Y. CHEN, D. C. SEO, J. CHUNG, Y. S. LEEV, J. W. YOO, W. C. KIM, Y. S. BAE, Y. M. JEONV, J. G. KWAK, M. KWON, the KSTAR Tea. Runaway Electron Suppression by ECRH and RMP in KSTAR[J]. Plasma Science and Technology, 2013, 15(2): 119-122. DOI: 10.1088/1009-0630/15/2/08 |
| [10] | SONG Xianying (宋先瑛), YANG Jinwei (杨进蔚), LI Xu (李旭), YUAN Guoliang (袁国梁), ZHANG Yipo (张轶泼). Synergetic Effects of Runaway and Disruption Induced by VDE on the First Wall Damage in HL-2A[J]. Plasma Science and Technology, 2012, 14(3): 207-214. DOI: 10.1088/1009-0630/14/3/05 |
| 1. | Ding, Y., Wang, N., Chen, Z. et al. Overview of the recent experimental research on the J-TEXT tokamak. Nuclear Fusion, 2024, 64(11): 112005. DOI:10.1088/1741-4326/ad336e |
| 2. | Reyes, L.-E., Blanco, M.-R., Pinillos, M.-J. Past, Present, and Future of Learning Agility: A Bibliometric and Content Analysis. Human Resource Development Review, 2024. DOI:10.1177/15344843241258522 |
