Analysis of inverse synthetic aperture radar imaging in the presence of time-varying plasma sheath

1.   Introduction

Inverse synthetic aperture radar (ISAR) imaging is a method for stationary radar to identify the type of target by observing moving targets [1, 2]. Owing to its active, all-day and all-weather imaging capability, the ISAR imaging technique has been widely used for civilian and military purposes. With respect to any hypersonic reentry objects, the ISAR can realize high-resolution two-dimensional images, which is of critical importance for subsequent operations of target recognition. Due to the reentry object's high moving speed, it is difficult to obtain high-resolution ISAR images by using conventional imaging methods. On one hand, the high moving speed of the object will cause severe range migration, resulting in defocused ISAR images. On the other hand, when a reentry object penetrates through the Earth's atmosphere, drastic friction happens to its surface with the atmosphere, generating extremely high temperatures. The tremendous heat dissociates and ionizes air molecules of the plasma sheath enveloping the object [3–5]. This will change the propagation characteristics of electromagnetic (EM) waves, to which the amplitude attenuation and phase shift occur inevitably [6–9]. Therefore, the plasma sheath enveloping the reentry object can deteriorate the performance of ISAR imaging. Most of the existing studies aim at correcting migration through range resolution cells (MTRC), whereas the work concerning the plasma sheath is still rare. In this work, we investigate the influence of the plasma sheath imposed on ISAR imaging.

Recently, researches concerning plasma sheaths have attracted much attention. Related studies have been conducted to evaluate the influence of the plasma sheath on the EM waves propagating within and through. Yang et al conducted an experiment and verified the parasitic modulation phenomenon of EM waves, in which sinusoidal time-varying plasma is considered [10]. Bai et al established the spatiotemporal model of reentry object enveloped with time-varying plasma sheath, and explored the instantaneous transmission characteristics of EM wave propagation in time-varying plasma sheath [11]. Yao et al proposed an electron density fluctuation model, which revealed the fluctuation law of electron density in the spatiotemporal frequency domain [12]. These studies mainly focused on the transmission coefficient of time-varying plasma sheath.

Furthermore, the influence of plasma sheath on radar signals was investigated. Liu et al analyzed the mono-static radar cross section (RCS) of EM waves from X- to Ku-band by a blunt cone with nonuniform plasma enveloping. This indicated that the RCS depends heavily on the inhomogeneous plasma parameters [13]. Song et al studied the intra-pulse modulation of chirp signal caused by sinusoidal time-varying uniform plasma, and calculated the correlation between the continuous time-varying plasma and linear frequency modulation (LFM) pulse [14]. The study revealed that the intra-pulse modulation causes the spectrum broadening phenomenon. Further, Chen et al studied the influence of sinusoidal time-varying nonuniform plasma sheath on radar echo. They revealed the relationship of the reflection coefficient with the varying electron density and thickness [15]. Through investigating the one-dimensional range profile of the radar echo coupled with plasma sheath, Ding et al found that the plasma sheath not only causes many false targets but also mitigates the range profile, thus resulting in the detection failure [16]. Despite the aforementioned achievements regarding radar detection in the presence of plasma sheath, however, those works mainly focused on one-dimensional radar signals. Hardly any research concerning two-dimensional ISAR is to be found so far.

In this work, we study the formation mechanism of ISAR imaging under the environment of time-varying plasma sheath, presenting a comprehensive analysis with respect to the effect of plasma sheath on ISAR imaging. Based on the transmission line matrix (TLM) method, the reflection characteristic of the time-varying plasma sheath is explored by calculating its magnitude and phase coefficients. Through incorporating the reflection coefficient, an ISAR imaging model enveloped with time-varying plasma is developed. Then, based on the obtained damaged ISAR image, a statistical analysis scheme is adopted and a comprehensive approach in terms of the range and azimuth dimensions is conducted. Simulation results suggest that the defocusing of ISAR images closely correlates with the time-varying characteristics of plasma sheath. The plasma sheath causes not only false peaks on the range dimension but also Doppler frequency diffusion on the azimuth dimension, thus resulting in defocusing of the ISAR images. In addition, the mechanism and property of the defocusing are verified through different simulations, and the performance of ISAR imaging is evaluated.

The remainder of this paper is organized as follows. Section 2 introduces the reflection coefficient of time-varying plasma sheath. Section 3 proposes the ISAR model of the reentry object enveloped by plasma sheath. Section 4 discusses the formation mechanism of false peaks on the range dimension. Section 5 analyzes the principle of Doppler frequency diffusion on the azimuth dimension. Section 6 analyzes simulation results of ISAR image defocusing caused by time-varying plasma sheath and evaluates the related influence effect. Section 7 summarizes conclusions.

2.   Reflection coefficient of plasma sheath

In this section, the plasma sheath that envelopes the reentry object is studied, and the reflection coefficient is deduced based on TLM [17].

2.1   Reflection coefficient calculation of layered plasma sheath

As a nonuniform and non-magnetized plasma fluid, the plasma sheath enveloping the reentry object imposes a modulation effect on EM waves, causing amplitude attenuation and phase distortion of the reflected EM waves. The parameters of plasma sheath mainly include electron density $N_{\mathrm{e}},$ plasma thickness $z_{\max }$ and collision frequency $v_{\mathrm{e}}.$ In this paper, the time-varying parameter of plasma sheath is the electron density, which is commonly used in analyzing the time-varying characteristics of plasma.

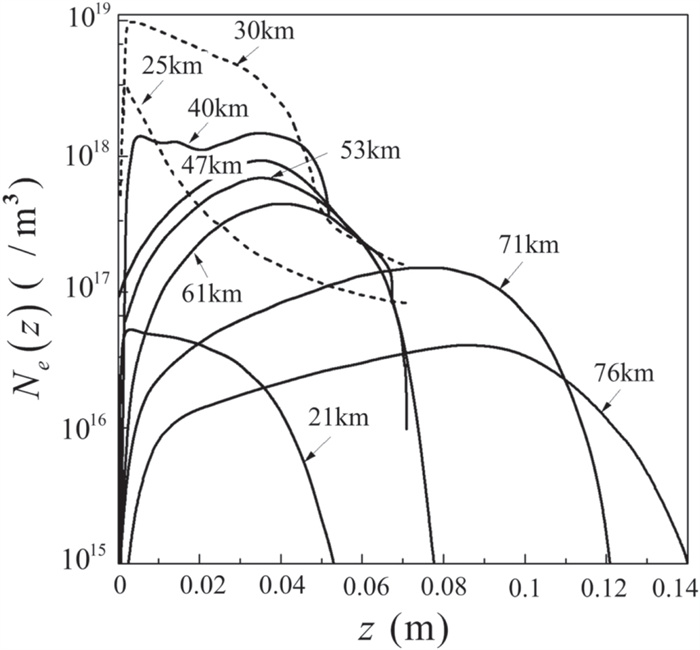
In the RAM-C project, the distribution of electron density with distance at different altitudes is obtained in figure 1. According to the research of RAM-C, the electron density distribution approximates a double Gaussian distribution [18], which can be expressed as

Figure  1.  Mean profiles of electron density at different altitudes of RAM-C project.

DownLoad: Full-Size Img PowerPoint

where $z$ is the distance from the plasma sheath to the surface of its enveloped reentry object, $N_{\mathrm{e}}^{\mathrm{peak}}$ and $z_0$ are the peak electron density and its position, respectively, $a_1$ and $a_2$ are the shape parameters.

According to the distribution property of electron density [19], the nonuniform plasma sheath is stratified into a model with $L$ layer uniform plasma. Therefore, the plasma sheath can be modeled by its neighboring homogenous plasma layers that are stratified. Figure 2 shows a discrete approximation of the stratified plasma sheath, where the plasma sheath is stratified into $L$ layers, and the $L+1$ layer is the object surface. In this case, the plasma frequency of the plasma sheath on the ith layer (denoted as ${\omega }_{\mathrm{p},i}$ ) can be expressed as

Figure  2.  Neighboring homogeneous stratification model of the plasma sheath's layers enveloping object surface.

DownLoad: Full-Size Img PowerPoint

where $i=1,2,3···L,$ $e$ is the unit charge, $m_{\mathrm{e}}$ is the electron mass, ${\epsilon }_0$ is the vacuum permittivity, $N_{\mathrm{e},i}$ is the electron density of the ith layer.

The complex permittivity of the ith layer ${\epsilon }_i$ is expressed as

where $\omega$ is the angular frequency of the incident wave.

Then, we can calculate the transmission constant ( $k_i$ ) and intrinsic impedance ( $Z_i$ ) of the ith layer through using the following equations

where ${\mu }_0$ is the vacuum permeability.

Supposing that ${\theta }_i$ is the incident angle of the ith layer, $d_i$ is the plasma thickness of the ith layer, then the transmission matrix of the ith layer plasma sheath is obtained using TLM [20, 21], which is expressed as

where $A_i,$ $B_i,$ $C_i$ and $D_i$ are the elements of the transmission matrix.

According to microwave network theory, the total transmission matrix of the plasma sheath can be obtained by successively multiplying the transmission matrix of each layer, namely:

Since the reentry object is enveloped with plasma sheath, the reflection of EM waves comes from both the plasma sheath and the surface. For ease of calculation, we presuppose that the surface of the reentry object is made of metal. Then the reflection coefficient of the plasma-sheath-enveloped object surface can be obtained using the following equation [22]

where $Z_0$ is the wave impedance of the EM waves in the incident medium.

Here, a simulation is conducted to evaluate the modulation effect of the plasma sheath. Specifically, a typical environment for an ISAR system is considered, where the transmitting frequency f₀ is set to X-band and the bandwidth is assumed as 1 GHz. The electron density and other parameters of the plasma sheath are obtained from computational fluid dynamics' flow-field simulation data of the RAM-C for reentry vehicles [23]. Throughout the simulation process, two scenarios involving steady-state and time-varying plasma sheaths are considered.

2.2   Reflection coefficient expression of steady-state plasma sheath

When the time-varying characteristics of electron density are not considered, the correlation among the incident frequency, the reflective amplitude and the reflective phase at different altitudes are shown in figure 3. Figures 3(a) and (b) show the amplitude and phase curves of $R$ versus ISAR bandwidth range with respect to different altitudes, respectively. It can be observed that during the bandwidth range, the amplitude remains almost constant at different altitudes, whereas the phase decreases linearly as the frequency increases. Based on the observation, the reflection coefficient $R$ in frequency domain can be approximately expressed as

Figure  3.  (a) Amplitude of reflection coefficient in ISAR frequency range, (b) phase of reflection coefficient in ISAR frequency range.

DownLoad: Full-Size Img PowerPoint

where the constant $A$ denotes the amplitude coefficient, ${\theta }_0$ denotes the initial phase, $k_{\theta }$ denotes the slope of the phase curve.

2.3   Reflection coefficient expression of time-varying plasma sheath

In practice, due to the presence of atmospheric turbulence and disturbance, the electron density of plasma sheath inevitably suffers from time-varying characteristics. The fluctuation frequency approximates to the burst frequency of the turbulence, which is supposed to vary from 20 kHz up to 100 kHz [24]. For ease of analysis, in this paper, the sinusoidal fluctuation mode is therefore adopted to approximate its fluctuation properties. By using this mode, the sinusoidally-varying electron density can provide a universal model of nonlinear variation of the plasma sheath [15, 25, 26].

Supposing that the plasma sheath is uniformly discretized into $L$ layers, then the time-varying electron density $N_{\mathrm{e}}^{\mathrm{tv}}$ is the function of the time $t$ and distance $z,$ so $N_{\mathrm{e}}^{\mathrm{tv}}$ can be expressed as

In the above equation, the electron density $N_{\mathrm{e}}$ follows a double Gaussian distribution, $\sigma$ is the fluctuation scale parameter, $f_{N_{\mathrm{e}}}$ is the electron density fluctuation frequency. Figure 4 illustrates the 3D diagram of the time-varying electron density, which shows that the time-varying electron density follows double Gaussian distribution in the distance domain and sinusoidal distribution in the time domain.

Figure  4.  3D diagram of the time-varying electron density.

DownLoad: Full-Size Img PowerPoint

Similarly, the reflection coefficient in the presence of the time-varying plasma sheath is calculated using TLM. The amplitude and phase of the reflection coefficient at different altitudes are shown in figure 5. It can be seen from figures 5(a) and (b) that the reflective amplitude curve of each altitude fluctuates sinusoidally, whereas the phase curve exhibits an approximately linear decreasing as the frequency increases.

Figure  5.  (a) Amplitude of reflection coefficient in ISAR frequency range, (b) phase of reflection coefficient in ISAR frequency range.

DownLoad: Full-Size Img PowerPoint

Motivated by the above findings, the frequency-domain reflection coefficient of the object surface enveloped with time-varying plasma sheath $R_{\mathrm{tv}}$ can be approximately expressed as

where $A_0$ is the initial amplitude, $A_1$ is the sinusoidal curve amplitude of the reflective amplitude, $f_{\mathrm{A}}$ is the sinusoidal fluctuation frequency of the amplitude curve, ${\phi }_0$ is the initial phase of the sinusoidal curve.

3.   ISAR imaging in the presence of time-varying plasma sheath

The relative motion between the reentry object and the radar includes two parts: translation and rotation [27, 28]. In this work, the MTRC caused by translation motion is supposed to be compensated by translation motion compensation (TMC) [29]. The motion target can be equivalent to the one rotating around the reference center in a fixed position during the coherent processing interval. Schematic diagram of the ISAR imaging is shown in figure 6, in which $o$ is the reference center, $r_0$ is the radial distance between radar and reference center, $r_{\mathrm{s}}$ is the radial distance between radar and scattering, $r_1$ is the distance of reference center and the scattering, $\omega$ is the rotation speed. The range dimension is divided into $N$ range cells from $t_1$ to $t_N,$ the azimuth dimension is divided into $M$ Doppler cells from ${\widehat{f}}_1$ to ${\widehat{f}}_M.$

Figure  6.  Diagram of ISAR imaging.

DownLoad: Full-Size Img PowerPoint

The LFM signal transmitted by the radar can be expressed as

where $T_{\mathrm{p}}$ is the pulse width, $f_0$ is the carrier frequency, $\mu$ is the chirp rate, $t_n$ is the nth range cell in one pulse period being referred to as fast-time.

For multiple echoes, the echo expression can be obtained by

where $v$ is the radial velocity of the object, $c$ is the speed of light, ${\widehat{t}}_m$ is the transmitting time of the mth pulse being referred to as slow-time.

When the time-varying plasma sheath is considered, the time-domain expression of reflection coefficient at the transmitting time of ${\widehat{t}}_m$ can be obtained by performing inverse Fourier transform (FT) on equation (11), which is expressed as

where $C_1=A_0\exp \left(\mathrm{j}{\theta }_0\right),$ $C_2=\frac{A_1}{2}\exp \left(\mathrm{j}{\theta }_0\right).$

Then the echo coupled with time-varying plasma sheath [13] is expressed as

where the symbol $\otimes$ denotes convolution processing.

Range-Doppler (RD) algorithm has been widely used in ISAR imaging, whose flowchart is shown in figure 7. It can be observed that the range profile of scattering is obtained from pulse compression. Then, the pulse compression results of all echoes are compensated by the TMC method including envelope alignment and phase focus. The TMC result can be calculated by

Figure  7.  Flowchart of ISAR imaging using RD method.

DownLoad: Full-Size Img PowerPoint

where ${\rho }_0$ is the phase coefficient.

By performing FT on equation (16) with respect to slow-time, the ISAR image result $\mathrm{I}\mathrm{S}\mathrm{A}{\mathrm{R}}_{\mathrm{tv}}\left(t_n,{\widehat{f}}_m\right)$ can be expressed as

where $C_0$ is a constant, ${\tau }_0$ is the echo delay of scattering, $f_{\mathrm{d}}$ is the Doppler frequency of scattering, ${\widehat{f}}_m$ is the mth Doppler cell, $r_{\mathrm{tv}}\left(t_n,{\widehat{f}}_m\right)$ is the FT of $r_{\mathrm{tv}}\left(t_n,{\widehat{t}}_m\right)$ with respect to slow-time. The expressions of these parameters can be written as

where $\mathrm{F}{\mathrm{T}}_{-{\phi }_0}\left({\widehat{f}}_m\right)$ and $\mathrm{F}{\mathrm{T}}_{{\phi }_0}\left({\widehat{f}}_m\right)$ represent the FT result of $\exp \left(-\mathrm{j}{\phi }_0\left({\widehat{t}}_m\right)\right)$ and $\exp \left(\mathrm{j}{\phi }_0\left({\widehat{t}}_m\right)\right),$ respectively.

Then equation (17) can be rewritten as

It can be obtained from the above equation (22) that the time-varying plasma sheath modulates the radar echo on the range and azimuth dimension, respectively. The existence of $\delta$ functions causes abnormal results of pulse compression. Furthermore, the presence of different initial phases destroys the coherence of the pulses, causing ineffective coherent accumulation. In subsequent sections, we analyze the influence of time-varying plasma sheath on ISAR imaging from two perspectives, i.e. range dimension and azimuth dimension. These analyses reveal the effect of pulse compression result on range dimension and the FT result on azimuth dimension caused by time-varying plasma sheath.

4.   Influence mechanism on range dimension

In this section, we investigate the mechanism of abnormal range dimension result through deducing the pulse compression formula coupled with time-varying plasma sheath.

4.1   Formation mechanism of false scatterings

For ease of analysis on range dimension, the independent parameters of fast-time are replaced with constants, and equation (22) can be rewritten as

where $C_{\mathrm{A1}}=\delta \left(2\pi \left({\widehat{f}}_m-f_{\mathrm{d}}\right)\right),$ $C_{\mathrm{A2}}=\delta \left(2\pi \left({\widehat{f}}_m-f_{\mathrm{d}}\right)\right)\mathrm{F}{\mathrm{T}}_{-{\phi }_0}\left({\widehat{f}}_m\right),$ $C_{\mathrm{A3}}=\delta \left(2\pi \left({\widehat{f}}_m-f_{\mathrm{d}}\right)\right)\mathrm{F}{\mathrm{T}}_{{\phi }_0}\left({\widehat{f}}_m\right).$

It can be seen from equation (23) that the range profile has three $\text{sinc}$ functions with three peaks at ${\tau }_0-\frac{k_{\theta }}{2\pi },$ ${\tau }_0-\frac{k_{\theta }}{2\pi }-f_{\mathrm{A}}$ and ${\tau }_0-\frac{k_{\theta }}{2\pi }+f_{\mathrm{A}},$ respectively. The above result suggests that the time-varying plasma sheath will cause an absolute echo delay offset of $\frac{k_{\theta }}{2\pi }$ from the actual echo delay ${\tau }_0$ and produce two false scatterings. These scatterings distribute on the left and right sides of the real scattering, exhibiting a relative echo delay offset of $f_{\mathrm{A}}$ from the real one.

Figure 8 shows the range profile. In order to conduct a fair comparison, the range profile without plasma sheath is shown in figure 8(a). The abscissa is the distance corresponding to echo delay, the peak position represents the distance of the scattering. It can be seen from figure 8(a) that when the plasma sheath is not considered, single scattering represents a single peak, occurring at the position of 50 000 m (actual distance of the scattering). This position is assumed as the actual position of scattering.

Figure  8.  (a) Range profile without plasma sheath enveloping, (b) range profile enveloped with time-varying plasma sheath.

DownLoad: Full-Size Img PowerPoint

Figure 8(b) demonstrates the range profile enveloped with time-varying plasma sheath. It can be seen that three peaks occur, among which the real scattering locates on the right of 50 000 m and the other two false scatterings distribute on both sides of the real one. This phenomenon demonstrates that the absolute echo delay offset $\frac{k_{\theta }}{2\pi }$ exists between the real peak and the actual position of scattering, and that the relative echo delay offset $f_{\mathrm{A}}$ exists between the real and the false scatterings.

4.2   Calculation of scattering position on range dimension

In equation (23), the offset parameters $\frac{k_{\theta }}{2\pi }$ and $f_{\mathrm{A}}$ represent the values of echo delay. The function relation between distance and echo delay can be expressed as

Then the distance offset corresponding to $\frac{k_{\theta }}{2\pi }$ and $f_{\mathrm{A}}$ can be written as

where $\mathrm{∆}{d}_{\theta }$ is the absolute distance offset on range dimension between the real scattering and the actual position, $\mathrm{∆}{d}_{\mathrm{A}}$ is the relative distance offset on range dimension between the real and false scatterings.

For LFM waves, the signal frequency traverses the whole bandwidth during pulse width. This indicates that the fluctuation of electron density during pulse width matches with the amplitude fluctuation of reflection coefficient in signal bandwidth.

Then, the expression of $f_{\mathrm{A}}$ can be written as

where $B$ is the bandwidth.

By substituting equation (27) into (26), we obtain

It can be seen that $\mathrm{∆}{d}_{\mathrm{\theta }}$ is correlated with the phase-frequency characteristics of the plasma sheath's reflection coefficient, and that $\mathrm{∆}{d}_{\mathrm{A}}$ is proportional to the fluctuation frequency of electron density $f_{N_{\mathrm{e}}}.$

5.   Influence mechanism on azimuth dimension

The azimuth dimension correlates with the Doppler frequency that is caused by scattering rotation, to which multiple echo data are needed to conduct coherent analysis.

5.1   Formation mechanism of Doppler diffusion

For ease of analysis on range dimension, independent parameters of slow-time are replaced with constants, hence, equation (22) can be rewritten as

where

The initial phase ${\phi }_0\left({\widehat{t}}_m\right)$ is the phase of plasma reflection coefficient amplitude curve when the pulse transmitted at ${\widehat{t}}_m$ time enters into the plasma sheath, which can be considered as sampling operation to the sinusoidal curve of the reflection coefficient amplitude.

The sampling frequency is $f_{\mathrm{r}},$ and the fluctuation frequency of amplitude curve is the same as the electron density $f_{N_{\mathrm{e}}},$ which ranges from 20 up to 100 kHz, exceeding $f_{\mathrm{r}}.$ For most of the time, $f_{N_{\mathrm{e}}}$ is not an integral multiple of $f_{\mathrm{r}}.$ Therefore, the initial phases corresponding to each echo ${\phi }_0\left({\widehat{t}}_m\right)$ will differ.

When ${\widehat{t}}_m={\widehat{t}}_1,{\widehat{t}}_2,{\widehat{t}}_3,···{\widehat{t}}_M,$ the initial phases of all echoes will form a new sinusoidal fluctuation with a new frequency, which is referred to as the initial phase fluctuation frequency, as shown in figure 9.

Figure  9.  Electron density fluctuation and initial phase fluctuation.

DownLoad: Full-Size Img PowerPoint

The initial phase fluctuation frequency is denoted as $f_{\mathrm{IP}},$ so the initial phase ${\phi }_0\left({\widehat{t}}_m\right)$ can be expressed as

Therefore, $\mathrm{F}{\mathrm{T}}_{-{\phi }_0}\left({\widehat{f}}_m\right)$ and $\mathrm{F}{\mathrm{T}}_{{\phi }_0}\left({\widehat{f}}_m\right)$ can be expressed as

By substituting equations (31) and (32), equation (29) can be rewritten as

It can be seen from the above equation (33) that the azimuth profile has three $\delta$ functions with three peaks at $f_{\mathrm{d}},$ $f_{\mathrm{d}}-f_{\mathrm{IP}}$ and $f_{\mathrm{d}}+f_{\mathrm{IP}},$ which are the Doppler frequency of the real scattering and the other two false scatterings, respectively.

Figure 10 shows the azimuth profile, in which figure 10(a) is the azimuth profile without plasma sheath enveloping. The curve peak represents the Doppler frequency of the real scatter, which is denoted as $f_{\mathrm{d}}.$ Figure 10(b) is the azimuth profile enveloped with time-varying plasma sheath.

Figure  10.  (a) The azimuth profile without plasma sheath enveloping, (b) the azimuth profile enveloped with time-varying plasma sheath.

DownLoad: Full-Size Img PowerPoint

In response to figure 10, the blue curve represents the azimuth profile with the range cell of $t_n={\tau }_0-\frac{k_{\theta }}{2\pi },$ the red curve and green curve represents the azimuth profile with the range cell of $t_n={\tau }_0-\frac{k_{\theta }}{2\pi }-f_{\mathrm{A}}$ and $t_n={\tau }_0-\frac{k_{\theta }}{2\pi }+f_{\mathrm{A}},$ respectively. Accordingly, the peak of the blue curve is Doppler frequency $f_{\mathrm{d}},$ the red is $f_{\mathrm{d}}-f_{\mathrm{IP}}$ and the green is $f_{\mathrm{d}}+f_{\mathrm{IP}}.$

5.2   Doppler frequency calculation of false scatterings on azimuth dimension

Due to the asynchronization existing between the fluctuation frequency of electron density and the pulse repetition frequency (PRF), the initial phase fluctuation frequency does not have a fixed value, which can be expressed as

where $f_{\mathrm{r}}$ is the PRF, $k$ is an integer.

Considering the limitation of Doppler ambiguity, the Doppler frequencies of the aforementioned two false scatterings are variable between the interval of $\left[-\frac{f_{\mathrm{r}}}{2},\frac{f_{\mathrm{r}}}{2}\right],$ then $k$ requires the following conditions to be satisfied

This suggests that the Doppler frequencies of the false scatterings can be any arbitrary value of the frequency ranging from $-\frac{f_{\mathrm{r}}}{2}$ to $\frac{f_{\mathrm{r}}}{2}$ with a certain combination of $f_{N_{\mathrm{e}}}$ and $f_{\mathrm{r}}.$

When $f_{N_{\mathrm{e}}}$ is an integral multiple of $f_{\mathrm{r}},$ the value of $f_{\mathrm{IP}}$ is zero, at which moment these two false scatterings have the same Doppler frequency $f_{\mathrm{d}}$ as that of the real one.

6.   Simulation analysis on ISAR imaging

In this section, we analyze the simulation results of ISAR imaging, in which the modulation type of radar signal is LFM, the carrier frequency is 10 GHz, the bandwidth is 1 GHz, the pulse width is 100 μs, the PRF $f_{\mathrm{r}}$ is 1 kHz. The peak electron density $N_{\mathrm{e}}^{\mathrm{peak}},$ the plasma thickness $z_{\max },$ the collision frequency $v_{\mathrm{e}}$ and other parameters originate from the computational-fluid-dynamics' flow-field simulation data of RAM-C for the reentry object with the altitude of 50 km and the speed of 15 Mach. The above parameters are listed out in table 1.

Table  1.  Values of $N_{\mathrm{e}}^{\mathrm{peak}},$ $z_{\max }$ and $v_{\mathrm{e}}.$

Altitude	Speed	$N_{\mathrm{e}}^{\mathrm{peak}}$	$z_{\max }$	$v_{\mathrm{e}}$
50 km	15 Mach	$2.26\times {10}^{18}{\mathrm{m}}^{-3}$	0.06 m	2.52 GHz

 | Show Table

DownLoad: CSV

Based on the analysis results of ISAR imaging effect on range and azimuth dimensions, different fluctuation frequencies of the electron density are set as some specific values to iterate the possibilities of various defocusing results on range and azimuth dimensions.

6.1   Simulation results of false scattering distribution on range dimension

In order to simulate the distribution of the false scatterings on range dimension, the fluctuation frequencies of electron density were set from 20 to 100 kHz with 20 kHz interval, during which the radar system parameters are considered. The value of $\mathrm{∆}{d}_{\mathrm{A}}$ was calculated using equation (28), $k$ and $f_{\mathrm{IP}}$ were calculated using equations (34) and (35), to which the results were presented in table 2. $\mathrm{∆}{d}_{\theta }$ is the same value at different electron density fluctuation frequencies. The value of $\mathrm{∆}{d}_{\theta }$ is 0.036 m, which is calculated using equation (25).

Table  2.  Values of $f_{N_{\mathrm{e}}},$ $\mathrm{∆}{d}_{\mathrm{A}},$ $k$ and $f_{\mathrm{IP}}.$

$f_{N_{\mathrm{e}}}$	20.0 kHz	40.0 kHz	60.0 kHz	80.0 kHz	100.0 kHz
$\mathrm{∆}{d}_{\mathrm{A}}$	0.3 m	0.6 m	0.9 m	1.2 m	1.5 m
$k$	20	40	60	80	100
$f_{\mathrm{IP}}$	0 Hz	0 Hz	0 Hz	0 Hz	0 Hz

 | Show Table

DownLoad: CSV

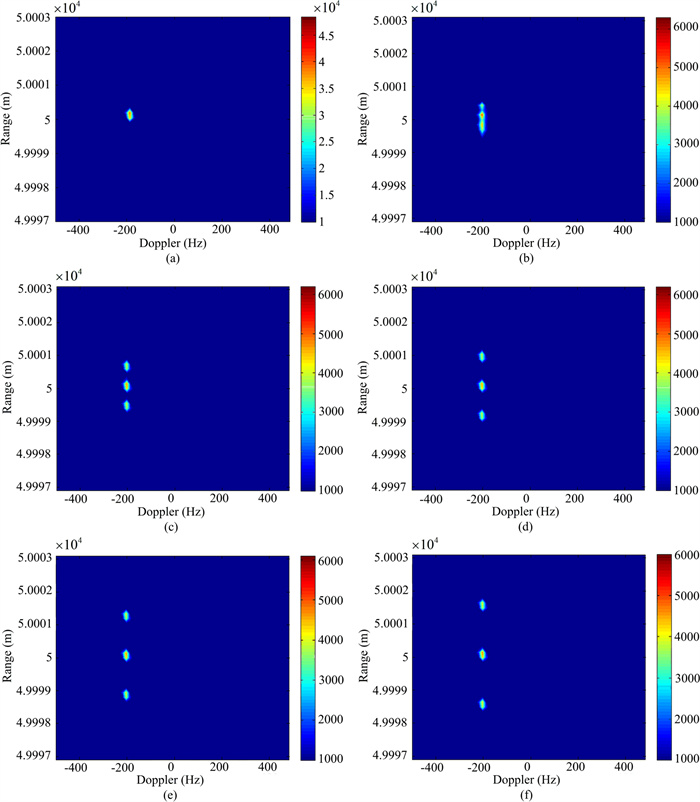
The ISAR imaging results of single scattering enveloped with time-varying plasma sheath featuring different electron density fluctuation frequencies are shown in figure 11. The ISAR image without plasma sheath enveloping is shown in figure 11(a). The range of real scattering is 50 km, and the Doppler frequency is -200 Hz. Figure 11(b) is the ISAR image of single scattering enveloped with 20 kHz time-varying plasma sheath. Two false scatterings appear above and below the real scattering respectively, and the relative distance offset between real and false scatterings is 0.3 m. The Doppler frequency of false scatterings is the same as the real one, suggesting that the value of $f_{\mathrm{IP}}$ is 0 Hz.

Figure  11.  (a) ISAR image without plasma sheath, (b) ISAR image with time-varying plasma sheath when $f_{N_{\mathrm{e}}}=20\mathrm{kHz},$ (c) ISAR image with time-varying plasma sheath when $f_{N_{\mathrm{e}}}=40\mathrm{kHz},$ (d) ISAR image with time-varying plasma sheath when $f_{N_{\mathrm{e}}}=60\mathrm{kHz},$ (e) ISAR image with time-varying plasma sheath when $f_{N_{\mathrm{e}}}=80\mathrm{kHz},$ (f) ISAR image with time-varying plasma sheath when $f_{N_{\mathrm{e}}}=100\mathrm{kHz}.$

DownLoad: Full-Size Img PowerPoint

Figures 11(c)–(f) are the ISAR images with electron density fluctuation frequencies at 40–100 kHz, from which it can be seen that the higher electron density fluctuation frequency, the larger relative distance offset between false scatterings and the real one.

6.2   Simulation results of false scatterings distribution in azimuth dimension

In order to simulate the distribution of the false scatterings in azimuth dimension, considering the radar system parameters, the frequencies of electron density fluctuation were set from 50 to 50.5 kHz with 0.1 kHz interval. The Doppler frequency of real scattering $f_{\mathrm{d}}$ is -200 Hz, the values of $\mathrm{∆}{d}_{\mathrm{A}}$ and $f_{\mathrm{IP}}$ were calculated. The results are listed in table 3.

Table  3.  Values of $f_{N_{\mathrm{e}}},$ $\mathrm{∆}{d}_{\mathrm{A}},$ $k,$ $f_{\mathrm{IP}},$ $f_{\mathrm{d}}-f_{\mathrm{IP}}$ and $f_{\mathrm{d}}+f_{\mathrm{IP}}.$

$f_{N_{\mathrm{e}}}$	50.0 kHz	50.1 kHz	50.2 kHz	50.3 kHz	50.4 kHz	50.5 kHz
$\mathrm{∆}{d}_{\mathrm{A}}$	0.75 m	0.7515 m	0.753 m	0.7545 m	0.756 m	0.7575 m
$k$	50	50	50	50	50	50
$f_{\mathrm{IP}}$	0 Hz	100 Hz	200 Hz	300 Hz	400 Hz	500 Hz
$f_{\mathrm{d}}-f_{\mathrm{IP}}$	-200 Hz	-300 Hz	-400 Hz	-500 Hz	500 Hz	400 Hz
$f_{\mathrm{d}}+f_{\mathrm{IP}}$	-200 Hz	-100 Hz	0 Hz	100 Hz	200 Hz	300 Hz

 | Show Table

DownLoad: CSV

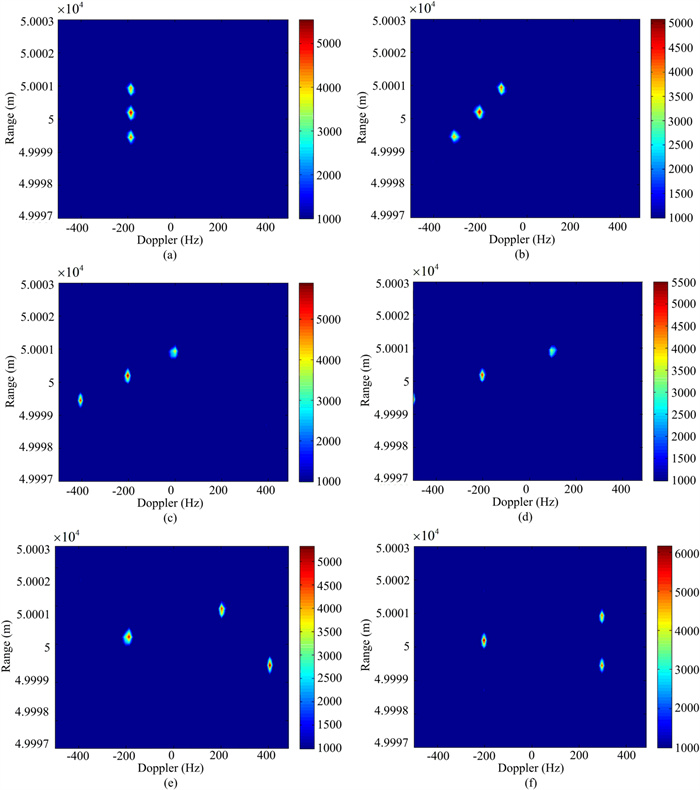
The ISAR imaging results of single scattering with different electron density fluctuation frequencies of time-varying plasma sheath are shown in figure 12. When $f_{N_{\mathrm{e}}}$ is 50 kHz, the real scattering is accompanied with two false scatterings above and below it. Since $f_{\mathrm{IP}}$ is 0 Hz, the real and the false scatterings have the same Doppler frequency -200 Hz, which is shown in figure 12(a). When $f_{N_{\mathrm{e}}}$ is 50.1 kHz, $f_{\mathrm{IP}}$ increases to 100 Hz, the Doppler frequencies of false scatterings ( $f_{\mathrm{d}}-f_{\mathrm{IP}}$ and $f_{\mathrm{d}}+f_{\mathrm{IP}}$ ) are no longer the same as that of the real one, and the false scatterings move along the azimuth dimension.

Figure  12.  (a) ISAR image with time-varying plasma sheath when $f_{N_{\mathrm{e}}}=50\mathrm{kHz},$ (b) ISAR image with time-varying plasma sheath when $f_{N_{\mathrm{e}}}=50.1\mathrm{kHz},$ (c) ISAR image with time-varying plasma sheath when $f_{N_{\mathrm{e}}}=50.2\mathrm{kHz},$ (d) ISAR image with time-varying plasma sheath when $f_{N_{\mathrm{e}}}=50.3\mathrm{kHz},$ (e) ISAR image with time-varying plasma sheath when $f_{N_{\mathrm{e}}}=50.4\mathrm{kHz},$ (f) ISAR image with time-varying plasma sheath when $f_{N_{\mathrm{e}}}=50.5\mathrm{kHz}.$

DownLoad: Full-Size Img PowerPoint

As shown in figure 12(b), the Doppler frequency of false scattering farther in range increases $f_{\mathrm{IP}},$ moving to right, whereas the false scattering closer in range reduces $f_{\mathrm{IP}},$ moving to left. Figures 12(c)–(f) are the ISAR images with electron density fluctuation frequencies from 50.2 to 50.5 kHz, $\mathrm{∆}{d}_{\mathrm{A}}$ of various frequencies exhibits small differences, and $f_{\mathrm{IP}}$ increases with the increasing of the frequency $f_{N_{\mathrm{e}}}.$ The Doppler frequency offset between the real and false scattering in azimuth dimension increases with $f_{\mathrm{IP}}.$ When the Doppler frequency of the false scattering exceeds $\pm \frac{f_{\mathrm{r}}}{2},$ the false scattering folds over to the other half of the ISAR image, as shown in figures 12(e) and (f).

6.3   Simulation and evaluation on ISAR imaging of object enveloped with time-varying plasma sheath

In this subsection, the distribution of false scatterings on the range and azimuth dimensions is analyzed, which correlates with the electron density fluctuation frequency. The objective of the aforementioned study is single scattering, hereinafter the analysis focuses on multiple scatterings of the plasma-sheath-enveloped object (reentry spacecraft model), after which the quality of defocused ISAR imaging is evaluated.

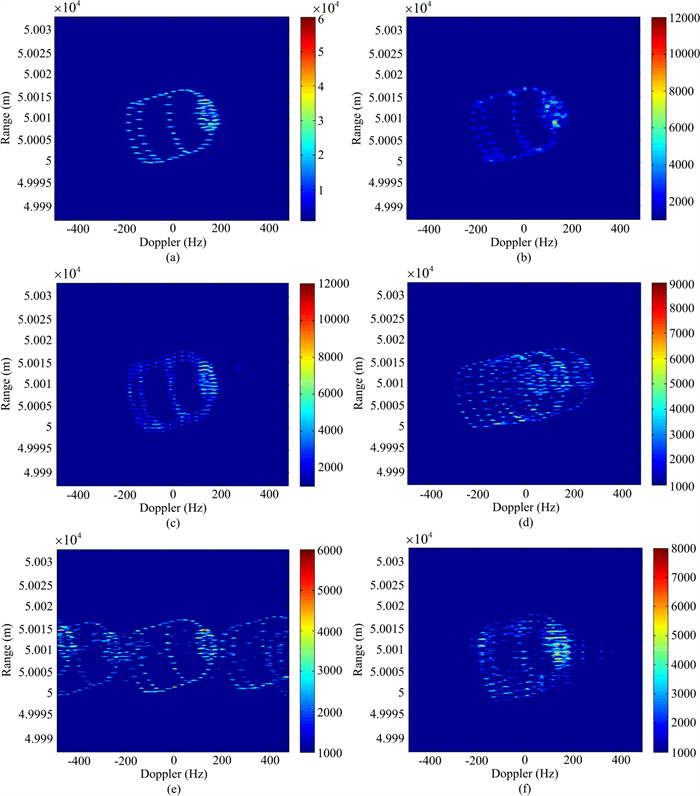
Figure 13 shows the ISAR imaging results of the reentry object with different electron density fluctuation frequencies. Specifically, figure 13(a) is the ISAR image of the object without plasma sheath enveloping, in which all the scatterings consisting of the object image can be clearly identified. Figure 13(b) is the ISAR imaging of the object with a 20 kHz plasma sheath which begins to blur. Figure 13(c) is the ISAR imaging of the object with a 50 kHz plasma sheath, whose blurry profile expands along the range dimension with a more obvious trend. Figure 13(d) is the ISAR imaging of the object with a 50.1 kHz plasma sheath, expanding along the range and azimuth dimensions. The expansion along the azimuth dimension is greater than that along the range dimension, which appears to be an overlapping of three object images of one real image and two false images. Figure 13(e) is the ISAR imaging of the object with a 50.4 kHz plasma sheath, whose expansion along the azimuth dimension is further increased. The false images separate from the real image, which may result in the misjudgment of ISAR imaging on the number of reentry objects. Figure 13(f) is the ISAR imaging of the object with a 100 kHz plasma sheath, in which the expansion does not exist on the azimuth dimension but exists on the distance dimension significantly.

Figure  13.  (a) ISAR image without plasma sheath, (b) ISAR image with time-varying plasma sheath when $f_{N_{\mathrm{e}}}=20\mathrm{kHz},$ (c) ISAR image with time-varying plasma sheath when $f_{N_{\mathrm{e}}}=50\mathrm{kHz},$ (d) ISAR image with time-varying plasma sheath when $f_{N_{\mathrm{e}}}=50.1\mathrm{kHz},$ (e) ISAR image with time-varying plasma sheath when $f_{N_{\mathrm{e}}}=50.4\mathrm{kHz},$ (f) ISAR image with time-varying plasma sheath when $f_{N_{\mathrm{e}}}=100\mathrm{kHz}.$

DownLoad: Full-Size Img PowerPoint

In order to evaluate the influence of false scatterings imposed on ISAR imaging, peak signal-to-noise ratio (PSNR) and structural similarity (SSIM) [30] were used to evaluate the results of ISAR imaging with plasma sheath.

PSNR is a common objective method being used to evaluate image quality. Supposing that the original image is $P\left(u,v\right)$ and the noisy image is $Q\left(u,v\right),$ then the mean square error (MSE) can be expressed as

where the two images have the same size $U\times V.$

PSNR can be obtained using the following equation

where $\mathrm{M}\mathrm{A}\mathrm{X}$ is the maximum pixel value of the image. A larger PSNR value implies less distortion and higher image quality.

SSIM is used to measure the similarity of two images. According to the realization of SSIM theory includes evaluation of brightness, contrast and structure. The expression can be obtained as

where ${\mu }_{\mathrm{P}}$ and ${\mu }_{\mathrm{Q}}$ are means of two images, ${\sigma }_{\mathrm{P}}^2$ and ${\sigma }_{\mathrm{Q}}^{\mathrm{2}}$ are standard deviations, ${\sigma }_{\mathrm{PQ}}$ is covariances of $P\left(u,v\right)$ and $Q\left(u,v\right).$ $h_1$ and $h_2$ are stability constants. The value of SSIM ranges from 0 to 1. A larger SSIM value suggests higher similarity between original and noisy images.

The values of PSNR and SSIM jointly determine the level of image deterioration. The corresponding evaluation results are listed in table 4. It can be found that $f_{N_{\mathrm{e}}}$ affects the values of PSNR and SSIM when $f_{\mathrm{IP}}=0,$ suggesting that $f_{N_{\mathrm{e}}}$ is negatively correlated with the values of PSNR and SSIM. When $f_{\mathrm{IP}}\ne 0,$ $f_{\mathrm{IP}}$ and $f_{N_{\mathrm{e}}}$ jointly affect the values of PSNR and SSIM, which implies that the relation between $f_{N_{\mathrm{e}}}$ and $f_{\mathrm{r}}$ imposes significant influence on the values of PSNR and SSIM.

Table  4.  Evaluation results of the influence on ISAR image imposed by time-varying plasma sheath.

$f_{N_{\mathrm{e}}}$	20.0 kHz	50.0 kHz	50.1 kHz	50.5 kHz	100.0 kHz
$\mathrm{∆}{d}_{\mathrm{A}}$	0.3 m	0.75 m	0.7515 m	0.7575 m	1.5 m
$f_{\mathrm{IP}}$	0 Hz	0 Hz	100 Hz	400 Hz	0 Hz
PSNR	28.45 dB	25.83 dB	22.67 dB	21.94 dB	23.36 dB
SSIM	0.747	0.546	0.335	0.269	0.391

 | Show Table

DownLoad: CSV

7.   Conclusions

With respect to reentry objects enveloped with time-varying plasma sheaths, this work analyzes the formation mechanism and the distribution rules of the ISAR imaging defocus. In this study, the TLM method is used to investigate the reflection coefficient of the plasma-sheath-enveloped object. Our research findings suggest that the coupling effect of echoes and reflection coefficient results in abnormal pulse compression results and the Doppler frequency diffusion, causing ISAR imaging defocus eventually. Furthermore, the corresponding analysis is conducted in terms of the range and azimuth dimensions.

In response to the range dimension, two false scatterings are caused by a single scattering of the object forms after pulse compression, distributing evenly on both sides of the real scattering. The real scattering is displaced from the actual position in distance and the absolute distance offset can be attributed to the phase-frequency characteristic of the reflection coefficient. The relative distance offset between the real scattering and either false scattering correlates with the electron density fluctuation frequency.

Regarding the azimuth dimension, the false scatterings have an additional Doppler frequency $f_{\mathrm{IP}}$ confirmed by combined values of $f_{N_{\mathrm{e}}}$ and $f_{\mathrm{r}},$ in which one false scattering has Doppler frequency $f_{\mathrm{d}}+f_{\mathrm{IP}}$ whereas the other one has Doppler frequency $f_{\mathrm{d}}-f_{\mathrm{IP}}.$ The distribution of the false scatterings on the azimuth dimension seriously impacts the ISAR images, thereby deteriorating the image quality.

Nevertheless, certain complicated changes in the electron density fluctuation frequencies and in the reentry object's appearance were not fully considered. This paper investigates the formation mechanism of the false scatterings and the property of the ISAR imaging defocus of reentry objects enveloped with time-varying plasma sheath. Our future research interest will be focusing on these changes and variations, laying a theoretical foundation for ISAR imaging and its applications in various fields.