Influence of adiabatic response of passing energetic ions on high-frequency fishbone

1.   Introduction

Fishbone instability driven by trapped energetic ions, first discovered in poloidal divertor experiment (PDX) plasmas [1], has drawn a great deal of attention. Later, fishbone instabilities were then observed in PBX [2], JET [3], DIII-D [4], HL-2A [5–8] and EAST [9]. These instabilities accompanied by a loss of energetic particles result in the reduction of heating efficiency. The fishbone instability observed in experiments is known to be excited by energetic particles, which can be one of trapped energetic ions, passing energetic ions or superthermal electrons, under different resonance conditions. For instance, the mode frequency of the fishbone instability was observed to be close to the toroidal precession frequency of the trapped energetic ions in the PDX while in the PBX it is around the toroidal circulation frequency of passing energetic ions [1, 2].

Based on these theories, we can understand reasonably well the physical mechanism of fishbone instability [10–14]. Fishbone instabilities are internal kink modes with dominant poloidal and toroidal wave numbers $m=1$ and $n=1$, and can be resonantly excited by energetic ions produced by both perpendicular and tangential neutral beam injections (NBIs) [1, 2]. With the perpendicular injection, there are two theoretical explanations for the exciting conditions of fishbone instability: first, the mode frequency is comparable to the toroidal precession frequency of the trapped energetic ions [10] while the mode is considered to be an energetic particle mode (EPM); the second is that the mode frequency is close to the thermal ion diamagnetic frequency [11] while the mode is considered to be a 'gap' mode. With the tangential injection, theoretical prediction indicates that the fishbone instability has two branches: low- [12] and high-frequency branches [13]. The former's mode frequency close to the thermal ion diamagnetic frequency is modeled as a 'gap' mode, and the latter's mode frequency determined by the toroidal circulation frequency of passing energetic ions is considered to be an EPM. The resonance condition of the high-frequency branch can be specifically expressed as ${\omega }_{\varphi }+p{\omega }_{\theta }-\omega =0$, where ${\omega }_{\varphi }$ and ${\omega }_{\theta }$ are the circulation frequencies in the toroidal and poloidal directions, respectively, and $p=0$ is for the EPM branch of high-frequency fishbone driven by passing energetic ions [13]. To date, the EPM branch of low-frequency fishbone for $p=-1$ has been well documented [14]. In this work, we focus mainly on the high-frequency EPM branch with $p=0.$

The ideal magnetohydrodynamic (MHD) description is usually employed to treat the thermal plasma in tokamaks. However, to handle the energetic ions the drift-kinetic description is often adopted, instead. By linearizing a drift-kinetic equation, we can obtain the adiabatic response (fluid contribution) and nonadiabatic response (kinetic contribution) of energetic ions [15]. When the ion energy is high, the ion collision frequency is low (compared to the Alfvén frequency). The drift-kinetic description is obviously more appropriate for the passing energetic ions driving high-frequency fishbone instability, i.e. the nonadiabatic response (which represents a resonance interaction) plays a dominant role. However, as they slow down, the energetic ions gradually approach Maxwellian distribution, and the contribution of adiabatic response becomes more important. Thus, nonadiabatic response alone becomes inadequate for representing the energetic ions in the course of the ions slowing down, as with most scenarios in tokamaks. In other words, both the adiabatic and nonadiabatic responses are important for the energetic ions in the intermediate energy range. However, in a previous work [13, 16], the contribution of adiabatic response to high-frequency fishbone instability has not been taken into account. Therefore, it is important to study the role of adiabatic response of passing energetic ions in high-frequency fishbone instability.

This paper is organized as follows. In section 2, the fishbone dispersion relation including the contribution of adiabatic response is derived. In section 3, the potential energy arising from the adiabatic contribution of energetic ions is deduced by simplifying the generic slowing-down distribution function. In section 4, the numerical results obtained using the extended dwk++ [16] code in the present work are then compared with our analytical results to verify the modified code with the simplified slowing-down distribution function. Then, the effects of the distribution function parameters, such as beam ion radial profile, critical energy, pitch parameter distribution and beam ion drift orbit width on fishbone instability are studied. Finally, conclusions are given in section 5.

2.   Fishbone dispersion relation including adiabatic contribution

In ideal MHD modes, the perturbation distribution function of passing energetic ions [15] can be expressed as the sum of the nonadiabatic and the adiabatic responses, $\delta F_{\mathrm{h}}=\delta F_{\mathrm{hk}}+\delta F_{\mathrm{hf}}$, where $\delta F_{\mathrm{hk}}$ and $\delta F_{\mathrm{hf}}$ correspond to the nonadiabatic and the adiabatic responses, respectively. The adiabatic response [17] can be written as,

where ${\xi }_r$ is the radial displacement and $q$ is the safety factor. Here, the coordinate of the minor radius, $r=\overline{r}+{\rho }_{\mathrm{d}}\cos \theta$, where $\overline{r}$ is the mean particle radius, $\theta$ is the poloidal angle, and the finite drift orbit width (FOW) [18], ${\rho }_{\mathrm{d}}\left(r,\mathrm{\Lambda },\mathit{ϵ}\right)=\left(q{\rho }_0/2\right)\sqrt{\mathit{ϵ}/\left(1-\mathrm{\Lambda }/b\right)}\left[\mathrm{\Lambda }/b+2\left(1-\mathrm{\Lambda }/b\right)\right]$, in which $\mathit{ϵ}=v^2/2$ is the energy of particle, ${\rho }_0$ is the gyro radius with injection energy, $\mathrm{\Lambda }=\mu B_0/\mathit{ϵ}$ is the pitch parameter, $\mu =v_{\perp }^2/2B$ is the magnetic moment, and the inverse of normalized magnetic field $b=B_0/B.$ Considering that energetic ions are produced by tangential NBI, a generalized energetic ion distribution function by using the slowing-down equilibrium distribution function for the energy of energetic ions and introducing Gaussian-type factors representing their pitch and radial dependences $F_{\mathrm{h}}$ can be expressed in the following form [16]:

In this expression, $C_{\mathrm{f}}={\displaystyle \int {\mathrm{d}}^{3}v}\mathrm{erfc}\left({\displaystyle \frac{\mathit{ϵ}-{\mathit{ϵ}}_0}{\mathrm{∆}\mathit{ϵ}}}\right)\exp \left[-{\left({\displaystyle \frac{\mathrm{\Lambda }-{\mathrm{\Lambda }}_0}{\mathrm{∆}\mathrm{\Lambda }}}\right)}^2\right]/\left({\mathit{ϵ}}^{3/2}+{\mathit{ϵ}}_{\mathrm{c}}^{3/2}\right)$ is the normalization factor, ${\mathrm{d}}^{3}v=\sqrt{2}\pi /\left(b\sqrt{1-\mathrm{\Lambda }/b}\right)\mathrm{d}\mathrm{\Lambda }{\mathit{ϵ}}^{1/2}\mathrm{d}\mathit{ϵ}$, ${\mathit{ϵ}}_{\mathrm{c}}$ is the critical energy, ${\mathit{ϵ}}_0$ is the injection energy of the beam ions, erfc is the complementary error function, and parameters $r_0$ and ${\mathrm{\Lambda }}_0$ represent at which position the peaks of $F_{\mathrm{h}}$ occur in the radial position and pitch parameter, respectively. In equation (2), $\mathrm{∆}\mathit{ϵ}$, $\mathrm{∆}r$ and $\mathrm{∆}\mathrm{\Lambda }$ are the characteristic widths of energy, radial and pitch distribution, respectively. The potential energy arising from the adiabatic contribution of energetic ions can be written in the following form [14, 17]:

where ${\mathrm{d}}^{3}x=2\pi r{R}_0\left(1+2\epsilon \cos \theta \right)\mathrm{d}r\mathrm{d}\theta$, $R_0$ is the major radius, $\epsilon$ is the inverse aspect ratio, $\mathit{\kappa }=\mathbf{b}·\mathrm{\nabla }\mathbf{b}$ is the magnetic field line curvature, ${\mathit{\xi }}_{\perp }^{*}$ is the conjugate of perpendicular displacement vector, and its specific form, which is assumed to be an internal kink-like mode structure, is given by equations (9) and (10) in [16]. According to [16, 19], the nonadiabatic contribution $\delta W_{\mathrm{hk}}$ from nonadiabatic response of energetic ions is given by,

Here, is a Jacobian of the coordinate, is the transit period of energetic particles [15] and is the transit frequency [20]. The quantity is the diamagnetic drift frequency, where and is the minor radius, and other quantities are defined as follows: $Y_p^\sigma(\Lambda, \bar{r} ; \sigma)=\tau_{\mathrm{b}}^{-1} \oint \mathrm{d} \tau G(\tau) \exp \left(-\mathrm{i} p \omega_{\mathrm{b}} \tau\right)$, where , , for the direction of , and is the transit harmonic. For convenience of physical analysis represented in section 4, it is important to note that and , which appear in equation (4), are damping and driving terms, respectively.

Based on the generalized variational principle, we assume that the internal kink mode is marginally unstable, i.e. $\delta W_{\mathrm{MHD}}\approx 0$, and subsequently obtain the following fishbone dispersion relation [16]:

where $\overline{\omega }=\omega /{\omega }_0$, ${\omega }_0=v_0/R_0$, ${\omega }_{\mathrm{A}}=v_{\mathrm{A}}/\left(3^{1/2}{R}_{0}s\right)$, $s=r_{\mathrm{s}}\mathrm{d}{q}_{r=r_{\mathrm{s}}}/\mathrm{d}r$, $v_{\mathrm{A}}=B/\sqrt{{\mu }_0{\rho }_{\mathrm{m}}}$, ${\rho }_{\mathrm{m}}=m_{\mathrm{i}}{n}_{\mathrm{i}}$, $v_0$ is the injection velocity, $r_{\mathrm{s}}$ is the radial position of $q=1$, ${\xi }_0$ is the amplitude of the displacement vector, ${\beta }_{\mathrm{h},0}=8\pi n_0{T}_0/B^2$, $C_{\mathrm{p}}=p_0/n_0{T}_0$, $p_0$ is the pressure of energetic ions at $\overline{r}=r_0$, $n_0$ is the density of energetic ions at $\overline{r}=r_0$, $T_0$ is the temperature corresponding to the injection energy, $\delta {\overline{W}}_{\mathrm{hk}}=\delta W_{\mathrm{hk}}/{\pi }^2{a}^2{R}_0{n}_0{T}_0$, $\delta {\overline{W}}_{\mathrm{hf}}=\delta W_{\mathrm{hf}}/{\pi }^2{a}^2{R}_0{n}_0{T}_0.$ This dispersion relation including adiabatic response will be solved numerically in section 4. However, we first derive analytically the potential energy arising from the adiabatic contribution of energetic ions to verify the modified code with a simplified slowing-down distribution function [14].

3.   Analytical description of adiabatic contribution for passing energetic ions

To obtain a clear expression of the dispersion relation, a simplified slowing-down distribution function [14] is employed:

where $P_{\mathrm{h}}$ is the energetic ion pressure and $H\left(x\right)$ is the Heaviside step function. With $\overline{r}=r-{\rho }_{\mathrm{d}}\cos \theta$, ${\xi }_r={\xi }_{0}H\left(r_{\mathrm{s}}-r\right)\exp \left(\mathrm{i}\left(\varphi -\theta -\omega t\right)\right)$, we expand equation (1) around $\overline{r}$ to first order in ${\rho }_{\mathrm{d}}/\overline{r}$ by using $r/\overline{r}=1+{\rho }_{\mathrm{d}}/\overline{r}\cos \theta$, $q\left(\overline{r}\right)/q\left(r\right)=1-\partial {\rho }_{\mathrm{d}}/\partial \overline{r}\cos \theta$, where $\partial {\rho }_{\mathrm{d}}/\partial \overline{r}={\rho }_{\mathrm{d}}S\left(\overline{r}\right)/\overline{r}$, $S\left(\overline{r}\right)=\left(\overline{r}\mathrm{d}q/\mathrm{d}\overline{r}\right)/q$, and quadratic terms in ${\rho }_{\mathrm{d}}/\overline{r}$ are ignored. Thus, from equation (1) we arrive at,

With the flux coordinates, ${\kappa }_r=-\cos \theta /R$, ${\kappa }_{\theta }=\epsilon \sin \theta$, ${\xi }_{\theta }=-\mathrm{i}r{\xi }_r$, and metric coefficients can be written as $g^{rr}=1+\epsilon \cos \theta /2$, $g^{r\theta }=-3\epsilon \sin \theta /2r$, and $g^{\theta \theta }=\left(1-5\epsilon \cos \theta /2\right)/r^2$, respectively [21]. Ignoring first-order terms of ${\rho }_{\mathrm{d}}/R$, this gives,

Compared to ${\mathit{\xi }}_{\perp }^{*}·\mathit{\kappa }=-{\xi }_{0}H\left(r_{\mathrm{s}}-\overline{r}\right)/R$ used in [17], we retain ${\mathit{\xi }}_{\perp }^{*}·\mathit{\kappa }$ up to the first order of the inverse aspect ratio $\epsilon$, which makes the integral of equation (3) more accurate than that in a previous work [17]. Substituting equations (6)–(8) in equation (3), $\delta W_{\mathrm{hf}}$ can easily be written in the following form:

where

Here, $P_{\mathrm{h}||}=2P_{\mathrm{h}}.$ Equation (10) comes from the contribution of the lowest order in ${\rho }_{\mathrm{d}}/\overline{r}$ while equation (11) is the contribution of the FOW.

Furthermore, following equation (19) in [16] the real part of the dispersion relation including the adiabatic contribution is given by,

where ${\mathrm{\Omega }}_{\mathrm{r}}={\omega }_{\mathrm{r}}/\left(v_0/R_0\right)$, ${\omega }_{\mathrm{r}}$ is the real part of $\omega .$ The last term on the left-hand side of the equation (12) represents the adiabatic contribution. We assume a polynomial $q$ profile, $q=q_0+q_1{r}^2$, and the energetic ion radial profile $P_{\mathrm{h}||}=p_0\exp \left(-{\left(\overline{r}/\mathrm{∆}r\right)}^2\right).$ For PBX parameters, $B=0.84\mathrm{T}$, $R_0=1.3\mathrm{m}$, $a=0.38\mathrm{m}$, ${\mathit{ϵ}}_0=25.142\mathrm{keV}$, $n_{\mathrm{i}}=1.7\times {10}^{19}{\mathrm{m}}^{-3}$, $q=0.8+1.385r^2$, $\mathrm{∆}r=0.2a.$ We find from equation (12) that ${\mathrm{\Omega }}_{\mathrm{r}}$ is equal to 0.843. As expected, the result is close to that of the previous study [16], because the adiabatic contribution effects on fishbone instability in this case are small. For adiabatic contribution effects on the fishbone instability, we will provide more detail below.

4.   Parameter dependence of high-frequency fishbone

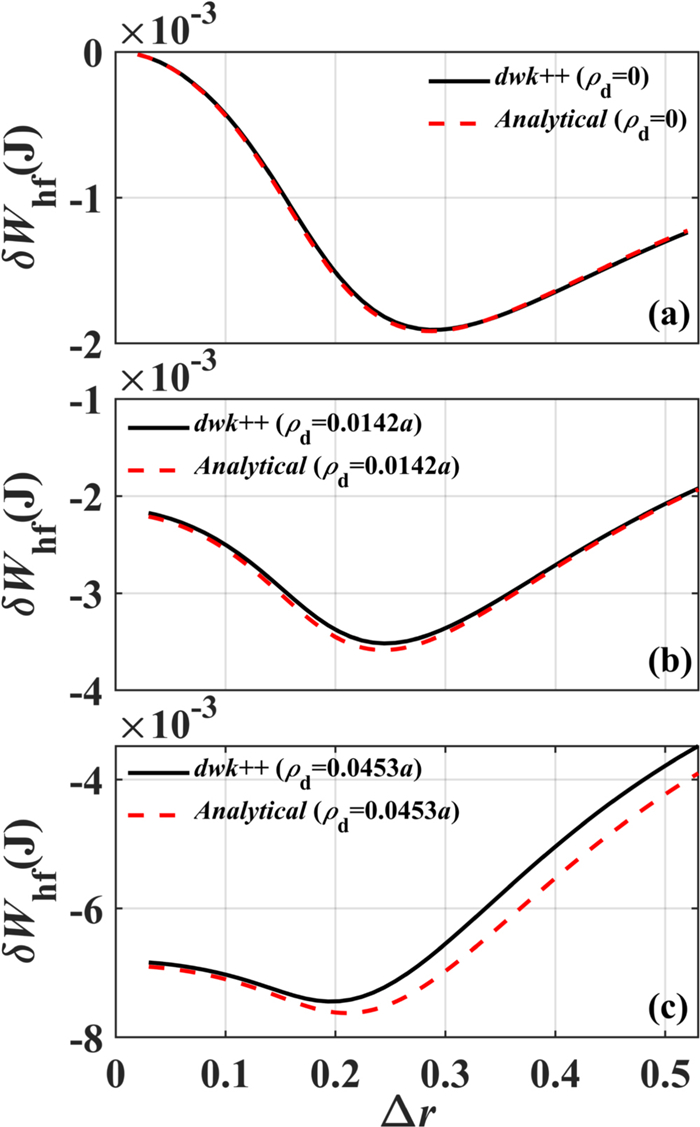
The previous version of the dwk++ code reported in [16], which has already been verified by comparison with analytical results and M3D-K results for ignoring the adiabatic contribution [16], is used to calculate $\delta W_{\mathrm{hk}}$ and solves for fishbone dispersion relation in tokamak plasmas. Considering that the mode is marginally unstable in the present work, we therefore take a small growth rate (${\mathrm{\Omega }}_{\mathrm{i}}={\omega }_{\mathrm{i}}/\left(v_0/R_0\right)=0.005$, where ${\omega }_{\mathrm{i}}$ is imaginary part of $\omega$) to treat the singularity $n{\omega }_{\varphi }+p{\omega }_{\mathrm{b}}-\omega =0$ of equation (4). Here, we have extended the model of the code to include the adiabatic contribution. In the following section, with and without the FOW effects the dwk++ results are compared with the analytical results for the simplified slowing-down distribution function to verify the modified code, and then we analyze the energetic ion parameter effects on the mode instability by using the code.

4.1   Code verification

The nonadiabatic contribution of the code has been verified. Therefore, we only need to validate the newly added adiabatic contribution. It is clear that without the FOW effects, i.e. ${\rho }_{\mathrm{d}}=0$, the code verification is easy for the analytical form equation (10). Based on operating parameters given in the previous section, we set ${\mathit{ϵ}}_{\mathrm{c}}=0.01{\mathit{ϵ}}_0$, ${\mathrm{\Lambda }}_0=0.01$, $\mathrm{∆}\mathrm{\Lambda }=0.02$ to be small in equation (2) for approaching the simplified slowing-down distribution function, and ${\xi }_0=0.01a$, while the 'inner' layer width and FOW effects are ignored. Figure 1 plots the adiabatic contribution of energetic ions $\delta W_{\mathrm{hf}}$ versus the radial profile width $\mathrm{∆}r$, in which the analytical results from equation (9) and dwk++ numerical results are shown for the different values of the finite orbit width ${\rho }_{\mathrm{d}}.$ From figure 1(a), without the FOW effects (${\rho }_{\mathrm{d}}=0$), it can clearly be observed that the analytical results are in good agreement with the numerical results. Since the expression of the finite orbit width ${\rho }_{\mathrm{d}}$ given in section 2 is complex, it is difficult to solve analytically equation (11) for the code verification with the FOW effects. Here, we choose the simplest way where we set the finite orbit width ${\rho }_{\mathrm{d}}$ as a small constant to reduce higher-order term effects on $\delta W_{\mathrm{hf}}$, which have been ignored during derivation of equation (11). With ${\rho }_{\mathrm{d}}=\mathrm{constant}$, we can rewrite equation (7) as,

Figure  1.  Adiabatic contribution of energetic ions $\delta W_{\mathrm{hf}}$ calculated by equation (9) (analytical results) and dwk++ (dwk++ results), respectively, versus radial profile width $\mathrm{∆}r$ for (a) ${\rho }_{\mathrm{d}}=0$, (b) ${\rho }_{\mathrm{d}}=0.0142a$ and (c) ${\rho }_{\mathrm{d}}=0.0453a$ by setting ${\mathit{ϵ}}_{\mathrm{c}}=0.01{\mathit{ϵ}}_0$, ${\mathrm{\Lambda }}_0=0.01$, $\mathrm{∆}\mathrm{\Lambda }=0.02.$

DownLoad: Full-Size Img PowerPoint

The corresponding $\delta W_{\mathrm{hf}}\left(O\left({\displaystyle \frac{{\rho }_{\mathrm{d}}}{\overline{r}}}\right)\right)$ is then given by,

Next, we set the 'inner' layer width $\mathrm{∆}{r}_{\mathrm{s}}=0.001a$ to be small for the amplitude of displacement to approach the Heaviside step function $H\left(x\right).$ Then, we compare the numerical results with those from equation (14) with the FOW effects (i.e. the second term of equation (9)), which are presented in figures 1(b) and (c). It can be seen that the numerical and analytical results are in good agreement for ${\rho }_{\mathrm{d}}=0.0142a.$ The small difference comes mainly from the higher-order term, which is not included in equation (14). When we set a larger ${\rho }_{\mathrm{d}}=0.0453a$, a greater effect of the higher-order term can be clearly seen from figure 1(c). Of course, if ${\rho }_{\mathrm{d}}$ continues to increase, then the analytical results will be seriously inconsistent with the numerical results.

4.2   Radial profile effects

It can be seen from equations (10) and (11) that the adiabatic contribution of energetic ions $\delta W_{\mathrm{hf}}$ is related to the radial gradient, second derivative and radial integral of the distribution inside the $q=1$ surface. Obviously, the radial profile of the equilibrium distribution can affect the fishbone instability significantly. Figure 2 plots the dependence of the fishbone mode frequency ${\mathrm{\Omega }}_{\mathrm{r}}$ and the critical beam ion beta ${\beta }_{\mathrm{c}}$ (which is the beam ion beta threshold for instability) on the radial profile width $\mathrm{∆}r$ with and without the adiabatic contribution at parameters set: ${\mathit{ϵ}}_0=2.142\mathrm{keV}$, ${\mathrm{\Lambda }}_0=0.1$, $\mathrm{∆}\mathrm{\Lambda }=0.1$, ${\mathit{ϵ}}_{\mathrm{c}}=0.36{\mathit{ϵ}}_0.$ Figure 2(b) shows that with the adiabatic contribution the critical beam ion beta ${\beta }_{\mathrm{c}}$ is larger than that without the adiabatic contribution, which indicates that the fishbone becomes more stable, while figure 2(a) shows that ${\mathrm{\Omega }}_{\mathrm{r}}$ decreases, especially for larger values of $\mathrm{∆}r.$ This is because when the adiabatic contribution is included, the total potential energy decreases. As shown in figure 3, it can be seen that with the adiabatic contribution the absolute value of the total potential energy $\delta W$ is smaller than that without the adiabatic contribution. Furthermore, the mode frequency, which is the solution of $\mathrm{real}\delta W_{\mathrm{hk}}+\mathrm{real}\delta W_{\mathrm{hf}}=0$, is sensitive to the ratio of the driving and damping contribution from equation (4) [16] and the adiabatic contribution. For off-axis NBI heating resulting in the energetic ion pressure peak not being at the axis, for example, $r_0=0.05a$ or $0.1a$, from figure 2(b) it can be clearly seen that with the adiabatic contribution the off-axis NBI heating can increase the critical beam ion beta ${\beta }_{\mathrm{c}}$, especially for larger values of $\mathrm{∆}r.$

Figure  2.  (a) Fishbone mode frequency ${\mathrm{\Omega }}_{\mathrm{r}}$, (b) critical beam ion beta ${\beta }_{\mathrm{c}}$ versus radial profile width $\mathrm{∆}r$ for different peaked positions, with and without adiabatic contribution (solid line and dashed line).

DownLoad: Full-Size Img PowerPoint

Figure  3.  Total potential energy $\delta W$ versus radial profile width $\mathrm{∆}r$ for $r_0=0.05a$, with and without adiabatic contribution (solid line and dashed line).

DownLoad: Full-Size Img PowerPoint

4.3   Critical energy effects

Critical energy is a very important parameter in the slowing-down distribution function, which affects the mode stability significantly [16, 22]. Figure 4 plots the mode frequency ${\mathrm{\Omega }}_{\mathrm{r}}$ and critical beam ion beta ${\beta }_{\mathrm{c}}$ as a function of the critical energy ${\mathit{ϵ}}_{\mathrm{c}}$ with $r_0=0.05a$, $\mathrm{∆}r=0.2a$, and the other parameters are the same as in section 4.2. It is clear from figure 4(b) that the adiabatic contribution has a significant stabilizing effect on the mode for small values of ${\mathit{ϵ}}_{\mathrm{c}}$ while it makes ${\mathrm{\Omega }}_{\mathrm{r}}$ decrease in figure 4(a). This can be understood by the fact that the change in magnitude ${\mathit{ϵ}}_{\mathrm{c}}$ has a direct effect on the equilibrium distribution function. The adiabatic contribution leads to an increase in the total potential energy, which makes the fishbone become more stable, especially for small values of ${\mathit{ϵ}}_{\mathrm{c}}.$

Figure  4.  (a) Fishbone mode frequency ${\mathrm{\Omega }}_{\mathrm{r}}$, (b) critical beam ion beta ${\beta }_{\mathrm{c}}$ versus critical energy ${\mathit{ϵ}}_{\mathrm{c}}$ with and without adiabatic contribution (solid line and dashed line).

DownLoad: Full-Size Img PowerPoint

4.4   Pitch parameter effects

We have assumed the pitch parameter to be zero (${\mathrm{\Lambda }}_0=0$) at the equilibrium distribution peak in the previous theoretical analysis, but it is not realistic to have a pitch parameter distribution in a small range in experiments. Figure 5 plots the dependence of the fishbone mode frequency ${\mathrm{\Omega }}_{\mathrm{r}}$ and the critical beam ion beta ${\beta }_{\mathrm{c}}$ on ${\mathrm{\Lambda }}_0$ at parameter set: ${\mathit{ϵ}}_{\mathrm{c}}=0.36{\mathit{ϵ}}_0.$ From figure 5(b), it can be observed that the adiabatic contribution has a weak stabilizing effect on the mode, i.e. the critical beam ion beta ${\beta }_{\mathrm{c}}$ increases slightly compared to that without the adiabatic contribution, and the mode frequency decreases slightly in figure 5(a). This is because the adiabatic contribution represents a non-resonant interaction, i.e. the adiabatic contribution has a weak dependence on the parameter—pitch parameter ${\mathrm{\Lambda }}_0$, which relates to the particle parallel velocity determining the passing particles' transit frequency.

Figure  5.  (a) Fishbone mode frequency ${\mathrm{\Omega }}_{\mathrm{r}}$, (b) critical beam ion beta ${\beta }_{\mathrm{c}}$ versus pitch parameter ${\mathrm{\Lambda }}_0$ with and without adiabatic contribution (solid line and dashed line).

DownLoad: Full-Size Img PowerPoint

4.5   Finite drift orbit width effects

The theory [10, 13] shows that the fishbone is an internal kink mode, which is destabilized by energetic particles. It is clear that the 'inner' layer width centered around the $q=1$ surface is important for the passing particles that have a finite drift orbit width. From equation (11) it can be seen that the adiabatic contribution is related to the finite orbit width ${\rho }_{\mathrm{d}}.$ Next, we focus on the adiabatic contribution effect on the fishbone instability with the FOW effect by setting the 'inner' layer width $\mathrm{∆}{r}_{\mathrm{s}}=0.02a.$ The FOW effect on the fishbone instability has already been discussed in [16], to which interested readers can refer. According to the FOW ${\rho }_{\mathrm{d}}\left(r,\mathrm{\Lambda },\mathit{ϵ}\right)=\left(q{\rho }_0/2\right)\sqrt{\mathit{ϵ}/\left(1-\mathrm{\Lambda }/b\right)}\left[\mathrm{\Lambda }/b+2\left(1-\mathrm{\Lambda }/b\right)\right]$, it can be seen that the FOW ${\rho }_{\mathrm{d}}$ increases with the ion energy. Therefore, here we choose parameter ${\mathit{ϵ}}_0$ to represent the FOW ${\rho }_{\mathrm{d}}.$ Figure 6 plots the fishbone mode frequency ${\mathrm{\Omega }}_{\mathrm{r}}$ and the critical beam ion beta ${\beta }_{\mathrm{c}}$ as a function of the injection energy of the beam ions ${\mathit{ϵ}}_0.$ As shown in figure 6, with the FOW effect the adiabatic contribution has weak stabilization of fishbone instability and makes the mode frequency ${\mathrm{\Omega }}_{\mathrm{r}}$ decrease slightly, especially for larger values of ${\mathit{ϵ}}_0.$ This is because when the ion energy is high, the nonadiabatic contribution dominates.

Figure  6.  Adiabatic contribution effects on (a) fishbone mode frequency ${\mathrm{\Omega }}_{\mathrm{r}}$ and (b) critical beam ion beta ${\beta }_{\mathrm{c}}$ with finite orbit width effects.

DownLoad: Full-Size Img PowerPoint

5.   Conclusion

We have extended the model used in the dwk++ code to include the adiabatic contribution. The code is verified by comparison with both analytical and numerical results with and without FOW effects, respectively. The dependence of the mode frequency and stability on beam ion radial profile, critical energy, pitch parameter distribution and beam ion drift orbit width is studied with considering the adiabatic contribution. Overall, the numerical analysis indicates that the adiabatic contribution plays a positive role in stabilizing the fishbone mode and makes the mode frequency decrease. More specifically, the adiabatic contribution has a significant effect on the mode instability for a larger value of the radial profile width $\mathrm{∆}r$ or a larger deviation of the deposition location of injected neutral beam from the plasma axis $r_0.$ For a small magnitude of the critical energy ${\mathit{ϵ}}_{\mathrm{c}}$, the adiabatic contribution effect on the mode instability is significant. Finally, the pitch parameter ${\mathrm{\Lambda }}_0$ and the FOW have a weak effect on the adiabatic contribution.