| Citation: | Borui ZHENG, Jianbo ZHANG, Shaojie QI, Jianghua XU, Yiche LI, Yuanzhong JIN, Dongliang BIAN. Spatiotemporal evolution laws of sector-shaped dielectric-barrier-discharge plasma actuator[J]. Plasma Science and Technology, 2024, 26(10): 105504. DOI: 10.1088/2058-6272/ad5d4f |
Dielectric barrier discharge (DBD) plasma actuators are widely used in active flow control due to their simple design and rapid responsiveness. However, they need more effectiveness and discharge extension. To overcome these limitations, a sector-shaped dielectric barrier discharge (SS-DBD) plasma actuator with an adjustable jet angle was developed to enhance flow control effectiveness. The flow field dynamics induced by the SS-DBD plasma actuator were quantitatively analyzed using particle image velocimetry (PIV). Experimental investigations showed that precise adjustments to the actuation voltage can modulate the maximum velocity of the induced jet. Furthermore, a quasi-linear relationship between the sector-shaped angles of the SS-DBD and the deflected jet angles was established, indicating that changes in the sector-shaped angles directly influence the direction of the deflected jet. This correlation enables precise control over jet angles, significantly enhancing flow control by adjusting the SS-DBD-PA’s sector-shaped angle.
Plasma aerodynamic actuation, an emerging active flow control technology, has garnered significant attention for its potential to enhance aircraft performance [1‒4]. Plasma actuation primarily includes dielectric barrier discharge (DBD), arc discharge, and spark discharge. DBD plasma actuation has become a focal point in international aerodynamics and plasma physics research due to its simple design and rapid response [5‒8]. A typical DBD plasma actuator configuration comprises an exposed electrode and a covered electrode, separated by a dielectric film. Applying high-frequency AC voltage to the exposed electrode initiates plasma formation above the covered electrode. Under the influence of the electric field, ionized particles generate controlled disturbances in the adjacent flow field [9]. However, low induced velocities and limited discharge extension significantly restrict the practical engineering applications of plasma actuators.
Despite its simple structure, the effectiveness of a typical DBD actuator is influenced by numerous factors. These factors include the electrodes’ geometrical parameters and the dielectric layer material choice [10‒14]. External actuation parameters such as waveform, amplitude, and power supply frequency exert considerable influence [15, 16]. Furthermore, the working environment, including air humidity, ambient temperature, and operating pressure during plasma discharge, plays a crucial role [17, 18]. Above all, the most significant determinant is the intrinsic structure of the plasma actuator itself. To enhance the effectiveness of DBD plasma actuators, scholars predominantly focus on optimizing the design through refinement of the actuator geometric structure.
Moreau et al initially proposed a three-electrode DBD actuator structure, featuring a double-electrode system driven by high AC voltage and a third electrode applying high positive DC voltage [19]. This configuration significantly enhances the ionic wind generated by the DBD plasma actuator, although the underlying physical mechanism remains unexplained. Mitsuhashi et al used the Pockels effect to measure potential distribution variations during surface DBD discharge and found that the dielectric surface charge distribution affects the discharge pattern, directly influences the induced jet velocity [20]. Thus, controlling the charge distribution is crucial for enhancing DBD plasma actuator performance.
In studying the non-constant flow characteristics of three-electrode sliding discharge with different actuation modes, Zheng et al found that an additional positive DC component attracts negative ions, increases the velocity and strength of the flow vortex during the negative half-cycle, and then analyzed the performance of different actuation modes [21]. Further investigations showed that optimizing the dielectric material and thickness can reduce power consumption and the flow control effectiveness of plasma actuation. Wojewodka et al investigated various dielectric materials (polyimide (Kapton), polytetrafluoroethylene (PTFE), and glass-reinforced epoxy (GRE)) with different thicknesses, actuation voltages, and frequencies [22]. They found that thinner dielectric materials performed better than thicker ones at constant voltage inputs. Additionally, Kapton, due to its higher dielectric constant, yields higher velocities among materials of the same thickness than PTFE and GRE. It is found that the carrier frequency of 12 kHz is more beneficial for plasma ionization, but the induced velocity remains unaffected by further frequency increase, thus limiting its application range.
Sato et al added an embedded electrodes in the coplanar discharge, achieving large-area discharge by disrupting the distribution of the symmetric electric field [23]. This approach generated a single ionic wind and advanced the development of plasma actuators without exposed electrodes. However, the insulation problem of coplanar discharge plasma actuators remains a significant challenge for practical industrial applications. The exploratory studies by these researchers provide valuable guidance for optimizing the performance, enhancing the aerodynamic characteristics of moving parts, and achieving effective flow control. However, the low induced velocity of conventional DBD plasma actuators remains a significant challenge in high-speed flow environments.
Various plasma actuator configurations have been proposed as innovative approaches to flow control designed to create unique flow fields [24‒27]. According to the study mentioned above, the three-electrode DBD actuator, actuated by both AC and DC power sources, can induce a maximum jet velocity of 3.2 m/s. The direction of the induced jet is significantly affected by the polarity and magnitude of the applied voltage at the third electrode [21]. On the other hand, an annular DBD actuator with an annular electrode can reach a peak jet velocity of 4.63 m/s at Vpp = 13 kV [9]. Consequently, a sector-shaped dielectric barrier discharge plasma actuator (SS-DBD-PA) with a controllable jet angle based on the annular DBD plasma actuator is proposed to enhance the actuation effectiveness of the plasma actuator. In contrast to the annular DBD actuator, when the actuation voltage is applied to the SS-DBD plasma actuator, it simultaneously generates a deflected jet at a certain angle to the wall and a horizontal jet, and the induced velocity of the deflected jet is greater than that of the conventional three-electrode actuator. This design overcomes the limitations of conventional DBD actuators, which include weak jet strength and the restriction to a single jet orientation along the wall.
This paper proposes an SS-DBD plasma actuator with an adjustable jet angle. The research investigates the effects of different actuation voltages and sector-shaped angles on the induced jet characteristics of the SS-DBD plasma actuator using electrodiagnostic techniques and particle image velocimetry (PIV). By analyzing the spatiotemporal progression of plasma actuation-induced jets and comprehending their energy transfer mechanisms, this investigation aims to enhance the efficiency of plasma actuation in active flow control.
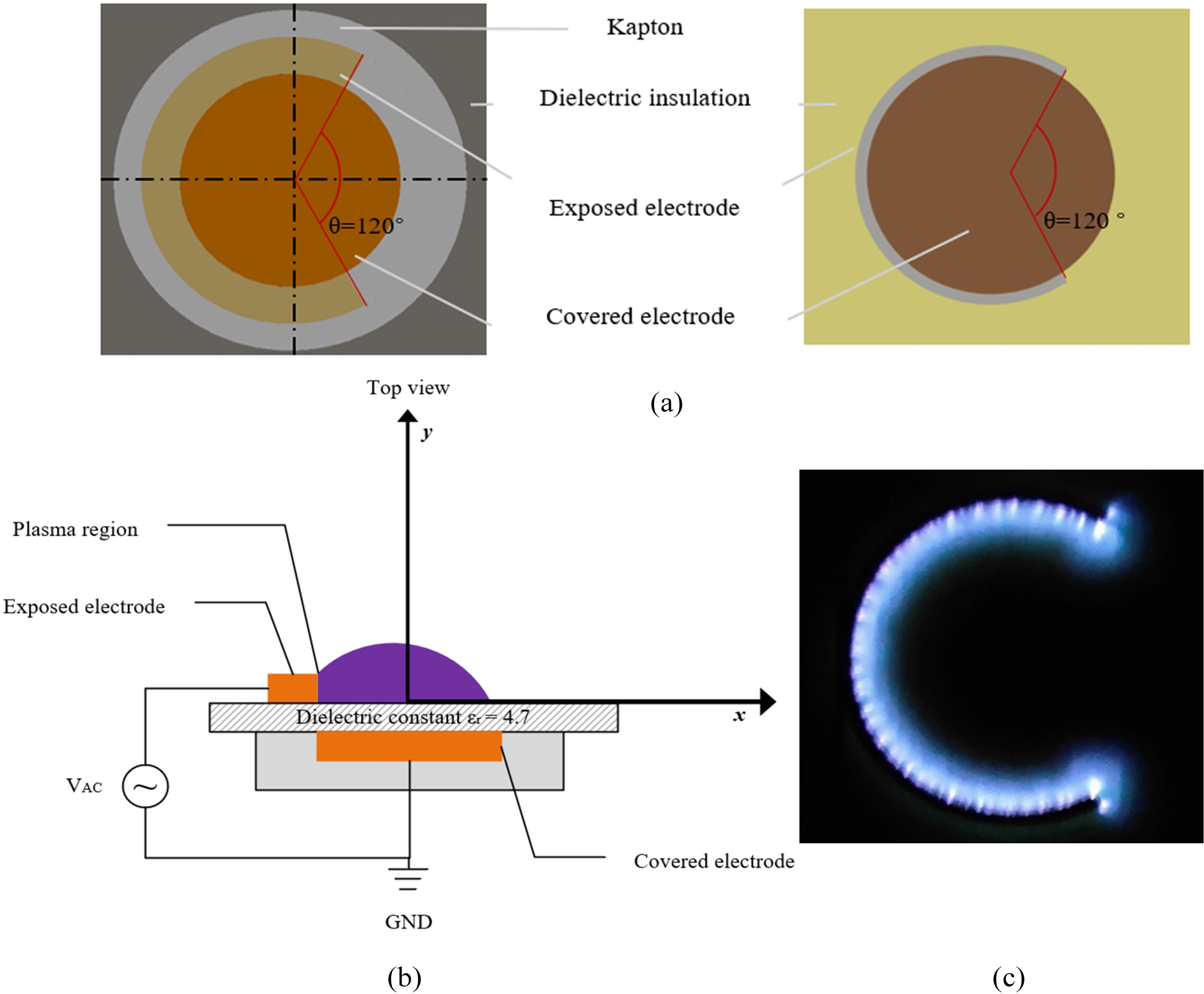
The sector-shaped DBD plasma actuator consists of a sector-shaped exposed electrode, an annular covered electrode, and an insulating dielectric layer in the middle. It is actuated by a high-frequency voltage, transferring momentum from ionized particles to neutral particles, generating body force and actively interfering with the surrounding flow field. The sector shaped angles θ are 60°, 120°, 180°, 240°, and 300°, with 120° shown in figure 1. The exposed and covered electrodes are made of 0.05 mm thick copper foil, while the dielectric layer is made of a 1 mm thick printed circuit board (PCB) board with a dielectric constant of 4.7. The entire assembly is fabricated as a single piece through an etching process. The exposed and covered electrodes are positioned on the upper and lower sides of the dielectric layer, respectively. The center of the electrode coincides with the axis, and the horizontal distance between them is 0 mm. During plasma discharge, the plasma spreads from the inner side of the high-voltage electrode to the covered electrode. The grounded electrode is covered with 0.3 mm thick Kapton to prevent arcing during plasma discharge.
For the experiment, the power supply is Nanjing Suman power supply CTP-2000K, with an output voltage range of 0‒30 kV and a carrier frequency range of 5–20 kHz. Considering that the focal point of this study revolves around the evolution of SS-DBD actuator under steady actuation, the frequencies discussed herein specifically pertain to the carrier frequency. The Tektronix P6015A high-voltage probe is used to measure the actuation voltage, while the Pearson Model 2877 current loop is adopted to calculate the actuation current. The voltage and current signals are matched with 1 MΩ and 50 Ω coaxial cable and then transmitted to the two ports of the Tektronix DPO2024 oscilloscope for measurement.
PIV is an advanced non-contact technique that overcomes the limitations of single-point flow field measurements. It can quickly and accurately measure velocity and vortex structures in a cross-section of a flow field. The flow field is quantitatively analyzed by using a the Dantec Dynamics two-dimensional PIV system, which consists of a dual-pulse laser, a light-slicing device, a light-guide arm, a CCD camera, a tracer particle generator, a synchronization controller, a computer, a three-dimensional calibration kit, and acquisition and analysis software. Detailed schematics and device connections are described in the literature [28]. Optimal alignment of the PIV system’s laser plane requires it to be perpendicular orientation to the plasma actuator’s spreading direction along the x-axis. Meticulous calibration ensures that the laser plane’s thinnest section aligns precisely with the actuator’s flow direction along the y-axis, as shown in figure 2(a) for elucidation.
The study employs an Nd:YAG laser from Beamtech Optronics Corp, featuring a maximum pulse energy of 200 mJ and a beam thickness of about 1 mm. The PIV measurements are conducted in a glass box measuring 600 mm in length, 300 mm in width, and 350 mm in height. The sector-shaped DBD actuator is actuated for 15 s before data collection to ensure a stable flow field. The tracer particles with a diameter of 2 µm and a density of 1000 kg/m3 are generated by the smoke cake, and the motion state of the tracer particles reflects the motion state of the flow field to be measured. To obtain a high-spatial resolution of the flow field, a CCD camera is chosen to cover an area of about 40 mm×30 mm, and the spatial resolution of the adjacent velocity vectors is less than 0.28 mm, the time interval between two laser pulses is 60 μs. In the experiment, a sheet light illuminates the entire flow field, and a CCD camera captures two consecutive particle images. Analysis of these images reveals the flow field’s dynamics.
To investigate the influence law of different actuation voltages on the induced flow field of SS-DBD plasma actuator, the covered electrodes of the actuator are selected with effective diameter d1 = 10 mm, exposed electrode diameter d2 =11 mm, width d0 = 1 mm, carrier frequency F = 10 kHz, and sector-shaped angle θ = 120°. In this study, the time-averaged velocities are sampled from a 40 mm×30 mm region located to the right of the inner ring of the SS-DBD actuator, as shown in figure 2(a). The evolution laws of SS-DBD plasma actuator induced flow field under actuation voltage Vpp = 9, 10, 11, 12, and 13 kV are investigated.
Time-averaged velocity clouds for the SS-DBD plasma actuator under various actuation voltages are depicted in figure 2(b). The SS-DBD plasma actuator generates a horizontal jet parallel to the wall along the x-axis and a deflected jet angled to the wall, reaching heights above 30 mm. As shown by the color changes in figure 2, the peak jet velocities generated by the SS-DBD plasma actuator all increase steadily as the actuation voltage increases. In particular, the peak horizontal jet velocity Vx-max (x = 15 mm, y = 1 mm) and the peak deflected jet velocity Vmax (x = 15 mm, y = 5 mm) increase from the initial 0.8 m/s and 1.2 m/s (figure 2, Vpp = 9 kV) to 2.3 m/s and 3.4 m/s (figure 2, Vpp = 12 kV), which are 187.5% and 183.3% higher, respectively, than the previous ones. In addition, the deflected jet flow is not affected by the viscous effect in the air, and the radius becomes larger and more dispersive with increasing height, but the average velocity gradually decreases with height.
The velocity profiles of the SS-DBD plasma actuator induced flow field at different y positions (y/d1 = 0.25, 0.5, 0.75, 1) are shown in figure 3. It can be seen from figures 3(a)‒(d) that as the actuator voltage increases, the velocity profiles at different positions are shifted upward, indicating rising energy injection by the actuator and a corresponding increase in jet velocity. At y/d1 = 0.25, it can be seen that a valley appears near the (x = 10 mm, y = 2.5 mm) position, indicating that the velocity decreases near the sector region at the bottom of the actuator, and this conclusion is confirmed by the fact that only a peak appears in the velocity profiles at y/d1 = 0.5 and 0.75 as the height of the profile increases. In the velocity profiles at y/d1 = 1, the wave valley reappears at actuation voltages of 12 kV and 13 kV, which is attributed to the fact that the energy of the deflected jet begins to diffuse to both ends as the height increases, and the velocity in the middle region gradually becomes smaller. Finally, the corresponding velocity profiles are gradually shifted to the right at the three different profile positions of y/d1 = 0.5, 0.75, and 1, where the peak velocity occurs at y = 5 mm, x = 15 mm, and the peak velocity reaches 3.52 m/s when the actuation voltage is 13 kV.
Velocity profiles for the SS-DBD plasma actuator’s induced flow field at x positions (x/d1 = 0.5, 1, 1.5, and 2) are displayed in figure 4. It is observed that when the actuation voltage is greater than 12 kV, there is a sudden increase in velocity, which is related to the impedance matching of the power supply. The two profile positions at x/d1 = 0.5 and 1 show a steeply rising and falling trend in velocity change, whereas the velocity change at x/d1 = 1.5 and 2 is slower. At actuation voltages of 12 kV and 13 kV, a valley forms near y = 2.5 mm. This occurs as the deflected and horizontal jets begin to bifurcate noticeably beyond x = 15 mm, with reduced energy in the inner region of the jets’ angle.
The relationship between the actuation voltage and the induced jet flow field has been elaborated above. To further investigate the influence of different sector-shaped angles on the aerodynamic actuation of the SS-DBD plasma actuator, the carrier frequency is fixed at 10 kHz and actuation voltage at 12 kV. The sector-shaped angles studied are 60°, 120°, 180°, and 240°. Time-averaged velocity clouds for different sector-shaped angles are shown in figure 5. It can be seen from figure 5 that the angle of the deflected jet starts to increase with the increase of the sector-shaped angle. Relational equations between the jet angle and sector-shaped angle are provided in table 1. During the change of the sector-shaped angle from 60° to 120°, the total increase of the deflected jet angle is 22.2°, and the growth trend is particularly obvious; on the other hand, the deflected jet angle increases only 11° when the sector-shaped angle is increased from 120° to 180°, and the growth rate of the deflected jet angle becomes flat during this period. Further observation of the sector-shaped angle from 180° to 240°, the deflected jet increases by 11.9°, and the deflected jet angle continues to grow flat. Comparing the evolutions of the whole jet in figures 5(a)‒(d), it can be seen that as the sector-shaped angle increases, the energy of the horizontal and deflected jets gradually spreads to the two sides. When the sector-shaped angle increases to 240°, the two jets begin to merge, and the energy of the jet is uniformly distributed in the inner region.
| Sector-shaped angle |
Deflected jet angle |
Fitting equation |
| 60° | 26.9° | y = −0.0007x2 + 0.4584x + 2.575 (R² = 0.9934) |
| 120° | 49.1° | |
| 180° | 60.1° | |
| 240° | 72° |
In this study, a sector-shaped DBD plasma actuator with an adjustable jet angle is devised to enhance its applicability in industrial settings. Employing the particle image velocimetry (PIV) system, an exhaustive quantitative examination is conducted on the flow field induced by the SS-DBD plasma actuator across different sector-shaped angles. The ensuing conclusions are derived from the observed discharge patterns of the actuator and an analytical exploration of its fundamental mechanisms.
(1) The SS-DBD plasma actuator generates a deflected jet exceeding 30 mm in height and a horizontal jet along the wall. Increasing the actuation voltage from 9 kV to 12 kV boosts the velocities of both jets by 187.5% and 183.3% respectively. The deflected jet is unaffected by air viscosity, expanding radially as height increases, albeit with a gradual decrease in average velocity.
(2) In the velocity profile at x/d1 = 0.25, velocity decay occurs within the sector region. At x/d1 = 1, the deflected jet’s energy disperses laterally, causing a progressive reduction in velocity within the central region. At x/d1 = 1.5 and 2, velocity changes are less prominent, accompanied by a distinct bifurcation between the deflected and horizontal jets, resulting in diminished energy within the inter-jet region.
(3) The deflected jet angle linearly increases with the sector angle, following the equation y = −0.0007x2 + 0.4584x + 2.575, where for the 120° sector shaped angle, the deflected jet is close to the ideal 45°, and for the 240° sector shaped angle, the deflected and horizontal jets tend to merge.
This investigation explores the spatiotemporal evolution of plasma actuation-induced jets and elucidates their energy transfer mechanisms, advancing developments in flow control. However, the ability of the actuation system to consistently maintain optimal performance in practical engineering applications remains uncertain. Consequently, forthcoming efforts will focus on further scrutinizing the effectiveness of flow control and its underlying mechanisms. This will be achieved through wind tunnel experiments incorporating flow field visualization techniques and computational fluid dynamics simulations. Moreover, the effectiveness of the actuator is significantly influenced by the choice of dielectric material. Therefore, future research endeavors will prioritize an in-depth exploration of the impact of dielectric material selection on actuation performance.
This work was supported by National Natural Science Foundation of China (Nos. 61971345 and 52107174).
| [1] |
Adamovich I et al 2017 J. Phys. D: Appl. Phys. 50 323001 doi: 10.1088/1361-6463/aa76f5
|
| [2] |
Wang J J et al 2013 Prog. Aerosp. Sci. 62 52 doi: 10.1016/j.paerosci.2013.05.003
|
| [3] |
Liang H et al 2016 J. Acta Aeronautica et Astronautica Sinica. 37 2603 (in Chinese)
|
| [4] |
Zheng B R et al 2019 AIAA J. 57 467 doi: 10.2514/1.J057596
|
| [5] |
Shao T et al 2016 High Volt. Eng 42 685 (in Chinese) doi: 10.13336/j.1003-6520.hve.20160308018
|
| [6] |
Sun J et al 2019 J. Exp. Fluid Mech. 33 81 (in Chinese)
|
| [7] |
Li Y H et al 2022 Adv. Mech. 52 1 (in Chinese)
|
| [8] |
Mohsenimehr S and von Keudell A 2023 Plasma Chem. Plasma Process 43 1633 doi: 10.1007/s11090-023-10405-z
|
| [9] |
Jin Y Z 2023 Research on synthetic jet plasma and its application in turbulent drag reduction. MSc Thesis Xi’an University of Technology, Xi’an, China (in Chinese)
|
| [10] |
Mohammadpour P, Mani M and Saeedi M 2021 AIP Adv. 11 105007 doi: 10.1063/5.0057284
|
| [11] |
Kelar J et al 2020 Vacuum 174 109180 doi: 10.1016/j.vacuum.2020.109180
|
| [12] |
Fridlender T et al Electrical characteristics and flow topology of ring-type dielectric barrier discharge plasma actuator In: AIAA SCITECH 2023 Forum National Harbor: AIAA 2023
|
| [13] |
Li J Q et al 2023 J. Appl. Phys. 133 063301 doi: 10.1063/5.0134362
|
| [14] |
Nunes-Pereira J et al 2022 Polym. Adv. Technol. 33 1278 doi: 10.1002/pat.5600
|
| [15] |
Zhang Y H et al 2021 Phy. Plasmas 28 023504 doi: 10.1063/5.0037035
|
| [16] |
Sato S, Yoshikawa T and Ohnishi N 2022 Actuators 11 333 doi: 10.3390/act11110333
|
| [17] |
Qi X H and Lei J Y 2019 J. Bohai Univ. (Natl. Sci. Ed.) 40 59 (in Chinese)
|
| [18] |
Shen L, Chen Z N and Wen C Y 2020 AIAA J. 58 3368 doi: 10.2514/1.J059264
|
| [19] |
Moreau E, Sosa R and Artana G 2008 J. Phys. D: Appl. Phys. 41 115204 doi: 10.1088/0022-3727/41/11/115204
|
| [20] |
Mitsuhashi K et al 2021 Plasma Sources Sci. Technol. 30 04LT02 doi: 10.1088/1361-6595/abefa7
|
| [21] |
Zheng B R et al 2023 Chin. Phys. B 32 095203 doi: 10.1088/1674-1056/acae76
|
| [22] |
Wojewodka M M, White C and Kontis K 2020 Sens. Actuat. A: Phys. 303 111831 doi: 10.1016/j.sna.2020.111831
|
| [23] |
Sato S, Sakurai M and Ohnishi N 2023 AIP Adv. 13 065001 doi: 10.1063/5.0150335
|
| [24] |
Mishra B K, Gupta A and Panigrahi P K 2022 Phys. Rev. Fluids 7 033702 doi: 10.1103/PhysRevFluids.7.033702
|
| [25] |
Yang L L, Cai J S and Kang L 2017 High Volt. Eng. 43 1910 (in Chinese)
|
| [26] |
Zhao X H et al 2010 J. Aerosp. Power 25 1791 (in Chinese)
|
| [27] |
Gao G Q et al 2017 Trans. China Electrotech. Soc. 32 55 (in Chinese)
|
| [28] |
Zheng B R et al 2023 Phys. Fluids 35 125129 doi: 10.1063/5.0172381
|
| [1] | Peng Gao, Taifei Zhao, Borui Zheng, Yuanzhong Jin, Bangdou Huang. Performance evaluation and design optimization of sector-shaped dielectric-barrier-discharge plasma actuator[J]. Plasma Science and Technology. |
| [2] | Guangyin ZHAO, Chang WANG, Yongdong YANG, Guoqiang LI, Zheyu SHI. Rotor performance enhancement by alternating current dielectric barrier discharge plasma actuation[J]. Plasma Science and Technology, 2023, 25(1): 015506. DOI: 10.1088/2058-6272/ac803a |
| [3] | Zheng LI (李铮), Zhiwei SHI (史志伟), Hai DU (杜海), Qijie SUN (孙琪杰), Chenyao WEI (魏晨瑶), Xi GENG (耿玺). Analysis of flow separation control using nanosecond-pulse discharge plasma actuators on a flying wing[J]. Plasma Science and Technology, 2018, 20(11): 115504. DOI: 10.1088/2058-6272/aacaf0 |
| [4] | Yadong HUANG (黄亚冬), Benmou ZHOU (周本谋). Active control of noise amplification in the flow over a square leading-edge flat plate utilizing DBD plasma actuator[J]. Plasma Science and Technology, 2018, 20(5): 54021-054021. DOI: 10.1088/2058-6272/aab5bb |
| [5] | QI Xiaohua (齐晓华), YANG Liang (杨亮), YAN Huijie (闫慧杰), JIN Ying (金英), HUA Yue (滑跃), REN Chunsheng (任春生). Experimental Study on Surface Dielectric Barrier Discharge Plasma Actuator with Different Encapsulated Electrode Widths for Airflow Control at Atmospheric Pressure[J]. Plasma Science and Technology, 2016, 18(10): 1005-1011. DOI: 10.1088/1009-0630/18/10/07 |
| [6] | R. KHOSHKHOO, A. JAHANGIRIAN. Numerical Simulation of Stall Flow Control Using a DBD Plasma Actuator in Pulse Mode[J]. Plasma Science and Technology, 2016, 18(9): 933-942. DOI: 10.1088/1009-0630/18/9/10 |
| [7] | JIN Di (金迪), LI Yinghong (李应红), JIA Min (贾敏), SONG Huimin (宋慧敏), et al.. Experimental Characterization of the Plasma Synthetic Jet Actuator[J]. Plasma Science and Technology, 2013, 15(10): 1034-1040. DOI: 10.1088/1009-0630/15/10/14 |
| [8] | ZHENG Borui (郑博睿), GAO Chao (高超), LI Yibin (李一滨), LIU Feng (刘锋), LUO Shijun (罗时钧). Flow Control over a Conical Forebody by Periodic Pulsed Plasma Actuation[J]. Plasma Science and Technology, 2013, 15(4): 350-356. DOI: 10.1088/1009-0630/15/4/08 |
| [9] | ZHENG Borui (郑博睿), GAO Chao(高超), LI Yibin(李一滨), LIU Feng(刘峰), LUO Shijun(罗时钧. Flow Control over a Conical Forebody by Duty-Cycle Actuations[J]. Plasma Science and Technology, 2012, 14(1): 58-63. DOI: 10.1088/1009-0630/14/1/13 |
| [10] | SONG Huimin, LI Yinghong, ZHANG Qiaogen, JIA Min, WU Yun. Experimental Investigation of the Characteristics of Sliding Discharge Plasma Aerodynamic Actuation[J]. Plasma Science and Technology, 2011, 13(5): 608-611. |
| Sector-shaped angle |
Deflected jet angle |
Fitting equation |
| 60° | 26.9° | y = −0.0007x2 + 0.4584x + 2.575 (R² = 0.9934) |
| 120° | 49.1° | |
| 180° | 60.1° | |
| 240° | 72° |