| Citation: | N A ASHURBEKOV, K O IMINOV, K M RABADANOV, G S SHAKHSINOV, M Z ZAKARYAEVA, M B KURBANGADZHIEVA. Simulation of the spatio-temporal evolution of the electron energy distribution function in a pulsed hollow-cathode discharge[J]. Plasma Science and Technology, 2023, 25(3): 035405. DOI: 10.1088/2058-6272/ac9aa8 |
This article presents the 2D simulation results of a nanosecond pulsed hollow cathode discharge obtained through a combination of fluid and kinetic models. The spatio-temporal evolution of the electron energy distribution function (EEDF) of the plasma column and electrical characteristics of the nanosecond pulsed hollow cathode discharge at a gas pressure of 5 Torr are studied. The results show that the discharge development starts with the formation of an ionization front at the anode surface. The ionization front splits into two parts in the cathode cavity while propagating along its lateral surfaces. The ionization front formation leads to an increase in the fast isotropic EEDF component at its front, as well as in the anisotropic EEDF component. The accelerated electrons enter the cathode cavity, which significantly contributes to the formation of the high-energy EEDF component and EEDF anisotropy.
Analysis of the spatio-temporal kinetic evolution of electrons in a nanosecond pulse plasma discharge in noble gases is of considerable current scientific and practical interest [1–4]. Since the properties of plasma as a whole are largely determined by the electrons, the electron energy distribution function (EEDF) constitutes one of the most important characteristics of nonequilibrium gas-discharge plasma [5]. Due to the high spatio-temporal gradients of the electric field and charged particles in nanosecond pulsed discharge plasma, EEDF quantification using experimental methods remains a difficult and, as yet, unsolved problem. In this regard, global models represent a simple yet powerful tool for the calculation of the EEDF in nanosecond pulsed discharge plasma [6, 7]. As is known, the most general method for calculation of the EEDF is numerically solving the electron Boltzmann equatio, i.e., a non-linear integro-differential equation; the solution constitutes an extremely difficult issue. Therefore, it is usual to make several simplifications, allowing the equation to be solved for particular cases. Of particular interest here is the type of discharge characterized by the formation of different groups of electrons in the plasma; for example, a pulsed discharge from an extended hollow cathode producing fast and slow electron groups [8, 9].
The calculation of the spatio-temporal EEDF in a pulsed discharge with a hollow cathode is an interesting and complex problem that has not yet been fully studied. Therefore, the present work aims to simulate the dynamics of the formation of a nanosecond pulsed argon discharge in an extended hollow cathode and to calculate the EEDF in various regions of the plasma column. Thus, this work is one count of attempted self-consistent modeling of pulsed discharge plasma with the hollow cathode, and we hope that the obtained results will help to understand plasma processes and create a more accurate numerical model of pulsed gas discharges with weak anisotropy of the EEDF.
The modeling object is a high-voltage pulsed nanosecond argon discharge in an extended hollow cathode. In such a discharge system, electron emission from a cold cathode is caused by its bombardment with high-energy ions and atoms, as well as their acceleration in the cathode potential drop (CPD) area. This results in the generation of accelerated electrons, which has a significant effect on the EEDF formation [9]. This design of a discharge chamber with a hollow cathode allows high current densities and radiation power to be obtained at relatively low applied voltages [9, 10]. To fully understand the physics of these processes and their effect on the main discharge properties, a more detailed study into the formation dynamics and spatio-temporal distribution of key discharge parameters is required.
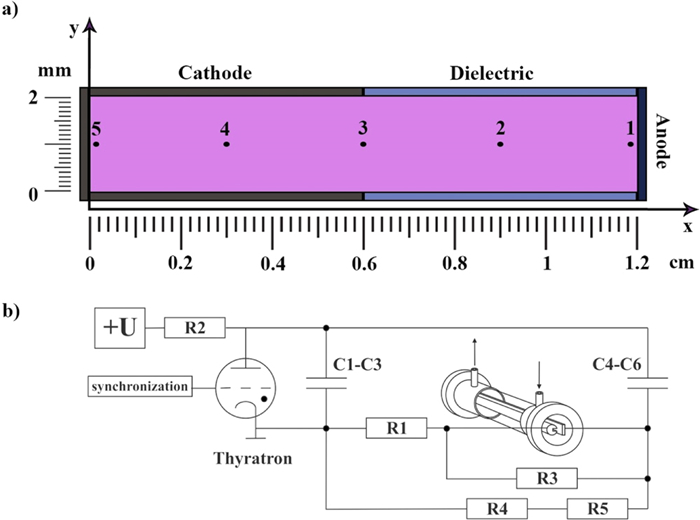
The discharge chamber is composed of a quartz tube with a diameter of 5 cm that contains a system of aluminium electrodes spaced 0.6 cm apart. The anode is a flat plate measuring 5 cm in length, 2 cm in width, and 0.5 cm in thickness. The cathode has the form of a cylindrical rod measuring 5 cm in length and 1.2 cm in diameter, along which a 0.2 cm wide and 0.6 cm deep slot is cut. For the entire electrode length, the discharge area is bounded by dielectric plates made of fibreglass laminate. The breakdown is carried out using a Blumlein-type high-voltage nanosecond pulse generator producing voltage pulses with a front edge of about 15 ns at an adjustable amplitude of up to 10 kV [10].
The modeling area is rectangular, measuring 1.2 cm in length and 0.2 cm in width (figure 1). The discharge area for the entire length of the electrodes is limited by dielectric plates made of fibreglass.
To calculate the EEDF, it is necessary to solve the Boltzmann equation. As noted above, the challenging nature of the pulsed discharge problem, both in terms of its solution and required computational resources, explains the current lack of programs or program codes. Most publicly available program codes enable the numerical solution of the Boltzmann equation for steady direct current (DC) discharge and determination of the steady-state isotropic EEDF component [11]. At present, there are software packages that allow us to calculate the EEDF in high-frequency discharges in a periodically changing electric field by applying a Fourier time series expansion at a given oscillation frequency [12].
For the considered case of a nanosecond pulsed discharge in a hollow cathode with complex boundary conditions, it is an even more complicated problem to simulate the discharge development and calculate the EEDF in the plasma column, which still remains unsolved. The present work is among the first to propose a model that uses two programs to calculate the EEDF in pulsed discharges, in which high-energy electrons are formed during the breakdown. In practice, some assumptions are typically made depending on the conditions of discharge formation and set aims to simplify the issue and permit its subsequent solution. The calculation of the EEDF in the cathode and near-cathode regions is a different, complex and still unsolved problem and requires additional studies [13–16]. Therefore, in our work, these areas of the discharge are not considered, since there is no local relation between the electric field and the EEDF of the cathode sheath plasma. Note that our experimental studies were carried out in a pressure range from 5 to 40 Torr. Therefore, to test the performance of the proposed method, the most critical (unfavourable) case for the local approximation of 5 Torr was taken, since the applicability of the local approximation is limited by higher pressures. Even under these conditions, as the below estimates show, the two-term approximation can be used to calculate the EEDF in the plasma column. Let us, therefore, establish two important conditions for the simulated discharge in argon at a gas pressure of p=5 Torr and an applied voltage of U0=1.2 kV, allowing the solution of the Boltzmann equation to be considerably simplified. The first step is to determine the mode of EEDF formation, while the second is to check the validity conditions of a two-term EEDF expansion.
The EEDF formation mode can be analysed through the introduction of a relaxation parameter [5]
| K=(νe+δνea+ν*)τd | (1) |
where
| ν*≪νea,eEλ/w≪1,λ≪L, | (2) |
where
| F(v,r,t)=∞∑l=0l∑m=-1Yml(θ,ϕ)Fml(υ,r,t) | (3) |
where
Since even in our case of a strong non-uniform electric field the anisotropy of electron velocity distribution remains low, the classical (local) two-member approximation of the EEDF is sufficient [17, 18]:
| F(w,r,t)=[f0(w)+f1(w)cosθ]ne(r,t) | (4) |
where
| 4√2π(me)3/2∫∞0f0(w)√wdw=1. | (5) |
The Boltzmann kinetic equation for the isotropic part
| ddw(W(w)f0(w)-D(w)df0(w)dw)=S*a(w). | (6) |
The anisotropic component
| f1(w)=-ENn1σm(w)df0(w)dw, | (7) |
where
| W(w)=-γw2σε(w)-3a(neNn)A1(w), | (8) |
| D(w)=γ3(ENn)2(wσm(w))+γkbTgew2σε(w)+2a(neNn)(A2(w)+w3/2A3(w)), | (9) |
| a=e2γ24πε20lnΛ, | (10) |
| Λ=12π(2eε0ˉw/3)3/2e3n1/2e, | (11) |
| A1(w)=∫u0w1/2f0(w)dw, | (12) |
| A2(w)=∫u0w3/2f0(w)dw, | (13) |
| A3(w)=∫∞uf0(w)dw, | (14) |
Here
The source term
| S*a(w)=∑k=inelasticCk(w), | (15) |
where
To conduct numerical experiments, a non-stationary two-dimensional axisymmetric model is developed taking into account the real geometry of the discharge area (figure 1), and our model consists of two modules: a COMSOL plasma fluid module [10] and a LisbOn KInetics Boltzmann (LoKI-B) kinetic module [4]. The LoKI-B program is written with object-oriented programming in MATLAB [19]. To communicate between COMSOL Multiphysics and MATLAB software, there is a special module called COMSOL LiveLink for MATLAB. To import/export this data from/to the LoKI-B program, a special m-function is created and described in the COMSOL model, which extracts the values of the reduced field and other parameters necessary for calculating the Boltzmann equation in the LoKI-B program. From the COMSOL plasma fluid module, the LoKI-B kinetic module receives the following values as input parameters at each instant of time and at each point of the problem solution domain: reduced electric field, gas pressure, gas temperature, electron and ion densities, and distributions of excited state populations of the working gas, which are used to solve equations (6) and (7). This function uses the extracted values as input parameters for the LoKI-B program, after which it starts the calculation of the EEDF. Further, after the calculation, this m-function returns the EEDF to the COMSOL model, which is used in the future to calculate the kinetic and transport coefficients. The solution of this problem is self-consistent. At startup, COMSOL automatically starts the MATLAB process, which calculates the function and returns its value to the COMSOL model.
A detailed algorithm for the numerical solution of the Boltzmann equation is provided in [20]. Using a finite difference scheme, the Boltzmann equation is discretized to find its solution. As output data, the LoKI-B kinetic module yields the isotropic
The following values are selected as the initial conditions:
The experimentally determined experiment boundary conditions are as follows:
(1)
(2)
(3) The boundary condition on the dielectric walls is as follows:
| -n·D=σs+ε0εrVref-Vds |
where n is a normal vector;
To set the form and parameters of the electric field potential, the voltage pulse U(t) applied to the discharge gap in the experiment is digitized. The characteristics of the discharge circuit are factored in through the introduction of a ballast resistor into the calculation model as per
The argon chemistry model considered in this investigation includes four species: electrons, ground state argon atoms, argon atoms in the lowest-lying excited states including metastable argon atoms, and argon positive ions.
The set of reactions for argon includes elastic collisions, the direct ionization of an atom by electron impact, excitation from the ground state, stepwise ionization from the metastable state, and Penning ionization. The set of plasma-chemical reactions is presented in table 1.
| Reaction | Data | Reaction title |
| Cross-section [21] | Momentum transfer | |
| Cross-section [21] | Metastable state excitation and de-excitation | |
| Cross-section [21] | Direct ionization from the ground state | |
| Cross-section [21] | Stepwise ionization from the metastable state | |
| Penning ionization |
The COMSOL Multiphysics plasma module is used to simulate the dynamics of discharge formation and development, yielding the spatio-temporal distribution of the main discharge plasma parameters in the gap and the cathode cavity. The LoKI-B package is simultaneously used to calculate the EEDF at various points in the discharge gap centre. As an example, figure 3 shows the results of simulating the distribution of electric potential and electric field, as well as the electron density in the gap and the cathode cavity. The distribution of potential and electric field along the discharge gap centre provides a means to trace the dynamics of discharge development and electric field penetration into the cathode cavity, as well as to estimate the maximum field values in the gap and the cathode cavity. In figure 3, the potential exhibits a gradual increase for the first 100 ns in the interelectrode gap when moving from the anode toward the cathode. After about 110 ns, it penetrates the cathode cavity, reaching the cavity bottom 150 ns after the moment the voltage is applied to the gap. Then, the potential continues to rise, reaching a maximum of 800 V after 200 ns at the cavity base (figure 3(c)). This is the point at which the cathode layer is completely formed with the redistributed potential. In the cathode layer, the drop amounts to 800 V, while the remaining potential drops are in the plasma column. Subsequently, a gradual reduction in the electric potential is observed in the gap and the cavity corresponding to the decrease in the applied voltage pulse (figure 3).
The simulation of the dynamics of the spatio-temporal electron density distribution indicates that at the initial stages of electrical gas breakdown formation, density redistribution of charged particles is observed due to the electrons being pulled to the anode. This factor leads to a decrease in electron density in the gap, whereas, in the cathode cavity and at the anode surface, it gradually rises. Approximately 80 ns after applying a voltage to the gap, a plasma bunch having an electron density of ~1010 cm-3 forms in the centre at the anode surface. An ionization front originating from this point propagates toward the cathode. As it propagates, the electron density increases rapidly at and behind the ionization front. At a propagation speed of ~3×107 cm·s-1, the ionization front reaches the middle of the gap after about 100 ns (figure 3(a)). The calculations suggest that the electric field is focused at the front while moving together with the ionization front. The field reduction at the front in the gap amounts to ~8×103 V·cm-1. By 110 ns, the electron density reaches ~1012 cm-3 and the ionization front penetrates the cathode cavity (figure 3(b)); then the ionization front splits into two parts, propagating along the lateral surfaces of the cavity and reaching its bottom. The discovered splitting effect of the ionization front allows us to understand the dynamics and mechanism of the formation of the spatio-temporal structure of ionization processes inside the hollow cathode. After that, a back ionization front is formed at the cavity bottom while filling the cavity with plasma, which leads to the formation of a plasma column in the cavity and the gap (figures 3(c) and (d)). The field is localized between the plasma column and the cathode cavity base in the cathode layer, where the maximum electric field drop reaches ~6×104 V·cm-1. The maximum electron density in the plasma column observed inside the cathode cavity reaches ~6×1014 cm-3 (figure 3(c)).
Figures 4 and 5 show the results of EEDF calculations obtained at five points in the discharge gap centre located at different distances from the anode surface: point 1 located at a distance of 0.01 cm from the anode surface; point 2 located in the interelectrode gap centre; point 3 located right at the cathode inlet; point 4 located in the middle of the cathode cavity; and point 5 located at the base of the cathode cavity at a distance of 0.01 cm from the cavity bottom (figure 1). The calculations indicate that the directed energy acquired by free electrons during the breakdown process depends on the distance from the calculation point to the anode surface. In the interelectrode gap, the energy of such electrons increases as they move from the anode to the cathode, while in the cathode cavity, the energy is initially observed to decrease, with a sharp increase in the directed electron energy at the cavity base. A similar result is obtained for all the EEDFs calculated at different points. In the high-energy EEDF region, the isotropic and anisotropic EEDF components are approximately the same, while in the low-energy region, the isotropic component exceeds the anisotropic component by almost two orders of magnitude (figures 4 and 5).
Figure 4(a) shows the isotropic and anisotropic EEDF components calculated at point 1. As can be seen from the figure, the high-energy EEDF part increases at the beginning of the breakdown process near the anode, reaching its maximum by 80 ns, followed by a gradual decline. With an increase in the voltage applied to the gap, the density of electrons near the anode rises, while some of these electrons are accelerated when being pulled to the anode in the anode potential drop area before ionization front formation. After about 90 ns from the application of voltage to the gap, an ionization front starts propagating from the anode, pushing the applied field ahead. Behind the front of the anode, a reduction in the field is observed, along with a decrease in the energy of free electrons (figure 4(a)). At the second point, the EEDF has not been completely formed by 90 ns, since the ionization front has not yet reached this point. However, the formation of EEDF 10 ns later coincides with the moment when the ionization front arrives at point 2 (figure 3(a)). A significant increase can be observed both in the isotropic and anisotropic EEDF components, while the tail of the anisotropic EEDF component is comparable to that of the isotropic component (figure 4(b)). This factor indicates the formation of accelerated electrons at the given point at this moment in time. As the ionization front propagates, virtually the entire electric field becomes concentrated at the ionization front [23].
The acceleration of free electrons while gaining energy in this region of the amplified electric field leads to a distortion of EEDF with an increase in its high-energy part. It can be seen that both the isotropic and anisotropic EEDF parts decrease over time. This means that the ionization front has passed, which leads to a decrease in the number of fast electrons at this point (figure 4(b)). At point 2, the energy of the fast EEDF part is nearly two times higher than those at point 3 (figure 5(a)) at the cathode inlet and point 4 (figure 5(b)) in the middle of the cavity. This can be attributed to the fact that the ionization front splits into two parts at the cavity inlet while propagating further along its lateral surfaces to the bottom. The electrons are primarily accelerated at the lateral surfaces of the cathode cavity, whereas in the centre of the cavity, a reduction in their number is observed (figures 5(a) and (b)). Figure 5(c) shows the EEDF calculated at point 5. It can be seen that the EEDF rapidly descends at 100 ns since the ionization front has not reached this point yet and few fast electrons are present. Over time, the EEDF becomes less steep. At 150 ns, the tail of the anisotropic EEDF component starts to exceed that of the isotropic EEDF component. Therefore, this is the moment at which high-energy electrons arrive at this point along with the ionization front. The high-energy EEDF part continues to grow to reach its maximum of 250 ns. The EEDF becomes markedly sloping, with a significantly greater tail of the anisotropic EEDF component than that of the isotropic EEDF component (figure 5(c)). This factor suggests that an additional beam of fast electrons arrives at point 1 at 250 ns, enriching the EEDF. As compared to a conventional anomalous glow discharge, hollow cathode discharge is distinguished by a sharp decrease in the CPD area. Its dimensions are such that the electrons emitted by the cathode pass the CPD area virtually without collisions to acquire an energy of
Let us estimate the size of the CPD area for the conditions of the discharge under consideration. Using the expression [24]
| dk×p≈7.5×10-2cm·Torr, |
we can obtain the CPD length
Therefore, the combined use of the two packages COMSOL Multiphysics and LoKI-B provides a tool to simulate the development of a non-uniform unsteady discharge for studying the spatio-temporal evolution of the EEDF. The calculations indicate that the discharge development starts with the formation of an ionization front at the anode surface. The ionization front, propagating from the anode to the cathode at 3×107 cm·s-1, covers the discharge gap while penetrating the cathode cavity. In the cavity, the ionization front splits into two parts, propagating along its lateral surfaces to reach the bottom. Next, a back ionization front is formed at the cavity bottom while filling the cavity with plasma, which leads to the formation of a plasma column in the cavity and the gap. The EEDF varies significantly at different points on the axis of the plasma column. In the interelectrode gap and at the cathode inlet, the EEDF is formed by plasma electrons and electrons accelerated at the ionization front. In the cathode cavity, electrons are accelerated in the CPD area, which enriches the high-energy EEDF part and makes a significant contribution to the EEDF formation. In conclusion, it should be noted that the issue of calculating the EEDF in the cathode layers of the discharge requires additional studies [13–15].
This work was supported by the Russian Foundation for Basic Research (No. 20–32–90150) and by State Assignment (No. FZNZ–2020–0002).
| [1] |
Trunec D, Bonaventura Z and Nečas D 2006 J. Phys. D: Appl. Phys. 39 2544 doi: 10.1088/0022-3727/39/12/012
|
| [2] |
Hoder T et al 2016 Plasma Sources Sci. Technol. 25 025017 doi: 10.1088/0963-0252/25/2/025017
|
| [3] |
Yuan C X et al 2017 Phys. Plasmas 24 073507 doi: 10.1063/1.4990030
|
| [4] |
Tejero-del-Caz A et al 2021 Plasma Sources Sci. Technol. 30 065008 doi: 10.1088/1361-6595/abf858
|
| [5] |
Tsendin L D 2010 Phys. -Usp. 53 133 doi: 10.3367/UFNe.0180.201002b.0139
|
| [6] |
Phelps A V 1994 J. Appl. Phys. 76 747 doi: 10.1063/1.357820
|
| [7] |
Hagelaar G J M and Pitchford L C 2005 Plasma Sources Sci. Technol. 14 722 doi: 10.1088/0963-0252/14/4/011
|
| [8] |
Ashurbekov N A et al 2010 Tech. Phys. 55 1138 doi: 10.1134/S1063784210080104
|
| [9] |
Ashurbekov N A and Iminov K O 2016 Generation of highenergy electrons in the nanosecond gas discharges with a hollow cathode ed V F Tarasenko Generation of Runaway Electron Beams and X-Rays in High Pressure Gases (New York: Nova Publishers) 421
|
| [10] |
Ashurbekov N A et al 2020 Plasma Sci. Technol. 22 125403 doi: 10.1088/2058-6272/abbb78
|
| [11] |
Morgan W L and Penetrante B M 1990 Comput. Phys. Commun. 58 127 doi: 10.1016/0010-4655(90)90141-M
|
| [12] |
Stephens J C 2018 Phys. Plasmas 25 103502 doi: 10.1063/1.5047809
|
| [13] |
Winkler R et al 1985 Beitr. Plasmaphys. 25 351 doi: 10.1002/ctpp.19850250405
|
| [14] |
Kolobov V I and Godyak V A 1995 IEEE Trans. Plasma Sci. 23 503 doi: 10.1109/27.467971
|
| [15] |
Li C et al 2012 Plasma Sources Sci. Technol. 21 055019 doi: 10.1088/0963-0252/21/5/055019
|
| [16] |
Kolobov V I and Tsendin L D 1992 Phys. Rev. A 46 7837 doi: 10.1103/PhysRevA.46.7837
|
| [17] |
Raizer Y P 1991 Gas Discharge Physics (Berlin: Springer)
|
| [18] |
Lieberman M A and Lichtenberg A J 2005 Principles of Plasma Discharges and Materials Processing 2nd edn (New York: Wiley)
|
| [19] |
MATLAB 2018 9.7.0.1190202 (R2019b). The MathWorks
Inc., Natick, Massachusetts
|
| [20] |
THE LoKI-B SIMULATION TOOL (https://github.com/ISTLisbon/LoKI)
|
| [21] |
Phelps database (https://us.lxcat.net/home/)
|
| [22] |
Bogaerts A and Gijbels R 1995 Phys. Rev. A 52 3743 doi: 10.1103/PhysRevA.52.3743
|
| [23] |
Vasilyak L M et al 1994 Phys. Usp. 37 247 doi: 10.1070/PU1994v037n03ABEH000011
|
| [24] |
Ashurbekov N A et al 2017 Plasma Sci. Technol. 19 035401 doi: 10.1088/2058-6272/19/3/035401
|
| [1] | Chunxia LIANG (梁春霞), Ning WANG (王宁), Zhengchao DUAN (段正超), Feng HE (何锋), Jiting OUYANG (欧阳吉庭). Experimental investigations of enhanced glow based on a pulsed hollow-cathode discharge[J]. Plasma Science and Technology, 2019, 21(2): 25401-025401. DOI: 10.1088/2058-6272/aaef49 |
| [2] | He GUO (郭贺), Xiaomei YAO (姚晓妹), Jie LI (李杰), Nan JIANG (姜楠), Yan WU (吴彦). Exploration of a MgO cathode for improving the intensity of pulsed discharge plasma at atmosphere[J]. Plasma Science and Technology, 2018, 20(10): 105404. DOI: 10.1088/2058-6272/aace9e |
| [3] | Shuichi SATO, Hiromu KAWANA, Tatsushi FUJIMINE, Mikio OHUCHI. Frequency dependence of electron temperature in hollow cathode-type discharge as measured by several different floating probe methods[J]. Plasma Science and Technology, 2018, 20(8): 85405-085405. DOI: 10.1088/2058-6272/aabfcd |
| [4] | Sen WANG (王森), Dezheng YANG (杨德正), Feng LIU (刘峰), Wenchun WANG (王文春), Zhi FANG (方志). Spectroscopic study of bipolar nanosecond pulse gas-liquid discharge in atmospheric argon[J]. Plasma Science and Technology, 2018, 20(7): 75404-075404. DOI: 10.1088/2058-6272/aabac8 |
| [5] | Shoujie HE (何寿杰), Peng WANG (王鹏), Jing HA (哈静), Baoming ZHANG (张宝铭), Zhao ZHANG (张钊), Qing LI (李庆). Effects of discharge parameters on the micro-hollow cathode sustained glow discharge[J]. Plasma Science and Technology, 2018, 20(5): 54006-054006. DOI: 10.1088/2058-6272/aab54b |
| [6] | Saravanan ARUMUGAM, Prince ALEX, Suraj Kumar SINHA. Feedback model of secondary electron emission in DC gas discharge plasmas[J]. Plasma Science and Technology, 2018, 20(2): 25404-025404. DOI: 10.1088/2058-6272/aa8e3f |
| [7] | N A ASHURBEKOV, K O IMINOV, O A POPOV, G S SHAKHSINOV. Current self-limitation in a transverse nanosecond discharge with a slotted cathode[J]. Plasma Science and Technology, 2017, 19(3): 35401-035401. DOI: 10.1088/2058-6272/19/3/035401 |
| [8] | HAN Qing (韩卿), WANG Jing (王敬), ZHANG Lianzhu (张连珠). PIC/MCC Simulation of Radio Frequency Hollow Cathode Discharge in Nitrogen[J]. Plasma Science and Technology, 2016, 18(1): 72-78. DOI: 10.1088/1009-0630/18/1/13 |
| [9] | ZHAO Guoming(赵国明), SUN Qian(孙倩), ZHAO Shuxia(赵书霞), GAO Shuxia(高书侠), ZHANG Lianzhu(张连珠). The Effect of Gas Flow Rate on Radio-Frequency Hollow Cathode Discharge Characteristics[J]. Plasma Science and Technology, 2014, 16(7): 669-676. DOI: 10.1088/1009-0630/16/7/07 |
| [10] | LI Shichao(李世超), HE Feng(何锋), GUO Qi(郭琦), OUYANG Jiting(欧阳吉庭). Deposition of Diamond-Like Carbon on Inner Surface by Hollow Cathode Discharge[J]. Plasma Science and Technology, 2014, 16(1): 63-67. DOI: 10.1088/1009-0630/16/1/14 |
| 1. | He, S., Zhang, H., Qi, R. et al. Simulation on the hollow cathode discharge in hydrogen. Physica Scripta, 2024, 99(10): 105610. DOI:10.1088/1402-4896/ad76e4 |
| Reaction | Data | Reaction title |
| Cross-section [21] | Momentum transfer | |
| Cross-section [21] | Metastable state excitation and de-excitation | |
| Cross-section [21] | Direct ionization from the ground state | |
| Cross-section [21] | Stepwise ionization from the metastable state | |
| Penning ionization |