Study of beam divergence and thrust vector eccentricity characteristics of the Hall thruster based on dual Faraday probe array planes and its applications

1.   Introduction

The Hall thruster [1–3] is highly appreciated with the increasing demand for spaceflight due to its outstanding advantages over traditional chemical thrusters, such as high specific impulse, high efficiency and simple structure. The advantages of Hall thrusters can substantially enhance the payload ratio of spacecraft and broaden the range of missions, making them suitable for in-orbit missions with a high level of control accuracies, such as attitude control, orbital maneuvering and north-south position keeping.

However, a number of problems [4–6] must be addressed and resolved if Hall thrusters are to be successfully integrated into spacecraft. One of these issues is the interaction of its beam with the spacecraft. Hall thruster beams [6, 7] contain numerous ions, electrons and neutral atoms. It is essential that the thruster beam divergence characteristics are accurately determined to improve the performance and lifetime of the thruster. The small magnitude of thrust deviation off-axis during Hall thruster operation is sufficient to produce large moments for a significant duration on the spacecraft, which can cause the spacecraft's altitude or orbit to deviate from its designed orbit if no correction is carried out. This is of great value to the evaluation of the Hall thruster thrust vector eccentricity characteristics for the design of spacecraft thrust vector adjustment mechanisms.

The study of beam divergence and thrust vector eccentricity for electric thrusters has been conducted by many scholars. The combination of a micro-thrust measuring device and a rotating mechanism was used by Gnizdor [8] to study the thrust vector and eccentricity of the SPT-70, SPT-100 and SPT-140 Hall thrusters under different operating conditions utilizing the torque balance principle. A far-field thrust vector probe was developed by Polk [9] and Snyder [10] at NASA to obtain the thrust vector eccentricity overtime during the 8000 h life test of the NSTAR ion thruster. The probe consists of 16 vertically and 16 horizontally placed graphite rods, each of which is 1.2 m long and 9 mm in diameter, biased at -20 V to repel electrons and capture ions. Reijen [11] adopted a vector probe consisting of an array of 37 retarding potential analyzers separated every 5 degrees to form a 1 m semicircular arc to obtain the thruster plume ion current distribution and determine the thrust vector of the high-efficiency multistage plasma thruster. However, because of the low transparency effect of multiple grids, it is necessary to dwell for a considerable time at each measurement location in the plume, which substantially increases the risk of probe failure. A linear array of 23 Faraday probes located 1 m from the center of the thruster was adopted by NASA's Benavides [12] to identify the beam density and thrust vector eccentricity of a 12.5 kW Hall thruster. The LIPS-200 [13] ion thruster beam current divergence characteristics were obtained using a Faraday probe array (FPA) combined with a moving mechanism. However, these studies were all based on the assumption that the center of the thruster beam is located at the center of the thruster exit plane, which is also assumed to follow an approximate point-source model. The center of the beam is located upstream from the thruster outlet. Moreover, the effect of the plume bipolar diffusion due to the annular discharge channel of the Hall thruster on beam divergence and thrust vector characteristics has not been thoroughly considered and investigated.

In this paper, to further improve the accuracy of the test and meet the demand for the 600 W LHT-70 Hall electric propulsion system for China's low-orbiting Internet constellation, a comprehensive in situ online test system based on the dual FPA planes Hall thruster system has been developed at the Lanzhou Institute of Physics. This overcomes the assumption that the center of the Hall thruster beam is located at the center of the thruster exit plane.

The remainder of this paper is organized as follows. Section 2 describes the experimental setup, section 3 discusses the raw and optimized test procedure, calculation method and specific examples of applications, and section 4 provides the conclusion.

2.   Experimental setup

2.1   Overall scheme

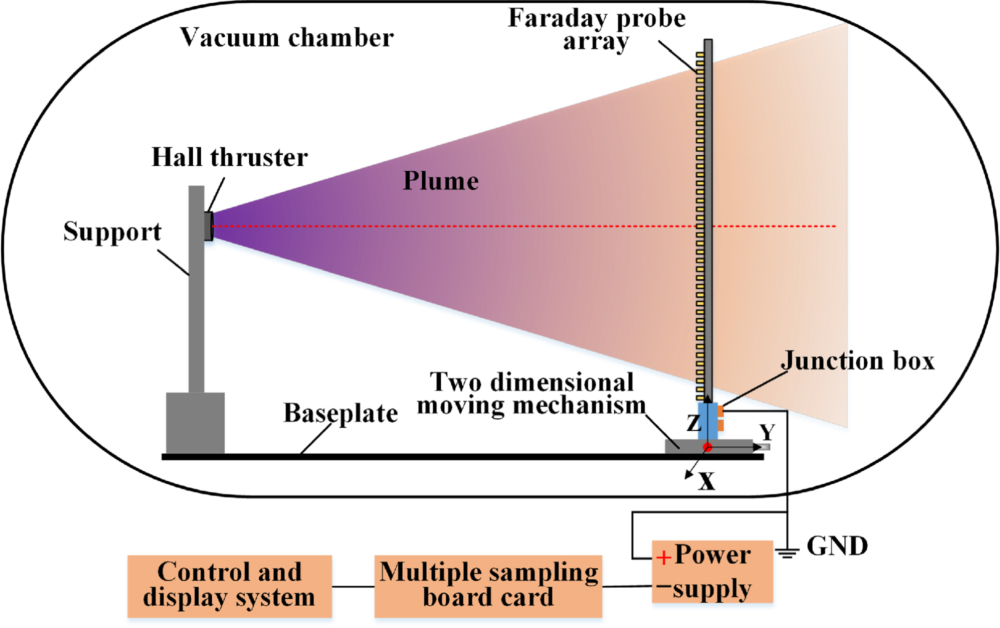
The schematic of the overall system scheme is shown in figure 1. The system consists of a Hall thruster (LHT-70), an FPA, a 2D moving mechanism, a multi-channel signal acquisition board, and a system control and display system. The Hall thruster (LHT-70) in the vacuum chamber is mounted on a bracket and they are both integrated on the base plate in the vacuum chamber. The FPA is mounted on a 2D moving mechanism (TDMM), both of which are mounted as a whole on a base plate inside the vacuum chamber, ensuring that the center of the thruster exit plane is flush with the center of the FPA. During operation of the thruster, the probe is moved from far to near in the OY direction after the determined axial position utilizing a 2D moving mechanism, and a linear sweep is made in the OXZ plane to acquire the spatial distribution of the ion current density in the OXZ plane. Then, the probe is moved to the next axial position in the OY direction and a linear sweep is made in the OXZ plane to acquire the spatial distribution of the ion current density in the OXZ plane. The ion current signal from the FPA for the whole test process passed through a hub box and signal cable, and a multi-channel signal acquisition card is stored in an automated data control, acquisition and storage system. The specific functions of the FPA system components are briefly discussed in the following sections.

Figure  1.  Schematic of the overall system.

DownLoad: Full-Size Img PowerPoint

2.2   Vacuum experiment system and LHT-70 Hall thruster

The test was conducted on the TS-6B electric propulsion vacuum experiment system at the Electric Propulsion Laboratory of the Lanzhou Institute of Physics (LIP). The dimensions of the TS-6B electric propulsion vacuum system are 3000 mm (diameter)×5000 mm (length). A 316L non-magnetic stainless steel is used for the chamber material. The operating vacuum is superior to 2.8 × 10^-3 Pa when the xenon mass flow rate during thruster operation is 2.4 mg s^-1. The schematic of the vacuum experimental system is shown in figure 2. The 600 W class LHT-70 Hall thruster prototype comprises three principal components, including the discharge chamber, cathode, magnetic circuit, etc. The thruster has a discharge diameter of 70 mm, a nominal power of 600 W and can deliver a thrust of 40 mN. The discharge chamber mainly consists of a BN-SiO₂ structure with a ring-shaped cavity. The cathode is a hollow LaB₆ hot cathode with a 3 A emission current. The relative position of the LHT-70 thrusters of the probe array is depicted in figure 3.

Figure  2.  Schematic of the vacuum experimental system (TS-6B).

DownLoad: Full-Size Img PowerPoint

Figure  3.  Relative position of the LHT-70 thrusters and the FPA.

DownLoad: Full-Size Img PowerPoint

2.3   Faraday probe array (FPA)

The FPA used for this experiment consists of 1 probe holder with a total length of 1200 mm uniformly distributed with up to 41 Faraday probes on 1000 mm. The center distance between individual Faraday probes is 25 mm. The individual Faraday probe [6, 14] consists of a collection electrode, guard ring, ceramic, and installation base. The collection electrode consists of a 10 mm diameter molybdenum disc and the guard ring consists of a 12 mm inner diameter stainless-steel cylindrical tube with a 1 mm wide gap between the outer wall of the collection electrode and the inner wall of the guard ring. The probe array and installation base mechanism are shown in figure 4. The entire FPA is assembled on a TDMM with an effective journey of 1000 mm×1000 mm and positioning accuracy of better than 0.1 mm. The whole system (including the moving platform) is powered by an AC power supply with a voltage of 220 ± 22 V and a frequency of 50 ± 2.5 Hz. The relative position of the LHT-70 thruster and the probe array is illustrated in figure 3.

Figure  4.  Schematic (left) and actual image (right) of the FPA.

DownLoad: Full-Size Img PowerPoint

3.   Results and discussion

3.1   Raw test procedure and calculation method

The test procedure for the integrated test system for electric propulsion beam dispersion diagnostics is as follows. First, the FPA is moved linearly in the XOZ plane from -500 mm to +500 mm at a fixed position in that direction (e.g. 400 mm in the axial direction (Y)) with step travel of 25 mm at a time until the ion current density distribution at a position 400 mm downstream from the thruster is available. Second, the probe array is moved to 500 mm in the axial direction position and repeated sequentially in the XOZ plane from -500 mm to +500 mm to achieve the ion current density distribution at the 500 mm position downstream from the thruster. Finally, the repeated steps above were performed until ion current density distributions were obtained at locations 600, 700, 800 and 900 mm downstream from the thruster. Consequently, a total of 10 086 measurement points (41 points × 41 probes × 6 surfaces) were recorded in a 3D spatial dimension to study the 3D ion current density spatial morphology of the LHT-70 Hall thruster. The schematic of the relative position between the FPA and the thrusters is shown in figure 5.

Figure  5.  Schematic of the relative position between the FPA and the thrusters.

DownLoad: Full-Size Img PowerPoint

The schematic of the FPA scan calculation area is shown in figure 6. As the FPA moves radially in the beam region, there is a scanned area $41t\times 41t$ (t = 25 mm), with the corresponding dotted array element area $t^2$ for each probe, as indicated in figure 5. In a square scan area of $41t\times 41t,$ the beam divergence angle is defined by first calculating the current ($I_{20t}$) in the circle with a radius of $R=20.5t.$ The beam divergence radius of $R_{90\%}$ is then calculated by numerical fitting or interpolation.

Figure  6.  Schematic of the FPA scan calculation area.

DownLoad: Full-Size Img PowerPoint

The calculation process for $R_{90\%}$ is as follows. The total integrated current I for an area of radius R is derived in figure 5 by assuming that the current density at the probe $P_{x,z}$ is $J_{x,z}.$

where $R$ is equal to $20.5t.$ and t is the length of the probe scanning dot element ($t=25\mathrm{mm}$). Equation (1) can be converted into a sum of the currents (number of dots in the grid) collected by all probes in the integration area, and then multiplied by the dotted metric area, resulting in the total beam current I. Assuming a current density of ${\eta }_{19}$ for the $P_{ij}$ probe, the range $I_n$ in $i^2+j^2\le n^2$ can be expressed as the following equation:

In equation (2), n is the number of probes in the radius of integration R, n = 0, 1, 2, …, 20 and $I_n$ represents the integrated current containing a range of n. When n is equal to 20, equation (2) containing the total current within the maximum integration area is I₂₀.

The definition of ${\eta }_n$ is the proportion of the local integral current to the total current as follows:

To quickly determine the radius corresponding to 90% of the ion current density, the calculation in equation (3) starts from the number of the 19th probe and then sequentially decreases the radius of integration. When $\left[\eta \right]$ is equal to 0.9, the corresponding calculation is terminated and $\left[\eta \right]$ is rounded. At this point, the corresponding radius is noted as $R_{90\%}.$

The diagram of the relative relationship between the beam divergence angle and the thrust vector eccentricity angle with respect to the Hall thruster is shown in figure 7. The beam divergence half angle ($\alpha$) is calculated as follows:

Figure  7.  Diagram of the relative relationship between the beam divergence angle and the thrust vector eccentricity angle with respect to the Hall thruster.

DownLoad: Full-Size Img PowerPoint

In equation (4), $R_{190\%}$ and $R_{290\%}$ respectively represent the radii of the beam corresponding to 90% of the two planes downstream from the thruster. $L_{x2},L_{x1}$ are the respective distances of the two FPA planes from the thruster exit plane.

The magnitude of the thrust vector eccentricity ($\gamma$) for different distances from the thruster plane can be obtained by determining the coordinates of the maximum ion current density in the two planes downstream from the thruster (XOZ). The formulas are presented in detail in equations (5)–(8).

where ($x_{01},z_{01}$) and ($x_{02},z_{02}$) are the coordinates of the plane swept by the previous and subsequent probe arrays corresponding to the maximum ion current density. $L$ is the distance ($L=L_{x2}-L_{x1}$) between the probe arrays and d is the distance between the maximum points where the probe measures the ion current density of the dual plane.

The FPA is constantly parallel to the thruster while scanning radially and axially. The LHT-70 Hall thruster discharge plume and 3D ion current density distribution with an anode mass flow rate of 2.6 mg s^-1 and discharge voltage of 310 V are shown in figures 8 and 9, respectively. As can be seen in figure 9, the ion current density of the plume exhibits a bipolar diffusion characteristic with well-defined symmetry and an extended length of the beam approaching 900 mm. Taking the 400 mm position as an example, the ion current density at the center of the thruster is approximately 5.6 mA cm^-2 and gradually decreases along the radial position to approximately 0 mA cm^-2 at the 500 mm radial position. With increasing axial distance, the current density generally decreases at the axial position (approximately 0.65 mA cm^-2 at 900 mm), while the beam distribution becomes broader and broader. The FPA characterizes the nearly complete ion current distribution of the LHT-70 Hall thruster.

Figure  8.  LHT-70 Hall thruster discharge plume with an anode mass flow rate of 2.6 mg s^-1 and a discharge voltage of 310 V.

DownLoad: Full-Size Img PowerPoint

Figure  9.  Measured 3D ion current density distribution with an anode mass flow rate of 2.6 mg s^-1 and a discharge voltage of 310 V.

DownLoad: Full-Size Img PowerPoint

In order to better visualize the distribution of ion current density for different cross-sections downstream from the LHT-70 Hall thruster, the 2D ion current density distribution at different positions apart from the thruster outlet with an anode mass flow rate of 2.6 mg s^-1 and discharge voltage of 310 V is shown in figure 10. As can be seen in figure 10, the ion current density at the identical cross-section downstream from the thruster shows a center-symmetrical distribution with a decreasing overall radial gradient. The magnitude of the beam divergence angle and the thrust vector eccentricity angle can be derived from equations (4) and (9). The beam divergence angle and thrust vector eccentricity angle are determined by employing equations (4) and (9), respectively, with the corresponding beam divergence angle and thrust vector eccentricity angle marked as ${\alpha }_{45},$${\alpha }_{56},$${\alpha }_{67},$${\alpha }_{78},$${\alpha }_{89}$ and ${\gamma }_{45},$${\gamma }_{56},$${\gamma }_{67},$${\gamma }_{78},$${\gamma }_{89}.$

Figure  10.  Measured 2D ion current density distribution at different positions apart from the thruster outlet with an anode mass flow rate of 2.6 mg s^-1 and a discharge voltage of 310 V.

DownLoad: Full-Size Img PowerPoint

The results of the calculation of the beam divergence angle and thrust vector eccentricity angle are shown in figure 11. The horizontal coordinates are abbreviated for the two calculation planes from the thruster outlet. For example, '45' stands for the abbreviation of two Faraday probe planes located 400 and 500 mm from the thruster outlet. The meaning of the other horizontal coordinates is as follows. As can be seen in figure 11, the beam divergence angles calculated at different cross-sections are in a range between 57.9° and 58.6°, with an average value of 58.26°. The thrust vector eccentricity angles calculated at different cross-sections lie between 2.12° and 2.89° with a mean value of 2.47°. The results of the beam divergence angle calculations varied little. However, as the position is closer to the thruster exit plane, the calculated magnitude of the thrust vector eccentricity angle increases. The ratios of the deviation between the maximum and minimum values of the beam divergence angle and the thrust vector eccentricity angle to the mean value are 1.2% and 31.1%, respectively. The calculated deviation of the thrust vector eccentricity angle is significantly higher than that of the beam divergence angle. The primary reason for this result is that the Hall thruster discharge channel is circular, resulting in a maximum ion current density that is not located at the center of the thruster. The 2D ion current density distribution at the X = 0 apart from the thruster outlet and the ion current density distribution at different cross-sections apart from the thruster outlet under an anode mass flow rate of 2.6 mg s^-1 and a discharge voltage of 310 V are shown in figures 12 and 13, respectively. The ion current density at the downstream thruster axis (at X = 0, Z = 0) in figure 12 is less than the ion current density in the adjacent radial direction, which has also been confirmed by other researchers in the study of Hall thrusters. Correspondingly, as illustrated in figure 13, the spatial distribution of ion current density for the center of the thruster exhibits a certain extent of 'concavity' [15], which in turn is reflected in the 'double-peaked' [6, 16, 17] structure of the corresponding cross-section.

Figure  11.  Results of the calculation of the beam divergence angle and thrust vector eccentricity angle.

DownLoad: Full-Size Img PowerPoint

Figure  12.  Measured 2D ion current density distribution at X = 0 apart from the thruster outlet under an anode mass flow rate of 2.6 mg s^-1 and a discharge voltage of 310 V.

DownLoad: Full-Size Img PowerPoint

Figure  13.  Measured ion current density distribution at different cross-sections apart from the thruster outlet under an anode mass flow rate of 2.6 mg s^-1 and a discharge voltage of 310 V.

DownLoad: Full-Size Img PowerPoint

3.2   Optimized test procedure and calculation method

The Hall thruster plume distribution is axisymmetric under ideal conditions. The combination of factors, such as machining, assembly accuracy and thermal deformation, during operation can cause an eccentricity for the thrust vector. The ratio of the deviation between the maximum and minimum values of the thrust vector eccentricity angle to the mean value is 31.1%, which is a relatively large test margin of error. To further reduce the testing error of the thrust vector eccentricity angle, research on experimental optimized methods was carried out.

The experimental and simulated studies of ion currents in the far-field [7, 18] plume region beyond four times the diameter of the Hall thruster indicated a typical smooth bell-shaped diffusion of the plasma profile along the radial direction. With regard to the far-field plume of the Hall thruster, the Gaussian function is now internationally acknowledged for determining the far-field ion current density. Therefore, on the basis of the spatial distribution of the ion current density in the far field of the Hall thruster, the thrust vector eccentricity of the thruster under certain operating conditions can be accessed by further fitting the coordinates of the symmetry axis obtained by solving the Gaussian surface model of the far-field plume through the adjacent scan planes. Assuming that the thrust vector eccentricities in the X and Z directions are calculated independently of each other, with an eccentricity mean of x₀ and z₀ and derivatives of ${\sigma }_x$ and ${\sigma }_z$, respectively, then the Gaussian function ($G(x,z)$) of the demand solution surface model is given by,

where, A is the coefficient and $(x_{01}^{\text{'}},z_{01}^{\text{'}})$ is the coordinate of the Gaussian-fitted ion current density for the scan plane. Under ideal operating conditions, the Hall thruster plume should be axisymmetric so that we have ${\sigma }_x$ = ${\sigma }_z.$ Both sides of the equation (9) are taken logarithmically simultaneously.

Equation (10) is a general two-quadratic polynomial fit expression. It can be simplified to the following equation:

where, $a=-\frac{1}{2{\sigma }_x^2},$$b=-\frac{1}{2{\sigma }_z^2},$$c=\frac{x_0}{{\sigma }_x^2},$$d=\frac{z_0}{{\sigma }_z^2},$$e=\ln (A)-\frac{{x_0}^2}{2{\sigma }_x^2}-\frac{{z_0}^2}{{\sigma }_z^2}.$

The solution is achieved using the least squares method, the residual sum of squares minimal principle.

where Q is the function to be solved.

Based on the minimal value condition, the partial derivatives of a, b, c, d and e are determined and set to zero $\left({\displaystyle \frac{\partial Q}{\partial a}}={\displaystyle \frac{\partial Q}{\partial b}}={\displaystyle \frac{\partial Q}{\partial c}}={\displaystyle \frac{\partial Q}{\partial d}}={\displaystyle \frac{\partial Q}{\partial e}}=0\right)$, respectively. The following equation is obtained after simplification:

The a, b, c, d and e can be obtained by solving the submatrix equations. Based on the weighted least squares fitting principle, the coordinates of the maximum value of the thrust vector can be achieved by minimizing the sum of the squares of the residuals between the fitted point and the data point.

The corrected thrust vector eccentricity angle and beam divergence half angle are thus calculated as follows:

In equation (12), $R{\text{'}}_{190\%}$ and $R{\text{'}}_{290\%}$ respectively represent the optimized radius of the beam corresponding to 90% of the two planes downstream from the thruster.

The optimized 3D ion current density distribution with an anode mass flow rate of 2.6 mg s^-1 and a discharge voltage of 310 V with the Gaussian distribution function is shown in figure 14. As can be seen in figure 14, the ion current density of the plume exhibits a bipolar diffusion characteristic along the radial direction with well-defined symmetry. Thus, the ion current density of the plume tends to be maximum at the position of the thruster axis and gradually decreases along the radial direction away from the axis. In order to obtain a clearer picture of the results of the ion current density fit, the optimized 2D ion current density distribution at the X = 0 under an anode mass flow rate of 2.6 mg s^-1 and a discharge voltage of 310 V with the Gaussian distribution function fitting is illustrated in figure 15.

Figure  14.  Optimized 3D ion current density distribution under an anode mass flow rate of 2.6 mg s^-1 and a discharge voltage of 310 V with the Gaussian distribution function fitting.

DownLoad: Full-Size Img PowerPoint

Figure  15.  Optimized 2D ion current density distribution at X = 0 under an anode mass flow rate of 2.6 mg s^-1 and a discharge voltage of 310 V with the Gaussian distribution function fitting.

DownLoad: Full-Size Img PowerPoint

The optimized 2D ion current density distribution at X = 0 under an anode mass flow rate of 2.6 mg s^-1 and a discharge voltage of 310 V with the Gaussian distribution function fitting is shown in figure 16. The fitted data for the ion current density at the center of the thruster is significantly improved in figure 16 in comparison to that of figure 13. The remarkable characteristics of the optimized results are that the ion current density peaks at different cross-sections are more pronounced and exhibit a single-peak structure, which facilitates the calculation of the thrust vector eccentricity angle.

Figure  16.  Optimized ion current density distribution with the Gaussian distribution function fitting at different cross-sections apart from the thruster outlet under an anode mass flow rate of 2.6 mg s^-1 and a discharge voltage of 310 V.

DownLoad: Full-Size Img PowerPoint

The beam divergence angle and thrust vector eccentricity angle are determined by employing equations (11) and (12) with the Gaussian distribution function fitting, respectively. The optimized results of the calculation of the beam divergence angle and thrust vector eccentricity angle are shown in figure 17. As can be seen in figure 17, the beam divergence angles calculated at different cross-sections are in a range between 57.0° and 57.8°, with an average value of 57.48°. The thrust vector eccentricity angles calculated at different cross-sections lie between 0.98° and 1.08° with a mean value of 1.03°. The results of the beam divergence angle calculation varied little. The ratios of the deviation between the maximum and minimum values of the beam divergence angle and the thrust vector eccentricity angle to the mean value are 1.4% and 11.5%, respectively. The calculation of the thrust vector eccentricity angle has been substantially improved, by approximately 20%. However, the results of the beam divergence angle tests hardly changed. The average values of the beam divergence angle and the thrust vector eccentricity angle at different cross-sections calculated by the dual-plane, Gaussian-fitted ion current density method are adopted as the final calculation results of the Hall thruster, which can effectively ensure the accuracy of the calculation of the thrust vector eccentricity angle and beam divergence angle under different operating conditions of the Hall thruster.

Figure  17.  Optimized results of the calculation of the beam divergence angle and thrust vector eccentricity angle.

DownLoad: Full-Size Img PowerPoint

3.3   Specific examples of applications

In order to obtain the evolution characteristics of the beam divergence angle and thrust vector eccentricity angle of the 600 W class LHT-70 Hall thruster with different anode masses, the calculation method based on a dual-plane Gaussian-fitted ion current density was used to also verify the stability of the method for calculating the beam divergence angle and thrust vector eccentricity angle of the Hall thruster.

The 3D ion current density distribution with different anode mass flow rates under a discharge voltage of 310 V is shown in figure 18. As can be seen in figure 18, the ion current density for different anode mass flows and different locations shows a symmetrical distribution along the thruster axis, indirectly reflecting the accuracy of the test results. Taking the anode mass flow rate of 2.0 mg s^-1 as an example, the maximum ion current density (up to 3.29 mA cm^-2) was observed at the center of the thruster at an axial position of 400 mm and gradually decreased away from the radial position of the thruster. As the axial distance increases (from 400 to 900 mm), the ion current density at the corresponding position gradually decreases, with the ion current density at the thruster axis reaching 0.58 mA cm^-2 at X = 900 mm. As can be seen in figure 18, the maximum ion current density showed an increasing trend as the anode mass flow rate increased from 2.0 to 2.6 mg s^-1, with the maximum ion current density in the center of the thruster increasing from 3.2 to 5.61 mA cm^-2, with a 75.3% increment in the maximum ion current density. This suggests that the ionization increases with increasing mass flow rate. The shift in the thruster mode of operation towards a focused mode, resulted in increased plasma orientation and beam focusing, which is consistent with the findings of Conversano [16] and others for the Hall thruster.

Figure  18.  3D ion current density distribution with different anode mass flow rates under a discharge voltage of 310 V.

DownLoad: Full-Size Img PowerPoint

The beam divergence and thrust vector eccentricity angles for different anode mass flow rates were obtained by averaging the beam divergence and thrust vector eccentricity angles calculated by the dual-plane Gaussian-fitted ion current density method for different cross-sections, as shown in figure 19. As can be seen in figure 19, the mean values of the beam divergence angle and thrust vector eccentricity angle for the anode mass flow rate that increase from 2.0 to 2.6 mg s^-1 are 57.35° and 1.03°, respectively, with mean square deviations of 0.33 and 0.11, respectively. This also indirectly demonstrates the accuracy of the calculation of the thrust vector eccentricity and beam divergence angle for the Hall thruster based on the dual-plane Gaussian-fitted method.

Figure  19.  Results of the beam divergence angle and thrust vector eccentricity angle under different anode mass flow rates.

DownLoad: Full-Size Img PowerPoint

4.   Conclusion

The accurate and reliable assessment of Hall thruster thrust vector eccentricity and beam divergence characteristics is not only essential for understanding and knowledge of Hall thruster physics mechanisms but is also extremely valuable for thruster integration into spacecraft thrust vector adjustment mechanisms. In this study, a method for thrust vector deviation angle and beam divergence of Hall thrusters based on dual FPA planes was proposed in respect of the Hall thruster beam characteristics. The results show that the beam divergence angle calculated using a Gaussian fitting to the optimized Faraday probe dual plane is approximately identical to the non-optimized one. The ratios of the deviation between the maximum and minimum values of the beam divergence angle and thrust vector eccentricity angle to the mean value are 1.4% and 11.5%, respectively. The calculation of the thrust vector eccentricity angle has been substantially improved, by approximately 20%. The beam divergence and thrust vector eccentricity angles for different anode mass flow rates were obtained by averaging the beam divergence and thrust vector eccentricity angles calculated by the dual-plane, Gaussian-fitted ion current density method for different cross-sections. The mean values of the beam divergence angle and thrust vector eccentricity angle for the anode mass flow rate that increase from 2.0 to 2.6 mg s^-1 are 57.35° and 1.03°, respectively, with mean square deviations of 0.33 and 0.11, respectively. This also indirectly demonstrates the accuracy of the calculation of the thrust vector eccentricity and beam divergence angle for the Hall thruster based on the dual-plane Gaussian-fitted method.