Experimental confirmation of the linear relation between plasma current and external vertical magnetic field in EXL-50 spherical torus energetic electron plasmas

1.   Introduction

Low-pressure radio-frequency (RF) capacitively coupled plasmas (CCPs) exhibit a nonlinear response to the driving RF voltage, causing high-order harmonics from the applied frequency [1, 2]. The harmonics may cause additional effects that significantly influence the discharge properties. For instance, Berry et al have demonstrated that the change in the harmonic contents significantly affects the ion energy distribution at the electrode [3]. Mussenbrock and Brinkmann proposed that the high-order harmonics may be more effectively excited under the plasma series resonance (PSR) condition, enhancing electron heating [4]. The electron heating effect was then extensively investigated either theoretically [5–12] or experimentally [13–19] by other researchers. Yamazawa found the electron density significantly increased when the high-order harmonics were excited by the PSR [15]. The contribution of the high-order harmonics to the electron density was also observed during a dual-frequency discharge when a harmonic of the lower frequency coincided with the higher frequency [14]. On the other hand, the enhanced high-order harmonics may worsen the discharge uniformity due to the electromagnetic standing wave effect [18, 20].

Sharma et al used Particle-in-Cell/Monte Carlo Collision (PIC/MCC) technology to simulate a voltage-driven symmetric CCP system [21]. Under very high frequency (VHF) conditions, they observed that high-order harmonics had significant effects on the electron density, electron temperature, and the transformation of the electron energy distribution function (EEDF) [21]. Despite the significant effects, most experimental studies of low-pressure RF CCPs seem not to be concerned about the self-excited harmonics, neither the harmonics being measured and analyzed nor the matching network being tuned with consideration for the harmonics. The influences of the harmonics on discharge properties are not well known. In this paper, we present an abnormal transition of the electron energy distribution with the second harmonic excitation in low-pressure RF CCPs. When the second harmonic is significantly excited by tuning the external circuit, the electron energy probability function (EEPF) changes drastically, indicating significant changes in the electron heating and the resultant electron density and electron temperature. We discuss the reason for the phenomena’s importance to practical applications by suggesting that the self-excited high-order harmonics are significant and must be considered in the operation of low-pressure RF CCPs.

2.   Experiments

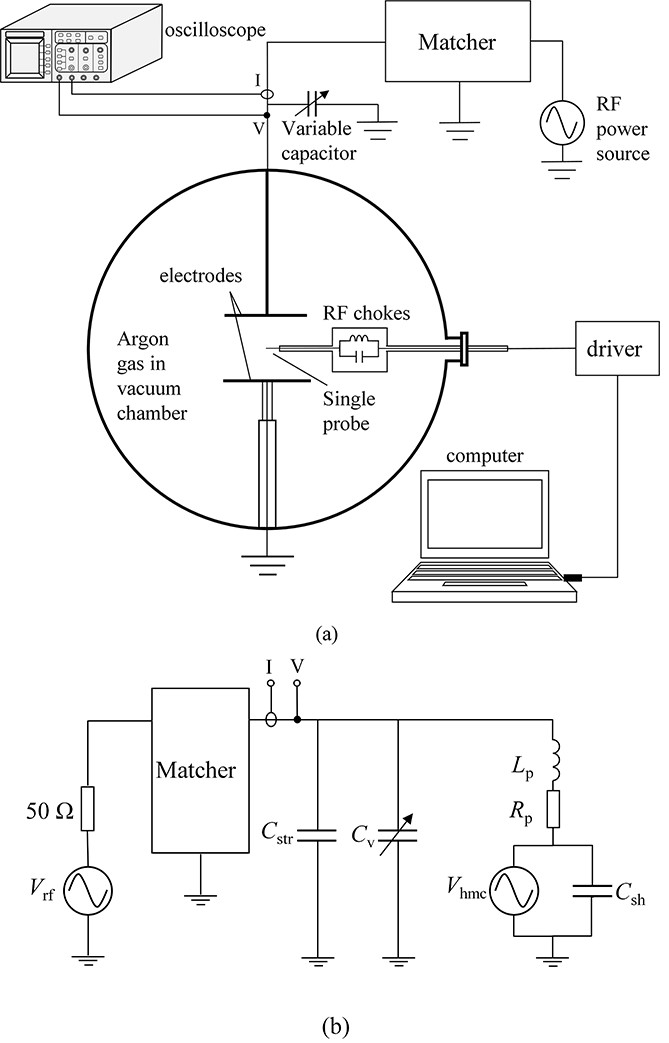
The experiments were conducted with a multi-purpose setup [22–27], which is shown pictorially diagram in figure 1(a). The vacuum chamber was a stainless-steel cylinder measuring 60 cm in diameter and 80 cm in length. The electrodes were made of circular stainless-steel plates of about 12 cm in diameter. The electrodes were parallel to each other and located at the center of the chamber, a 6 cm distance between the electrodes. The upper electrode was driven through a matcher by a wideband power source capable of outputting RF power at frequencies between 25 and 60 MHz, while the lower electrode was grounded. A variable capacitor of 7–100 pF was connected between the upper electrode and ground to tune the harmonics. Similar techniques have been employed by Yamazawa [15] and Lim et al [20]. A homemade Langmuir single probe [28] was extended horizontally into the discharge region for plasma diagnostics. The probe was made of a 0.2 mm thick tungsten wire, insulated by a 1 mm thick alumina tube, except for a 5 mm long tip of the wire exposed to the plasma. An RF choke was inserted in the probe circuit just outside the discharge region to eliminate the RF perturbations [29]. In addition, the waveforms of the discharge voltage (V) and current (I) were measured by an oscilloscope (Tektronics 2022B) through a high-voltage probe (Tektronics P5100A) and a current sensor (Pearson 2877), respectively as shown in figure 1(a).

Figure  1.  (a) Pictorial diagram of the experimental setup, and (b) equivalent schematic circuit for the RF discharge.

DownLoad: Full-Size Img PowerPoint

The equivalent circuit for the whole electric network can be sketched as shown in figure 1(b), in which C_sh, L_p, and R_p represent the sheath capacitance, plasma inductance, and plasma resistance; C_str and C_v are the stray capacitance and the variable capacitor; and V_rf and V_hmc are the RF power source and the source of the self-excited harmonics, respectively. The harmonic source is drawn in the plasma branch because it is generated there due to the nonlinear response of the sheath capacitance to the RF driving voltage. In addition, the 50 Ω resistor connected in series with V_rf represents the impedance of the RF power source and the transmission cable.

In the experiments, pure argon gas was discharged at a lower pressure of 3 Pa and a higher pressure of 16 Pa for comparison. The RF power source was fixed at an output of 36 MHz and 20 W. By tuning the variable capacitor, the harmonics were excited to different amplitudes to investigate their influence on the discharge. In all cases, the discharge voltage and current waveforms, as well as the Langmuir probe characteristics, were recorded simultaneously. The waveforms were analyzed by fast Fourier transform (FFT) to extract the harmonic components. The amplitudes and phases of the voltage and current of each harmonic were obtained. The waveforms were also used to calculate the active power by integrating the product of voltage and current. On the other hand, the EEPF was calculated from the second derivative of the Langmuir probe characteristics by the Druyvesteyn formula [30], which is

where $f\left(E\right)$ is the EEPF, $e$ and $m$ are electron charge and mass, and $V$, $I$, and $A$ are the probe voltage, current, and surface area, respectively. The electron energy $E$ equals $e({V}_{\mathrm{s}}-V)$, where ${V}_{\mathrm{s}}$ is the plasma space potential. The electron density ${n}_{\mathrm{e}}$ and electron temperature ${T}_{\mathrm{e}}$ were obtained by integrating $f\left(E\right)$ that

3.   Results and discussion

3.1   Effect of harmonic excitation at the lower pressure

A typical waveform of the discharge voltage and current between the two electrodes in the presence of the argon gas and their FFT spectra are shown in figures 2(a) and (b), respectively. The voltage waveform contains apparent harmonics up to the fourth order. We focus on the first and second harmonics because the higher ones are negligibly weak. Figure 3 shows the amplitudes of the first and second harmonics of the voltage and current changing as a function of the capacitance change from capacitor C_v in the 36 MHz discharge at 3 Pa. The subtle change of C_v between 20 and 30 pF excited significant second harmonics in both voltage and current, with a maximum being attained at ${C}_{\mathrm{v}}\approx 26\;\text{pF.}$ The phenomenon we are describing is different from what is caused by the plasma series resonance (PSR) effect [15], in which the harmonics are excited significantly only in current and not in voltage due to the low impedance of PSR. We suggest that the enhancement of harmonics in our experiment was due to improved matching between the external circuit and the plasma at the harmonic frequency.

Figure  2.  (a) The representative voltage and current waveform and (b) their FFT spectra, containing self-excited high-order harmonics.

DownLoad: Full-Size Img PowerPoint

Figure  3.  The amplitudes of the first and second harmonics of (a) voltage and (b) current versus C_v (for 36 MHz discharge at 3 Pa).

DownLoad: Full-Size Img PowerPoint

Figure 4 shows the active power and the self-bias voltage changing with C_v. The decrease in the trend of the active power and the self-bias (less negative) was possibly due to some mismatching induced by the adjustment of C_v. We did not tune the matcher during the process to avoid artificial influence. With an enhanced second harmonic, the active power slightly oscillated while the self-bias drastically decreased to be less negative. The decrease in the self-bias was possibly due to the electrical asymmetric effect (EAE), in which the self-bias varies with the relative phase difference between the first and second harmonics [31–33]. Figure 5 shows the relative phase difference between the first and second harmonics of the voltage and current changing with C_v. An apparent phase shift occurs between the C_v values of 24 and 28 pF with the excitation of the second harmonic (see figure 3), which provides evidence for the occurrence of EAE.

Figure  4.  The active power and the self-bias voltage are plotted with the change in C_v (for 36 MHz discharge at 3 Pa).

DownLoad: Full-Size Img PowerPoint

Figure  5.  The phase is given in radians (rad) of the second harmonic of the voltage and current relative to the first, with the change in C_v (for 36 MHz discharge at 3 Pa).

DownLoad: Full-Size Img PowerPoint

The EEPFs for different C_v quantities are shown in figure 6. With increasing C_v up to 25.6 pF, the low-energy electron population increases continuously, suggesting increasing stochastic heating [34]. At $C_{\mathrm{v}}=26.4\ \text{pF}$, the low-energy electron population drops abruptly, indicating a drastic decrease in stochastic heating. While at $C_{\mathrm{v}}=27.1\ \text{pF}$, the high-energy electron population in EEPF also reduces, indicating a reduction in ohmic heating [34]. At $C_{\mathrm{v}}=27.9\ \text{pF}$, the ohmic heating recovers, and with further increasing C_v, the stochastic heating also gradually recovers. You et al found that stochastic heating was inefficient at high frequencies [35]. The decrease of stochastic heating in our experiment may be due to the increase of the high-frequency component (the second harmonic of the base frequency).

Figure  6.  Change in EEPF with increasing C_v (for 36 MHz discharge at 3 Pa).

DownLoad: Full-Size Img PowerPoint

The changes in electron heating caused drastic variations in the electron density and electron temperature, as shown in figure 7. These variations were related to the excitation of the second harmonic. However, it can be noted that the abrupt changes in the electron density and electron temperature do not occur at the second harmonic’s maxima (see figure 3), which suggests that the harmonic amplitude was not the unique factor affecting the discharge. In addition, neither the active power nor the self-bias behaviors can be explained because their behaviors (shown in figure 4) are not consistent with those of the electron density and electron temperature. We proposed that the relative phase difference between the first and second harmonics, in addition to the harmonic amplitude, played an important role in the discharge. Comparing figures 7 with 5, the electron density decreases where the relative phase difference decreases toward zero. The influence of the relative phase difference on the electron density has also been observed by Bienholz et al [36], which was qualitatively consistent with our observation.

Figure  7.  Changes of the electron density and the electron temperature with increasing C_v (for 36 MHz discharge at 3 Pa).

DownLoad: Full-Size Img PowerPoint

3.2   Effect of harmonic excitation at the higher pressure

The above experiments were repeated under a higher chamber pressure of 16 Pa, with the other conditions being kept the same. Figure 8 shows the amplitudes and phases of the first and second harmonics for both voltage and current, changing with C_v. All behaviors shown in the figure are quite similar to what is observed at the lower pressure of 3 Pa. However, their influences on the discharge are different — the changes in EEPFs as well as the electron density and temperature are insignificant in the 16 Pa case, as shown in figure 9. The difference is due to the different electronic heating mechanisms at the pressure of 3 Pa and 16 Pa. At the pressure of 16 Pa, the plasma heating was dominated by the ohmic (collisional) heating occurring in the bulk plasma, while at 3 Pa, the plasma heating includes stochastic heating in addition to ohmic heating, which was related to the sheath motion [34]. We propose that the harmonics influenced the cause of the discharge via stochastic heating. This is reasonable because the harmonics are excited over the sheath (see figure 1(b)) due to its nonlinear feature. The discharge at higher pressure was therefore less affected by the harmonics due to its lack of stochastic heating.

Figure  8.  Changes of the amplitudes of the first and second harmonics of (a) voltage and (b) current, and (c) relative phase measured in radians (rad) of voltage and current with increasing C_v (for 36 MHz discharge at 16 Pa).

DownLoad: Full-Size Img PowerPoint

Figure  9.  Changes in (a) the EEPF and (b) the electron density and temperature with the increase in C_v (for 36 MHz discharge at 16 Pa).

DownLoad: Full-Size Img PowerPoint

To the best of our knowledge, there has not been a theory elucidating how the harmonics affect the electron heating with their relative phase difference. A detailed investigation into the phenomena causing the physical behaviors we observed in this experiment is needed to understand the theoretical aspects causing these behaviors. However, for practical applications, our observations suggest that self-excited high-order harmonics must be considered in the operation of low-pressure RF CCPs.

4.   Conclusions

The self-excitation of the second harmonic was significantly enhanced in the RF CCPs while tuning an external variable capacitor. At the lower pressure of 3 Pa, an abnormal transition of EEPF was observed with changes in the amplitude and relative phase of the harmonics, resulting in abrupt changes in the electron density and temperature. In contrast, the changes in EEPF as well as the electron density and temperature were insignificant at the higher pressure of 16 Pa under similar changes in the harmonics. The phenomena were related to the influence of the second harmonic on stochastic heating which was caused by both the amplitudes and the relative phase of the harmonics. Our results are meaningful to practical applications where self-excited high-order harmonics must be sufficiently considered utilizing low-pressure RF CCPs.