| Citation: | Guangjia WANG, Shidong FANG, Baoguo LIN, Chengzhu ZHU, Jie SHEN. Mechanistic study on 4, 4'-sulfonylbis removal with CO2/Ar gas-liquid DBD plasma[J]. Plasma Science and Technology, 2024, 26(10): 105501. DOI: 10.1088/2058-6272/ad5118 |
In this study, a single dielectric barrier discharge (DBD) coaxial reactor was used to degrade 4, 4'-sulfonylbis (TBBPS) in water using greenhouse gas (CO2) and argon as the carrier gases. The investigation focused on CO2 conversion, reactive species formation, gas-liquid mass transfer mechanism, and degradation mechanism of TBBPS during the discharge plasma process. With the decrease of CO2/Ar ratio in the process of plasma discharge, the emission spectrum intensity of Ar, CO2 and excited reactive species was enhanced. This increase promoted collision and dissociation of CO2, resulting in a series of chemical reactions that improved the production of reactive species such as ·OH, 1O2, H2O2 and O3. These reactive species initiated a sequence of reactions with TBBPS. Results indicated that at a gas flow rate of 240 mL/min with a CO2/Ar ratio of 1:5, both the highest CO2 conversion rate (17.76%) and TBBPS degradation rate (94.24%) were achieved. The degradation mechanism was elucidated by determining types and contents of reactive species present in treatment liquid along with analysis of intermediate products using liquid chromatography-mass spectrometry techniques. This research provides novel insights into carbon dioxide utilization and water pollution control through dielectric barrier discharge plasma technology.
The spherical tokamak device is a type of magnetic confinement fusion device that requires heating the plasma and maintaining sufficiently high temperature, density, and confinement time to achieve the Lawson criterion and sustain continuous light nucleus fusion. Effective heating of the plasma in the toroidal device is one of the important subjects in spherical tokamak plasma physics research [1–4]. Plasma heating methods are diverse, with one of the simplest being ohmic heating, akin to the principle of a transformer operating within the plasma. However, as the plasma’s resistivity decreases with temperature increasing, other auxiliary heating methods become necessary. One such method is neutral beam injection (NBI) heating, while another is radiofrequency (RF) wave heating. RF heating, in the context of ICRH and lower hybrid (LH) waves heating, involves selecting a specific frequency range of electromagnetic waves and coupling the high power generated by a wave power generator into the plasma via antennas or waveguides. This generates propagating eigenwaves in the plasma at the corresponding frequency bands, achieving heating of the plasma electrons or ions through various physical mechanisms such as cyclotron resonance, Landau damping, and transit-time magnetic pumping [5, 6].
Ion cyclotron resonance frequency (ICRF) heating method utilizes ion cyclotron resonance to heat ions, with additional mechanisms including Landau damping and transit-time magnetic pumping for direct electron heating, alongside mode conversion heating. Experimental evidence from tokamaks such as JET, EAST, Alcator C-Mod, and ASDEX Upgrade demonstrates the effectiveness of ICRF heating [7–10]. Importantly, fast waves in the ion cyclotron frequency range show no high-density limit, enabling propagation into the high-temperature, high-density core of the plasma, driving central currents, and yielding a broad distribution of heating and current drive [11, 12]. While the efficiency of fast wave current drive may be notably lower than achievable with LH waves, it remains quite acceptable given appropriate launcher design. Moreover, optimal efficiency alone is not the sole criterion for selecting a current drive (CD) system in ignited plasmas. Utilizing fast waves in this frequency range offers distinct advantages. High-power generators are readily available, facilitating penetration to the plasma core, and launching, comparatively simpler when contrasted with higher frequency heating and current drive methods [12].
Last year, a joint effort between the National Institute for Fusion Science in Japan and the US-based TAE Technologies successfully achieved the first measurements of hydrogen-boron fusion experiments in magnetic confinement fusion plasmas [13]. Subsequently, several fusion companies have shifted their focus towards the hydrogen-boron fusion route. Currently, hydrogen-boron fusion offers at least three advantages: firstly, it does not produce neutrons; secondly, it boasts abundant fuel resources; and thirdly, it enables direct conversion to electricity, unlike Deuterium-Tritium (D-T) fusion which primarily produces high-energy neutrons requiring thermal conversion processes. In hydrogen-boron (p-11B) fusion, the primary reaction products are high-energy alpha particles (helium nuclei), which are charged and can be directly converted to electricity using electrostatic fields. However, it faces the challenge that hydrogen-boron fusion requires ion temperatures of at least 150 keV to achieve high reaction rates. ICRF is the only RF heating method capable of directly heating ions, making it crucial for proton-boron plasmas [14].
The fundamental principles of ICRF physics mechanisms, such as enhanced left-hand RF polarization at the ion-ion hybrid (IIH) layer and Doppler shift effects for fast ions, have been widely recognized in the ICRF community for many years. Currently, a key focus is on the synergistic effect of NBI and ICRF heating to optimize high fusion performance in tokamaks. Another emphasis is on three-ion heating scenarios, as well as the feasibility of utilizing intrinsic impurities like 9Be to provide bulk ion heating for D-T plasmas. For instance, recent simulations for D-T plasmas on JET have assessed the optimal concentration ranges for ICRF fusion enhancement and bulk ion heating. For hydrogen (H) and 3He, simulations suggest that the 3He concentration should be maintained above 1.2%, while H should remain below 2.2% [15]. In experiments, the effectiveness of accelerating D-NBI ions has been demonstrated successfully in D-3He plasmas on JET. In this scenario, deuterons from the NBI system are accelerated to higher energies within the plasma core through ICRF, resulting in the generation of alpha particles from D-3He fusion reactions [16, 17].
Research on ICRF heating in p-11B plasma is just beginning, with future studies aiming to heat ions to the level of several hundred keV. From this perspective, ICRF heating in p-11B plasmas is of paramount importance. This work will investigate the primary heating characteristics of p-11B plasma, focusing particularly on minority heating, second harmonic heating, and the three-ion scheme. The structure of the paper is as follows. Section 2 presents the EHL-2 spherical tokamak and its parameters. Section 3 provides a scoping study of ICRF. In section 5, full-wave simulations of ion heating schemes are discussed. Finally, conclusions are drawn in section 5.
EHL-2 is designed as the next-generation spherical torus device to expedite the realization of commercial fusion using proton-boron as fuel [14]. The primary objectives of this device include achieving the p-11B thermal fusion reaction and validating the existence of hot ion modes characterized by a high ion-to-electron temperature ratio (Ti/Te⩾) at elevated ion temperatures. The design scenario of EHL-2 is hot-ion mode with T_{\mathrm{i}0}\sim30 keV, T\mathrm{_i}/T\mathrm{_e}\geqslant\text{ }2 , n_{\mathrm{e}0}\sim\left(1-3\right)\times 10^{20} m−3, I_{\mathrm{p}}\sim3 MA, {B_0} \sim 3 T. As the phase I stage of the roadmap for p-11B fusion based on spherical torus in ENN (Energy iNNovation), the construction of EHL-2 is estimated to be completed by 2026.
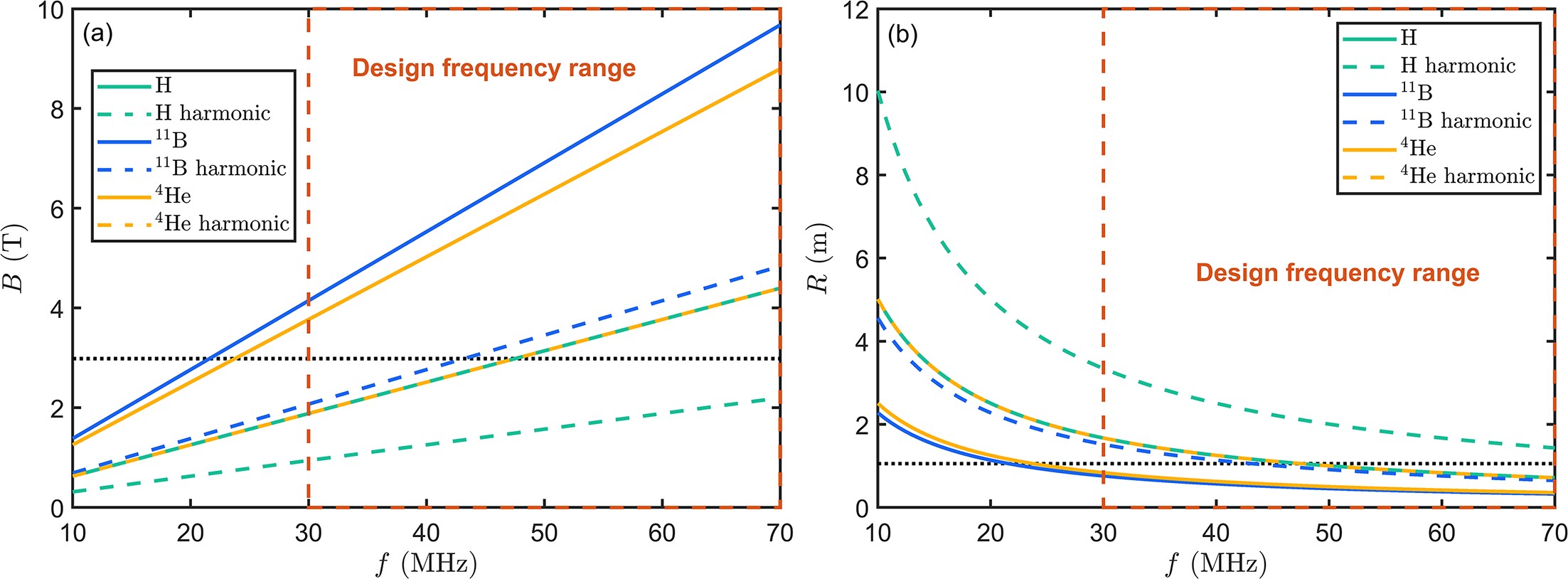
One of the challenges to achieving these target parameters is how to increase the ion temperature. As the only method that heating ions directly among all the RF heating methods, the ICRH system would be a favorable choice. To enhance the ion cyclotron heating efficiency in the device, it is essential to optimize the antenna’s parameter design. This involves scanning the frequency and spectrum of the antenna to determine a frequency and wave vector that yield superior ion heating effects. The full wave code TORIC has been used to finish this [18]. The data used in simulation are illustrated in figure 1, including the central magnetic field {B_0} \sim 2.984 T, the major radius R \sim 105.632 cm and a profile which gives the central electron density n_{\mathrm{e}0}\sim9.637\times10^{19} m−3, central electron temperature T_{\mathrm{e}0}\sim13.551 keV and the hot-ion mode with T\mathrm{_i}/T\mathrm{_e}=\text{ }1.5 . Figure 2(a) shows the magnetic field strength as a function of frequency to ensure that the resonance will be located on axis. Within the design frequency range of the EHL-2 device represented by the horizontal axis from 30 MHz to 70 MHz, only the curve of H fundamental resonance (which is overlapped by 4He second harmonic resonance) and 11B second harmonic resonance crossed the black dashed line, which indicates that the H fundamental, 11B and 4He harmonic on-axis resonance heating can be operated under the considered EHL-2 parameters with the frequency in the range of 40–50 MHz. One can also detect that the boron needs a little lower frequency compared to that of hydrogen at an identical magnetic field, and the location of boron will be little farther from the antenna than that of hydrogen. It suggests that the wave excited from the low-field side antenna will first encounter the fundamental resonance layer of hydrogen and suppress the harmonic resonance of boron. Consequently, we can expect a significant hydrogen fundamental resonance absorption with the proton-boron plasma in the condition of the EHL-2 device parameters.
To further determine the optimal heating frequency, we conducted a frequency scan using the TORIC code for the EHL-2 device. We performed TORIC simulations at intervals of 5 MHz within the range of 30–70 MHz, calculating the absorption profiles for different types of particles. We employed hydrogen-boron (H(11B)) plasma, with boron as the minority ion and X\left(^{11}\mathrm{B}\right)\text{ }=\text{ }6\% , {N_\phi } = {\text{ }}18 .
Figure 3 shows the power fraction absorbed by different particles species versus frequencies. Obviously, almost all the frequency ranges have a dominant electron absorption except the 36–46 MHz range for highly hydrogen fundamental absorption and the 16–18 MHz range for highly boron fundamental absorption. The curves related to boron ions exhibit sudden jumps at the positions of 16, 18, and 34 MHz. We have carefully examined the convergence of these simulations and the power balance, and found that even at very high resolutions, the simulations at these positions cannot maintain power balance. Moreover, the poloidal Fourier representation of the solution to the wave equation at the boundary position ( r/a = {\text{ }}0.9 ) fails to converge (see figure 4, m is the mode of Fourier spectrum). All these cases share the common feature: at the corresponding frequencies, the resonance layer of boron appears at the edge of the plasma, and the calculations indicate an inexplicable strong edge absorption. Therefore, we suspect that this may be caused by the steep temperature and density conditions at the plasma edge. In addition, Brambilla mentioned that it is also challenging to obtain convergent results when calculating hydrogen plasmas with a minority of 3He ions [19]. This is similar to the hydrogen-boron plasma discussed in our work, where the heavy ions act as minority ions. Although the calculation results for these cases are not very reliable, it is fortunate that we are not concerned with situations where the resonance layer is located at the edge of the plasma. What truly matters to us are the frequencies corresponding to the ion resonance layer or the ion-ion hybrid resonance layer being located in the core of the plasma.
In the low-frequency range (10–30 MHz), although the fundamental resonance of boron falls within this band, the absorption is still predominantly governed by electrons in most cases. For cases with frequencies below 14 MHz, there are no ion resonance layers within the plasma, resulting in all the absorption being due to electrons. Figure 5(a) shows the one-dimensional absorption profile at 20 MHz, where the fundamental resonance layer of boron ions is located at r/a = 0.2 . At this point, the ion-ion hybrid resonance layer has not yet entered the plasma, so boron ion absorption can be observed at the resonance layer position. However, the overall absorption is still dominated by electrons. As the frequency increases, the ion-ion hybrid resonance layer gradually enters the plasma, and most of the power is deposited onto electrons through Landau damping of the mode-converted ion Bernstein wave (IBW). Therefore, in figure 3, electron absorption is the main component of absorption in the 20–30 MHz range. This conclusion is consistent with results from other devices like BPX [20] and JET [21, 22] using heavy ion minority species, where a very low concentration of minority ions is typically required to observe significant ion absorption. This is because the presence of heavy minority ions causes the wave to first encounter the hybrid resonance layer, undergo mode conversion, and then reach the minority resonance layer. Additionally, even a slightly higher concentration of minority ions will cause the minority resonance layer to move away from the hybrid resonance layer, significantly reducing minority ion absorption.
The 36–46 MHz range, particularly around 40 MHz, is a frequency band with significant ion absorption. The fundamental absorption of hydrogen ions peaks at 42 MHz (~ 60%). We choose 40 MHz as a typical representative of this frequency band for further analysis. Figure 5(b) shows the particles’ absorption distribution along the radial direction when f = 40 MHz, most of the power deposition occurs within the range of r/a = 0.1–0.3, and most power in this range is deposited on hydrogen by its fundamental absorption. Hence, we can choose the frequency range from 38 to 44 MHz as the EHL-2 operation frequency range to obtain a more effective on-axis ion heating, and this frequency range is within the initial designed range exactly. On the other hand, heating hydrogen will be better than boron because of the large charge-mass ratio ( Z_{\mathrm{H}}/A\mathrm{_H}=\text{ }1 ) and the higher abundance ( n_{\mathrm{H}}/n\mathrm{_e}=\text{ }0.7 ) of hydrogen in plasma, it is also the expected result of ICRF system in EHL-2. The question is why the second harmonic absorption of boron is so low (excluding cases with numerical non-convergence). We can infer the reason from the information in figure 2. Since the charge-to-mass ratio of boron is approximately 1/2, the second harmonic resonance layer of boron is close to the fundamental resonance layer of hydrogen and is situated closer to the high-field side compared to the fundamental resonance layer of hydrogen. Therefore, in almost all cases, the waves emitted by the antenna first encounter the fundamental resonance layer of hydrogen and deposit most of the power into hydrogen ions.
Above 65 MHz, harmonic absorption of hydrogen occurs. However, it will exceed the designed frequency range if we want an excellent hydrogen harmonic absorption, prompting exclusion of hydrogen harmonic absorption from consideration. In other frequency ranges, electron absorption predominantly takes place, especially at 65 MHz, where electron absorption constitutes over 90%. Figure 5(c) shows that the absorption is concentrated in the core region of the plasma. Therefore, this frequency can be used as the operation scenario for fast wave current drive (FWCD). By comparing figures 2 and 3, we observe an approximate 5 MHz shift in the absorption fractions calculated by TORIC when compared to the theoretical estimates based on resonance location. This discrepancy may be attributed to the Shafranov shift of magnetic axis [19]. Apart from this shift, the overall features are generally consistent.
The 2D contour of Re(E+) field has been shown in figure 6. Corresponding to the analysis above, the wave field exhibits a helical structure when f = 10 MHz, whole of the plasma region shows a significant weak polarization and there is no resonance layer for ions throughout the entire plasma region. As a result, almost all wave power is absorbed by electrons. As the increase in frequency, the fundamental resonance layer of boron ion starts moving into the plasma, and the boron ion absorption is observed, but due to the weaker left-hand polarization at the resonance layer, the absorption proportion is lower than that of electrons. As the ion-ion hybrid resonance layer enters the plasma, mode-converted short-wavelength waves appear. In the 2D electric field plot at 30 MHz shown in figure 6(c), the propagation of the IBW towards the high-field side on the equatorial plane and the backward propagation of the ion cyclotron wave (ICW) along the poloidal direction can be clearly seen.
Previous results show that there is significant majority hydrogen absorption around 40 MHz, which differs from the conventional ion absorption theory. According to single species theory, it has been observed that fundamental absorption is generally weak due to the zero left-hand polarization field at the resonance layer [1, 23]. To address this issue, a solution is to introduce minority ions to deposit power into minority, which then transfer energy to the majority through collisions. In contrast to hydrogen plasmas with minority of boron ions, the charge-to-mass ratio of the minority ions typically needs to be greater than that of the majority one. In this scenario, significant majority ion absorption is generally not observed. In our situations, the introduction of boron modifies the polarization at the position of the hydrogen resonance layer, causing it to deviate from zero, thereby enhancing the absorption of hydrogen’s fundamental frequency. One can see the enhanced left-hand polarization at the resonance position in figure 6(d). The pronounced absorption observed around the resonance layer location in figure 7 corroborates the aforementioned findings. Additionally, besides the strong absorption observed near the resonance layer position, we can also see a wide range of fundamental hydrogen absorption within the plasma. This is due to the use of the hot-ion mode in our simulations, where the high ion temperature broadens the Doppler width of the resonance. Consequently, hydrogen ions over a large area can resonate and absorb power. Beyond a frequency of 40 MHz, the wave field exhibits typical large-scale fast wave structures in most regions of the poloidal section. That is because the ion resonance layer is so distant and most of the power is directly absorbed by electrons before the wave reaches the resonance layer.
In summary, both the intensity and location of fundamental absorption for hydrogen are relatively favorable, making it a more suitable choice as the frequency parameter for the ICRF antennas. Therefore, we suggest that fundamental heating of H at the frequency of ~ 40 MHz is the optimal ion heating scheme for H-(11B) plasma under the parameters of EHL-2, and the frequency of ~ 65 MHz can be used as the low frequency operation scenario for FWCD.
The results of the frequency scan provide us with a general understanding of how antenna parameters influence the absorption. Here we also scanned the toroidal mode number {N_\phi } , corresponding to the wave spectrum k|| as {N_\phi } \sim {k_{||}}R , where R is the radial position of the antenna, to find the best ion heating parameters of antenna for EHL-2. The power fraction coupled to various species versus the {N_\phi } is shown in figure 8. Obviously, opposite influence on hydrogen ion and electron has been exhibited when f = 40 MHz, the large {N_\phi } is better for the heating of hydrogen so that the parameter of EHL-2 antenna can take as {N_\phi } = 26 for an optimal heating of H. While f = 65 MHz, which is suggested operation frequency for current drive, the impact of {N_\phi } on absorption is small, the electron absorption consistently exceed 80% at almost all the values of {N_\phi } . Within the range of {N_\phi } = 16–26, nearly all the power coupled to the electron. Comparing the particle absorption at two different frequencies, we can see a similar low toroidal mode number range for the absorption trends in change. Specifically, this range represents {N_\phi } = 4–14 in both panel (a) and panel (b) of figure 8.
To delve further into the intricacies of ion absorption as a function of the toroidal mode number or wave spectrum, we present the radial profiles of fundamental absorption for hydrogen (H) at {N_\phi } = 4–30 in figure 9 and the 2D contours of poloidal section with the Re(E+) field and hydrogen fundamental absorption in figure 10. Combining figures 9 and 10, we can clearly understand what happens as {N_\phi } increases. It arises from the fact that {N_\phi } , or more precisely {k_{||}} , can influence the accessibility of fast waves to the plasma. A higher toroidal mode number indicates a larger parallel wave vector, facilitating the permeation of waves into the core plasma with greater difficult as the corresponding perpendicular wave number is relatively low. From the two-dimensional polarized electric field plot, we can clearly see this point. When {N_\phi } = 4, the wave has a stronger ability to propagate in the poloidal plane, reaching the hybrid resonance layer located on the side farthest from the antenna. This results in a significant amount of short-wavelength mode-converted waves, leading to more electron absorption compared to ion absorption. Additionally, the left-hand polarization at the hydrogen ion resonance layer is relatively weaker, resulting in weaker hydrogen ion absorption in the plasma core region compared to higher {N_\phi } . As {N_\phi } increases, the wave’s ability to propagate in the poloidal plane weakens, the perpendicular wave number decreases, and the wavelength of the fast wave observed in the electric field plot increases. This leads to a certain degree of enhanced polarization at the resonance layer position (which is particularly evident in the {N_\phi } = 28 plot of figure 10(c)). Consequently, there is a significant and concentrated amount of fundamental hydrogen absorption in the plasma core, which is shown in figures 8 and 10(f).
In summary, whether in the context of ion heating at f = 40 MHz or current drive at f = 65 MHz, a higher toroidal mode number translates to improved performance. Specially, within the parameter range we investigated, {N_\phi } = 26 can be an optimal choice for both ion heating and current drive applications.
Several typical ion cyclotron heating schemes include single ion fundamental heating, minority heating, majority second harmonic heating and three-ions heating. In the aforementioned antenna parameter scan, a hydrogen plasma doped with a small fraction of boron ions (X(11B) = 6%) was utilized. Based on the resonance location analysis and parameter scan results, it can be concluded that the effectiveness of minority heating is not satisfactory across the entire antenna design frequency range (30–70 MHz). Relatively, the majority fundamental heating tends to be more effective, but it also faces a challenge where the proportions of electron absorption are comparable with it of ion absorption, this is the primary problem we want to resolve next. Here, we consider the heating schemes of hydrogen plasma with a boron minority, and the optimal minority ion concentration will be explored for the effective ion heating.
According to classical minority ion heating theory, changes in minority ion concentration can influence the location of the hybrid resonance layer, thereby impacting the efficiency of ion heating [24]. Considering the charge conservation of plasma, we maintain the concentration of 11B within the range of 1%–19.5%, scanning at intervals of ΔX(11B) = 0.5%. Figure 11 illustrates the absorption distribution on each species with different concentrations of 11B, the impact of concentration on absorption is relatively minor, with significant variations occurring mainly in the high concentration range. At f = 40 MHz and the lower concentration range of boron, absorption is primarily contributed by the fundamental absorption of hydrogen and electron absorption, with their difference not particularly pronounced (ions being 20%–30% higher than electrons). However, after X(11B) exceeds 7%, the contribution of hydrogen fundamental absorption shows an increasing trend. Correspondingly, electron absorption decreases. If we consider a boron ion concentration exceeding 10%, which means that the hydrogen ion concentration will be below 50% due to charge conservation, ion absorption peaks at X(11B) = 17%, with the absorption component exceeding 80%. At this point, the hydrogen ion concentration is only 15%, resembling more of a heating scheme where hydrogen ions act as the minority species.
To show that how minority concentration influences the location of hybrid resonance and the absorption of fundamental of hydrogen, we present the 2D contours of E+ with different 11B concentrations in figure 12. We first focus on the low boron concentration cases shown in panel (a), which represent a boron ion concentration of 6%. Due to the significant charge-to-mass ratio difference between hydrogen and boron ions, the width between their fundamental resonance layers is large. At the frequency of 40 MHz, the fundamental resonance of hydrogen is located in the plasma core, while the fundamental resonance layer of boron is already at the high-field side edge. Increasing the boron ion concentration from zero essentially shifts the position of the hybrid resonance layer from the boron fundamental resonance location towards the hydrogen fundamental resonance location. At low concentrations ( \leqslant 7%), the hybrid resonance layer is closer to the high-field side edge boron resonance layer, too far from the hydrogen fundamental resonance layer to affect hydrogen fundamental absorption. Additionally, since the hydrogen ion fundamental resonance layer is the closest resonance layer to the antenna, the particle absorption is mainly provided by hydrogen ions and electrons. As the concentration of boron ions continues to increase, even exceeding 10%, where the hydrogen ions no longer constitute the majority component of the plasma, leading to a scenario similar to classical minority heating. When the hybrid resonance layer moves sufficiently close to the low-field side, the fast wave reaches the hybrid resonance layer, undergoes mode conversion, and generates backward-propagating ICW, thereby enhancing polarization and absorption at the hydrogen fundamental resonance position. This process is clearly illustrated in figures 12(b)–(d). Finally, as the boron ion concentration increases further, the hybrid resonance layer gets so close to the hydrogen fundamental resonance layer that hydrogen fundamental absorption competes with electron absorption. Consequently, the proportion of electron absorption begins to increase, while ion absorption decreases. The corresponding changes in absorption components can be observed in figure 10 when the boron ion concentration is in the range of 17%–19.5%.
Here we conclude that achieving higher ion absorption requires reducing the concentration of hydrogen ions and increasing the concentration of boron ions. Strictly speaking, due to the fact that hydrogen ions carry a charge of 1, while boron ions have a charge of 5, the contribution of boron ions to ion charge accounts for 80%. Consequently, in this scenario, the state is closer to the conventional minority fundamental heating scheme where light ions (H) serve as the minority. Extensive experiments and simulations have demonstrated the feasibility of this heating scheme. However, for proton-boron fusion, the increase in boron concentration is a subject of debate. On one hand, very low boron ion concentrations are necessary to effectively heat the minority species, but this may reduce the fusion reaction rate. On the other hand, increasing the boron concentration to focus absorption on hydrogen ions, as demonstrated in this paper, may present another feasible approach. Therefore, considering the specific requirements of proton-boron fusion, choosing a moderate boron ion concentration becomes crucial. The simulation results presented in this paper can provide valuable insights for making such decisions.
In this study, we conducted parameter scans for EHL-2 ICRH, using the TORIC code, and discussed the feasibility of various heating schemes. In summary, the scenarios that can be used for heating and current drive are identified as follows.
(i) For H fundamental heating in H(11B) plasma, the parameters of f = 42 MHz and Nϕ = 28 are recommended for a suggested boron concentration of 17%.
(ii) For current drive in plasma of H majority and 11B minority, the frequency of ~ 65 MHz can be used as an operation scenario for FWCD.
Unlike the more common fusion reactions such as D-T or D-D fusion, p-11B fusion involves fuel ions with significantly different charge-to-mass ratios. Additionally, the conditions required for initiating the fusion process are more stringent, increasing the complexity of selecting an efficient heating scheme. Our research conducted a parameter scan using the TORIC code for EHL-2 and discussed the feasibility of various heating schemes. The results are notably distinct from D-T or D-D fusion; we find that H, as the majority species, exhibits more efficient absorption compared to the minority 11B ions.
In more conventional fusion heating schemes, minority ions (usually light ions) are typically heated at the fundamental frequency, while heating of the majority cannot be sufficiently achieved due to polarization reasons. However, in our consideration of the unique characteristics of p-11B fusion, we adopt 11B as the minority ion. Under the configuration of EHL-2, the distance between the two cyclotron resonance layers is significant, such that when one fundamental resonance position is at the core, the other resonance position is already almost beyond the plasma’s range. In the low-frequency range, if only the fundamental resonance layer of boron ions is within the plasma, ion absorption will be less than electron absorption due to weak electric field polarization. To improve the polarization of the resonance layer using the hybrid resonance layer, a very low minority ion concentration is required. However, given the significant charge-to-mass ratio difference between hydrogen and boron, it is challenging to find an appropriate minority ion concentration that makes boron absorption significant. Additionally, since both hydrogen and boron are direct fuels for the EHL-2 fusion scheme, the boron concentration cannot be set too low, as it would adversely affect the fusion reaction rate. Under this situation, we have found a method to enhance the fundamental absorption of hydrogen by increasing the concentration of boron ions, which brings the hybrid resonance layer closer to the fundamental resonance layer of hydrogen. At around 40 MHz, with a boron ion concentration of 17% and a hydrogen ion concentration of 15%, hydrogen ion absorption can be maximized, reaching up to 80% of ion absorption. This heating method is of great value for hydrogen-boron fusion. Certainly, this contradicts our initial consideration of using boron ions as the minority species.
Despite this, there are still many issues in our study that require further investigation. The first is the convergence problem of the TORIC code. When the boron ion resonance layer is located at the plasma edge, the Fourier mode coefficients at the edge do not converge. This issue may be caused by the large temperature and density gradients at the plasma edge in the profiles we used, and the impact of these edge conditions is magnified due to the large mass of boron ions. Additionally, in our simulations, we used a plasma profile with the hot-ion mode. The high ion temperature significantly expands the range of hydrogen ion absorption through Doppler broadening, as clearly seen in the 2D absorption results. Since the importance of the hot-ion mode in the ENN hydrogen-boron fusion roadmap, further research is needed to understand the enhancement effect of high ion-to-electron temperature ratio on absorption. While there has been a few research on heavy ion minority heating (or inverted minority heating) in stellarator devices [25, 26] and tokamak devices [20–22, 27]. Most researches focus on minority ions heating, so even with a very low minority concentrations, electron absorption remains dominant in these results. In simulations of the reverse minority (D plasma with T minority) in ITER by Yin et al [28], the large scale of the ITER device allowed the D resonance layer to be moved outside the plasma, enhancing the absorption of minority T. In our work, the EHL-2 device is much smaller than ITER, and due to the convergence issues of TORIC when dealing with high-mass minority ions, we did not observe significant boron ion absorption. This issue requires further simulation and experimental analysis. Recently, the three-ion heating scheme, where the plasma components satisfy the charge-to-mass ratio constraint of (Z/A)2 < (Z/A)3 < (Z/A)1, has been implemented across various devices [16, 24, 25, 28, 29]. Unlike EHL-2, these devices utilize the three ions with similar charge-to-mass ratios, and heat minority directly. In future work, a detailed three-ion heating scheme for hydrogen-boron plasmas deserves to be investigated. This has significant implications for devices that utilize hydrogen-boron as fusion fuel.
Moreover, these insights shed light on operational considerations for EHL-2 and offer valuable guidance for its practical implementation. They also hold promise for informing related research endeavors within the fusion studies domain. As a precursor project to EHL-2, EXL-50U has been operational since early this year. The findings presented in this paper pertaining to p-11B fusion can be further validated on this platform, thereby advancing our understanding, and paving the way for future developments in fusion research.
This work was supported jointly by National Natural Science Foundation of China (No. 51877208), Anhui Provincial Key R&D Programmers (No. 202004a07020047).
| [1] |
Xiong P et al 2019 Environ. Sci. Technol. 53 13551 doi: 10.1021/acs.est.9b03159
|
| [2] |
Pirard C and Charlier C 2018 Chemosphere 211 918 doi: 10.1016/j.chemosphere.2018.08.012
|
| [3] |
Wang R et al 2023 Environ. Pollut. 334 122121 doi: 10.1016/j.envpol.2023.122121
|
| [4] |
Fu Y Y et al 2019 Water Res. 150 12 doi: 10.1016/j.watres.2018.11.051
|
| [5] |
Gou Y L et al 2020 Chem. Eng. J. 394 125040 doi: 10.1016/j.cej.2020.125040
|
| [6] |
Trenchev G and Bogaerts A 2020 J. CO2 Util. 39 101152 doi: 10.1016/j.jcou.2020.03.002
|
| [7] |
Yan X et al 2014 Chem. Eng. J. 245 41 doi: 10.1016/j.cej.2014.01.089
|
| [8] |
Wang Y L et al 2019 ACS Catal. 9 10780 doi: 10.1021/acscatal.9b02538
|
| [9] |
Wang L et al 2020 Chem. Commun. 56 14801 doi: 10.1039/d0cc06514e
|
| [10] |
Shang K F et al 2022 Chem. Eng. J. 431 133916 doi: 10.1016/j.cej.2021.133916
|
| [11] |
Shang K F, Li J and Morent R 2019 Plasma Sci. Technol. 21 043001 doi: 10.1088/2058-6272/aafbc6
|
| [12] |
Lukes P Interactions of non-equilibrium plasma with liquids: physics, chemistry and applications In Proceedings of 2018 IEEE International Conference on Plasma Science Denver: IEEE 2018: 1 doi: 10.1109/icops35962.2018.9575834
|
| [13] |
Wang X P et al 2021 Environ. Sci.: Water Res. Technol. 7 610 doi: 10.1039/d0ew00985g
|
| [14] |
Yin N Y et al 2018 Environ. Sci. Technol. 52 5459 doi: 10.1021/acs.est.8b00414
|
| [15] |
Zhou X F et al 2023 J. Phys. D: Appl. Phys. 56 455202 doi: 10.1088/1361-6463/acec81
|
| [16] |
Nayak G et al 2023 Plasma Process. Polym. 20 2200222 doi: 10.1002/ppap.202200222
|
| [17] |
Mei D H and Tu X 2017 ChemPhysChem 18 3253 doi: 10.1002/cphc.201700752
|
| [18] |
Mercado-Cabrera A et al 2017 Plasma Sci. Technol. 19 077501 doi: 10.1088/2058-6272/aa6715
|
| [19] |
Scholtz V et al 2015 Biotechnol. Adv. 33 1108 doi: 10.1016/j.biotechadv.2015.01.002
|
| [20] |
Wang L et al 2018 ACS Catal. 8 90 doi: 10.1021/acscatal.7b02733
|
| [21] |
Mei D H et al 2015 Plasma Sources Sci. Technol. 24 015011 doi: 10.1088/0963-0252/24/1/015011
|
| [22] |
Mora E Y, Sarmiento A and Vera E 2016 J. Phys.: Conf. Ser. 687 012020 doi: 10.1088/1742-6596/687/1/012020
|
| [23] |
Nagassou D et al 2020 J. CO2 Util. 38 39 doi: 10.1016/j.jcou.2020.01.007
|
| [24] |
Bogaerts A et al 2017 Plasma Sources Sci. Technol. 26 063001 doi: 10.1088/1361-6595/aa6ada
|
| [25] |
Shen J et al 2019 Chem. Eng. J. 362 402 doi: 10.1016/j.cej.2019.01.018
|
| [26] |
Yoshio Nosaka et al 2017 Chem. Rev. 117 17 doi: 10.1021/acs.chemrev.7b00161
|
| [27] |
Hall R D et al 1989 J. Biol. Chem. 264 7900 doi: 10.1016/S0021-9258(18)83128-3
|
| [28] |
Ceppelli M et al 2021 Plasma Sources Sci. Technol. 30 115010 doi: 10.1088/1361-6595/ac2411
|
| [29] |
Ramakers M et al 2015 Plasma Process. Polym. 12 755 doi: 10.1002/ppap.201400213
|
| [30] |
Xu S J, Whitehead J C and Martin P A 2017 Chem. Eng. J. 327 764 doi: 10.1016/j.cej.2017.06.090
|
| [31] |
Sarani A, Nikiforov A Y and Leys C 2010 Phys. Plasmas 17 063504 doi: 10.1063/1.3439685
|
| [32] |
Zhang Z L et al 2017 Plasma Chem. Plasma Process. 37 415 doi: 10.1007/s11090-017-9784-z
|
| [33] |
Liu K et al 2022 Vacuum 198 110901 doi: 10.1016/j.vacuum.2022.110901
|
| [34] |
Liu Z J et al 2023 IEEE Trans. Plasma Sci. 51 2295 doi: 10.1109/tps.2023.3296112
|
| [35] |
Blajan M and Shimizu K 2012 IEEE Trans. Plasma Sci. 40 1730 doi: 10.1109/tps.2012.2190994
|
| [36] |
Moldgy A et al 2020 J. Phys. D: Appl. Phys. 53 434004 doi: 10.1088/1361-6463/aba066
|
| [37] |
Wang X P et al 2020 Sep. Purif. Technol. 240 116659 doi: 10.1016/j.seppur.2020.116659
|
| [38] |
Zhang C et al 2017 J. Hazard. Mater. 326 221 doi: 10.1016/j.jhazmat.2016.12.034
|
| [39] |
Wang T C et al 2018 Chem. Eng. J. 346 65 doi: 10.1016/j.cej.2018.04.024
|
| [40] |
Wang C et al 2018 Chem. Eng. J. 346 159 doi: 10.1016/j.cej.2018.03.149
|
| [41] |
Ozkan A et al 2016 Plasma Sources Sci. Technol. 25 025013 doi: 10.1088/0963-0252/25/2/025013
|
| [42] |
Takamatsu T et al Investigation of bacterial inactivation by various gas plasmas and electron microscopic observation of treated bacteria In: Proceedings of 2015 IEEE International Conference on Plasma Sciences Antalya: IEEE 2015 doi: 10.1109/plasma.2015.7179641
|
| [1] | Kerong HE (何科荣), Hui CHEN (陈辉), Sanqiu LIU (刘三秋). Effect of plasma absorption on dust lattice waves in hexagonal dust crystals[J]. Plasma Science and Technology, 2018, 20(4): 45001-045001. DOI: 10.1088/2058-6272/aaaadb |
| [2] | Jiafeng JIANG (蒋佳峰), Jiangang LI (李建刚), Yuanhua DONG (董元华). Effect of cold plasma treatment on seedling growth and nutrient absorption of tomato[J]. Plasma Science and Technology, 2018, 20(4): 44007-044007. DOI: 10.1088/2058-6272/aaa0bf |
| [3] | Mohsen AFSHARMANESH, Morteza HABIBI. Directional power absorption in helicon plasma sources excited by a half-helix antenna[J]. Plasma Science and Technology, 2017, 19(10): 105403. DOI: 10.1088/2058-6272/aa8030 |
| [4] | Xingyu GUO (郭星宇), Zhe GAO (高喆), Guozhang JIA (贾国章). One-dimensional ordinary–slow extraordinary–Bernstein mode conversion in the electron cyclotron range of frequencies[J]. Plasma Science and Technology, 2017, 19(8): 85101-085101. DOI: 10.1088/2058-6272/aa6a50 |
| [5] | F. PETERS, J. HIRSCHBERG, N. MERTENS, S. WIENEKE, W. VI¨OL. Comparison of Nitric Oxide Concentrations in μs-and ns-Atmospheric Pressure Plasmas by UV Absorption Spectroscopy[J]. Plasma Science and Technology, 2016, 18(4): 406-411. DOI: 10.1088/1009-0630/18/4/13 |
| [6] | DONG Yunsong (董云松), YANG Jiamin (杨家敏), SONG Tianming (宋天明), ZHU Tuo (朱托), HUANG Chengwu (黄成武). Radiation Hydrodynamic Simulations in the Planar Scheme for the Fundamental Studies of Shock Ignition[J]. Plasma Science and Technology, 2016, 18(4): 376-381. DOI: 10.1088/1009-0630/18/4/08 |
| [7] | M. HANIF, M. SALIK, M. A. BAIG. Diagnostic Study of an Fe-Ni Alloy Plasma Generated by the Fundamental (1064 nm) and Second (532 nm) Harmonics of an Nd: YAG Laser[J]. Plasma Science and Technology, 2013, 15(5): 397-402. DOI: 10.1088/1009-0630/15/5/01 |
| [8] | Y. YOSHIMURA, S. KUBO, T. SHIMOZUMA, H. IGAMI, H. TAKAHASHI, M. NISHIURA, S. OGASAWARA, R. MAKINO, T. MUTOH, H. YAMADA, A. KOMORI. High Density Plasma Heating by EC-Waves Injected from the High-Field Side for Mode Conversion to Electron Bernstein Waves in LHD[J]. Plasma Science and Technology, 2013, 15(2): 93-96. DOI: 10.1088/1009-0630/15/2/02 |
| [9] | XI Yanbin (奚衍斌), LIU Yue (刘悦). FDTD Simulation on Power Absorption of Terahertz Electromagnetic Waves in Dense Plasma[J]. Plasma Science and Technology, 2012, 14(1): 5-8. DOI: 10.1088/1009-0630/14/1/02 |
| [10] | LI Bin, LI Hong, CHEN Zhipeng, XIE Jinlin, FENG Guangyao, LIU Wandong. Experimental and Simulational Studies on the Theoretical Model of the Plasma Absorption Probe[J]. Plasma Science and Technology, 2010, 12(5): 513-518. |
| 1. | Ma, H.-J., Xie, H.-S., Sun, G.-L. et al. Simulation of ion cyclotron range of frequencies heating in the proton-boron plasma of the spherical tokamak. Plasma Physics and Controlled Fusion, 2025, 67(2): 025019. DOI:10.1088/1361-6587/ada8dc |
| 2. | Jiang, X., Shi, Y., Song, S. et al. Physics design of current drive and strategy of heating system for EHL-2 spherical torus. Plasma Science and Technology, 2025, 27(2): 024012. DOI:10.1088/2058-6272/adae71 |