Surface charge characteristics in a three-electrode surface dielectric barrier discharge

1.   Introduction

Ion cyclotron resonance heating (ICRH) stands out as a primary auxiliary heating method in numerous stellarators and tokamak devices [1–4]. Its key advantage lies in its ability to achieve central power deposition at high plasma densities while directly heating the plasma ions [1]. The ICRH system encompasses radio frequency (RF) generators, a transmission system, an ICRH antenna, and an impedance matching system. A significant challenge for achieving high-power and long-pulse operation in both tokamaks and stellarators is enhancing the power coupling while minimizing the reflected power of the ICRH antenna system. Various experimental studies and simulations have been conducted to improve the antenna coupling and the associated impedance matching techniques [5–10].

The initial attempt to theoretically formulate antenna-plasma coupling utilized the slab model. This model assumed the antenna had infinite poloidal length (2D model) and finite toroidal length [11]. The assumption included representing the current-carrying conductor as a current sheet with uniform current distribution, focusing solely on exciting the fast-wave component of ICRH in the plasma. However, handling the mesh of the 3D antenna fine structure (septum, limiter, antenna box, etc.) in these codes proves more challenging [12]. Furthermore, these codes demand significant computational resources for accurate spectral transform calculations. Subsequently, the 3D electromagnetic (EM) code CST MWS [13] was employed to handle the complex 3D ICRH antenna structure with a dielectric medium instead of plasma loading. This code is limited to loading isotropic plasma, utilizing materials with a high dielectric constant, like sea water or barium titanate ceramic powders mixed with water [14]. Commercial software like COMSOL is commonly used for handling complex wave launcher geometry and an inhomogeneous-anisotropic radiation mediums using the finite element method (FEM) in plasma simulation [4, 12, 15, 16]. Many studies leverage COMSOL simulations to explore the coupling between a 3D antenna and an inhomogeneous plasma for ICRH, predicting relative coupling resistance, power spectrum, Poynting vector, and other relevant parameters [5, 12, 17].

Considering that the antenna impedance is influenced by varying boundary conditions in front of it, three main methods are employed to address these variations. The first matching method involves inserting additional impedances between the antenna and the transmitter, achieving impedance matching by adjusting the complex impedance of the load. Common adjustable loads on tokamak devices include adjustable capacitors, liquid stub tuners, and ferrite tuners [18–23]. The second method compensates for changes in the antenna input impedance by adjusting the load of the Conjugate-T loop, ensuring that the input impedance of the equivalent load matches the characteristic impedance of the transmission line [24–26]. The third matching method utilizes network components (Circulator, 3 dB hybrid coupler) to achieve impedance matching by transferring reflected power to the virtual load, ensuring that the transmitted signal remains unaffected by load changes. The network components can be combined with any of the above methods to provide a pre-matching, but at a higher cost [8]. Ferrite tuners (FT), capitalizing on the change in magnetic properties of a ferritic material in the presence of an applied magnetic field, offer the advantage of operating in milliseconds [8]. The Alcator C-Mod tokamak, for instance, employs a double-stub FT system, along with one constant-length stub, on the transmission line of the ICRH antennas, maintaining real-time reflection power to the transmitters at less than 1% across a wide range of plasma conditions [23]. The high-power fast-response double-stub FT on EAST demonstrated good performance [27], in terms of response time, differential phase shift, and insertion loss during ion cyclotron heating, warranting further study on the impedance matching effect of ferrite stubs for EAST ICRH antennas.

In this study, we present a program developed using COMSOL to integrate the coupling of EAST four-strap antenna with plasma and the impedance matching of double-stub FT. The program can extract various experimentally relevant physical quantities, including the impact of the fine antenna structure on coupling impedance, antenna power spectrum, reflection coefficients, S-matrix, voltage distribution, and optimal matching settings. The organization of this paper is as follows.

In section 2, we introduce the models and equations of the code for the coupling between the EAST four-strap antenna and plasma. Section 3 discusses the relationships between reflection coefficients, S-matrix, and frequency. Section 4 demonstrates the impact of the fine structure, including the FS and antenna ports, on coupling characteristics. Section 5 briefly introduces the models and equations of the code for the double-stub FT impedance matching during the four-strap ICRH antenna heating plasma, and also compares the simulation results of the optimized antenna model and the reference antenna model. The conclusions of the study are presented in section 6.

2.   Models and formulation

2.1   Models

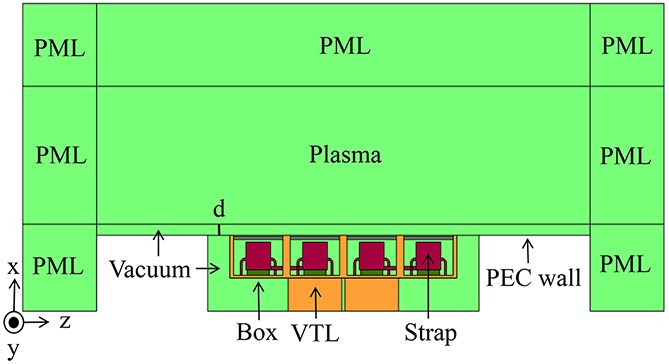
Figure 1 shows a 3D model of the ICRH antenna and plasma coupling with Perfectly Matched Layers (PML) boundary in the ICACIMSFT code (the ICRH antenna coupling with the impedance matching system of the ferrite tuner). The radial, poloidal, and toroidal directions of the tokamak are represented by x, y, and z, respectively. The $x'y'z'$ coordinate system is a new system with the magnetic field ${\mathit{B}}_{0}$ along the $z'$-axis direction. The metal wall of the tokamak is assumed to be a perfect electrical conductor (PEC) wall, positioned at a distance of d = 4 cm from the plasma. The antenna model, based on the four-strap ICRH antenna installed in EAST, includes a FS, four folded toroidal straps, vacuum transmission lines, and an antenna box (figures 2(a) and (b)). The box dimensions are 821 mm in height, 900 mm in width, and 155 mm in depth. Three septums in the antenna box prevent coupling between the straps, and the FS rods are inclined at an angle of 7° to the horizontal plane. The strap is fed by a vacuum transmission line (VTL). RF waves propagate between the inner and outer conductors, while the outer conductors are grounded for safety. The current distribution on the straps is shown in figure 2(c). The polar positions of the upper and lower VTL are symmetrical with respect to the center O-point of the antenna, and the polar distance between VTL 1 and VTL 2 is denoted as ${d}_{\mathrm{p}\mathrm{o}\mathrm{r}\mathrm{t}}$ (figure 2(d)).

Figure  1.  Schematic diagram of coupling between 3D ICRH antenna and plasma.

DownLoad: Full-Size Img PowerPoint

Figure  2.  Overview of the 3D EAST four-strap antenna. (a) Front face of the antenna with FS, (b) four CSs fed with VTL, (c) current distribution on the straps, and (d) equivalent circuit diagram for the CS #2.

DownLoad: Full-Size Img PowerPoint

2.2   Boundary conditions

The antenna and antenna box are both used as impedance boundaries, and are made of 316L stainless steel [28]. The vacuum chamber wall is a PEC boundary, surrounding one side of the plasma. The remaining five sides of the plasma are surrounded by PML [29].

2.3   Settings for the plasma model

In the plasma region, a cold-plasma model is employed, where the background magnetic field forms a 7-degree angle with the z-axis. The plasma, composed of 90% deuterium and 10% hydrogen, remains charge-neutral. The plasma density distribution is divided into four regions, (I) the scraping layer, (II) the index attenuation region, (III) the parabolic region, and (IV) the center region. The electron density in each region is expressed as follows:

Adjusting a₁, a₂ and a₃ can change the profile of density (figure 3). The magnetic field profile satisfies $B\left(x\right) = {B}_{0}/[1+(-x+{ap}_{0})/{R}_{0}]$, and ${B}_{0}$, ${R}_{0}$ and ${ap}_{0}$ are the magnetic field at plasma core, the major radius, and the distance from the antenna to the center of the plasma, respectively (figure 3). The simulation parameters include the following values: ${N}_{0}$ = 2.8×10¹⁹ ${\mathrm{m}}^{-3}$, ${N}_{1}$ = 2×10¹⁹ ${\mathrm{m}}^{-3}$, ${N}_{2}$ = 5×10¹⁷ ${\mathrm{m}}^{-3}$, ${a}_{1}$ = 0.27 m, ${a}_{2}$ = 0.07 m, ${a}_{3}$ = 0.03 m, ${B}_{0}$ = 2.5 T, ${R}_{0}$ = 1.75 m, ${ap}_{0}$ = 0.4 m.

Figure  3.  Density and magnetic field profiles above the antenna.

DownLoad: Full-Size Img PowerPoint

The plasma, considered a linear medium, allows the time-harmonic perturbation of the electromagnetic field to be represented as $\sim \mathrm{e}\mathrm{x}\mathrm{p}\left(\mathrm{j}{\omega }_{0}t\right)$. The excitation of the field in plasma is governed by the Maxwell equations [16], expressed for magnetized plasma:

where the dielectric tensor ε is the complex conjugate of usual cold dielectric tensor [30]. Since the effect of the magnetic field is all included in the dielectric tensor, the permeability is $\boldsymbol{\mu }={\mu }_{0}\boldsymbol{I}$.

In the coordinate system of the actual tokamak device, where the magnetic field direction does not align with the z-axis, the dielectric tensor ${\boldsymbol{\varepsilon }}'$ in any magnetic field direction can be obtained by applying a rotation transformation to the original magnetized medium tensor ε [31].

In this model, the rotation matrix is:

The simulation region is terminated by PML [12, 16]. A common form of PML stretching function [16] is:

where the variable “r” represents the spatial position in Cartesian coordinates. The subscript “u” represents the coordinate component. ${L}_{u}$, ${L}_{\mathrm{P}\mathrm{M}\mathrm{L}u}$, ${P}_{u}$, $S _u^\prime$, and $S _u^{\prime\prime}$ respectively represent the position of the medium/PML interface, the PML depth, the order of the stretching function, and the real and imaginary parts of the stretching [29]. In this study, $S _u^\prime$ = 1, $S _u^{\prime\prime}$ = 2, and ${P}_{u}$ = 2.

The field in the PML meets the modified Maxwell equations [16]:

where

Electric and magnetic fields in the PML are ${\boldsymbol{E}}_{\mathrm{P}\mathrm{M}\mathrm{L}}=\boldsymbol{S}\cdot\boldsymbol{E}$, ${\boldsymbol{H}}_{\mathrm{P}\mathrm{M}\mathrm{L}}=\boldsymbol{S}\cdot\boldsymbol{H}$, respectively.

2.4   Input impedance, reflection coefficient and antenna efficiency

Using impedance boundary conditions and the FEM, the port power ${P}_{\mathrm{p}\mathrm{o}\mathrm{r}\mathrm{t}\_i}$ (where ${P}_{\mathrm{p}\mathrm{o}\mathrm{r}\mathrm{t}\_i}=(1-{\left|{\varGamma }_{i}\right|}^{2}){P}_{\mathrm{f}\mathrm{w}\mathrm{d}\_i}$), the S-matrix ${S}_{ik}$, the port complex impedance ${Z}_{\mathrm{p}\mathrm{o}\mathrm{r}\mathrm{t}\_i}$ (where ${Z}_{\mathrm{p}\mathrm{o}\mathrm{r}\mathrm{t}\_i}={R}_{i}+\mathrm{j}{X}_{i}$), the port coupling impedance ${R}_{\mathrm{c}\_i}$ (where ${R}_{\mathrm{c}\_i}=(1-|{\varGamma }_{i}\left|\right)/ (1+|{\varGamma }_{i}\left|\right){Z}_{0}$), and the electric field distribution can be solved. Here, the indices i and k represent the circuit ports (i, k = 1, 2, 3, 4). In section 4, the forward wave power of each port is ${P}_{\mathrm{f}\mathrm{w}\mathrm{d}\_i}$ = 5.29 MW (i = 1, 2, 3, 4), and the power generated by one power source is equal to the port power ${P}_{\mathrm{p}\mathrm{o}\mathrm{r}\mathrm{t}\_i}$.

Then the reflection coefficient ${\varGamma }_{\mathrm{p}\mathrm{o}\mathrm{r}\mathrm{t}\_i}$ at port is expressed as follows:

where ${Z}_{0}$ = 50 Ω is the characteristic impedance of the transmission line.

The heat loss power at the antenna surface is expressed as follows:

where $\sigma$, ${E}_{\mathrm{t}}$, $\delta$ are respectively the electrical conductivity, surface electric field, and skin depth.

Based on the single absorption hypothesis [32], we believe that the power entering the plasma is completely absorbed by the plasma. The coupling power can be expressed as follows:

where the asterisk denotes the complex conjugate, S denotes the plasma surface.

The calculation plane for the antenna power spectral distribution is located at the interface between cold plasma and vacuum, and the calculation formula is:

where ${k}_{y'}$, ${k}_{z'}$, ${E}_{y'}$ and ${H}_{z'}$ represent the $y'$-axis components of the wave vector, the $z'$-axis components of the wave vector, the $y'$-axis component of the electric field, and the $z\mathrm{'}$-axis component of the magnetic field, respectively. The power spectrum of parallel wavenumber can be obtained through ${p}_{\mathrm{r}\mathrm{a}\mathrm{d}}$ integrated along ${k}_{y'}$. The power spectrum of parallel wavenumber is later referred to as the power spectrum.

The heat loss efficiency of the antenna is defined as follows:

and the coupling efficiency of the antenna is defined as follows:

3.   Relationships between S-matrix, reflection coefficients, port complex impedance and frequency

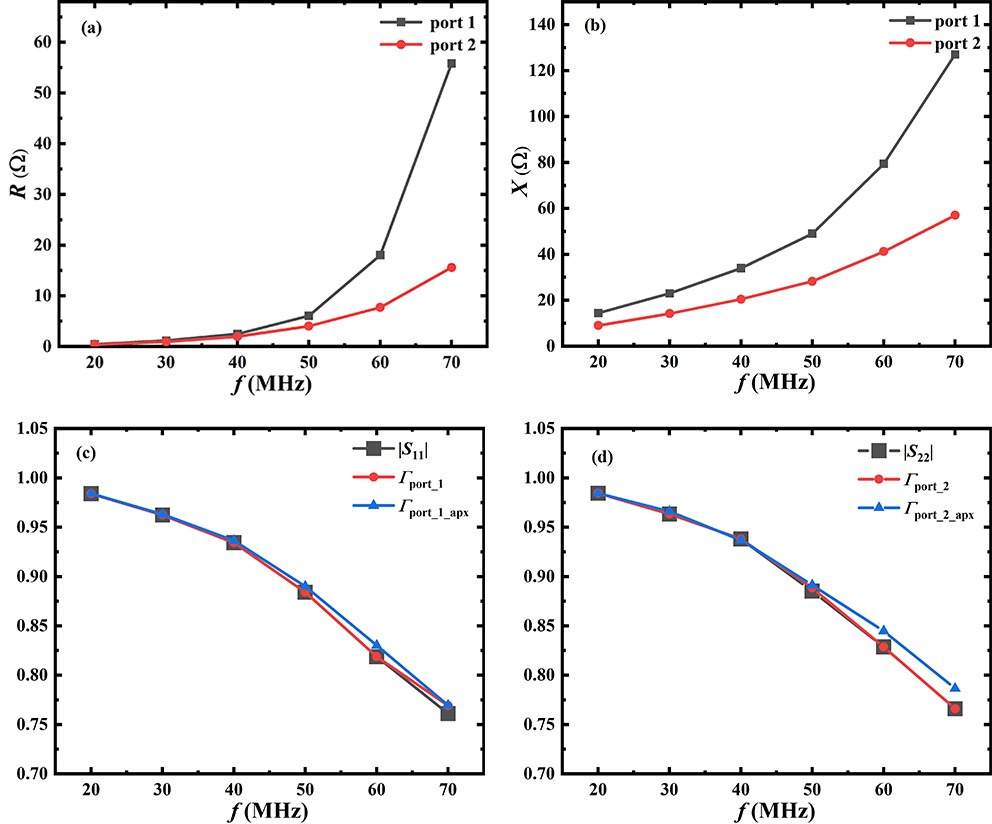
The frequency range of the EAST ICRH antenna straps is 25–70 MHz [33]. Due to the symmetry of the four-strap antenna (figure 2), it is only necessary to calculate the S-matrix of two ports (e.g. port 1 and port 2 in figure 2). It is noted that the direction of the magnetic field is not symmetrical, making little difference in the S parameters between ports 1 and 4, as well as ports 2 and 3. Figure 4 illustrates the relationship between the S-matrix of the four-strap antenna and frequency. $\left|{S}_{11}\right|$ is slightly smaller than $\left|{S}_{22}\right|$ when the frequency changes from 20 to 70 MHz (figure 4(a)). As the frequency increases, both $\left|{S}_{11}\right|$ and $\left|{S}_{22}\right|$ decrease significantly, indicating the less reflection power at high frequencies. The mutual coupling coefficients ($\left|{S}_{21}\right|$, $\left|{S}_{31}\right|$, $\left|{S}_{41}\right|$, $\left|{S}_{12}\right|$, $\left|{S}_{32}\right|$, $\left|{S}_{42}\right|$) between different ports are very small (figure 4(b)).

Figure  4.  The relationship between the S-matrix of the four-strap antenna and frequency. (a) $\left|{S}_{11}\right|$ and $\left|{S}_{22}\right|$, (b) $\left|{S}_{21}\right|$, $\left|{S}_{31}\right|$, $\left|{S}_{41}\right|$, $\left|{S}_{12}\right|$, $\left|{S}_{32}\right|$, and $\left|{S}_{42}\right|$.

DownLoad: Full-Size Img PowerPoint

Figure 5 shows the relationships between ${|\varGamma }_{\mathrm{p}\mathrm{o}\mathrm{r}\mathrm{t}\_i}|$, $\left|{S}_{ii}\right|$ (i = 1, 2), port complex impedance, and frequency at the current phase of (0, π, 0, π) on the CSs. When the coupling between ports is small and the forward wave voltage amplitude is the same for each port, the reflection coefficient of port i is approximately equal to $\left|{S}_{ii}\right|$ (figures 5(c) and (d)). Additionally, as the frequency increases, both ${|\varGamma }_{\mathrm{p}\mathrm{o}\mathrm{r}\mathrm{t}\_i}|$ (i = 1, 2) decrease significantly.

Figure  5.  The relationships between ${|\varGamma }_{\mathrm{p}\mathrm{o}\mathrm{r}\mathrm{t}\_i}|$, $\left|{S}_{ii}\right|$ (i = 1, 2), port complex impedance and frequency. (a) The real part of the port complex impedance ${R}_{i}$ (i = 1, 2), (b) the imaginary part of the port complex impedance ${X}_{i}$ (i = 1, 2) and (c) $\left|{S}_{11}\right|$, ${|\varGamma }_{\mathrm{p}\mathrm{o}\mathrm{r}\mathrm{t}\_1}$| and |${\varGamma }_{\mathrm{p}\mathrm{o}\mathrm{r}\mathrm{t}\_1\_\mathrm{a}\mathrm{p}\mathrm{x}}$|, and (d) $\left|{S}_{22}\right|$, ${|\varGamma }_{\mathrm{p}\mathrm{o}\mathrm{r}\mathrm{t}\_2}|$ and |${\varGamma }_{\mathrm{p}\mathrm{o}\mathrm{r}\mathrm{t}\_2\_\mathrm{a}\mathrm{p}\mathrm{x}}$|.

DownLoad: Full-Size Img PowerPoint

Since ports 1 and 2 are connected to different CSs, the impedances of ports 1 and 2 are significantly different. Figures 5(a) and (b) show that the real ${R}_{i}$ (i = 1, 2) and the imaginary ${X}_{i}$ (i = 1, 2) of port 1 and port 2 meet the condition (1) ${R}_{i}^{2}\ll {{X}}_{i}^{2}$ at 20–70 MHz. Substituting condition (1) into equation (18) results in an approximate equation (24). Figures 5(c) and (d) show that the change of ${|\varGamma }_{\mathrm{p}\mathrm{o}\mathrm{r}\mathrm{t}\_i\_\mathrm{a}\mathrm{p}\mathrm{x}}|$ is consistent with ${|\varGamma }_{\mathrm{p}\mathrm{o}\mathrm{r}\mathrm{t}\_i}|$ at 20–70 MHz.

It is logical to simplify equation (18) based on condition (1). First, this condition holds true for most ICRH antennas. Furthermore, in the design of the antenna structure, the real and imaginary parts of the port complex impedance are relatively independent. When using complex impedance, it is easy to overlook their independence from each other. Therefore, equation (24) is more intuitive and convenient in some cases.

4.   The effect of antenna fine structure on coupling characteristics

4.1   The effect of Faraday screen on antenna coupling characteristics

The FS is an essential component of the antenna and is located between the CSs and the plasma. Its primary purpose is to protect the antenna from plasma damage and filter out the slow wave component. Moreover, it reduces the intensity of the near-field electric field, preventing breakdown and suppressing the coaxial mode. Nevertheless, the FS also presents some drawbacks, including a decrease in magnetic coupling efficiency and an increase in thermal loss for the antenna [34, 35]. The optimized design of the FS can provide better coupling efficiency for the antenna, and the following sections 4.1.1–4.1.3 show the influence of the position and structure of FS on the coupling characteristics of the EAST four-strap antenna at a frequency of 40 MHz.

4.1.1   The effect of the angle on the complex impedance of the port

As shown in figure 2(a), $\theta$ represents the angle between the FS and the magnetic field. Figure 6 shows the relationship between the angle and the port complex impedance. The angle has little effect on the imaginary part of the port complex impedance ${X}_{i}$ (i = 1, 2) (figure 6(b)). As the angle increases from 0° to 15°, the real part of the port complex impedance ${R}_{i}$ (i = 1, 2) initially increases and then decreases (figure 6(a)). The larger ${R}_{i}$ (i = 1, 2), the stronger the coupling effect between the antenna and the plasma. ${R}_{i}$ (i = 1, 2) reaches its maximum when the FS is parallel to the direction of the magnetic field (angle equals 7°).

Figure  6.  The relationship between the angle of the FS with the magnetic field and the port impedance at f = 40 MHz. (a) Real part of the port complex impedance ${R}_{i}$ (i = 1, 2) and (b) imaginary part of the port complex impedance ${X}_{i}$ (i = 1, 2).

DownLoad: Full-Size Img PowerPoint

4.1.2   The effect of Faraday screen transparency on coupling power

The transparency of FS is defined as follows:

where r is the radius of the Faraday rod and ${X}_{\mathrm{r}\mathrm{o}\mathrm{d}}$ is the vertical distance between the axes of the two adjacent Faraday rods.

The higher the transparency, the lower the density of the FS. Figure 7 illustrates the relationships between coupling power, heat loss efficiency, and the transparency. The coupling power initially increases and then decreases with transparency. Figure 7 shows that the transparency of the FS for the EAST four-strap antenna is 0.6 at this plasma density to obtain higher coupling power. The change in coupling power could be attributed to the fact that FS with too large transparency cannot filter slow waves, which reduces the coupling power. On the other hand, FS with too small transparency prevents waves from entering the plasma. Additionally, as transparency decreases, the rate of heat loss rises rapidly (figure 7(b)), which may be the reason for the rapid decrease in coupling power after transparency is less than 0.4. To achieve high coupling power and reduce heat loss, the optimal design of the FS transparency needs to be considered. For the optimization of FS transparency, this is only a preliminary simulation result. More simulation and experimental data are needed to determine the best transparency of the FS.

Figure  7.  (a) The antenna coupling power changes with the transparency of the FS at f = 40 MHz and (b) the heat loss efficiency of the antenna changes with the transparency of the FS at f = 40 MHz.

DownLoad: Full-Size Img PowerPoint

4.1.3   The effect of the distance from the Faraday screen to the current straps on coupling power

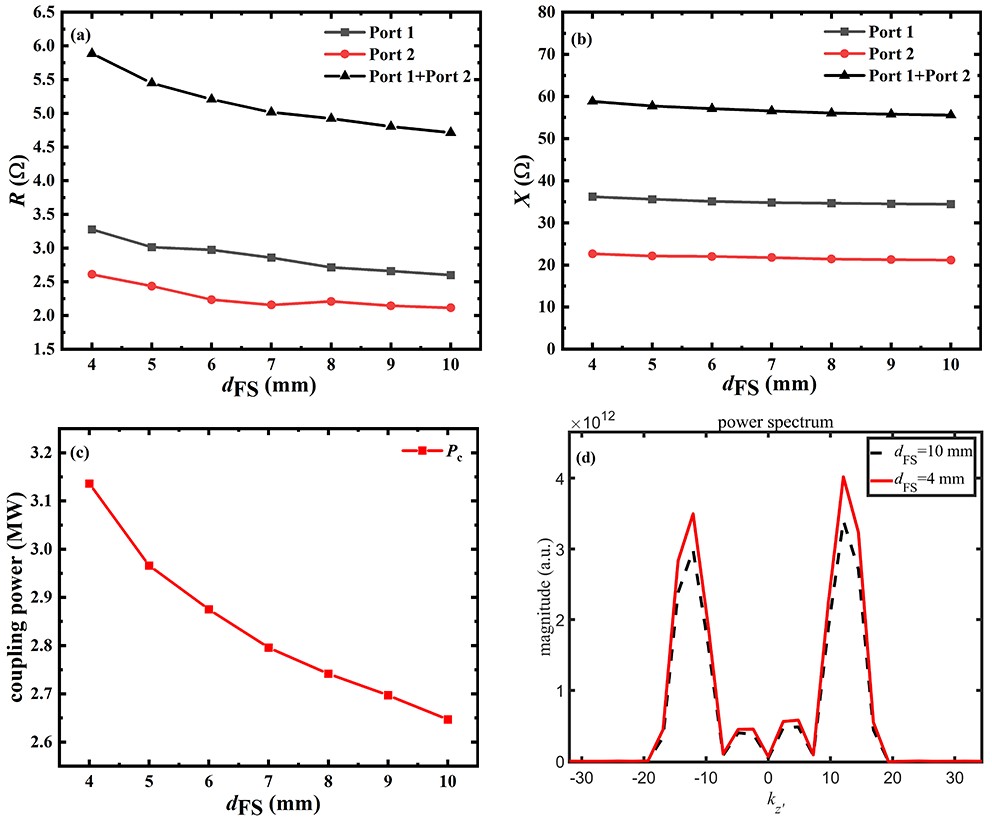
Maintaining a constant distance from the CSs to the plasma, figure 8 shows the relationships between the port complex impedance ${Z}_{i}={R}_{i}+\mathrm{j}{X}_{i}$, coupling power ${P}_{\mathrm{c}}$, radiation power spectrum ${p}_{\mathrm{r}\mathrm{a}\mathrm{d}}$, and the distance ${d}_{\mathrm{F}\mathrm{S}}$ from the FS to the CS. As the distance increases from 4 mm to 10 mm, the overall trend of ${R}_{i}$ (i = 1, 2) decreases, while the ${X}_{i}$ (i = 1, 2) changes little (figures 8(a) and (b)). Notably, as the distance increases from 6 mm to 8 mm, the values of ${R}_{1}$ and ${R}_{2}$ deviate from their original trend. However, the trend of the total resistance R of the two ports remains unchanged, possibly attributed to the change in mutual coupling effect between the ports. As ${d}_{\mathrm{F}\mathrm{S}}$ increases, the coupling power gradually decreases (figure 8(c)). Changing the distance ${d}_{\mathrm{F}\mathrm{S}}$ does not alter the position of the main peak $\ k_{z'\mathrm{_-m}\mathrm{a}\mathrm{i}\mathrm{n}}$ in the spectrum (figure 8(d)).

Figure  8.  The relationships between the port impedance, coupling power ${P}_{\mathrm{c}}$, radiation power spectrum ${p}_{\mathrm{r}\mathrm{a}\mathrm{d}}$ and the distance ${d}_{\mathrm{F}\mathrm{S}}$ at f = 40 MHz. (a) ${R}_{i}$ (i = 1, 2), (b) ${X}_{i}$ (i = 1, 2), (c) ${P}_{\mathrm{c}}$, and (d) $p_{\mathrm{r}\mathrm{a}\mathrm{d}}\left(k_{z'}\right)$.

DownLoad: Full-Size Img PowerPoint

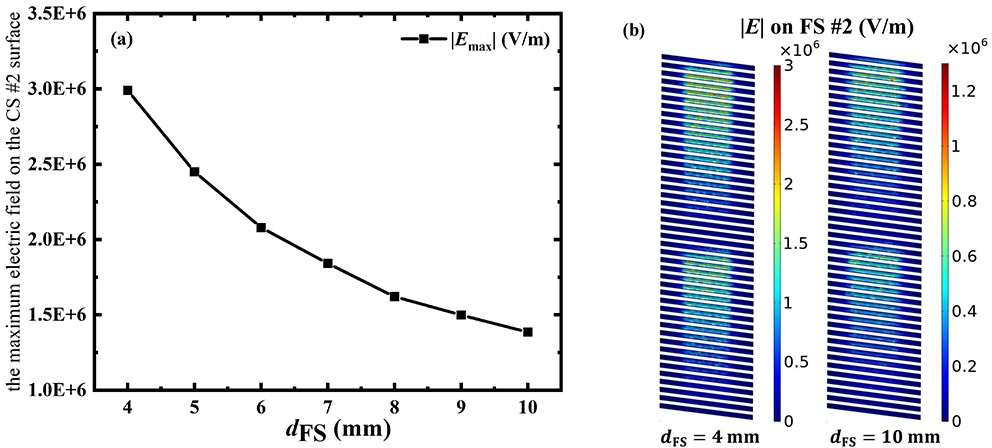
We further examined the impact of the distance ${d}_{\mathrm{F}\mathrm{S}}$ on the absolute value of the electric field $\left|E\right|$ on the FS and the CS. When ${d}_{\mathrm{F}\mathrm{S}}$ was reduced from 10 mm to 4 mm, the coupling power increased by approximately 20% (figure 8(c)). Additionally, the maximum electric field on the surface of the CS #2 increased by approximately 120% (figure 9(a)), and the magnitude of the electric field on the surface of the FS facing strap #2 increased by approximately 140% (figure 9(b)). In this study, the maximum breakdown electric field reference value of the FS is 3.5 MV/m, and the electric field of the FS does not exceed this limit. The distance between the FS and the CS can be set to 4 mm.

Figure  9.  The relationship between the absolute value of the electric field $\left|E\right|$ with ${d}_{\mathrm{F}\mathrm{S}}$ at f = 40 MHz. (a) The maximum electric field on the surface of the radiated the CS #2 and (b) the electric field distribution on side surface of FS facing strap #2 at ${d}_{\mathrm{F}\mathrm{S}}$ = 10 mm and ${d}_{\mathrm{F}\mathrm{S}}$ = 4 mm.

DownLoad: Full-Size Img PowerPoint

The closer the FS is to the CS, the stronger the Faulconer shielding effect of the FS becomes. This results in a decrease in the parallel components of the electric field (in the $z'$-axis direction) and an increase in the vertical components of the electric field radiated by the antenna [34]. The larger vertical electric field excites a larger fast wave within the plasma. This may be the reason for the increased power coupling between the antenna and the plasma.

4.2   The effect of the relative position of the port and the characteristic impedance of the transmission line on antenna coupling characteristics

4.2.1   The effect of the relative position of the port on coupling power and radiation power

${d}_{\mathrm{p}\mathrm{o}\mathrm{r}\mathrm{t}\_1\_2}$ represents the distance between port 1 and port 2 (figure 2(d)). Figure 10 illustrates the relationships between the coupling power ${P}_{\mathrm{c}}$, the absolute value distribution of the electric field $\left|E\right|$ of the CS #2, and the distance ${d}_{\mathrm{p}\mathrm{o}\mathrm{r}\mathrm{t}\_1\_2}$ at f = 40 MHz. The coupling power ${P}_{\mathrm{c}}$ of four-strap increases with the distance ${d}_{\mathrm{p}\mathrm{o}\mathrm{r}\mathrm{t}\_1\_2}$ (figure 10(a)). The electric field of the upper antenna radiation strap #2 increases with the increase of ${d}_{\mathrm{p}\mathrm{o}\mathrm{r}\mathrm{t}\_1\_2}$, whereas the lower antenna radiation strap #2 decreases (figure 10(b)).

Figure  10.  The relationships between the coupling power ${P}_{\mathrm{c}}$, the surface current distribution of the CS, and the distance ${d}_{\mathrm{p}\mathrm{o}\mathrm{r}\mathrm{t}\_1\_2}$ at f = 40 MHz. (a) Coupling power ${P}_{\mathrm{c}}$ and (b) the surface electric field of the CS #2 in the y-axis direction.

DownLoad: Full-Size Img PowerPoint

The reason for this may be that as the relative distance between ports 1 and 2 increases, the circuit length from port 2 to the upper radiant strap #2 decreases, while the length to the lower radiant strap #2 increases. Due to the influence of capacitance and inductance distributed in the antenna, the electric field is attenuated along the circuit from the port. Consequently, the longer the circuit length, the smaller the electric field on the radiated current strap. This results in the increase of electric field in the upper strap and the reduction in the lower radiation strap #2 (while strap #1 exhibits an opposite trend to strap #2). The increase in electric field in the upper radiation strap surpasses the reduction in the lower radiation strap (figure 10(b)). Under the conditions of the maximum electric field that the FS and straps can tolerate, in order to achieve high coupling power, it is necessary to maximize the distance between ports placed in the poloidal direction.

4.2.2   Characteristic impedance of the transmission line

The ICRH antenna typically employs a 50 Ω transmission line for connectivity. From the previous results, it is evident that the impedances of ports 1 and 2 differ (figures 6 and 8), and the impedance of the transmission line does not match the port impedance. The coupling performance of the EAST four-strap antenna will be studied using transmission lines with various characteristic impedance values. The analysis of the impact on the power spectrum of the EAST four-strap antenna has been carried out. In this study, the characteristic impedance of the transmission line is changed by manipulating the radius of the inner conductor. Figures 11(a)–(c) show the power spectrum with the current phases of (0, π, 0, π), (0, 0, π, π), and (0, π, π, 0) at the frequency of 40 MHz, respectively. The simulations demonstrate that the position of the main peak ${k}_{z\_\mathrm{m}\mathrm{a}\mathrm{i}\mathrm{n}}$ in the spectrum remains relatively stable when ${Z}_{0}$ varies from 15 Ω to 75 Ω. In addition, the four-strap antenna with ${Z}_{0}$ = 25 Ω exhibits a higher level of radiation power compared to the other cases. The reason is that when port complex impedance meets ${R}_{i}\ll {X}_{i}$, the port reflection coefficient has a minimum value if the characteristic impedance ${Z}_{0}$ is approximately equal to ${X}_{i}$, as obtained in equation (24). The characteristic impedance of 25 Ω is close to the average value of the imaginary part of the port complex impedance at f = 40 MHz. At a frequency of 40 MHz, the imaginary part of the impedance of ports 1 and 4 is about 35 Ω, while the imaginary part of the impedance of ports 2 and 3 is about 20 Ω. At the current phase of (0, π, π, 0), the four-strap antenna with ${Z}_{0\_1\_4}$ = 35 Ω transmission lines for ports 1 and 4, and ${Z}_{0\_2\_3}$ = 20 Ω transmission lines for ports 2 and 3, results in increased radiation power compared to the antenna with ${Z}_{0}$ = 25 Ω transmission lines for all four ports (figure 11(d)). This shows that the antenna with the coaxial line ${Z}_{0}$ ≈ ${X}_{i}$ under the condition of ${R}_{i}\ll {X}_{i}$ can effectively mitigate reflections and enhance the radiated power of the antenna.

Figure  11.  The relationships between the characteristic impedances of transmission lines, the phase difference of four strap, and the antenna radiation power spectrum at f = 40 MHz. (a) Four ports with the same transmission lines, the current phase of (0, π, 0, π), (b) four ports with the same transmission lines, the current phase of (0, 0, π, π), (c) four ports with the same transmission lines, the current phase of (0, π, π, 0), and (d) comparison of four ports with the transmission lines of ${Z}_{0}$ = 25 Ω (red solid line) and ports 1 and 4 with transmission lines of ${Z}_{0\_1\_4}$ = 35 Ω, ports 2 and 3 with transmission lines of ${Z}_{0\_2\_3}$ = 20 Ω (black dashed line) for the current phase of (0, π, π, 0).

DownLoad: Full-Size Img PowerPoint

5.   3D antenna coupling with impedance matching system

This section outlines the procedure for simulating the 3D EAST four-strap antenna with a double-stub ferrite impedance matching system. This method allows for the simulation of RF wave propagation from the transmitter to the plasma, the determination of optimized impedance matching settings, and the prediction of the distribution of standing wave voltage peaks along the ICRH antenna system.

The schematic diagram of the double-stub ferrite tuner impedance matching system with antenna heating plasma is shown in figure 12. The distance between the power source and the antenna is L, and the distance from the power source to point 1 and point 2 is ${L}_{\mathrm{p}1}$ and ${L}_{\mathrm{p}2}$, respectively. The FT has short circuit terminal structures. The space of the transmission line is filled with yttrium iron garnet (YIG) material. The length of the transmission line and ferrite line elements remains constant, represented by ${L}_{\mathrm{V}k}$ and ${L}_{\mathrm{f}k}$, respectively, where the indices k denote ferrite stub (k = 1, 2). V₁ and I₁ represent the voltage and current flowing from the power source to point 1. V_A and I_A represent the voltage and current propagating from point 2 to the antenna. The relative permeability of the YIG ferromagnetic material can be obtained using the following formula: ${\mu }_{\mathrm{r}}={\mu }_{\mathrm{r}\mathrm{e}\mathrm{a}\mathrm{l}}+\mathrm{j}{\mu }_{\mathrm{i}\mathrm{m}\mathrm{a}\mathrm{g}}$. ${\mu }_{\mathrm{r}\mathrm{e}\mathrm{a}\mathrm{l}}$ is a function of the biased magnetic field H and the saturation magnetization strength ${M}_{\mathrm{s}}$, written as ${\mu }_{\mathrm{r}\mathrm{e}\mathrm{a}\mathrm{l}}=1+{M}_{\mathrm{s}}/H$, and ${M}_{\mathrm{s}}$ is taken as 1200 kA/m in this chapter. The imaginary part of the relative permeability ${\mu }_{\mathrm{i}\mathrm{m}\mathrm{a}\mathrm{g}}$ of ferrite is related to the loss of ferrite. When the applied bias magnetic field $H$ is in the range of 80–150 kA/m, the value of ${\mu }_{\mathrm{i}\mathrm{m}\mathrm{a}\mathrm{g}}$ is close to 0 [36]. The impedance of the ferrite stub is defined as follows [37]:

Figure  12.  The schematic diagram of a double-stub ferrite tuner impedance matching system with antenna heating.

DownLoad: Full-Size Img PowerPoint

where $\beta$ and ${Z}_{0}$ are the propagation coefficient and characteristic impedance of the VTL, respectively.

The ICACIMSFT program involves a comprehensive model with four double-stub FT, four-strap antenna, and plasma, built using COMSOL software. Based on the given parameter, it can achieve impedance matching with a reflection coefficient of no more than 1% for each power source. The simulation parameters include the following values: ${L}_{\mathrm{p}1}$ = 10 m, ${L}_{\mathrm{p}2}$ = 20 m, L = 35 m, f = 40 MHz, ${L}_{\mathrm{V}i}$ = 1 m, ${L}_{\mathrm{f}i}$ = 2.1 m (i = 1, 2), and the current phase of (0, π, 0, π).

Based on the optimized results in section 4, we simulate the effect of optimization of FS and port structure on the voltage distribution of the main transmission line and the maximum coupling power under the condition of impedance matching. Other parameters remain fixed. We compare two cases, case 1 uses the reference parameters: [the transparency of the FS, ${d}_{\mathrm{F}\mathrm{S}}$, ${d}_{\mathrm{p}\mathrm{o}\mathrm{r}\mathrm{t}\_1\_2}$, ${Z}_{0}$] = [0.43, 10 mm, 38 cm, 50 Ω], while case 2 uses the optimized parameters of the FS and port structure: [the transparency of the FS, ${d}_{\mathrm{F}\mathrm{S}}$, ${d}_{\mathrm{p}\mathrm{o}\mathrm{r}\mathrm{t}\_1\_2}$, ${Z}_{0}$] = [0.6, 4 mm, 50 cm, 25 Ω]. The data for the reference model comes from the EAST four-strap ICRH antenna. The data for the optimized model comes from the simulation results in section 4. Figure 13 shows the voltage and current distributions of transmission lines i (i = 1, 2) under the condition of impedance matching for case 1 and case 2, respectively. The power of the source in case 1 is limited by the condition that the maximum voltage of the transmission line with a characteristic impedance of 50 Ω cannot exceed 45 kV. Under these conditions, the voltage and current distributions of case 1 on the main transmission line are shown in figures 13(a) and (b), and the maximum current on the main transmission line is about 850 A. The total port power $\sum {P}_{\mathrm{p}\mathrm{o}\mathrm{r}\mathrm{t}\_i}$ (i = 1, 2, 3, 4) of the antenna system is approximately 2.77 MW, and the coupling power ${P}_{\mathrm{c}}$ is around 2.65 MW with impedance matching. For case 2, the maximum limiting voltage of a transmission line with a characteristic impedance of 25 Ω is set at 34 kV. The voltage and current distributions of case 2 on the transmission line are depicted in figures 13(c) and (d), and the maximum current on the main transmission line is about 1350 A, which is about 1.6 times the maximum current in case 1. The antenna system can approximately obtain 5.26 MW total port power $\sum {P}_{\mathrm{p}\mathrm{o}\mathrm{r}\mathrm{t}\_i}$ (i = 1, 2, 3, 4) and 5.1 MW coupled power ${P}_{\mathrm{c}}$ under the condition of impedance matching, which almost doubles the coupling power of case 1. In addition, in figure 13, the VTL2 of case 2 has higher voltage and current values between x = −25 m and −15 m than the corresponding values of VTL2 of case 1 because of the difference in antenna impedance and the bias magnetic field of double-stub. The underlying causes of this phenomenon require further research.

Figure  13.  The voltage and current distributions of the ICRH antenna system when double-stub ferrite tuners get impedance matching. (a) VTL 1 of case 1, (b) VTL 2 of case 1, (c) VTL 1 of case 2 and (d) VTL 2 of case 2.

DownLoad: Full-Size Img PowerPoint

Figure 14 shows the electric field distribution and the voltage distribution of the optimized antenna model at impedance matching. This demonstrates that the program can accurately predict the size of the voltage peak and the location where the breakdown of the electric field may occur. By modeling a realistic transmission system, instead of assuming a long straight lines, the calculation results can pinpoint the specific location where the antenna system may break down, proving more useful for predicting the overall performance of the ion cyclotron resonance heating antenna.

Figure  14.  The distributions of voltage and electric field ${E}_{y}$ of the optimized ICRH antenna system at impedance matching at the current phase of (0, π, 0, π).

DownLoad: Full-Size Img PowerPoint

6.   Conclusions

A program based on COMSOL has been developed to integrate the EAST four-strap antenna coupling with plasma and the double-stub FT impedance matching. This program enables the prediction of various parameters, including port complex impedance, port S-matrix, port reflection coefficients, 3D electric field distribution, heat loss of the antenna, the coupling power to the plasma, and optimal matching settings for the double-stub FT.

In this study, we first employ it to discuss the relationships between the S-matrix, reflection coefficients, port complex impedance, and frequency for the EAST four-strap antenna. As the frequency increases from 20 to 70 MHz, ${|\varGamma }_{\mathrm{p}\mathrm{o}\mathrm{r}\mathrm{t}\_i}|$ and $\left|{S}_{ii}\right|$ (i = 1, 2, 3, 4) decreases, indicating that more power is fed into the antenna. Due to the minimal mutual coupling among the four ports, ${|\varGamma }_{\mathrm{p}\mathrm{o}\mathrm{r}\mathrm{t}\_i}|$ and $\left|{S}_{ii}\right|$ are approximately equal.

Then, we discuss the impact of the angle between the FS and the magnetic field, the FS transparency, the distance between the FS and the CS, the relative distance between ports, and the characteristic impedance of the transmission line on the coupling characteristics of the EAST four-strap antenna at a frequency of 40 MHz and a given plasma density and magnetic field profile for the current phase of (0, π, 0, π):

(1) When the FS is aligned parallel to the magnetic field, with an angle of 7°, and the transparency equals to 0.6, the four-strap antenna can achieve high coupling power and minimize heat loss.

(2) If the distance between the CS and the plasma remains fixed, adjusting the distance $\mathit{d}_{\mathrm{FS}}$ between the FS and the CS from 10 mm to 4 mm leaves the position of the main peak $\mathit{k}_{\mathit{z_-\mathrm{main}}\mathbf{\mathbf{\ \mathbf{\mathbf{ }}\mathbf{ }}}}$ in the power spectrum unchanged, and the coupling power increases.

(3) Under the condition that the electric field of the FS does not exceed the maximum electric field limit, increasing the distance in the polarization direction between the ports can result in higher coupling power.

(4) If the resistance of antenna ports is much less than reactance, connecting the coaxial line with its characteristic impedance approximately equal to reactance allows the antenna to achieve high radiation power.

Finally, the effect of the FS and port structure on the voltage and current distributions of the transmission line and the maximum coupling power under the condition of impedance matching is discussed. The simulations show that with optimal parameters the coupling power roughly doubles. The related results provide a certain guidance for the future design of high-power long-pulse operation ICRH antenna systems.