Central-cell injection of compact toroid in KMAX

1.   Introduction

In a magnetic mirror, the initial plasma is typically generated by plasma guns positioned at one or both ends, allowing only particles with sufficiently high axial speeds to travel through the magnetic mirror and enter the central cell [1, 2]. Many plasma particles may not pass through the mirror, making it inefficient to populate the central cell completely. For applications in fusion or as a neutron source [3], a magnetic mirror would need to be extended to achieve energy break-even [4], increasing plasma loss through recombination or other atomic processes may discourage such injection methods. Given the renewed interest in magnetic mirror fusion [4] and the establishment of several new mirror experiments [5], a more efficient injection system is required.

Injecting plasma from the axial end into the central cell complicates the measurement of the axial loss rate, which is crucial for understanding mirror confinement [6]. However, the axial confinement time can be inferred from radial confinement measurements, given knowledge of the total confinement time. Due to axial non-uniformity in linear devices, accurately estimating axial loss would require multiple radial loss measurements, which is both challenging and potentially inaccurate. A direct measurement of the axial loss rate is desirable, but particles lost from the central cell in the axial direction mix with counter-traveling particles from the end cells, complicating identification. While it is possible to distinguish between these two particle populations, a more effective way to assess the axial loss rate would be to eliminate plasma injection from the ends. Injecting plasma directly into the central cell would thus help enhance our understanding of mirror confinement.

Compact toroid (CT) [7] injection has previously been used as a refuelling method in tokamak devices [8] and in the C-2U device [9], a field-reversed configuration with a geometry similar to that of a magnetic mirror.

For a CT to penetrate the magnetic field, it must satisfy the condition that its kinetic energy exceeds the magnetic energy, represented by the equation: $\rho_{\text{CT}}v_{\text{CT}}^2/2\text{ } > \text{ }B^2/\left(2\mu_0\right)$, where ${\rho _{{\mathrm{CT}}}}$ denotes the CT’s mass density, ${v_{{\text{CT}}}}$ the velocity, and B the magnetic field intensity at the intended penetration point. This requirement guides the design of the central-cell injection experiment, which is conducted on the axisymmetric tandem mirror device, KMAX (Keda Mirror with AXisymmetricity). A magnetized coaxial plasma gun (MCPG) is installed to generate a single compact toroid. The remainder of this paper is organized as follows: section 2 provides an overview of the MCPG and its diagnostics; section 3 presents and analyzes the experimental results; section 4 summarizes our findings.

2.   Design of the MCPG and diagnostics

2.1   MCPG

Figure 1 shows the MCPG designed for the KMAX, featuring a compact, coaxial configuration with inner and outer electrodes, extension electrode, two gas ports, and two isolators.

Figure  1.  Cross-sectional view of the MCPG.

DownLoad: Full-Size Img PowerPoint

The two electrodes are made of stainless steel (304L). The inner electrode is coated with tungsten, with a thickness of 0.3±0.1 $\mu\mathrm{m}$. The outer electrode features two gas inlets positioned 180 degrees apart to improve gas distribution uniformity. A ceramic break separates the two electrodes.

The inner electrode has a length of 417 mm and an outer diameter of 54 mm, while the outer electrode has a length of 220 mm and an inner diameter of 84 mm. The inner electrode is hollow to house a solenoid, which generates the poloidal magnetic flux of the CT [10]. The solenoid consists of 140 turns in two layers, using 1 mm enamelled wire, and is wrapped in polyamide for high-voltage insulation. The coil has an inductance of 24 $\mu\mathrm{H}$ and a resistance of 0.9 Ω.

The inner electrode’s wall thickness is 3 mm, resulting in an estimated magnetic penetration time of approximately 12 $\mu\mathrm{s}$, calculated from the magnetic diffusion equation: $\tau \sim \mu {{\delta ^2}}/ \eta$ , where $\mu$ is the magnetic permeability, δ is the thickness of the inner electrode, and η is the resistivity of stainless steel. This penetration time is significantly shorter than the bias circuit’s discharge time of about 1 ms, as shown in figure 2.

Figure  2.  Discharge current of the bias system.

DownLoad: Full-Size Img PowerPoint

The solenoid within the inner electrode connects to a 5 mF capacitor bank to provide the initial biased poloidal flux. The typical operating voltage is 110 V, generating a maximum magnetic field of approximately 0.4 T.

The main pulsed power system, connected to the outer and inner electrodes, consists of a 5 $\mu\mathrm{F}$ pulse capacitor, a 30 kV power supply, and a hydrogen thyratron switch. Figure 3(a) shows the circuit, where ${{{R}}_1}$ and ${{{L}}_1}$ represent the resistor and inductor of the feedline, with values of 40 mΩ and 430 nH, respectively. $R\mathrm{_p}$ and $L\mathrm{_p}$ correspond to the plasma’s equivalent resistance and inductance, with typical values of 20 mΩ and 70 nH, respectively. These values are estimated by comparing simulated waveforms (figure 3(b)) with the measured waveform (figure 3(c)).

Figure  3.  (a) Schematic of the charge circuit and its primary discharge, (b) simulation results of the primary discharge at 20 kV, (c) real discharge waveforms of current and voltage at 20 kV.

DownLoad: Full-Size Img PowerPoint

The maximum current amplitude is approximately 50 kA at a voltage of 20 kV, with a period of about 10 μs. This period is much shorter than the flat-top period of the bias field, which lasts around 0.25 ms (see figure 2). Therefore, the bias field is quasi-static during the main bank discharge. Table 1 shows test results of such gun for different voltages when injecting into KMAX vacuum.

Table  1.  List of the base characteristics of the central-cell injection plasma generated by MCPG under different voltages. “-” indicates no data available for this condition.

Voltage (kV)	Current (kA)	Line-averaged density (×1017 m−2)	Velocity (km/s)
8	24	0.45	-
14	42	3.2	4.0
18	49	9.6	5.8
20	51	-	14.8

 | Show Table

DownLoad: CSV

The primary goal with this radial plasma injection is to increase the plasma density. A fast solenoid valve [11] is applied to minimize neutral gas leakage into the central cell. Figure 4 shows its performance. The valve is sealed with an aluminium plate and an O-ring. A solenoid coil positioned directly opposite to the aluminium plate generates an upward force caused by Lenz’s law when voltage is applied, pushing the plate to complete the gas puffing process. Figure 4(a) shows the test results, displaying the measured pressure as a function of the applied voltage. Figure 4(b) presents the number of gas molecules per injection, calculated from the equilibrium pressure and the device’s volume.

Figure  4.  (a) Ionization gauge pressure signal at different voltages, (b) number of particles injected into the device at different voltages.

DownLoad: Full-Size Img PowerPoint

In the experiment, a voltage of 400 V is typically applied, enabling the injection of approximately 5×10¹⁹ gas particles per operation.

2.2   The KMAX device and diagnostics

As shown in figure 5(a), KMAX consists of a central cell and two end cells. The central cell is 5.2 m in length and 1.22 m in diameter, the ambient pressure is ~ 2×10⁻⁴ Pa during experiments. For the central-cell injection experiments, the magnetic field generated by the supercapacitor bank is typically 260 Gauss in the central cell, which is shown in figure 5(b).

Figure  5.  (a) Schematic of the KMAX device and diagnostics, (b) background magnetic field strength.

DownLoad: Full-Size Img PowerPoint

The location and arrangement of MCPG and high-speed camera are shown in figure 6. The MCPG is at Z = 0 m. Location and arrangement of diagnostics used in this experiment can be found in figure 5(a). For instance, the interferometer is located at Z = −0.27 m with an impact parameter of 0.07 m below and perpendicular to the axis of the device. The Mid-APD diagnostics has a collection lens at Z = 0 m focusing on the axis of the device with APD (Thorlabs APD410A2, where APD represents Avalanche PhotoDiode) as the detector, which is sensitive to the light in the 200–1000 nm wavelength range. Note that in this experiment, no filter was used. By analyzing the arrival time of the APD signal peak, the moment the plasma reaches the axis can be determined, enabling the estimation of its velocity and confirmation of successful plasma penetration. The South-APD and Mirror-APD detectors, situated at Z = −0.75 m and −3.23 m, respectively, observe plasma evolution after injection. A diamagnetic loop at Z = +0.33 m mounted perpendicular to the axis reveals the plasma energy injected to the vacuum chamber.

Figure  6.  (a) Mounted position of the central-cell injection system, (b) camera setup and line of sight.

DownLoad: Full-Size Img PowerPoint

3.   Experimental results and analysis

3.1   Typical injection results

In the experiment, the supplied voltage of the MCPG’s main bank is fixed at 8 kV, with the trigger time for the main switch set to t = 0 ms. The plasma’s kinetic energy is insufficient to penetrate the magnetic field at this voltage. Figure 7(a) shows an image of the initial discharge taken by a high-speed camera, while figure 7(b) shows a photograph taken from the side of the MCPG’s port. Figure 7(a) suggests that the formed plasma ring is relatively uniform due to possibly the symmetrical arrangement of the gas injection from two opposite and tangential gas ports, however, figure 7(b) shows that the plasma remains close to the gun muzzle and does not penetrate the middle of the chamber in this case.

Figure  7.  (a) Image of the discharge at 8 kV (front view), (b) image of the discharge at 8 kV (side view).

DownLoad: Full-Size Img PowerPoint

Confirmed by optical measurements, see figure 8, the Mid-APD located at Z = 0 m and aimed at the chamber axis, does not detect significant signals, even when the background magnetic field strength is 65 Gauss. The signal is even weaker at higher field strengths, such as 600 Gauss. Interestingly, the tails of the signals look similar in each case, which may indicate that this is due to a diffusion process. Overall, the results are consistent with expectations.

Figure  8.  Mid-APD signals under different background magnetic fields, with a discharge voltage of 8 kV.

DownLoad: Full-Size Img PowerPoint

When the voltage is increased to 14 kV and 18.8 kV, visible plasma emerges from the MCPG, as shown in figures 9(a) and (c). Figures 9(b) and (d) present the Mid-APD signals for 14 kV and 18.8 kV, respectively, where both shots exhibit double peaks during the discharge. Notably, in the 18.8 kV case, the Mid-APD signal actually exhibits three peaks occurring at approximately 10 μs (1st), 104 μs (2nd), and 117 μs (3rd), respectively. Based on camera footage and Mid-APD signals observed at lower voltages, the first peak is interpreted as the light from the arc and reflected light signal during the initial phase of the discharge, as the risetime of the first peak closely follows the trigger time which has an agreement with the cases of 8 kV and 14 kV. The second peak is attributed to the main plasma reaching the device’s axis. For the 18.8 kV case, the third peak is possibly attributed to the slower remain plasma caused by the blow-by effect [12–14]. In the MCPG, the unbalanced Lorentz force profile exerted on the plasma might lead to a radial spread in the axial velocity of the plasma. Such radial profile in the axial velocity may result in that the faster moving plasma could overtake the slower moving plasma. This phenomenon can be observed obviously from the APD signal at higher voltage 20 kV which is shown in figure 10. Furthermore, the travel times from the MCPG muzzle to the axis under different voltages are approximately 150 μs, 104 μs, 40 μs, yielding a velocity of ~ 4.0 km/s, 5.8 km/s, 14.8 km/s, respectively.

Figure  9.  (a) Image of the discharge at 14 kV (side view), (b) intensity of Mid-APD at 14 kV, (c) image of the discharge at 18.8 kV (side view), (d) intensity of Mid-APD at 18.8 kV.

DownLoad: Full-Size Img PowerPoint

Figure  10.  Intensity of Mid-APD at 20 kV.

DownLoad: Full-Size Img PowerPoint

In the 14 kV case, note that the plasma blob travels only a limited distance in the radial direction before either remaining or oscillating near the exit of the MCPG port. This 14 kV condition may correspond to the threshold at which the plasma gains enough energy to penetrate the magnetic field.

Upon the bank voltage fixed to 20 kV, compared to the case of the 14 kV, the speed of central-cell plasma increases with the bank energy, the Mid-APD’s signal amplitude has increased ~ 30 times. The results suggest that the operation condition must remain above 14 kV or higher to have a decent plasma in the central cell.

Figure 11 presents the line-averaged density in the central cell, as measured by the interferometer located at Z = −0.27 m under voltages of 14 kV and 18.8 kV. The line-averaged densities are measured to be 3.2×10¹⁷ m⁻² and 9.6×10¹⁷ m⁻² for 14 kV and 18.8 kV, respectively. The peak density is observed earlier than the APD signal due to the plasma undergoing an expansion process after exiting the gun muzzle. This expansion allows the interferometer, which measures along the line of sight, to detect the density earlier.

Figure  11.  Line-averaged density of the radial injection measured by the interferometer: (a) 14 kV, (b) 18.8 kV.

DownLoad: Full-Size Img PowerPoint

3.2   Central plasma interaction with background plasma

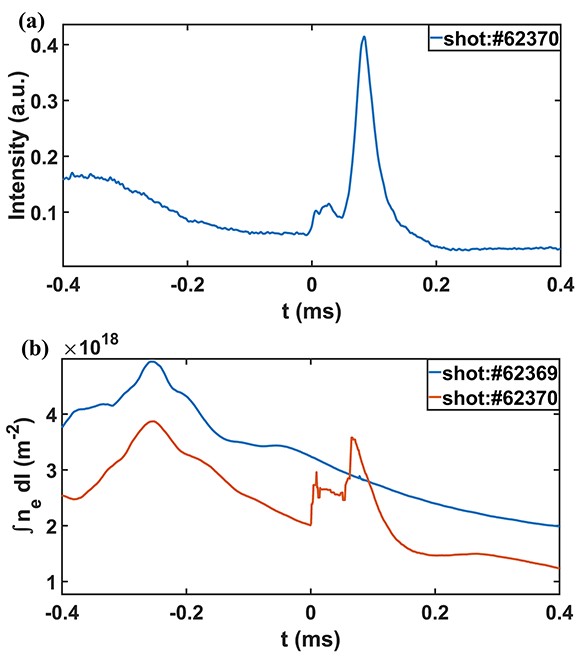
Since only a single directional side is available on KMAX, its filling effect can be partially observed. Before installing additional central-cell injectors, it may be insightful to investigate the interaction with background plasma. Figure 12(a) presents the diamagnetic signal of the central-cell injected plasma, while figure 12(b) shows the diamagnetic signal for a standard KMAX mirror experiment setup, where the plasma is produced by washer gun located at one end. Typically, the pulse width of the actual discharge lasts approximately 1.2 ms and the density is in the range of 10¹⁸ m⁻³. Figure 12(c) displays the result of central plasma injection into the mirror plasma. In this experiment, t = 0 ms defines the moment when the MCPG is triggered with a bank voltage of 14 kV, and the washer gun fires at t = −1.6 ms to ensure that the central plasma is refuelled at the tail end of the background plasma. In figure 12(c), the MCPG is fired into the mirror plasma, resulting in a spike at t = +0.09 ms. The signals are measured using a diamagnetic loop at Z = +0.33 m. Mid-APD signal is shown in figure 13(a), the spike can be clearly observed after MCPG fired.

Figure  12.  (a) Loop signal of central-cell injection without background plasma (at 14 kV), (b) loop signal of background plasma without central-cell injection but thyratron was triggered, (c) loop signal of background plasma with central-cell injection (at 14 kV).

DownLoad: Full-Size Img PowerPoint

Figure  13.  (a) Mid-APD signal of background plasma with central-cell injection, (b) line-averaged density. The blue curve represents the line-averaged density of background plasma without central-cell injection, while the red case is the line-averaged density of background plasma with central-cell injection.

DownLoad: Full-Size Img PowerPoint

The line-averaged density increase is also observed through interferometer measurements. As shown in figure 13(b), The blue curve represents the line-averaged density of background plasma without central-cell injection, while the red case is the refuelling scenario. Since the experiment is performed under the tandem mirror configuration instead of the cusp configuration, the line-averaged density of background plasma has a difference between the two shots due to the flute instability which is consistent with our previous measurement [15, 16]. Notably, not only the diamagnetic flux but also the line-averaged density increases surpass the diagnostic values for a single central-cell injection plasma. This is due to the interactions between the injected central plasma and the background plasma, such as collisions, which enhance plasma retention and facilitate capture by the magnetic mirror.

There is an increase in internal energy with central plasma injection. Researchers have used CT as a refuelling technique and have observed density increases in various experiments [17, 18]. Thus, the increase in diamagnetism is primarily attributed to the rise in density according to our refuelling experiment, although we also expect a temperature increase, as the kinetic energy of the central plasma may transfer to thermal energy during its interaction with the background plasma. Future experiments will require improved diagnostics to better characterize the performance of this central-cell injection system.

4.   Summary

The experimental setup for the central-cell injection system has been constructed on the KMAX machine, including performance measurements. There is minimal plasma in the central cell until the voltage is raised from 8 kV to 14 kV. Upon further increasing the voltage to 20 kV, radial plasma injection becomes visible both on the high-speed camera and the APD. The peak current reaches approximately 50 kA, accelerating the plasma to ~ 14.8 km/s.

For the 14 kV case, it appears that the plasma cannot penetrate fully and instead falls to the edge, as observed in the camera footage, suggesting that 14 kV may be the threshold voltage for this injection method on the KMAX device.

In the existing experiment, there is only one MCPG on the KMAX, but the setup will be upgraded. The objective is to achieve a plasma density of ~ 10²⁰ m⁻³, substantially higher than in previous magnetic mirror experiments. The upgrade will include multiple MCPGs, and efforts will focus on eliminating trapped flux or potentially producing a plasma stream instead of a current-driven toroidal CT configuration.

In future work, multiple MCPGs are applied to enable head-on collisions in the central cell. In a counter-helicity collision, a field-reversed mirror could form [19, 20], although this is not the primary objective of the injection. Since CTs inevitably lose particles and flux, if the mirror can confine these particles for a sufficient duration, a stable mirror plasma could be established once the CT flux has decayed.