| Citation: | Yuxuan HUANG, Ming ZHANG, Yong YANG, Fangwei LYU, Xiaopeng YI, Chaofan LYU, Yisong ZHANG, Bo RAO. Design and experimental study of a field-reversed configuration plasma thruster prototype[J]. Plasma Science and Technology, 2025, 27(3): 035507. DOI: 10.1088/2058-6272/ada376 |
The field-reversed configuration (FRC) plasma thruster driven by rotating magnetic field (RMF), abbreviated as the RMF-FRC thruster, is a new type of electric propulsion technology that is expected to accelerate the deep space exploration. An experimental prototype, including diagnostic devices, was designed and constructed based on the principles of the RMF-FRC thruster, with an RMF frequency of 210 kHz and a maximum peak current of 2 kA. Under the rated operating conditions, the initial plasma density was measured to be 5 × 1017 m−3, and increased to 2.2 × 1019 m−3 after the action of RMF. The coupling efficiency of RMF was about 53%, and the plasma current reached 1.9 kA. The axial magnetic field changed in reverse by 155 Gauss, successfully reversing the bias magnetic field of 60 Gauss, which verifies the formation of FRC plasma. After optimization research, it was found that when the bias magnetic field is 100 Gauss, the axial magnetic field reverse variation caused by FRC is the highest at 164 Gauss. The experimental results are discussed and strategies are proposed to improve the performance of the prototype.
With the development of space technology, deep space exploration has become a hot topic of research in countries around the world. Deep space exploration has the characteristics of long operation distance, long work cycle, and complex mission process, which puts forward higher requirements for the performance of thrusters, and indicates that they should support spacecraft to reach the mission site in a shorter time and carry more loads to effectively achieve mission objectives [1, 2]. The commonly used thrusters mainly include chemical thrusters and conventional electric thrusters. Chemical thrusters carry a large amount of fuel, but have a low specific impulse and low efficiency, which cannot meet the needs of future deep space exploration missions. Conventional electric thrusters have higher specific impulse and efficiency than chemical thrusters, and are mainly used for spacecraft position maintenance, orbit correction, etc [3]. However, electrode erosion caused by plasma sputtering limits their application in long-duration propulsion tasks. Therefore, new electric propulsion technology with high specific impulse, high efficiency, and long service life is an urgent need for deep space exploration as one of the most critical technical supports [4, 5].
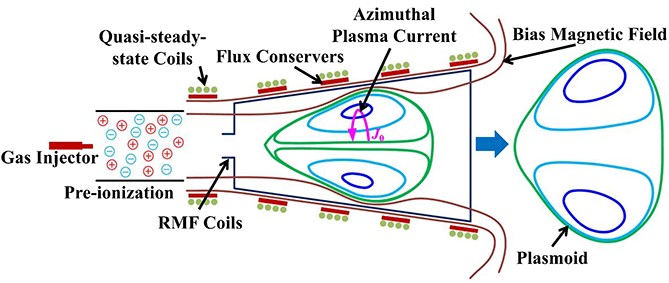
The RMF-FRC thruster is a new type of electric thruster, as shown in figure 1. Its operation process is as follows: (1) the injected propellant is pre-ionized to obtain the initial plasma; (2) the RMF drives electrons to rotate, forming an azimuthal plasma current Jθ and generating the FRC; (3) the axial Lorentz force Jθ×Br is generated by the plasma current and the radial component of the bias magnetic field Br, which accelerates and ejects the FRC to generate force [6]. Therefore, the plasma acceleration during ejection is mainly determined by electromagnetic forces rather than thermal expansion, theoretically achieving high specific impulse, high thrust, and high efficiency.
The plasma current in most inductive pulsed plasma thrusters depends on the amplitude of the coil current, and when expanding to high power, it is necessary to increase the voltage of power supplies, which is a difficult engineering task. When the RMF fully penetrates the plasma in the RMF-FRC thruster, the plasma current is proportional to the RMF frequency and independent of the amplitude of the RMF. This means that there is no need to apply excessive current to the RMF coils, which can significantly reduce the requirements for switch performance in the circuit [7]. In addition, the RMF-FRC thruster does not require electrodes and the plasma is isolated in the chamber, which will not touch the pre-ionization antenna, thus maximizing its service life. The RMF-FRC thruster has become a cutting-edge research direction in the aerospace field, with the potential to produce further breakthroughs in deep space exploration.
The research on plasma currents induced by an RMF can be traced back to 1952, when Thonemann et al used an RMF to drive electrons to rotate together in a circular quartz device, thereby forming an azimuthal current in the plasma [8]. Subsequently, with the proposal of the FRC and the deepening of related research, scholars around the world have explored the application of the RMF in the FRC through simulations and experiments, such as the formation, maintenance, and constraint of the FRC [9‒12].
In contrast, research on RMF-FRC thrusters started relatively late, around the late 2000s. Sercel et al designed a 5 kW RMF-FRC thruster prototype and observed the process of plasma formation and ejection using a high-speed camera [13]. The thrust of a single pulse was measured to be 8 mN, with a maximum specific impulse of 400 s. Weber tested the effects of xenon, nitrogen, water vapor, and carbon dioxide as propellants, demonstrating the potential for in-situ resource utilization of RMF-FRC thrusters [14]. Gill et al calculated the efficiency of each part of the prototype, and found that the overall efficiency was less than 1% [15]. The analysis revealed that the dominant loss processes of energy were collisional excitation radiation and wall losses. Furukawa et al measured the effects of operating conditions such as the gas flow rate and RMF frequency on ion velocity, and ultimately obtained a maximum ion velocity of 4 km/s [16, 17]. Liu et al observed the plume in the prototype and found that the plume length was positively correlated with the bias magnetic field [18]. The thrust of the prototype was estimated to be 16.1 mN based on the measured plasma density. Thus, it can be seen that the RMF-FRC thruster is still in its infancy. Although theoretically it has the potential for deep space exploration, its current performance cannot compete with other more mature electric propulsion technologies.
The key factor determining the performance of RMF-FRC thrusters is the rapid formation process of a high-quality FRC. However, current research mainly focuses on testing of the final performance of the prototype, resulting in a lack of analysis on this critical process. In addition, unlike the quasi-steady state RMF-FRC that has been extensively studied in the magnetic confinement fusion field, an RMF-FRC suitable for propulsion operates in a pulsed state, and the research on the mechanism in this field is still lacking.
Therefore, the goal of this work is to determine the parameters of the RMF-FRC thruster prototype based on theoretical calculations, prototype construction, and experimental tests on the formation of a pulse-state RMF-FRC. The magnitude of the plasma current and axial magnetic field variation in reverse are measured to verify the formation of FRC plasma. In addition, the influence of the bias magnetic field on the RMF-FRC is studied. According to the results, the strategies to improve the performance of the prototype are put forward.
Figure 2 shows the formation of plasma current driven by the RMF. The RMF coils are composed of two sets of orthogonal coils. When a sinusoidal current with a phase difference of 90° is applied to each coil, the RMF will be generated in the conical discharge region, and the field can be expressed as
| BRMF=Bωcos(ωt)→x + Bωsin(ωt)→y, | (1) |
where Bω is the amplitude of the RMF, and ω is the angular frequency of the RMF, which is consistent with the current passing through the coils.
The initial plasma generated by pre-ionization is introduced into the conical discharge region. There are two conditions that need to be satisfied in order to form the plasma current [19]:
(1) ωci < ω < ωce, where ωci and ωce are the ion and electron cyclotron frequencies. Under this condition, the electrons are bound to the RMF, while the ions are not affected.
(2) ωce ≫ νei, where νei is the electron-ion collision frequency. Due to the fact that the ion velocity is much lower than the electron velocity, the ions are considered as a fixed background. Assuming that the electron pressure is uniform, the generalized Ohm’s law can be expressed as:
| E=η(J+1enηJ×B), | (2) |
where the first term in the right-hand equation is the Ohmic resistance term, and the second term is the Hall term [20]. η = mvei/ne2 is the plasma resistivity, m is the electron mass, n is the plasma density, e is the unit discharge. When ωce ≫νei, the Hall term will dominate. At this point, the response of electrons to the axial electric field is limited, but they rotate synchronously with the RMF, forming an azimuthal current.
According to Milroy’s simulation [21, 22], the penetration of the RMF can be determined by two dimensionless parameters, namely the electron magnetization parameter γ = ωce/vei and the plasma dimension parameter λ = Rp/δω, where Rp is the plasma radius and δω is the classical skin depth, expressed as:
| δω=√2ηωμ0. | (3) |
When γ reaches the critical value γc, the RMF can completely penetrate the plasma, and γc is expressed as:
| {γc=1.12λ, λ⩽ | (4) |
In addition, the larger the value of \gamma , the faster the RMF penetrates. The time required for complete penetration, τp, can be expressed as:
| \tau_{\mathrm{p}}=\frac{\lambda^2}{2}\sqrt{\frac{\gamma_{\mathrm{c}}}{\gamma-\gamma_{\mathrm{c}}}}. | (5) |
It is worth noting that τp is a dimensionless parameter that needs to be multiplied by 2π/ω to obtain the actual penetration time.
When the RMF completely penetrates the plasma and drives all the electrons to rotate, the azimuthal plasma current density Jθ can be expressed as a function related to the radial position r
| {J_\theta }(r) = - ne\omega r, | (6) |
where the negative sign indicates that the direction of the azimuthal current is opposite to the rotation direction of the RMF.
For the RMF system, the core design parameters include the coil radius rω, the current amplitude Iω, and frequency f. The amplitude of the RMF Bω can be calculated using the model shown in figure 3. A set of RMF coils consists of two coils (red and green, respectively). The angle between the two wires in one coil and the center point is 2θ. Assuming that the length of the coil is infinite and θ = 60°, the total magnetic field Bω generated by a set of RMF coils at the center point is:
| {B_\omega } = \frac{{2{\mu _0}{I_\omega }\sin \theta }}{{ {\text{π}} {r_\omega }}} = \frac{{\sqrt 3 {\mu _0}{I_\omega }}}{{ {\text{π}} {r_\omega }}}. | (7) |
Due to the fact that the RMF coils are attached to the surface outside the vacuum chamber, it is expected that the plasma radius Rp will be similar to the coil radius rω. Therefore, rω will be used instead of Rp in the design. According to equation (7) and the conditions for complete penetration of the RMF and formation of plasma currents described above, the following inequalities are obtained:
| \frac{e}{m}\frac{{\sqrt 3 {\mu _0}{I_\omega }}}{{ {\text{π}} {r_\omega }}} > \omega , | (8) |
| \frac{{{q_i}}}{{{M_i}}}\frac{{\sqrt 3 {\mu _0}{I_\omega }}}{{{\text{π}}{r_\omega }}} < \omega , | (9) |
| \frac{e}{m}\frac{\sqrt{3}\mu_0I_{\omega}}{\text{π}r_{\omega}}\gg\nu\mathrm{_{ei}}, | (10) |
| \frac{\sqrt{6\mu_0}}{\text{π}\sqrt{nm\nu_{\mathrm{ei}}}}\frac{I_{\omega}}{r_{\omega}^2} > \sqrt{\omega}. | (11) |
where qi is the ion charge, Mi is the ion atomic mass.
The coil radius rω should match the dimension of the thruster. For the prototype designed in this work, rω is 0.07 m. In this experiment, argon was used as the propellant. Due to the good ionization effect of the RMF, it is expected that the plasma density n during the action of RMF will reach 2 × 1019 m−3, and the electron temperature Te will be 10 eV. Therefore, the parameter range of the coil current amplitude Iω at different RMF frequencies f is calculated using equations (8)‒(11) as shown in figure 4, where the shaded area represents the effective range. It can be seen that the parameter interval is mainly determined by equations (9) and (11).
According to equation (6), the RMF frequency f directly affects the magnitude of the plasma current. Therefore, it is necessary to maximize f, but an excessively high f will reduce the RMF penetration rate, which is not conducive to the formation of the plasma current. Considering the limitation of the power supply, f was ultimately chosen to be 200 kHz. Integrating equation (6), the plasma current generated is expected to reach 2 kA.
Figure 5 shows the relationship between the RMF penetration time and the coil current amplitude Iω when f is 200 kHz. When Iω reaches 2 kA, the effect of further increasing Iω on reducing the penetration time τp is not significant. In addition, considering the insulation level of the circuit, Iω was ultimately determined to be 2 kA. At this time, the actual penetration time is about 25 μs.
When the plasma current is formed, the axial magnetic field variation \Delta {B_z} can be expressed as:
| \Delta B_z=-\frac{\mu_0ne\omega}{2}\left(R_{\mathrm{p}}^2-r^2\right). | (12) |
Under the above conditions, \Delta {B_z} at r = 0 mm is approximately 124 Gauss. Correspondingly, when the bias magnetic field Bbias is less than 124 Gauss, the axial magnetic field can be reversed and the FRC is formed. Bbias was determined to be 60 Gauss, towards the downstream direction. Due to the unclear impact of Bbias on the experimental results of RMF-FRC thrusters, the value determined here may not be the optimal condition. Therefore, in experiments with the prototype, we studied the influence of Bbias on the formation of the RMF-FRC and optimized the operating parameter.
The main structure of the RMF-FRC thruster prototype is shown in figure 6(a). The middle is a quartz vacuum chamber, divided into a pre-ionization zone and an RMF zone. Figure 6(b) shows the dimensions of the vacuum chamber, which is divided into two parts, namely a pre-ionization zone and an RMF zone. The pre-ionization zone has an outer diameter of 100 mm and a length of 240 mm. The RMF zone has a conical structure with a half cone angle of 9.93°, an outer diameter of 170 mm at the downstream end, and an axial length of 200 mm. The installation locations of diagnostic parts, such as B-dot probes, Langmuir probes, and the Roche coil, are marked in figure 6(b).
Figure 7 is a physical image of the RMF-FRC thruster prototype constructed in this work, with the red arrow pointing downstream. The device is equipped with a mechanical pump and a molecular pump for vacuum pumping. The mechanical pump has a pumping speed of 40 m3/h and the molecular pump has a pumping speed of 1200 L/s. At the downstream outlet of the prototype, an ionization gauge is used to measure the vacuum chamber pressure, which can reach a minimum of 10−4 Pa without operation of the thruster. Argon is injected into the vacuum chamber through a flow controller, and the gas flow rate can be set to 0−50 mL/min.
A helicon antenna powered by a 13.56 MHz RF power supply is used as the pre-ionization source and is expected to produce an initial plasma with a density of 1017−1018 m−3. Nine quasi-steady-state coils located at the outermost edge of the vacuum chamber are used to generate a bias magnetic field Bbias parallel to the vacuum chamber wall to constrain the plasma and accelerate the FRC to ejection by its radial component. Bbias can be adjusted between 0 and 400 Gauss, including the set value of 60 Gauss mentioned above.
The RMF coils are wound on the surface outside the vacuum chamber. In order to generate an oscillating current with a frequency of 200 kHz in the RMF coils, the coils are connected in series with a discharge capacitor through an IGBT to form an LC circuit. A high-voltage power supply is used to charge capacitors and the IGBT is turned on through optical fibers to achieve the sequential pulse discharge of two LC circuits. It can produce a damped oscillating current through each coil, with a maximum peak of 2 kA and a frequency of 210 kHz, which is slightly higher than the design. The phase difference between the two coil groups is 90°.
In this study, diagnostics mainly include B-dot probes, Langmuir probes, Roche coils, and current probes. B-dot probes can be used to measure the magnetic field variation during the action of the RMF, according to Faraday’s law, and they are installed at the middle of the RMF zone (z = 100 mm) in the vacuum chamber at two radial positions: the axis (r = 0 mm) and near the vacuum chamber wall (r = 56 mm). The axial magnetic field variation \Delta {B_z} can be calculated using the induced electromotive force at both ends of the B-dot probe. According to the concept of the FRC, its internal magnetic field is reversed and the external magnetic field is enhanced. The magnetic field measurement results at r = 0 mm can help evaluate whether the field reverses and the degree of field reversal, while the B-dot probe at r = 56 mm is used to measure whether the axial magnetic field outside the plasma is enhanced. The effective area S of each B-dot probe is calibrated using the Helmholtz coils, and it is approximately 60 cm2.
The Langmuir probe is the most commonly used plasma diagnostic equipment, and triple Langmuir probes can be utilized to measure the plasma density and electron temperature in a certain location at the actual time. The Langmuir probe used in the experiment is made of three tungsten rods with a diameter of 0.8 mm and a length of 5 mm. A Roche coil was designed and fabricated based on the dimension of the RMF zone in the vacuum chamber, and installed inside the chamber to measure plasma currents. The current waveform of the RMF coil can be measured by the current probe.
In this section, we detail the experimental results of the RMF-FRC thruster prototype under rated conditions, as well as the influence of a bias magnetic field on the prototype performance parameters, such as the plasma currents.
According to the designed parameters, the maximum peak current of the RMF coil is 2 kA, and the bias magnetic field is 60 Gauss. When the pressure is 0.8 Pa and the argon flow rate is 20 mL/min, the plasma density under the action of the RMF can reach 2.2 × 1019 m−3 as measured by the Langmuir probe. Under these conditions, RMF coil currents, plasma currents, and axial magnetic field variation were measured.
Figure 8 shows the RMF current waveforms under vacuum and with plasma. Since the circuit parameters of each RMF coil are basically the same, only the current waveform of one coil is selected for analysis here. The blue curve represents the current waveform under vacuum conditions, with an oscillation frequency of 210 kHz and a maximum peak current of 2 kA, which is in line with the designed parameters of the RMF system. The red curve represents the current waveform when the plasma is loaded. By comparison, it can be found that the RMF current with plasma decreases significantly from the 3rd cycle, with a faster decay rate and higher frequency throughout the entire discharge process. This indicates that the coupling between the plasma and the RMF coil changes the impedance of the circuit.
From the energy perspective, the RMF current indirectly represents the energy loss in the circuit, and the decrease in the RMF current with plasma indicates that part of the RMF energy is coupled into the plasma. The coupling efficiency {\eta }_{\text{c}} can be calculated according to the following formula:
| {\eta }_{\text{c}}=1-\frac{{\displaystyle \int R\left({i}_{x}^{'2}+{i}_{y}^{'2}\right)}{\mathrm{d}}t}{{E}_{\text{in}}}=1-\frac{{\displaystyle \int \left({i}_{x}^{'2}+{i}_{y}^{'2}\right)}{\mathrm{d}}t}{{\displaystyle \int \left({i}_{x}^{2}+{i}_{y}^{2}\right)}{\mathrm{d}}t}, | (13) |
where R is the total resistance of the RMF system (including the power supply and transmission line), which is considered not to be affected by plasma. ix and iy are the total current of the two groups of RMF coils under vacuum, respectively, while {i}_{x}^{'} and {i}_{y}^{'} are the current with plasma. Ein is the total energy of each pulse of the RMF system, which can be calculated by the Ohmic loss under vacuum conditions. The coupling efficiency {\eta }_{\text{c}} of this prototype is calculated to be approximately 53%.
The coupling efficiency {\eta }_{\text{c}} , as a key component of the overall efficiency of the prototype, directly affects the final performance of the prototype. At present, the coupling efficiency of other RMF-FRC thruster prototypes in the world is generally not high, at about 25% [15]. The experimental results of this prototype have shown significant improvement in this aspect.
There are two main ways in which RMF energy is coupled to plasma: ionizing neutral particles and driving plasma currents. As it is difficult for the ionization rate of the pre-ionization rate to reach 100%, and there is recombination of electrons and ions during the experiment, there are still some neutral particles in the vacuum chamber, and the RMF will enhance the ionization. In the thruster, if the RMF loses too much energy in ionization, its effect on driving plasma currents will be weakened. The plasma density before and after the RMF action was measured using the Langmuir probe, and it was found that the density increased from 5 × 1017 m−3 to 2.2 × 1019 m−3, indicating that the ionization in the RMF energy coupling is quite significant. In the future, it is necessary to increase the initial plasma density generated by the pre-ionization source, so that more RMF energy can be used to drive plasma currents rather than enhance ionization.
The waveform of the plasma current ip during RMF action was directly measured using the Roche coil, as shown by the red curve in figure 9. Here, the rotation direction of the RMF is selected as the reference direction. The black dashed curve represents the corresponding RMF current waveform. It can be seen that during the first cycle of the RMF (about 5 μs), there was no plasma current induced, indicating that the RMF has not yet produced any effect at this stage. The plasma current began to form during the second cycle of the RMF and continued to increase, with a direction opposite to the RMF, so it was recorded as negative, which is consistent with theory. At around 20 μs, the plasma current peaked at approximately 1.9 kA. The formation process of the plasma current actually represents the penetration of the RMF, and the time required was not much different from the expectation. Subsequently, the plasma current gradually decreased, and disappeared at around 60 μs, which may be due to the deterioration of the RMF drive effect, but more likely, the plasma was accelerated and exhausted downstream. In other words, the measurement result of the Roche coil indicates that the designed prototype successfully induced the plasma current.
Assuming that the plasma is uniformly distributed in the RMF zone and that all electrons are driven to rotate by the RMF, under the experimentally measured plasma density, the plasma current density Jθ = 4645·r kA/m2 is obtained according to equation (6), where r is in unit of meter. Then, by integrating the cross-sectional area, the plasma current Ip is calculated to be about 2.28 kA, slightly higher than the peak value measured using the Roche coil, because the actual range of the plasma current is not as large as assumed.
The axial magnetic field variation \Delta {B_z} measured by B-dot probes can indirectly verify the formation of plasma current. Based on the degree of field reversal, we can determine whether the experimental results meet the design expectations of the prototype.
Figure 10 shows the \Delta {B_z} as a function of time, where the direction pointing downstream is considered positive. The black dashed curve represents the corresponding RMF current waveform, while the red and blue curves represent \Delta {B_z} at r = 0 mm and r = 56 mm, respectively. It can be seen that during the first cycle of the RMF (about 5 μs), the axial magnetic field at both locations had little changes. At 5 μs, the axial magnetic field at r = 0 mm began to decrease, reverse, and strengthen in the opposite direction. The peak value of \Delta {B_z} reached −155 Gauss at 20 μs, and the corresponding magnetic field Bz was about −95 Gauss. On the contrary, the axial magnetic field at r = 56 mm increased, and the peak value of \Delta {B_z} was about 51 Gauss. The result indicates that the RMF successfully drove the plasma current, causing {B_z} at the axis to reverse and form an FRC, while the magnetic field lines of the bias magnetic field were squeezed between the plasma separatrix and the quasi-steady-state coils, resulting in the increase of {B_z} near the vacuum chamber wall. During 20‒60 μs, \Delta {B_z} at both positions gradually returned from the peak to 0. On one hand, this is because the RMF continued to decay, making it difficult to maintain the plasma current. On the other hand, the FRC was accelerated by the Lorentz force and moved downstream. The trend of \Delta {B_z} is very consistent with the trend of the plasma current, which proves the accuracy of the experimental results and further indicates that the plasma current was successfully driven by the RMF in the prototype.
In addition, the measurement results between the B-dot probe and the Roche coil correspond numerically, indicating that the diagnostics in this work are relatively reliable.
Under the operating conditions of the design scheme, the results are close to the expected ones, meaning that the design scheme adopted in this work is feasible and has certain reference significance for the design of RMF-FRC thrusters.
Based on the experiments under rated operating conditions, we explored the influence of the bias magnetic field Bbias on the RMF-FRC, in order to optimize the operation scheme.
Figure 11 shows the RMF current curves when Bbias is 20, 60, 100, 140, and 180 Gauss, respectively. As Bbias increased, the time required for the RMF to start coupling increased, and the RMF current decay rate slowed down. Therefore, increasing Bbias will delay the effect of the RMF on the plasma and reduce energy coupling.
We calculated the RMF coupling efficiency under different Bbias, as shown in figure 12. It can be observed that the coupling efficiency decreased with an increase of Bbias. As a result, Bbias in the RMF-FRC thruster cannot be too large, otherwise it will have a significant negative impact on the RMF coupling efficiency, thereby limiting the overall efficiency of the prototype.
The main influence of Bbias on the formation of RMF-FRC is reflected in the plasma current and axial magnetic field variation \Delta {B_z} . Since \Delta {B_z} at r = 0 mm corresponds numerically to the plasma current and reflects the degree of magnetic field reversal, the analysis here is based on \Delta {B_z} at r = 0 mm.
The \Delta {B_z} curves at r = 0 mm under different Bbias were measured separately, as shown in figure 13. As Bbias gradually increased from 20 Gauss to 180 Gauss, the moment when {B_z} changed lagged behind in sequence, consistent with the pattern exhibited by the RMF current curves. In addition, the peak value of \Delta {B_z} increased first and then decreased. Increasing Bbias helps the initial plasma to diffuse from the pre-ionization zone to the RMF zone, thereby enhancing the formation of FRC. However, excessive Bbias will limit electrons from rotating with the RMF, resulting in a reduced plasma current. When Bbias was 180 Gauss, the peak value of \Delta {B_z} was only −118 Gauss, and the axial magnetic field was not reversed, so an FRC did not form. When Bbias was 100 Gauss, the peak value of \Delta {B_z} reached its maximum of −164 Gauss, and the axial magnetic field can be reversed. At this point, the formation effect of an FRC was the best. According to the measurement result of the Roche coil, the corresponding plasma current was about 2.1 kA. Therefore, the optimal Bbias of the prototype is around 100 Gauss from the perspective of plasma currents. However, the RMF coupling efficiency is not the highest at this time, and further research on the value of Bbias is necessary.
In fact, the basic performance of the prototype still has much room for further improvement. On one hand, the initial plasma density was low, with a value of only 5 × 1017 m−3, resulting in a large loss of RMF energy to ionization, and the density during the action of RMF was only 2.2 × 1019 m−3. Even if all electrons rotate with the RMF, the plasma current cannot meet the standard of high specific impulse and high thrust. On the other hand, the RMF coupling efficiency was not satisfactory and would limit the overall efficiency. It is necessary to appropriately enhance the RMF strength, which will help reduce the RMF penetration time, thereby improving the RMF coupling efficiency and the FRC formation rate. Therefore, improving the pre-ionization source, and enhancing the RMF strength are the future priorities.
In this work, we have outlined the principle of an RMF-FRC thruster and analyzed the main parameters of a prototype based on theoretical formulas. On this basis, an experimental prototype was constructed, with an RMF frequency of 210 kHz and a maximum peak RMF current of 2 kA.
Under the preset operating conditions, we compared the RMF current waveforms and analyzed the effect of the RMF from the perspective of energy. The coupling efficiency was calculated to be about 53%, which is higher than other known prototypes. The peak values of the plasma current and the axial magnetic field reverse variation were approximately 1.9 kA and 155 Gauss, respectively. The results demonstrated that the RMF in the prototype can successfully drive plasma currents with high parameters, and the reversal of the axial magnetic field indicated the formation of an FRC. Therefore, the design method in this work is feasible under the experimental results.
From the results under different Bbias, it can be found that increasing Bbias will reduce the RMF coupling efficiency and delay the effect of the RMF on the plasma. When Bbias was 100 Gauss, the peak value of the plasma current was 2.1 kA, which was the highest. Although the performance is already at a relatively high level, there is still room for further improvement. At present, the main problem is the low plasma density. In the next step, we will improve the pre-ionization source and increase the initial plasma density.
In summary, our work provides a reliable reference method for the design of RMF-FRC thrusters and the experimental research of RMF-FRC in the propulsion field has been carried out in a pioneering way.
This work was supported by National Natural Science Foundation of China (NSFC) (Nos. 62201217 and 51821005).
| [1] |
Polzin K et al 2020 Aerospace 7 105 doi: 10.3390/aerospace7080105
|
| [2] |
Sun X F et al 2018 J. Rocket Propuls. 44 44 (in Chinese) doi: 10.3969/j.issn.1672-9374.2018.01.007
|
| [3] |
Brown N P and Walker M L R 2020 Appl. Sci. 10 3775 doi: 10.3390/app10113775
|
| [4] |
Furukawa T, Kuwahara D and Shinohara S 2021 Phys. Plasmas 28 073507 doi: 10.1063/5.0035383
|
| [5] |
Furukawa T et al 2017 AIP Adv. 7 115204 doi: 10.1063/1.4998248
|
| [6] |
Weber T E, Slough J T and Kirtley D 2012 Rev. Sci. Instrum. 83 113509 doi: 10.1063/1.4759000
|
| [7] |
Bathgate S N, Bilek M M M and Mckenzie D R 2017 Plasma Sci. Technol. 19 083001 doi: 10.1088/2058-6272/aa71fe
|
| [8] |
Thonemann P C, Cowhig W T and Davenport P A 1952 Nature 169 34 doi: 10.1038/169034a0
|
| [9] |
Slough J T and Miller K E 2000 Phys. Plasmas 7 1945 doi: 10.1063/1.874019
|
| [10] |
Steinhauer L C 2011 Phys. Plasmas 18 070501 doi: 10.1063/1.3613680
|
| [11] |
Glasser A H and Cohen S A 2022 Rev. Sci. Instrum. 93 083506 doi: 10.1063/5.0101665
|
| [12] |
Velas K M and Milroy R D 2014 Phys. Plasmas 21 012502 doi: 10.1063/1.4861131
|
| [13] |
Sercel C L, Gill T M and Jorns B A 2023 Plasma Sources Sci. Technol. 32 105017 doi: 10.1088/1361-6595/acfd5a
|
| [14] |
Weber T 2010 The electrodeless Lorentz force thruster experiment PhD Thesis University of Washington, Seattle, USA
|
| [15] |
Gill T M, Sercel C L and Jorns B A 2024 Plasma Sources Sci. Technol. 33 015006 doi: 10.1088/1361-6595/ad107a
|
| [16] |
Furukawa T et al 2018 Rev. Sci. Instrum. 89 043505 doi: 10.1063/1.5013214
|
| [17] |
Furukawa T et al 2017 Phys. Plasmas 24 043505 doi: 10.1063/1.4979677
|
| [18] |
Liu X X et al 2022 IEEE Trans. Plasma Sci. 50 2138 doi: 10.1109/TPS.2022.3175526
|
| [19] |
Jones I R and Hugrass W N 1981 J. Plasma Phys. 26 441 doi: 10.1017/S0022377800010837
|
| [20] |
Slough J T and Miller K E 2000 Phys. Rev. Lett. 85 1444 doi: 10.1103/PhysRevLett.85.1444
|
| [21] |
Milroy R D 1999 Phys. Plasmas 6 2771 doi: 10.1063/1.873234
|
| [22] |
Milroy R D 2000 Phys. Plasmas 7 4135 doi: 10.1063/1.1290279
|
| [1] | Muhammad Ajmal KHAN, Jing LI (李静), Heping LI (李和平), Hafiz Imran Ahmad QAZI. Characteristics of a radio-frequency cold atmospheric plasma jet produced with a hybrid cross-linear-field electrode configuration[J]. Plasma Science and Technology, 2019, 21(5): 55401-055401. DOI: 10.1088/2058-6272/ab004b |
| [2] | Syed ZAHEERUDDIN, Yufan LI (李煜璠), Dongmei ZHAO (赵冬梅), Xinwen MA (马新文), Jie YANG (杨杰). The effect of the direct current electric field on the dynamics of the ultracold plasma[J]. Plasma Science and Technology, 2018, 20(8): 85001-085001. DOI: 10.1088/2058-6272/aac166 |
| [3] | S N BATHGATE, M M M BILEK, D R MCKENZIE. Electrodeless plasma thrusters for spacecraft: a review[J]. Plasma Science and Technology, 2017, 19(8): 83001-083001. DOI: 10.1088/2058-6272/aa71fe |
| [4] | Y WANG (王宇), G ZHAO (赵高), C NIU (牛晨), Z W LIU (刘忠伟), J T OUYANG (欧阳吉庭), Q CHEN (陈强). Reversal of radial glow distribution in helicon plasma induced by reversed magnetic field[J]. Plasma Science and Technology, 2017, 19(2): 24003-024003. DOI: 10.1088/2058-6272/19/2/024003 |
| [5] | CHANG Lei (苌磊), LI Qingchong (李庆冲), ZHANG Huijie (张辉洁), LI Yinghong (李应红), WU Yun (吴云), ZHANG Bailing (张百灵), ZHUANG Zhong (庄重). Effect of Radial Density Configuration on Wave Field and Energy Flow in Axially Uniform Helicon Plasma[J]. Plasma Science and Technology, 2016, 18(8): 848-854. DOI: 10.1088/1009-0630/18/8/10 |
| [6] | WU Ding (吴鼎), LIU Ping (刘平), SUN Liying (孙立影), HAI Ran (海然), DING Hongbin (丁洪斌). Influence of a Static Magnetic Field on Laser Induced Tungsten Plasma in Air[J]. Plasma Science and Technology, 2016, 18(4): 364-369. DOI: 10.1088/1009-0630/18/4/06 |
| [7] | ZHAO Qing(赵青), XING Xiaojun(邢晓俊), XUAN Yinliang(宣银良), LIU Shuzhang(刘述章). The Influence of Magnetic Field on Antenna Performance in Plasma[J]. Plasma Science and Technology, 2014, 16(6): 614-619. DOI: 10.1088/1009-0630/16/6/14 |
| [8] | ZHAO Bingbing(赵兵兵), HE Liming(何立明), DU Hongliang(杜宏亮), ZHANG Hualei(张华磊). Electrical Characteristics of an Alternating Current Plasma Igniter in Airflow[J]. Plasma Science and Technology, 2014, 16(4): 370-373. DOI: 10.1088/1009-0630/16/4/12 |
| [9] | SHEN Wulin (沈武林), MA Zhibin (马志斌), TAN Bisong (谭必松), WU Jun (吴俊). Ion Heating in an ECR Plasma with a Magnetic Mirror Field[J]. Plasma Science and Technology, 2013, 15(6): 516-520. DOI: 10.1088/1009-0630/15/6/06 |
| [10] | MU Zongxin (牟宗信), WANG Chun (王春), MU Xiaodong (牟晓东), JIA Li (贾莉), LIU Shengguang (刘升光), DONG Chuang(董闯). Experimental Study of the Effect of Applied Magnetic Field on Plasma Properties of Unbalanced Magnetron Sputtering[J]. Plasma Science and Technology, 2010, 12(5): 571-576. |