The spectrum analysis of RMP coils with multiple connection modes and the design of high field side coils on J-TEXT

1.   Introduction

Multiple locked modes enhance plasma heat transport and flatten the temperature profile, which has an important effect on the thermal quench during disruptions [1–5]. The current simulated studies show that both an m/n = 3/1 island width and the central electron temperature are sensitive to the phase difference between m/n = 2/1 island and m/n = 3/1 island (Δξ) [6], where m and n are the poloidal and toroidal mode numbers, respectively. The width of the 3/1 island is suppressed and the central electron temperature remains at a high value when Δξ is located in the region of Δξ∈[100°, 200°], which is expected to prevent island overlap and disruption [6]. To verify the above simulation result, it is needed to generate resonant magnetic perturbations (RMPs) with adjustable phase difference Δξ and sufficient amplitudes to induce multiple locked islands.

Saddle coils are widely used to generate RMPs in existing tokamaks. The saddle coils are mostly installed on the low field side (LFS) than the high field side (HFS), such as DIII-D [7], KSTAR [8], JET [9], ASDEX-U [10], EAST [11] etc. On J-TEXT, three rows of saddle coils are installed at the top, LFS-midplane, and bottom of the vacuum vessel [12, 13]. Although RMP coils have been widely used, almost all the attention has been paid to the amplitudes of various components, i.e. the amplitude of the spectrum, ignoring the role of the phases of those components.

In this work, we analyze the spectrum of the J-TEXT RMP coils and focus on the phases and amplitudes of the 2/1 and 3/1 RMP fields. It is found that those RMP coils could only generate RMPs with limited phase difference, Δξ∈[−75°, 75°], or a weak RMP whose Δξ tends to be 180° but the amplitude is too small to penetrate. To extend the adjustable range of the phase difference, installing saddle coils on the HFS is proposed. By applying the HFS and LFS coils, the phase difference Δξ of RMP can be adjusted from −180° to 180° by changing the phase difference and current proportion between the HFS and LFS coils.

This paper is arranged as follows. In section 2, the calculation process of the RMP spectrum in the flux coordinate is introduced. In section 3, the adjustable range of Δξ when applying the current RMP coils on J-TEXT is discussed in detail. In section 4, the design of HFS coils and the extended Δξ range are introduced. Finally, section 5 is the conclusion.

2.   Calculation of the RMP spectrum

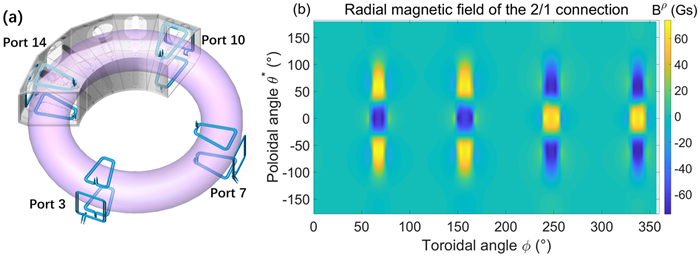
On the J-TEXT tokamak, a set of two-turn in-vessel coils has been installed as three rows of coils at the top, bottom, and LFS-midplane, as shown in figure 1(a). The connection of each coil could be changed separately. The two sets of power supplies are used to supply the RMP coils, the toroidal phase of the RMP could be adjusted by changing the current proportion. As a result, various RMP spectra can be realized by applying RMP coils with different connection modes and current proportions.

Figure  1.  (a) Double-turn RMP coils on J-TEXT, (b) radial magnetic field of the 2/1 connection, I_RMP = 1 kA.

DownLoad: Full-Size Img PowerPoint

The RMP connection with the dominant m/n = 2/1 component is taken as an example. To mainly provide n = 1 magnetic perturbation (MP) components, every two coils with a 180° toroidal separation are connected with opposite current directions. To make the m = 2 components as large as possible, the current fed in the top coils is the same as the current in the bottom coils and opposite to the current in the LFS-midplane coils. This connection generates the strongest 2/1 RMP component, called the 2/1 connection. The distribution of the radial magnetic field at the last closed flux surface (LCFS) of this connection is shown in figure 1(b), the positive direction of the radial magnetic field is from the magnetic axis to the LCFS.

To quantitatively investigate the effects of the perturbation field, Fourier spectrum analysis is commonly used. The spectrum of the perturbation field should be evaluated in a corresponding coordinate, and the Fourier coefficients depend on the choice of poloidal angle of the coordinate [14]. In the cylindrical coordinate (r, θ, ϕ), considering the poloidal asymmetry of the toroidal magnetic field B_ϕ, the magnetic field lines are not straight on the θ–ϕ plane. The wave vectors of the decomposed components are not always perpendicular to the field line, and the resonant relationship between the RMP components and the equilibrium field line on the resonant surface cannot be fully described. To ensure the resonant relationship, the poloidal coordinate needs to be substituted by θ^*, and the field lines are straight on the θ^*–ϕ plane. The spectrum should be calculated in the flux coordinate (ρ, θ^*, ϕ), where $\rho =\sqrt{(\psi -{\psi }_0)/({\psi }_a-{\psi }_0)}$ is the normalized minor radius, ψ is the poloidal flux, ψ₀ and ψ_a are the poloidal flux at the magnetic axis and the LCFS, respectively, the straight field line angle θ^*(θ) satisfying the relation dθ^*/dϕ = 1/q is the poloidal angle, and ϕ is the toroidal angle. The flux coordinate is obtained by a selected equilibrium from EFIT. The plasma equilibrium chosen in this paper has a minor radius of 25.5 cm, a toroidal field of 1.7 T, a plasma current of 155 kA, and an edge safety factor of 3.4. At the plasma edge, the deviation of θ^* from θ is larger, and the distribution of θ^* is dense on the HFS and sparse on the LFS, as shown in figure 2(b).

Figure  2.  (a) Cylindrical coordinate, (b) flux coordinate, (c) spectrum of 2/1 connection in the cylindrical coordinate (I_RMP = 1 kA), (d) spectrum of 2/1 connection in the flux coordinate (I_RMP = 1 kA).

DownLoad: Full-Size Img PowerPoint

The vacuum magnetic field B in the plasma region produced by RMP coils can be calculated by using the Biot–Savart law, neglecting the size of the coil conductors and without taking plasma responses into account. The component perpendicular to the magnetic surface B^ρ has the strongest effect on plasma, it is also a radial-like component for the circular plasma of J-TEXT. The B^ρ could be calculated by the following formula

B^ρ can be expressed as

where b_m/n^ρ is the m/n component on the chosen magnetic surface, ξ_m/n is the helical phase of the m/n component. The m/n component can be calculated by Fourier transform. The spectra of the 2/1 connection on the LCFS in the cylindrical coordinate and the flux coordinate are shown in figures 2(c) and (d). In the flux coordinate, the magnitude of the 2/1 component is not as large as expected, and the 3/1 component is much larger than that in the cylindrical coordinate, the effect of the 3/1 component cannot be neglected. The result calculated in the flux coordinate is more reliable, the analysis in this paper is carried out in the flux coordinate.

3.   The influence of coil connections on the phase difference Δξ

By adjusting the phase of different rows of coils, various spectra of the MPs can be formed. Considering the radial position of the resonant surface and the difference in magnetic topology, the 2/1 and 3/1 components are calculated on the q = 2 and q = 3 surfaces, respectively, in the flux coordinate, and the helical phases of the 2/1 and 3/1 components are ξ_2/1 and ξ_3/1, respectively. The phase difference between 2/1 and 3/1 components, Δξ = ξ_2/1 − ξ_3/1, can be adjusted by applying multiple rows of coils. A row of coils is divided into two groups with a 90° phase difference in the toroidal direction, and two coils of the same group with a 180° toroidal separation are connected with opposite current directions. The phase of the n = 1 MP generated by the row can be adjusted by changing the current ratio between the two groups of coils.

Two rows of coils at the top and bottom, with poloidal phases of 75° and −75° in the flux coordinate, respectively, are used to illustrate the adjustment of coil connections, whose B^ρ distributions are shown in figure 3. The dashed line indicates the distribution of the n = 1 MPs generated by each row of coils, the toroidal phase of its maximum value is called the phase of that row of coils. The phases of the top, LFS-midplane, and bottom rows of coils are noted as ϕ_T, ϕ_LFS, and ϕ_B, and the toroidal phase difference between top and bottom coils currents is Δϕ_TB = ϕ_T − ϕ_B. Fixing I_T = I_B = 1 kA and ϕ_T, changing ϕ_B, Δϕ of top and bottom coils are changing continuously, namely Δϕ_TB scan. The radial magnetic field distributions at Δϕ_TB = 30°, 60° and 90°, respectively, are shown in figure 3. The Fourier decomposition of the magnetic field yields that the amplitudes of the 2/1 and 3/1 components are different in the three cases, but Δξ in those cases equals 0°.

Figure  3.  Radial magnetic field distributions on the LCFS under different Δϕ_TB, fixing I_T = I_B = 1 kA and ϕ_T = 247.5°. (a) Δϕ_TB = 30°, (b) Δϕ_TB = 60°, (c) Δϕ_TB = 90°.

DownLoad: Full-Size Img PowerPoint

Further, the complete Δϕ_TB scan is performed, as shown in figure 4. The amplitudes of the 2/1 and 3/1 components vary sinusoidally with Δϕ_TB. The phase difference Δξ = 180° in the narrow interval of Δϕ_TB∈[100°, 200°], and Δξ = 0° in the rest of the interval.

Figure  4.  Changes of the amplitudes and phases of 2/1 and 3/1 components with Δϕ_TB, fixing I_T = I_B = 1 kA and ϕ_T = 0°. (a) Amplitudes, (b) phases.

DownLoad: Full-Size Img PowerPoint

The relationship between Δξ and Δϕ_TB could be explained analytically. The helical phase of the m/n component produced by a row of coils can be approximately expressed by the formula

where ξ_m/n is the helical phase of the m/n component, ϕ_c is the toroidal phase of the row of coils. Due to the difference in magnetic helicity, θ_{c, q}^* is the poloidal phase of the coils on the q = m/n resonant surface. From equation (3), Δξ of the 2/1 and 3/1 RMPs generated by a row of coils is Δξ = 3θ_{c, q=3}^* − 2θ_{c, q=2}^*. The difference between θ_{c, q}^* of adjacent rational surfaces is not significant, so the relationship can be simplified as Δξ ≈ θ_{c, q}^*.

The MP components satisfy the vector superposition principle, so the MP components generated by the rows of the top and bottom coils can be superimposed linearly to obtain the total components. When I_T = I_B, |b_T| = |b_B|, the amplitudes of n = 1 MPs generated by the top and bottom coils are equal, and the total m/n component is

The poloidal positions of the top and bottom coils are symmetric about the midplane, θ_{B, q}^* = −θ_{T, q}^*. The helical phase of the m/n component is related to ϕ_T + ϕ_B and the sign of cos(mθ_{T, q}^* + Δϕ_TB/2), independent of the poloidal mode number m. Hence, ξ_2/1 and ξ_3/1 are always the same (or with 180° separation). The poloidal phases of the top coils on the 2/1 and 3/1 resonant surfaces are θ_{c, q=2}^* = 85° and θ_{c, q=3}^* = 75°, respectively. When Δϕ_TB ∈ [100°, 200°], the sign of cos(2θ_{T, q=2}^* + Δϕ_TB/2) is different from cos(3θ_{T, q=3}^* + Δϕ_TB/2), so the phase difference between 2/1 and 3/1 RMP components is Δξ = 180°. The amplitude of each component is related to cos(mθ_{T, q}^* + Δϕ_TB/2). Hence, it is difficult to adjust the phase difference between these two components at other values. These analytical results fully explain the calculating results in figure 4.

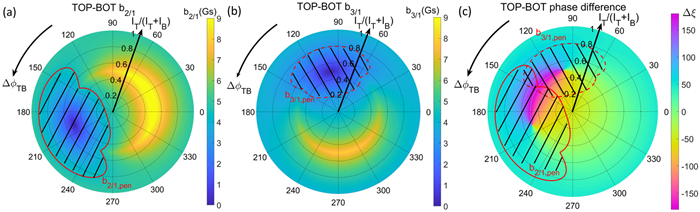
Δξ is also affected by the current ratio between two sets of coils, which is defined as I_T/(I_T + I_B). I_T and I_B are the currents fed in the top and bottom coils, respectively. The calculating results of the Δϕ scan of the top and bottom coils are shown in the polar coordinate in figure 5, where the poloidal coordinate is Δϕ_TB and the radial coordinate is I_T/(I_T + I_B).

Figure  5.  Adjustable range of Δξ applying top and bottom coils, where the poloidal coordinate is the phase difference Δϕ_TB and radial coordinate is the current ratio of top coils I_T/(I_T + I_B). (a) and (b) are the contour maps of the amplitudes of 2/1 component b_2/1, and 3/1 component b_3/1, respectively, with a maximum RMP current of max (I_T, I_B) = 7 kA, (c) is the contour map of phase difference Δξ between 2/1 and 3/1 components, the red solid line and red dashed line indicate the penetration thresholds of 2/1 and 3/1 components, respectively.

DownLoad: Full-Size Img PowerPoint

Figures 5(a) and (b) show the amplitudes of 2/1 and 3/1 RMP components on corresponding resonant surfaces. In J-TEXT, we choose the discharge parameters as B_T = 1.7 T, I_p = 160 kA, n_e = 0.8 × 10¹⁹ m⁻³, the penetration thresholds are b_{pen, 2/1} = 4.5 Gs and b_{pen, 3/1} = 3 Gs under this condition [15]. The maximum current of RMP coil power supplies is 7 kA. For the shaded region inside the red solid line in figure 5(a), the 2/1 MP component is too weak to penetrate. The 3/1 MP component is also too weak to penetrate the shaded region within the red dashed line in figure 5(b).

Figure 5(c) shows the relationship between Δξ and the coil currents. Δξ changes continuously in the interval Δξ∈[−180°, −50°] ∪ [50°, 180°] when Δϕ_TB ∈ [100°, 200°] with I_T/(I_T + I_B). For example, when I_T/(I_T + I_B)∈[0.4, 0.6] and Δϕ_TB = 150°, Δξ has a wide adjustable range of [−180°, −120°]∪[120°, 180°]. However, Δξ only changes from −50° to 50° when Δϕ_TB are other values.

To verify the simulation result [6], the RMP amplitudes should be large enough to penetrate and cause both 2/1 and 3/1 locked modes at the same time. Hence, only the region outside the red solid line and outside the red dashed line in figure 5(c) could be used to cause the locked modes, this region is called the usable region. In the usable region, Δξ is only adjusted in a limited range of [−75°, 75°]. Hence, the RMPs only generated by the top and bottom coils cannot fully meet the requirements for verifying the simulation results.

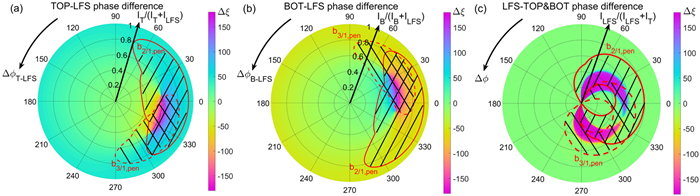
By applying the top coils and LFS coils, the range of Δξ close to 180° is also narrow and the amplitudes of RMP in this region cannot reach the penetration thresholds, as shown in figure 6(a). With sufficient RMP amplitudes, Δξ can only vary from 0° to 50° during the scan of Δϕ_T-LFS, which is the phase difference between the top and LFS coils, Δϕ_T-LFS = ϕ_T − ϕ_LFS. Another similar case is applying the bottom and LFS coils, whose Δξ can only vary from 0° to −50° in the usable region, as shown in figure 6(b).

Figure  6.  The contour maps of the phase difference Δξ, where the poloidal coordinate is the phase difference Δϕ and the radial coordinate is the ratio of currents in two rows of coils. (a) Δϕ scan between the top and LFS coils, (b) Δϕ scan between the bottom and LFS coils, (c) Δϕ scan applying three rows of coils, fixing Δϕ = Δϕ_T-LFS = Δϕ_LFS-B and I_T = I_B.

DownLoad: Full-Size Img PowerPoint

Three rows of coils on J-TEXT provide greater flexibility for spectrum control, but it is impossible to test all spectral variations. Similar to the work on KSTAR [8], n = 1 top–bottom symmetric cases are considered, where Δϕ = Δϕ_T-LFS = Δϕ_LFS-B and I_T = I_B. The variables of coil currents are reduced to the current ratio I_LFS/(I_LFS + I_T) and the phase difference Δϕ, as shown in figure 6(c). In this case, Δξ only equals 0° or 180° among the region and the amplitudes of MPs are too small when the two components are anti-phase. Actually, the phase differences Δξ generated by the top, LFS, and bottom coils are 50°, 0° and −50°, respectively. For two rows of coils that generate RMPs with phase differences Δξ_a and Δξ_b, respectively, it is easy to adjust Δξ from Δξ_a to Δξ_b when applying the two rows of coils. If Δξ is required to be larger than 90°, at least one component is too weak to penetrate, which is caused by the mutual cancellation of magnetic fields generated by different coils. As a result, it is difficult to generate RMPs with a large adjustable Δξ range and enough amplitudes of the 2/1 and 3/1 components for the 2/1 and 3/1 locked modes exciting at the same time by the current RMP coils in J-TEXT.

4.   Design of HFS coils and $\mathrm{\Delta }\xi$ generated by HFS coils

The phase difference Δξ when applying a single row of coils is expressed as Δξ ≈ θ_c^* by equation (3). For two rows of coils with poloidal positions θ_a^* and θ_b^*, they generate RMPs with phase differences Δξ_a ≈ θ_a^* and Δξ_b ≈ θ_b^*. When applying the two rows of coils, it is easy to adjust Δξ from Δξ_a to Δξ_b based on the results in section 3, i.e. Δξ∈[θ_a^*, θ_b^*]. Therefore, choosing θ_b^* = 180° can broaden the range of Δξ as large as possible. To generate RMPs with adjustable Δξ and verify the simulation result [6], new coils need to be constructed on the HFS.

For coils on the HFS, Δξ = θ_HFS^* = 180°, 2/1 and 3/1 RMPs are anti-phase and have sufficient amplitudes to induce locked islands. Then the HFS coils are expected to be designed as saddle loops in the vacuum vessel, simplified as rectangle coils.

The toroidal positions of HFS coils are similar to other coils, the radial position is R = 0.77 m. Considering the span of each port, the maximum toroidal angular span of each HFS coil is 18°, the width of each HFS coil is d = 24 cm, then the poloidal span Z is the only variable that needs to be optimized. Designing the HFS coils requires making the low poloidal mode number RMP components (especially 2/1 and 3/1) as large as possible. Because of the denser distribution of θ^* on the HFS, coils should have a smaller span than the LFS coils (Z_LFS = 45 cm). The relationship between the magnitude of each component and the poloidal span Z of the HFS coils is shown in figure 7(b), where Z is the span of the coil in the vertical direction. It could be found that both 3/1 and 2/1 components change non-monotonically with Z and the maximum values of the amplitudes of b_2/1 and b_3/1 are different. After fully considering the amplitude of both components, Z is chosen to be 20 cm, the following calculations are based on HFS coils of this size. Similar to the current RMP coils, the HFS coils are designed as 2-turn coils.

Figure  7.  (a) 2-turn RMP coils and HFS coils, (b) amplitude of the 2/1 and 3/1 components generated by HFS coils changing with the poloidal span Z.

DownLoad: Full-Size Img PowerPoint

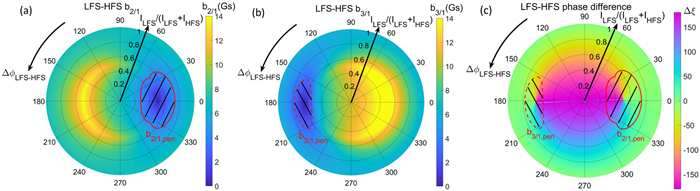
The HFS coils could generate 2/1 and 3/1 RMP fields with Δξ = 180°. The amplitudes of b_2/1 and b_3/1 are much stronger, and the shaded areas where the magnetic islands cannot be excited are smaller than those in figure 5, as shown in figures 8(a) and (b). When only applying the HFS coils, corresponding to the original point in the polar coordinate, the amplitudes of 2/1 and 3/1 components are still strong enough to penetrate. The adjustable range of Δξ is shown in figure 8(c), Δξ can select any value by changing the phase difference Δϕ_LFS-HFS and the current ratio I_LFS/(I_LFS +I_HFS). For the RMPs with a large range of Δξ, both the 2/1 and 3/1 components generated by the LFS coils could be enhanced by the HFS coils. The shaded areas become smaller and the usable region becomes larger through optimization. Hence, by applying the HFS and LFS coils, Δξ can vary from −180° to 180° continuously with sufficient RMPs.

Figure  8.  Adjustable range of Δξ applying the LFS and HFS coils, where the poloidal coordinate is the phase difference Δϕ_LFS-HFS and radial coordinate is the current ratio I_LFS/(I_LFS + I_HFS). (a) and (b) are the contour maps of the amplitude of b_2/1 and b_3/1, respectively, with a maximum RMP current of 7 kA, (c) is the contour map of the phase difference Δξ between the 2/1 and 3/1 components.

DownLoad: Full-Size Img PowerPoint

The poloidal phase difference between the top and HFS coils is about 105°. When applying the HFS and top coils, though Δξ also could be adjusted from −180° to 180°, the usable region with Δξ∈[−90°, 0°] is much smaller than the case of applying the HFS and LFS coils. On the other hand, the usable region with Δξ∈[0°, 90°] is much broadened and the RMP amplitudes are enhanced in this region, as shown in figure 9(a). The calculating result of applying the HFS and bottom coils is presented in figure 9(b), which is similar to the case of applying the HFS and top coils due to the poloidal symmetry between the top and bottom coils. After all, by applying the HFS and other coils, sufficient RMPs with an adjustable Δξ are realized.

Figure  9.  The contour maps of the phase difference Δξ, where the poloidal coordinate is the phase difference Δϕ and the radial coordinate is the ratio of currents in two rows of coils. (a) Δϕ scan between the top and HFS coils, (b) Δϕ scan between the bottom and HFS coils.

DownLoad: Full-Size Img PowerPoint

5.   Conclusions and discussions

The current RMP coils in J-TEXT are arranged at θ_{T, q=3}^* =75°, θ_{LFS, q=3}^* = 0° and θ_{B, q=3}^* = −75°, respectively. The phase difference Δξ of RMPs generated by a single row of coils approximately equals θ_c^*. According to equations (3) and (4), at least one component is not sufficient to excite the locked island due to the mutual cancellation of magnetic fields generated by different coils when Δξ takes a value near 180°. The phase difference Δξ could only be adjusted from −75° to 75°. Similar to J-TEXT, saddle coils in most tokamaks are installed close to the LFS, θ_{c, q}^* < 90°. Therefore, Δξ could not be adjusted in a wide range by scanning the toroidal phase difference and current ratio among different rows of coils in those tokamak devices.

Based on equations (3) and (4), RMP coils with a large Δξ are needed to broaden the adjusting range of Δξ. The HFS coils, with a moderate geometric shape, could generate RMPs with Δξ = 180° and sufficient amplitudes. By applying the HFS and LFS coils, the phase difference Δξ can be easily adjusted from −180° to 180°. This work provides a feasible solution for flexible adjustment of the phase difference between m and m + 1 RMP, which might facilitate the study of major disruptions and their control. The HFS coils might be constructed on J-TEXT in the future. The RMPs with different Δξ are expected to be used to investigate the influence of coupled magnetic islands on the thermal quench and mitigate the thermal quench in future devices.

Those results are obtained in the vacuum condition. The plasma response and toroidal mode coupling have an effect on the conclusions. The plasma with toroidal rotation provides a screening effect against the RMP. The amplitude of the RMP component decreases, and the phase is shifted compared with the vacuum condition. The screening effect is influenced by the local resistivity and toroidal rotation on the resonant surface [16]. When the RMP is sufficient to penetrate, a locked island is excited and the plasma rotation at the resonant surface approaches zero, the total magnetic field is enhanced [17, 18]. The penetration threshold will be reduced by non-resonant components through toroidal mode coupling [19, 20], so the penetration thresholds are different under various RMP spectra. Considering these effects, the amplitudes and phases of RMP components on the resonant surfaces are different from those under vacuum conditions, which will be investigated in the future.