| Citation: | Rui LI, Qihan WANG, Fucheng LIU, Kuangya GAO, Xiaohan HOU, Mengmeng JIA, Qing LI, Weili FAN. Reconfigurable (4, 62) and (4, 82) Archimedean plasma photonic crystals in dielectric barrier discharge[J]. Plasma Science and Technology, 2024, 26(6): 064008. DOI: 10.1088/2058-6272/ad341f |
Archimedean photonic crystal has become a research area of great interest due to its various unique properties. Here, we experimentally demonstrate the realization of reconfigurable (4, 62) and (4, 82) Archimedean plasma photonic crystals (APPCs) by use of dielectric barrier discharges in air. Dynamical control on both the macrostructures including the lattice symmetry and the crystal orientation, and the microstructures including the fine structures of scattering elements has been achieved. The formation mechanisms of APPCs are studied by time-resolved measurements together with numerical simulations. Large omnidirectional band gaps of APPCs have been obtained. The tunable topology of APPCs may offer new opportunities for fabricating multi-functional and highly-integrated microwave devices.
Archimedean lattices are infinite tessellation patterns made up of regular convex polygons [1]. These lattices have been known since ancient times, while their complete description of all 11 possible tilings was first depicted by Kepler in Harmonices Mundi II 1619 [2]. Recently, photonic crystals (PCs) with Archimedean lattices have gained increasing interest due to their advantages in terms of large photonic gap width, completeness, isotropy, and improved refraction characteristics [3–8]. The symmetry reduction in Archimedean PCs lifts the degeneracy of photonic bands at high symmetry points, leading to the enlargement of photonic band gaps (PBGs) or even omnidirectional band gaps (OBGs) [9–11]. The isotropy of bands can also be realized in Archimedean-like (quasicrystal) lattices with a high order of local symmetries. Such crystals exhibit substantially lower optical sensitiveness to the direction of light propagation, making them suitable for some special applications such as LEDs [7, 8]. Moreover, Archimedean PCs offer possibilities for negative refraction and left-handedness [3, 4]. These unique features bring about stirring applications such as filters, optical switching, wireless communications, and sensors [12].
Plasma photonic crystal (PPC) is a periodic structure consisting of plasma and dielectric materials or arranged with spatially periodic plasma densities [13, 14]. As a tunable PC, it allows for fast reconfiguration and real-time control of the photonic bands. So far, different proposals have been proposed to realize PPCs, via artificial array of gas discharge tubes [15, 16], by microplasma assemblies [17], by embedding dielectric arrays into plasma background [18, 19], or via self-organization of discharge filaments as we previously reported [20–22]. More recently, novel layered structures combining the photonic crystal and the nonlinear plasmas have been suggested, which opened up a new way to produce tunable PPCs [23–25]. They bring about a variety of extraordinary optical properties, including superior tunable bandwidth, Janus features, and optical nonreciprocity, due to the asymmetric and nonlinear properties of the materials. So far, various one-dimensional (1D) [26, 27], two-dimensional (2D) [28, 29] PPCs with square or triangular symmetries and three-dimensional (3D) ‘woodpile’ PPCs [30] have been obtained, possessing band gaps in the range of microwaves or terahertz waves. However, most PPCs reported are regular lattices composed of equal polygons, whereas a (4, 82) “bathroom” Archimedean lattice and a (32, 4, 3, 4) “ladybug” Archimedean lattice have been proposed [6]. Moreover, these PPCs are typically comprised of arrays of cylindrical plasma elements with identical sizes and shapes. Once the PPCs have been completed, the structures of plasma elements as well as the crystal orientation can hardly be modulated dynamically. Tunability is restricted to some extent. Exploration of tunable Archimedean PPCs allowing for flexible control of structural configurations including the crystal orientation, lattice symmetry, and fine structures of scattering elements is desired, which may lead to new exploitable properties for various applications.
In this paper, we present the experimental realization of reconfigurable Archimedean PPCs by use of dielectric barrier discharges (DBDs) with uniquely designed mesh-water electrodes. Dynamical control of both the macrostructures involving the lattice symmetry and crystal orientation, and the microstructures involving the fine structures of plasma elements has been achieved. Rapid reconfiguration among the square lattice, (4, 62) Archimedean lattice and (4, 82) Archimedean lattice is realized. An intriguing square lattice composed of composite plasma elements with tunable shapes and fine structures is proposed. Moreover, the band diagrams of PPCs are calculated by means of the finite-element method. Microwave diagnosis on PBGs has been implemented, which is in good agreement with the simulation results. OBGs located in the range of 36.7–40.2 GHz are achieved for Archimedean superlattices. Compared to the simple square lattices, Archimedean lattices possess better properties in terms of the location and width of the band gaps.
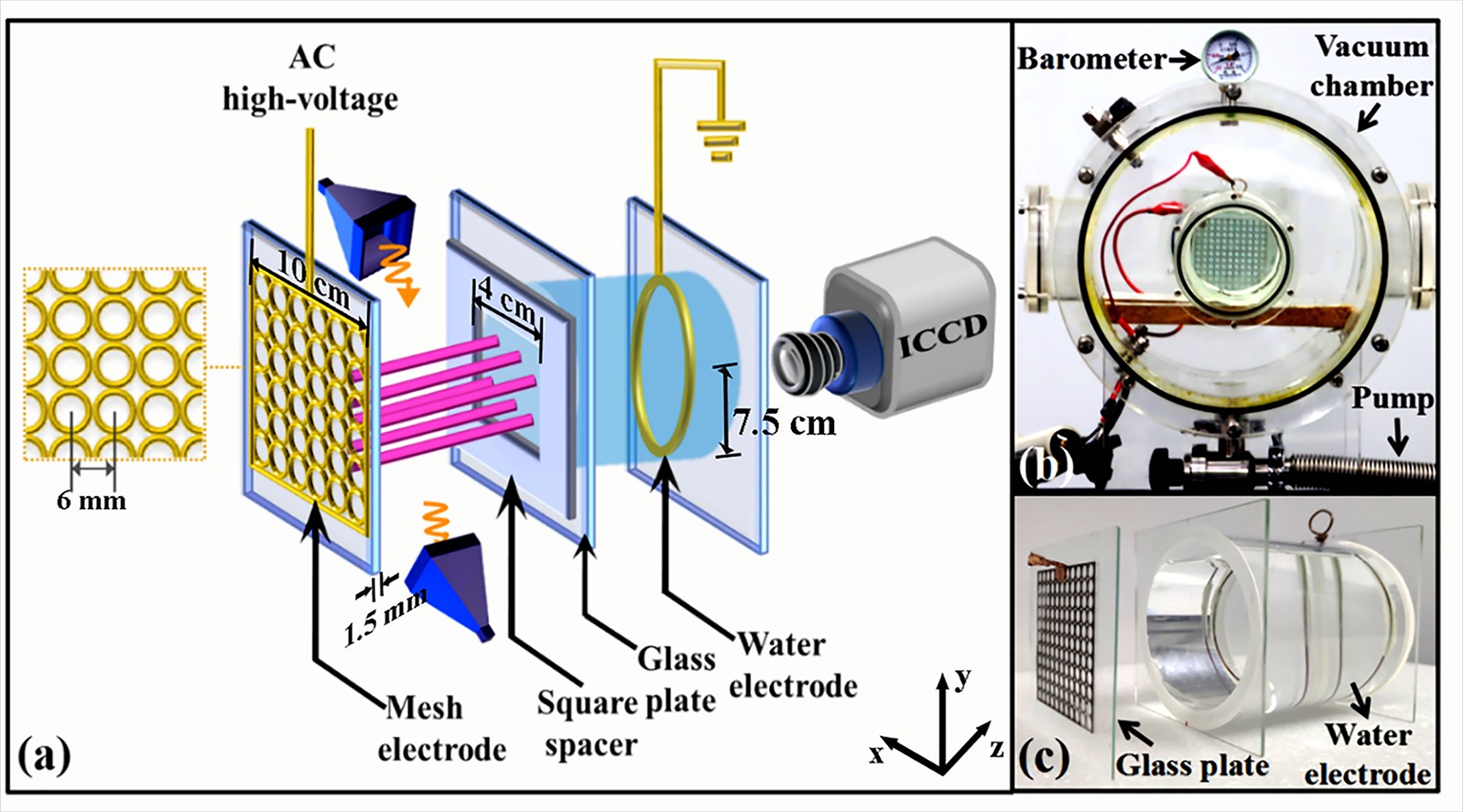
The schematic of the DBD device with mesh-water electrodes is presented in figure 1. A cylindrical container filled with tap water with a diameter of 7.5 cm is employed as the water electrode. Water is also used as a coolant and transparent medium for the detection of discharge filaments. A grounded metal ring is placed in the water. A 10 cm×10 cm metal mesh composed of circular cells is connected to the sinusoidal power supply. The lattice constant a = 6 mm, defined as the distance between the centers of two neighboring circular cells. The metal mesh electrode is covered with a quartz glass layer with a thickness of 1.5 mm. As the lateral discharge boundary, a 2 mm thick square glass plate with the side length of 4 cm is sandwiched between two electrodes. The whole cell is placed in a large vacuum chamber, which could be pumped and filled with gas mixture. The time-resolved measurements of discharges are made using an intensified charge-coupled device camera (ICCD: Andor DH334T) at the end of the water electrode. A trigger realizes the synchronization between the discharge current waveform and ICCD camera. The current and voltage waveforms are respectively obtained via a current probe (Tektronix TCP0030A) and a high-voltage probe (Tektronix P6015A 1000×), recorded by an oscilloscope (Tektronix TDS3054B). Images of plasma lattices are taken by a camera (Canon EOS 6D).
Microwave diagnostics are performed to detect the electromagnetic transmission characteristics of plasma lattices. The microwaves are excited at a vector network analyzer (Rohde & Schwarz ZNB40, 100 kHz–40 GHz) and launched from a broad-band horn antenna (26.5–40 GHz, XB-GH28-20K). It transmits through the aperture with an opening of 4 cm×8 mm adjacent to the PPC. A pyramidal horn antenna receiver (26.5–40 GHz, XB-GH28-20K) is placed on the other side. The transverse magnetic (TM) mode of the microwave is employed. The distance between the transmitting and receiving antennas is about 30 cm. The positions of band gaps can be surmised via the transmission spectra S21.
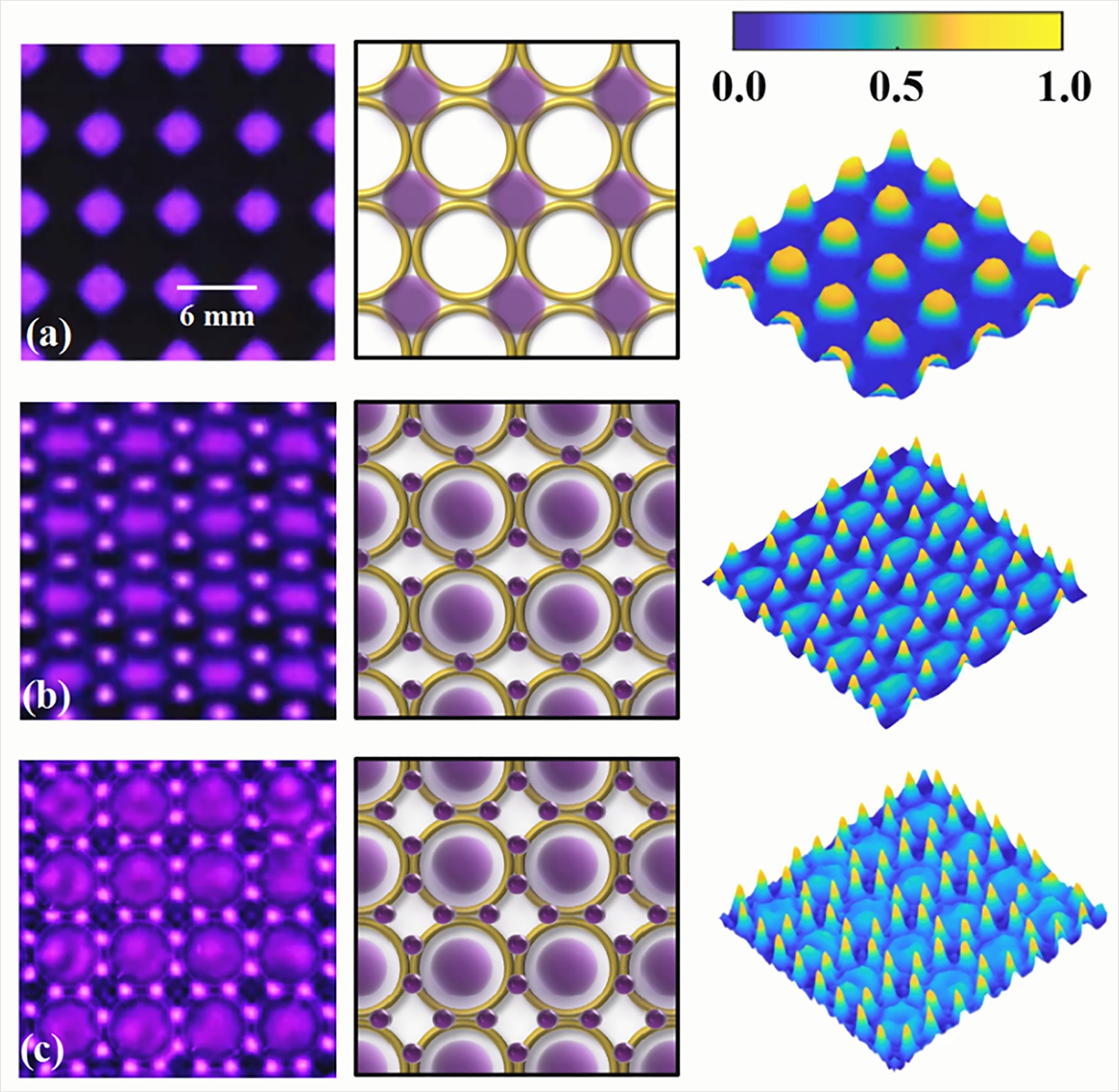
Figure 2 illustrates the reconfiguration of the simple square lattice – (4, 62) Archimedean superlattice – (4, 82) Archimedean superlattice with increasing applied voltage. At U = 4.2 kV, the filaments at the four corners of each circular cell are ignited, forming a square structure with a lattice constant a = 6 mm (figure 2(a)). These filaments exhibit large sizes with radius r = 2 mm. At U = 5.2 kV, (4, 62) Archimedean superlattice is produced (figure 2(b)). The bright filaments appear along the border of each cell and arranged into a well-defined (4, 62) Archimedean lattice according to the Grunbaum-Shephard notation. Additionally, localized uniform discharges with lower light intensity appear in the middle of each cell, which form a square lattice. Thus, the (4, 62) Archimedean superlattice results from an interleaving of (4, 62) Archimedean lattice and square lattice, which have entirely different symmetries. Further increasing the applied voltage results in a novel (4, 82) Archimedean superlattice as shown in figure 2(c). Eight bright filaments are ignited along the border of each cell and arranged into a typical (4, 82) Archimedean lattice. Besides, diffuse plasmas almost occupy each circular cell and constitute a square lattice. Consequently, both (4, 62) and (4, 82) Archimedean superlattices possess higher-order local symmetry on base of the primary square lattice. Such composite configurations are predicted to have various advantageous features over simple lattices, such as improved bandgap size, negative refraction, and left-handedness [1–5]. The plasma lattices are often quite stable and can survive for a long time unless the discharge parameters have been varied. It is also reversible with great repeatability, changing from (4, 82) Archimedean superlattice to square lattice as the applied voltage is reduced. The reconfiguration occurs rapidly with a response time of only several seconds. The rapid response offers inspiration for the fabrication of fast-modulated and highly-integrated optical devices. Importantly, all these plasma structures are realized in 100% air without special treatment. This offers a robust strategy for large-scale and low-cost production in practical applications.
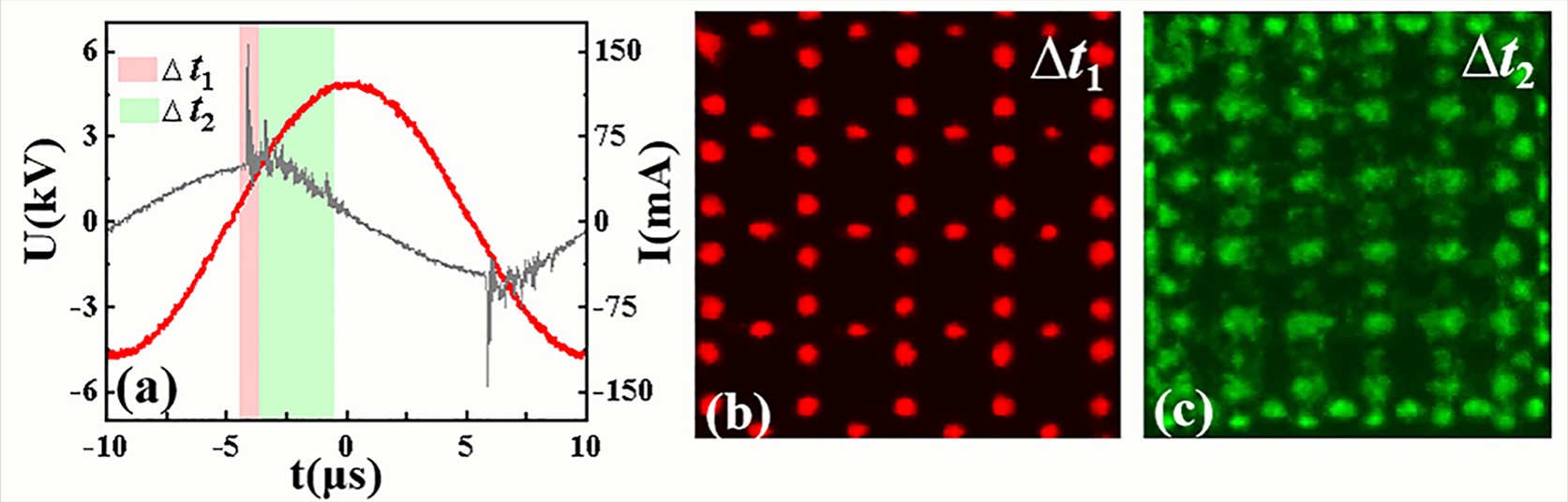
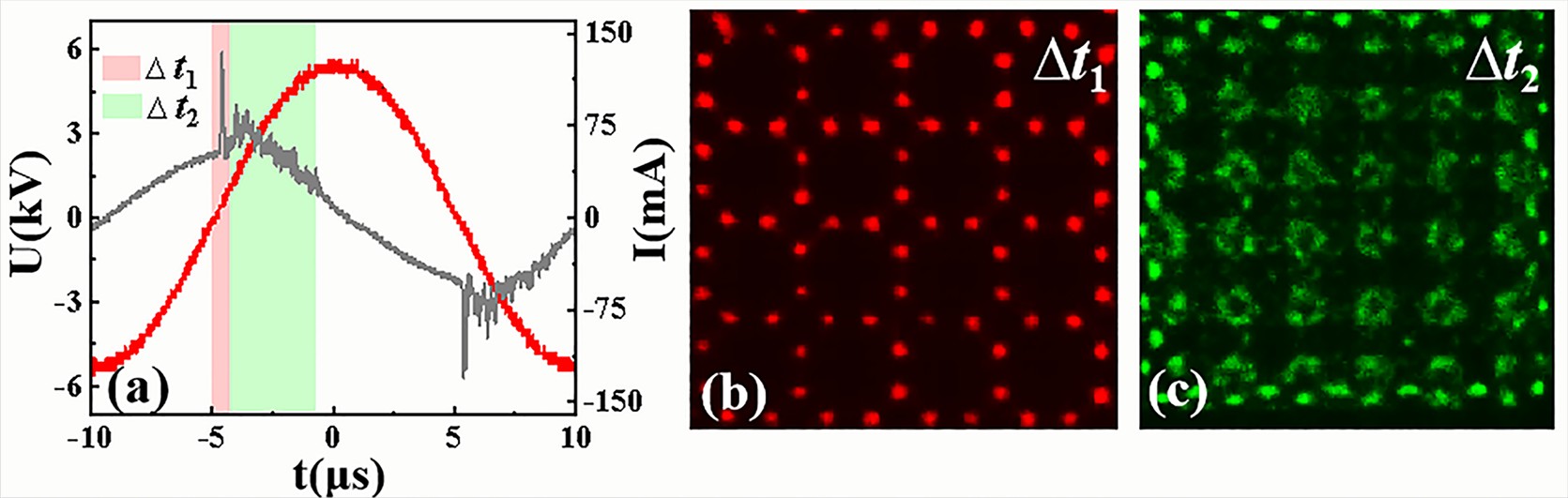
The plasma lattices not only allow for active control in space, but also can be modulated in time. For the simple square lattice depicted in figure 3, there is one single current peak emerging during a half period of applied voltage. It implies that all filaments are concurrently ignited in a time window of 0.47 μs. They repeat the same way in each following half-cycle, with the jitter of current peaks less than 25 ns. Therefore, one can control the formation of lattice temporally by modifying the voltage frequency. Besides, the filaments are produced at the four corners of each unit cell, where the Laplacian potentials are large as shown in figure 4. The distribution of the Laplacian potential is obtained by solving the Poisson equation using the COMSOL Multiphysics software according to the geometry of mesh electrode [31]. For (4, 62) and (4, 82) Archimedean superlattices, they are both time-averaged structures comprised of two instantaneous substructures. Figure 5 presents the spatio-temporally resolved measurements of (4, 62) Archimedean superlattice using a fast camera. In each half-cycle, a leading current peak appears first, followed by a group of stochastic weak peaks. (4, 62) Archimedean lattice appears during the leading peak with ∆t1 = 0.51 μs, while a square lattice composed of large spots appears during the stochastic current peaks. Thus, (4, 62) Archimedean superlattice results from an integration of the transient (4, 62) Archimedean lattice and the square lattice. Similarly, (4, 82) Archimedean superlattice also consists of two distinct sublattices nested with each other. As illustrated in figure 6, (4, 82) Archimedean lattice emerges in a time window ∆t1 = 0.42 μs during the leading current peak, while the square lattice composed of plasma rings is produced during the stochastic peaks. This is much different from the time-averaged structures as we see by naked eyes that diffuse plasmas occupy nearly the whole circular cells. For both (4, 62) and (4, 82) Archimedean superlattices, the two sublattices appear alternatively and repeat the same behavior per each half-cycle of voltage. Their excellent temporal periodicity enables an effective control of the generation of Archimedean superlattices via modulating the voltage frequency. Such spatio-temporally controllable plasma lattices show a variety of applications, including microwave filters, highly sensitive sensors, plasma stealth aircraft, multichannel communication, etc.
The formation mechanism of Archimedean superlattices can be explained by a joint interaction of the space charges, surface charges, and the spatially periodic electric fields produced by the mesh electrode. As an example, figure 7 shows the discharge behaviors of (4, 62) Archimedean superlattice during a half period of applied voltage. The discharge first occurs along the border of each circular cell, leading to the formation of (4, 62) Archimedean lattice at ∆t1 (figure 7(a)). Owing to the presence of dielectric layers, the charged particles will accumulate on the dielectric surface. They establish a surface charge field Es in opposition to the applied electric field Eapp (also referred to Laplacian field). As the applied sinusoidal voltage increases, the electric field in the center of each cell will reach the breakdown threshold. This leads to the formation of large-sized plasma columns that constitute a square lattice. The polarity of the applied voltage is reversed during the next half-cycle, resulting in the same direction of Es and Eapp. Hence, Es will assist the next discharge in the same position as known as the “memory effect”. The surface charge densities of two different plasma sublattices can be estimated by time integration of two current peaks marked by ∆t1, ∆t2 in figure 5(a), respectively, with σ=∫IdtS. For (4, 62) Archimedean lattice, the surface charge density σ1 = 3.53×10−2 C/m2, which is much greater than that of the square lattice σ2 = 8.66×10−3 C/m2. Consequently, the (4, 62) Archimedean lattice (A) is ignited first again in the next half-cycle and then the square sublattice (S), with the order of A-S-A-S in each period, which is harmonic with the applied voltage.
With the reconfiguration from square lattice to (4, 82) Archimedean superlattice, the photonic band diagrams will change significantly. The dispersion relations of PPCs under TM mode have been calculated using COMSOL software based on the Lorentz-Drude model (the detailed theoretical derivation can be found in Appendix A). From Maxwell’s equations, the propagation of electromagnetic (EM) waves in terms of the electric field can be written as,
|
∇×[1μ∇×E(r)]−ε(ωc)2E(r)=0, |
(1) |
where E(r) is the electric field at position r, ω is the angular frequency, μ and ε are the permeability and dielectric constant, respectively. According to Bloch’s theorem, E(r) has the form,
|
E(r)=u(r)exp(ik⋅r)u(r)=u(r+k), |
(2) |
where u(r) represents the periodic function of the lattice, k is the wave vector. Figure 8 displays the schematics of different plasma lattices and the corresponding irreducible Brillouin zones. They consist of cylindrical plasma rods embedded in a gas background with dielectric constant εa = 1. The plasma dielectric constant is given as
|
εp(ω)=1−ω2peω(ω+iνm). |
(3) |
Here νm denotes the electron-neutral elastic collision frequency which is determined by the gas composition and pressure [32, 33]. The plasma frequency ωpe is given as
|
ωpe=√nee2meε0. |
(4) |
ne denotes the electron density that scales with the applied voltages and gas pressure, which normally ranges from 1013 to 1015 cm−3 (calculation of ne and νm can be found in Appendix B). A free triangular mesh is selected in the simulation. The normalized eigenfrequencies (ωɑ/2π) for each wave vector k along the boundary of the irreducible Brillouin zone M-Γ-X-M have been calculated and the band structures can be obtained (simulation process is provided in Appendix C).
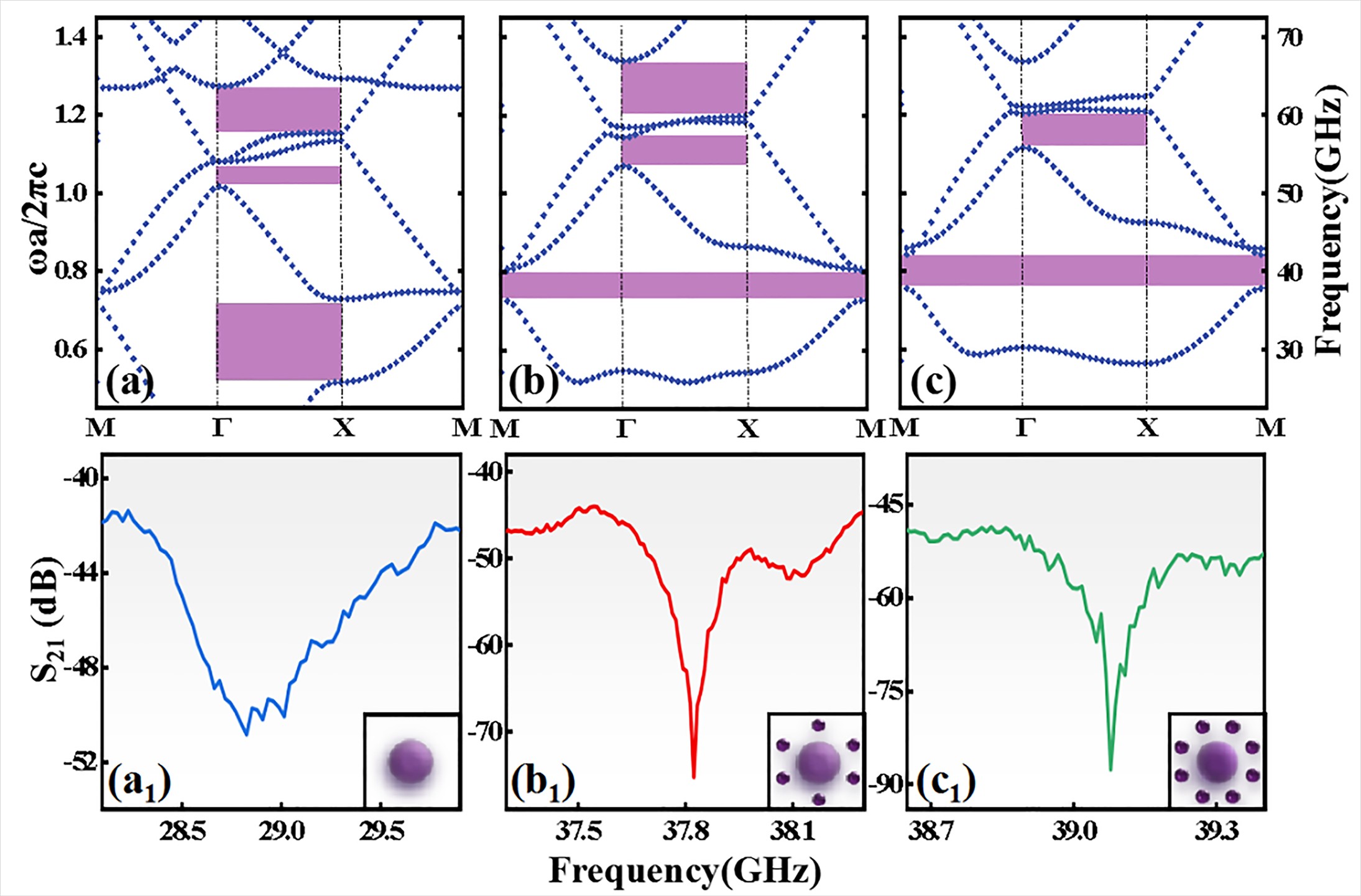
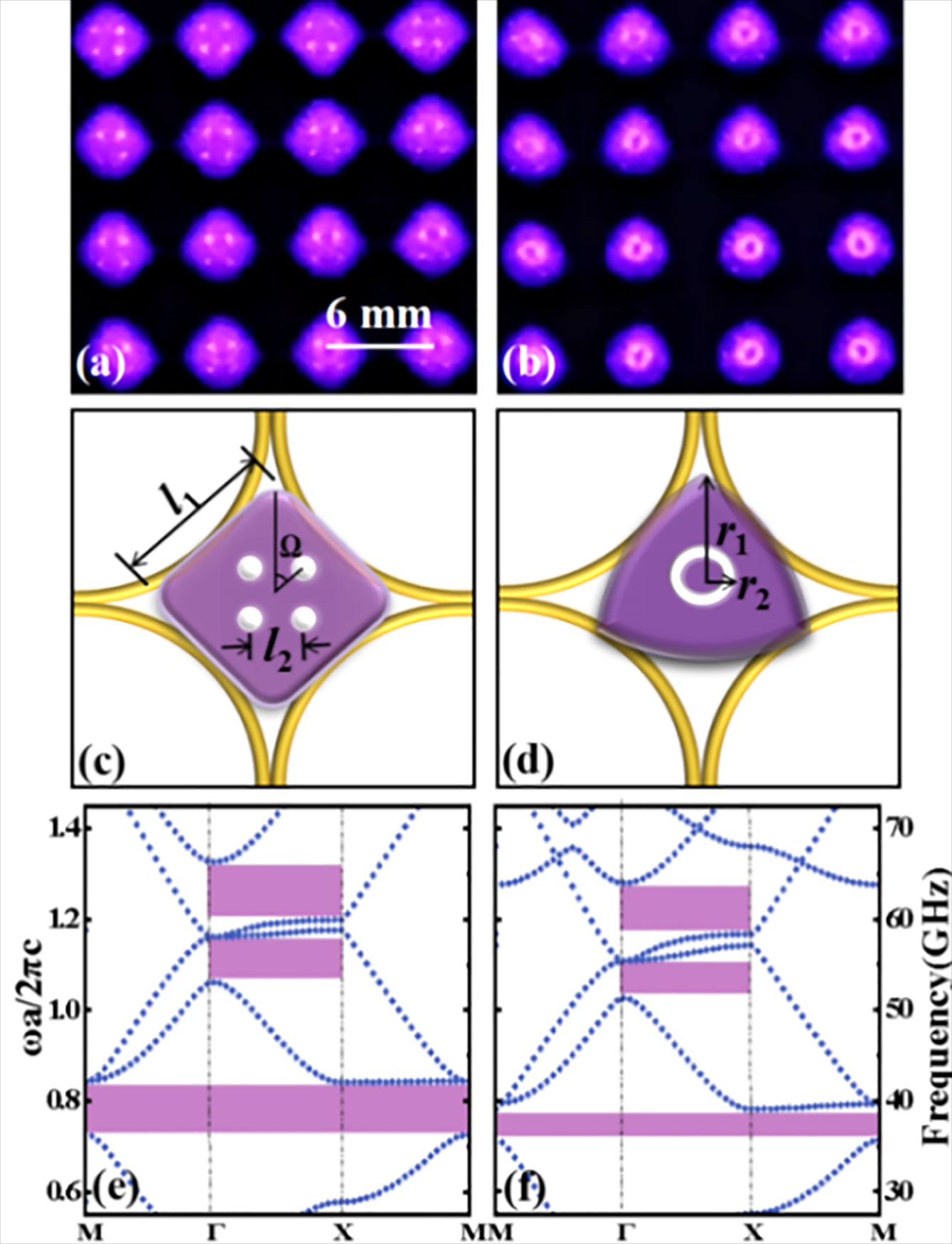
Figure 9 illustrates the numerical and experimental studies on photonic band structures of different plasma lattices. For the simple square lattice, no OBG emerges while multiple unidirectional band gaps (UBGs) are produced at 25.8–30.6 GHz and 57.1–59.1 GHz in Γ-X direction, respectively. In comparison to the simple square lattice, the Archimedean lattices exhibit superior performance. Remarkable OBGs that are regardless of propagation direction can be observed at 37.0–38.5 GHz and 38.1–41.8 GHz for both of (4, 62) and (4, 82) Archimedean superlattices, respectively. The creation of OBGs enables higher efficiency of the Archimedean PPCs. Moreover, the plasma density increases with the reconfiguration from the simple square lattice to Archimedean lattices as the applied voltage increases. As a result, the positions of band gaps upshift towards a higher frequency. This is required in wireless communications in practical applications, since the high-frequency transmission not only can carry more information but also has the features of good direction and strong penetrating power [13]. The simulation results are consistent with the experimental measurements as shown in figures 9(a1)–(c1). It can be seen that the band gaps occur at frequencies of 28.8 GHz, 37.8 GHz and 39.1 GHz, corresponding to the PBGs of simple square lattice, (4, 62) and (4, 82) Archimedean superlattice, respectively. If the frequency of incident microwaves is located in the band gap, for example, f = 37.7 GHz as shown in figure 10(a), the microwaves are attenuated sharply near the first two rows of plasma columns. Similarly to the electronic band gaps in semiconductors, the destructive interference caused by Bragg scattering is responsible for the exponential attenuation of the energy of EM waves in the band gap. Alternatively, if the frequencies are within the pass band, for instance, f = 49.7 GHz, the microwaves could propagate through the whole plasma lattice with low-loss (figure 10(b)). Obviously, the Archimedean geometry provides an effective route to yield OBGs.
We realize an active control on the crystal orientation of (4, 62) Archimedean superlattice as presented in figure 11. With the increase of applied voltage, the crystal orientation changes π/6 as indicated by the dashed hexagons. Intriguingly, a critical state with coexistence of two different crystal orientations has been observed (figure 11(b)). This can potentially be used as a heterostructure, which is predicted to have unique potential applications [34]. Large OBGs can be obtained using a 2D photonic crystal heterostructure [35]. An efficient multiple-channeled optical filtering has been realized by introducing heterostructure defects [36, 37]. Asymmetric transmission device (ATD) as well as a PC switch can also be fabricated based on heterostructure waveguides [38]. Qualitatively, changing the crystal orientation has a comparable impact with altering the incident angle of EM waves, which will significantly influence the transmission characteristics. In previous studies, the orientation of PCs is normally adjusted by mechanically rotating the entire PCs. Here, we present an efficient method for dynamically tuning the crystal orientation of Archimedean lattices by simply changing the applied voltage.
An additional control on the fine structures of plasma elements (microstructures in small scale) is also achieved, besides of the symmetry and crystal orientation described above (macrostructures in large scale). Figure 12 presents two new types of square lattice, in which each plasma element is a composite of multifarious fine structures. For the square lattice-I (figure 12(a)), the plasma elements have a square geometry, with another smaller square composed of bright filaments located in the middle. The side-length ratio of two squares l1/l2 = 2.0, and the rotation angle Ω = 45°. As the gas pressure and applied voltage increase, the square lattice-II emerges (figure 12(b)). Each element has a reuleaux triangular geometry with the appearance of a bright circular ring in the center with the radius ratio r1/r2 = 2.5. The presence of composite plasma elements yields a great improvement in the band gap size as shown in figures 12(e) and (f). Remarkable OBGs can be obtained for both the square lattices I and II, located at 37–42 GHz and 36–38 GHz, respectively. By contrast, no OBG is obtained for the simple square counterpart as shown in figure 9. It is believed that the formation of composite plasma structures results from a combined effect of self-organization and artificial lattice modulation induced by the mesh electrode. As seen in the figure, the composite plasma elements are confined in polygons surrounded by four circular cells of the mesh electrode, given a constrained symmetry and lattice constant. On the other hand, the filaments self-organize into different fine structures in each element and thus modify the fundamental structures of PPCs. Its novel architecture provides a significant route for yielding large band gaps and may lead to new applications such as low-threshold lasers, quantum cascade laser, high-Q photonic crystal nanocavities, etc.
In summary, we have experimentally demonstrated the generation of spatial-temporally controllable Archimedean superlattices in DBD with meshed-liquid electrodes. Fast reconstruction among the simple square lattice, (4, 62) Archimedean superlattice and (4, 82) Archimedean superlattice has been realized in 100% air by readily changing the applied voltage. A new type of square lattice composed of different composite plasma elements is proposed. In time, the plasma lattices are time-averaged structures comprised of multiple dynamic sublattices that have good temporal periodicity. This provides a possibility to temporally control the generation of Archimedean superlattices by modulating the voltage frequency. In space, both the macrostructures of Archimedean superlattices including the lattice symmetry and crystal orientation, and the microstructures including the fine structures of plasma elements can be tuned actively. In addition, the photonic band diagrams of various PPCs are studied by means of the finite-element method. The simulation results are well consistent with the experimental measurements. Remarkable OBG located in the range of 36.7–40.2 GHz is obtained for Archimedean superlattices. Our work provides an effective method to create reconfigurable Archimedean plasma lattices, which may have broad application prospects in the manipulation of terahertz waves and microwaves, such as multi-channel optical switches, plasma lenses, cloaking, and wireless communications. Further studies of the interaction between different Archimedean plasma lattices and EM waves are our ongoing efforts.
Data included in this study are available upon request by contact with the authors.
The propagation of electromagnetic waves in photonic crystals can be described by Maxwell’s equations, which have the form given below (suppose that there is no current or electric charge):
|
∇⋅B=0∇×E+∂B∂t=0∇⋅D=0∇×H−∂D∂t=0, |
(A1) |
where E and H represent the electric and magnetic fields of electromagnetic waves, which can be expressed as:
|
E(r,t)=E(r)exp(−iωt)H(r,t)=H(r)exp(−iωt), |
(A2) |
D and B represent the electric displacement vector and magnetic induction intensity, respectively. Assuming that the medium is isotropic, they can be expressed as:
|
D=εE=ε0ε(r)EB=μH=μ0μ(r)H. |
(A3) |
Here ε and μ denote the dielectric constant and permeability of materials, ε0 and μ0 denote the vacuum permittivity and permeability of vacuum, ε(r) and μ(r) are the relative permittivity and relative permeability, respectively. Substituting equations (A2) and (A3) into equation (A1), Maxwell’s equations can be reduced to the following equations:
|
∇×[1μ∇×E(r)]−ε(ωc)2E(r)=0, |
(A4) |
|
∇×[1ε∇×H(r)]−μ(ωc)2H(r)=0. |
(A5) |
Equations (A4) and (A5) are the eigen equations corresponding to the transverse magnetic (TM) mode and transverse electric (TE) modes, respectively. In this work, the electromagnetic transmission characteristics under TM mode have been considered. According to Bloch’s theorem, the eigenvector E(r) has the form
|
E(r)=u(r)exp(ik⋅r)u(r)=u(r+k), |
(A6) |
where u(r) represents the periodic function of the lattice, k is the wave vector. Substituting equation (A6) into equation (A4), the normalized eigenfrequencies (ωɑ/2π) for each wave vector k along the boundary of the irreducible Brillouin zone M-Γ-X-M can be calculated and the band structure diagram can be obtained.
The plasma frequency and collision frequency are key parameters affecting the microwave transmission characteristics. They can be determined by the following method:
(I) The plasma frequency ωpe is given by equation (4). Two methods are generally used to measure the electron density ne in our experiment, which are the emission spectral method and the current integration method, respectively. The emission spectral method is based on Stark broadening, which is suitable for the discharges with high gas pressures and high electron densities (generally higher than 1020 or 1021 m−3). For the discharges with low gas pressures as demonstrated in this manuscript, ne can be estimated by the transferred electric charges during the discharge current pulse, which has the form
|
ne=QeSd, |
(B1) |
where e is the elementary charge, S is the area of instantaneous filaments, and d denotes the distance of discharge gas gap. Q is the averaged transferred electric charges calculated by integration of different current pulses (Q=∫Idt). For instance, the (4, 62) Archimedean superlattice results from the superposition of a (4, 62) Archimedean sublattice and a simple square sublattice, which are ignited during the first current pulse and second current pulse, respectively (figures 5(b) and (c)). Therefore, the integration interval is within ∆t1 for (4, 62) Archimedean sublattice and ∆t2 for the simple square sublattice. The specific parameters have been calculated and listed in table B1.
| Q (nC) | r (mm) | ne (cm−3) | |
| (4, 62) Archimedean sublattice | 11.9 | 0.20 | 3.94×1014 |
| Square sublattice | 15.9 | 1.03 | 4.96×1013 |
Based on the measured plasma densities, the plasma frequencies can be obtained correspondingly. For instance, if ne = 3.94×1014 cm−3, the plasma frequency ωpe = 1.1×1012 rad s−1 according to equation (4).
(II) The values of the electron-neutral elastic collision frequency νm can be calculated based on the empirical relations [32, 33]:
|
νm(air)=(3.39×109s−1Torr−1)p, |
(B2) |
|
νm(Ar)=1.56×107p√Te, |
(B3) |
where p is the gas pressure. When the experimental gas is a mixture of air and argon, we will perform weighted calculation based on the proportion of the two gases.
The dispersion relations of PPCs are calculated using COMSOL software based on the finite-element method. The main simulation process can be concluded as follows:
(1) Select the model. Based on the real structural configurations of the PPCs obtained in the experiment, the electromagnetic wave frequency domain model in the wave optics module is selected in a 2D space and solved using the built-in partial differential equations.
(2) Set the parameters in global definitions module. We set the parameters required for the geometric model (lattice constant, geometric radius of plasma elements, etc.) as well as the material property parameters (electron density, collision frequency, plasma frequency, etc.) into the model.
(3) Define the irreducible Brillouin zone boundary using functions. The equation for wave vector k is set up by adding functions - segments for subsequent solving.
(4) Define different domains in terms of the material properties. The material properties (relative dielectric constant, conductivity, etc.) are assigned to the materials of different domains so as to distinguish the air and plasmas in different sublattices. Here, the different materials can be described by different wave equations.
(5) Set the boundary conditions. According to the spatial symmetry of plasma lattice, the Floquet boundary conditions are applied to the computational domain boundary.
(6) Divide the grid. We set appropriate mesh size for the computational domain and discretize the region. In our model, a free triangular mesh has been selected with appropriate curvature factor and maximum cell growth rate. Here, we set the curvature factors to 0.25 and the maximum cell growth rate to 1.
(7) Set up a solver. A parameter scanning solver is added to the model, whose specific parameters can be modified such as solution step size, number of eigenfrequencies, etc. The eigenfrequencies corresponding to each wave vector k along the irreducible Brillouin zone boundary which has been set in step (3) will be calculated.
(8) Post processing. The exported calculation data will be post-processed using drawing software.
This work was supported by National Natural Science Foundation of China (Nos. 12275065 and 11975089); Natural Science Foundation of Hebei Province (Nos. A2021201010 and A2021201003); Interdisciplinary Research Program of Natural Science of Hebei University (No. DXK202108); Hebei Provincial Central Government Guiding Local Science and Technology Development Funds (No. 236Z1501G); Scientific Research and Innovation Team Foundation of Hebei University (No. IT2023B03); The Excellent Youth Research Innovation Team of Hebei University (No. QNTD202402) and Regional Key Projects of National Natural Science Foundation of China (No. U23A20678).
| [1] |
Ueda K, Dotera T and Gemma T 2007 Phys. Rev. B 75 195122 doi: 10.1103/PhysRevB.75.195122
|
| [2] |
Suding P N and Ziff R M 1999 Phys. Rev. E 60 275 doi: 10.1103/PhysRevE.60.275
|
| [3] |
Mao Q P et al 2016 Appl. Opt. 55 B139 doi: 10.1364/AO.55.00B139
|
| [4] |
Gajić R et al 2008 Opt. Mater. 30 1065 doi: 10.1016/j.optmat.2007.05.024
|
| [5] |
Stelson A C, Britton W A and Liddell Watson C M 2017 J. Appl. Phys. 121 023101 doi: 10.1063/1.4973472
|
| [6] |
Zhang H F, Liu S B and Jiang Y C 2014 Phys. Plasmas 21 092104 doi: 10.1063/1.4894213
|
| [7] |
David A et al 2006 Appl. Phys. Lett. 88 073510 doi: 10.1063/1.2168673
|
| [8] |
Bergenek K et al 2008 Appl. Phys. Lett. 93 41105 doi: 10.1063/1.2963030
|
| [9] |
Meng F et al 2017 J. Lightw. Technol. 35 1670 doi: 10.1109/JLT.2017.2667681
|
| [10] |
Bagci F and Akaoglu B 2012 Opt. Commun. 285 1486 doi: 10.1016/j.optcom.2011.10.035
|
| [11] |
Shi P, Huang K and Li Y 2012 Opt. Commun. 285 3128 doi: 10.1016/j.optcom.2012.02.069
|
| [12] |
Guo B 2013 Phys. Plasmas 20 074504 doi: 10.1063/1.4816808
|
| [13] |
Sun P P et al 2019 Appl. Phys. Rev. 6 041406 doi: 10.1063/1.5120037
|
| [14] |
Gregório J, Parsons S and Hopwood J 2017 Plasma Sources Sci. Technol. 26 02LT03 doi: 10.1088/1361-6595/26/2/02LT03
|
| [15] |
Zhang W D et al 2020 Phys. Plasmas 27 063508 doi: 10.1063/1.5134560
|
| [16] |
Tan H Y et al 2018 IEEE Trans. Plasma Sci. 46 539 doi: 10.1109/TPS.2018.2795613
|
| [17] |
Sakai O, Naito T and Tachibana K 2010 Phys. Plasmas 17 057102 doi: 10.1063/1.3314334
|
| [18] |
Ramezani A H, Fathollahi Khalkhali T and Moghadam M R 2018 J. Opt. 47 489 doi: 10.1007/s12596-018-0478-6
|
| [19] |
Li J F et al 2023 Nanophotonics 12 1847 doi: 10.1515/nanoph-2022-0800
|
| [20] |
Fan W L et al 2022 APL Photonics 7 116105 doi: 10.1063/5.0109016
|
| [21] |
Fan W L et al 2019 Phys. Rev. Appl. 11 064057 doi: 10.1103/PhysRevApplied.11.064057
|
| [22] |
Liu F C et al 2022 Plasma Sources Sci. Technol. 31 025015 doi: 10.1088/1361-6595/ac4dde
|
| [23] |
Rao S S et al 2022 Ann. Phys. 534 2200337 doi: 10.1002/andp.202200337
|
| [24] |
Sui J Y et al 2023 Ann. Phys. 535 2200661 doi: 10.1002/andp.202200661
|
| [25] |
Rao S S et al 2021 J. Phys. D: Appl. Phys. 54 455205 doi: 10.1088/1361-6463/ac15d2
|
| [26] |
Yao J F et al 2016 Phys. Plasmas 23 083524 doi: 10.1063/1.4961233
|
| [27] |
Kamboj G K, Yadav R P and Kaler R S 2021 Phys. Plasmas 28 053509 doi: 10.1063/5.0030425
|
| [28] |
Iwai A et al 2020 Phys. Plasmas 27 023511 doi: 10.1063/1.5112077
|
| [29] |
Li J F et al 2023 APL Photonics 8 066102 doi: 10.1063/5.0149985
|
| [30] |
Wang B et al 2021 Phys. Plasmas 28 043502 doi: 10.1063/5.0043336
|
| [31] |
Wang J et al 2020 Phys. Plasmas 27 073503 doi: 10.1063/5.0009695
|
| [32] |
Matlis E H et al 2018 J. Appl. Phys. 124 093104 doi: 10.1063/1.5037469
|
| [33] |
Ginzburg V L 1970 The Propagation of Electromagnetic Waves in Plasmas 2nd ed (Oxford: Pergamon
|
| [34] |
Hu S Y et al 2022 Front. Mater. 9 843265 doi: 10.3389/fmats.2022.843265
|
| [35] |
Wang L et al 2004 J. Appl. Phys. 95 424 doi: 10.1063/1.1634367
|
| [36] |
Gao H et al 2019 J. Opt. 48 393 doi: 10.1007/s12596-019-00541-1
|
| [37] |
Wang F et al 2021 AIP Adv. 11 095013 doi: 10.1063/5.0061986
|
| [38] |
Ren C, Kang F and Cao D Z 2019 Opt. Commun. 437 75 doi: 10.1016/j.optcom.2018.12.053
|
| [1] | Liting GUO, Yuyang PAN, Guanglin YU, Zhaoyang WANG, Kuangya GAO, Weili FAN, Lifang DONG. Controllable and tunable plasma photonic crystals through a combination of photonic crystal and dielectric barrier discharge patterns[J]. Plasma Science and Technology, 2023, 25(8): 085501. DOI: 10.1088/2058-6272/acb52b |
| [2] | Haifeng ZHANG (章海锋), Hao ZHANG (张浩). The features of band structures for woodpile three-dimensional photonic crystals with plasma and function dielectric constituents[J]. Plasma Science and Technology, 2018, 20(10): 105001. DOI: 10.1088/2058-6272/aacf87 |
| [3] | Pan CHEN (陈攀), Jun SHEN (沈俊), Tangchun RAN (冉唐春), Tao YANG (杨涛), Yongxiang YIN (印永祥). Investigation of operating parameters on CO2 splitting by dielectric barrier discharge plasma[J]. Plasma Science and Technology, 2017, 19(12): 125505. DOI: 10.1088/2058-6272/aa8903 |
| [4] | Fangmin HUANG (黄芳敏), Zhouyang LONG (龙洲洋), Sa LIU (刘飒), Zhenglong QIN (秦正龙). Dielectric barrier discharge plasma pretreatment on hydrolysis of microcrystalline cellulose[J]. Plasma Science and Technology, 2017, 19(4): 45504-045504. DOI: 10.1088/2058-6272/aa4c20 |
| [5] | ZHANG Kaiming (张开明), SUN Dongsheng (孙东升). The Photonic Band Gaps in the Two-Dimensional Plasma Photonic Crystals with Rhombus Lattice[J]. Plasma Science and Technology, 2016, 18(6): 583-589. DOI: 10.1088/1009-0630/18/6/01 |
| [6] | QI Limei (亓丽梅), LI Chao (李超), FANG Guangyou (方广有), GAO Xiang (高翔). The Absorbing Properties of Two-Dimensional Plasma Photonic Crystals[J]. Plasma Science and Technology, 2015, 17(1): 4-9. DOI: 10.1088/1009-0630/17/1/02 |
| [7] | NIU Jinhai(牛金海), ZHANG Zhihui(张志慧), FAN Hongyu(范红玉), YANG Qi(杨杞), LIU Dongping(刘东平), QIU Jieshan(邱介山). Plasma-Assisted Chemical Vapor Deposition of Titanium Oxide Films by Dielectric Barrier Discharge in TiCl 4 /O 2 /N 2 Gas Mixtures[J]. Plasma Science and Technology, 2014, 16(7): 695-700. DOI: 10.1088/1009-0630/16/7/11 |
| [8] | S. PRASAD, Vivek SINGH, A. K. SINGH. Study on the Reflection Spectra of One Dimensional Plasma Photonic Crystals Having Exponentially Graded Materials[J]. Plasma Science and Technology, 2013, 15(5): 443-447. DOI: 10.1088/1009-0630/15/5/10 |
| [9] | Laxmi Shiveshwari. Some New Band Characteristics in One-Dimensional Plasma Dielectric Photonic Crystals[J]. Plasma Science and Technology, 2011, 13(4): 392-396. |
| [10] | Vadim Yu. PLAKSIN, Oleksiy V. PENKOV, Min Kook KO, Heon Ju LEE. Exhaust Cleaning with Dielectric Barrier Discharge[J]. Plasma Science and Technology, 2010, 12(6): 688-691. |
| Q (nC) | r (mm) | ne (cm−3) | |
| (4, 62) Archimedean sublattice | 11.9 | 0.20 | 3.94×1014 |
| Square sublattice | 15.9 | 1.03 | 4.96×1013 |