| Citation: | Yifei ZHAO, Yueqiang LIU, Guangzhou HAO, Zhengxiong WANG, Guanqi DONG, Shuo WANG, Chunyu LI, Guanming YANG, Yutian MIAO, Yongqin WANG. Loss of energetic particles due to feedback control of resistive wall mode in HL-3[J]. Plasma Science and Technology, 2024, 26(10): 104002. DOI: 10.1088/2058-6272/ad547e |
Effects of three-dimensional (3D) magnetic field perturbations due to feedback control of an unstable n=1 (n is toroidal mode number) resistive wall mode (RWM) on the energetic particle (EP) losses are systematically investigated for the HL-3 tokamak. The MARS-F (Liu et al 2000 Phys. Plasmas 7 3681) code, facilitated by the test particle guiding center tracing module REORBIT, is utilized for the study. The RWM is found to generally produce no EP loss for co-current particles in HL-3. Assuming the same perturbation level at the sensor location for the close-loop system, feedback produces nearly the same loss of counter-current EPs compared to the open-loop case. Assuming however that the sensor signal is ten times smaller in the close-loop system than the open-loop counter part (reflecting the fact that the RWM is more stable with feedback), the counter-current EP loss is found significantly reduced in the former. Most of EP losses occur only for particles launched close to the plasma edge, while particles launched further away from the plasma boundary experience much less loss. The strike points of lost EPs on the HL-3 limiting surface become more scattered for particles launched closer to the plasma boundary. Taking into account the full gyro-orbit of particles while approaching the limiting surface, REORBIT finds slightly enhanced loss fraction.
The research on long air gap discharge is of great significance for the insulation design and lightning protection of ultra-high voltage (UHV) transmission lines [1–4]. Leader propagation is a crucial phase in long air gap discharge. While it is commonly considered that positive leader propagation is continuous, researches have shown that under conditions of slowly varying impulse voltages or high atmospheric humidity, leader propagation can be discontinuous. The period when ionization activity in the discharge channel vanishes is called the dark period [5]. Following the end of dark period, positive leader propagation is characterized by abrupt brightening and elongation of the leader channel, a phenomenon known as re-illumination [5, 6]. Due to the randomness and complexity of leader re-illumination, there is currently no consensus regarding the mechanism of leader re-illumination [7]. Therefore, experiments are necessary to observe the characteristics of leader re-illumination.
Among multiple experimental observation methods, the optical image observation is the most intuitive method to understand the propagation characteristics of the whole discharge, which has been widely utilized in the acquisition of crucial physical parameters during the discharge development [8–12]. In order to observe the development of leader, Zhou et al conducted a discharge experiment in a 6.1 m transmission tower gap and captured the propagation of leaders using a high-speed camera [13]. In the experiment, the leader re-illumination occasionally occurred during continuous propagation, and the velocity of the leaders in re-illumination mode was much higher than that of continuous propagation. But the researcher did not explore the optical and morphological characteristics of the leaders in further detail. Under the condition of absolute humidity of 15.5–16.5 g/m3, Chen et al first discovered two types of streamers at the head of the leaders in re-illumination mode: “diffuse type” and “branch type” [14]. They speculated that the “branch type” streamer might be caused by high humidity. In literature [15], Kostinskiy et al used a two-frame, high-speed video camera with image enhancement to observe the properties of positive and negative leaders developing in 4–10 m air gaps. They found that in positive leader re-illumination mode, the morphological structures of the streamers resemble those of the negative leader. However, due to the limitation of the camera frame rate, they did not carry out further research on this observation. While researchers have observed some morphological structures of the leader-streamer system, the re-illumination happened by accident during the continuous leader propagation in the experiments, resulting in a lack of more comprehensive and coherent observation results of the whole leader propagation process in re-illumination mode.
Given the particularity of the dark period compared with the continuous propagation leader, other scholars have also studied the gas temperature evolution of leader channels during the dark period in detail, to better understand the re-illumination phenomenon. Zhao et al proposed using quantitative Schlieren techniques to accurately measure the temperature of leader discharge channels [16]. They set up a calibrated Toepler’s lens-type Schlieren system to measure the radial temperature profile of leader discharge channels and gave guidance on the use of quantitative Schlieren techniques. Some researchers investigated the decay and reactivation of positive leaders in long air gap discharges by an improved time resolved quantitative Schlieren observation system [17]. They found that when a positive leader begins to decay, the temperature drops below 3000 K. Besides, it is possible for a decaying leader to be reactivated before it transforms into an aborted leader and the temperature drops below 2000 K. Liu et al observed optical and thermal characteristics of discharge channels during dark periods by an integrated experimental platform. They noted weak luminosity of ionization activity in the leader channels during the dark period and observed a narrow thermal channel which may facilitate the subsequent re-illumination [18]. The above researches provide a detailed observation of the thermal evolution of leader channels during the dark period, deepening our understanding of the streamer-leader transition process. Unfortunately, due to the lack of corresponding refined and comprehensive optical images of the leader structures in re-illumination mode, the research results about streamer-leader transition have not been self-consistent.
In summary, in previous experiments, re-illumination events occurred accidentally during continuous leader propagation, making it challenging to observe leader structures carefully. Furthermore, previous studies primarily focused on the causes of dark period and the thermal evolution of leader channels during the dark period, without establishing a connection between the morphological characteristics of the leader-streamer system and the process of streamer-leader transition.
To comprehensively and meticulously observe the leader morphology in re-illumination mode, we conducted long sparks experiments in a 10 m UHV transmission line gap under 3376 kV switching impulse voltages of long wave front time (1000/5000 μs) in a high humidity environment (20.0–30.1 g/m3). In this environment, the positive leaders predominantly propagated discontinuously, with almost no significantly continuous propagation occurring. Discharge current and voltage waveforms and optical morphological images of the leader-streamer system were captured by a comprehensive observation platform. Based on the analysis of the leader-streamer system characteristics, we proposed a hypothesis about streamer-leader transition in leader re-illumination mode.
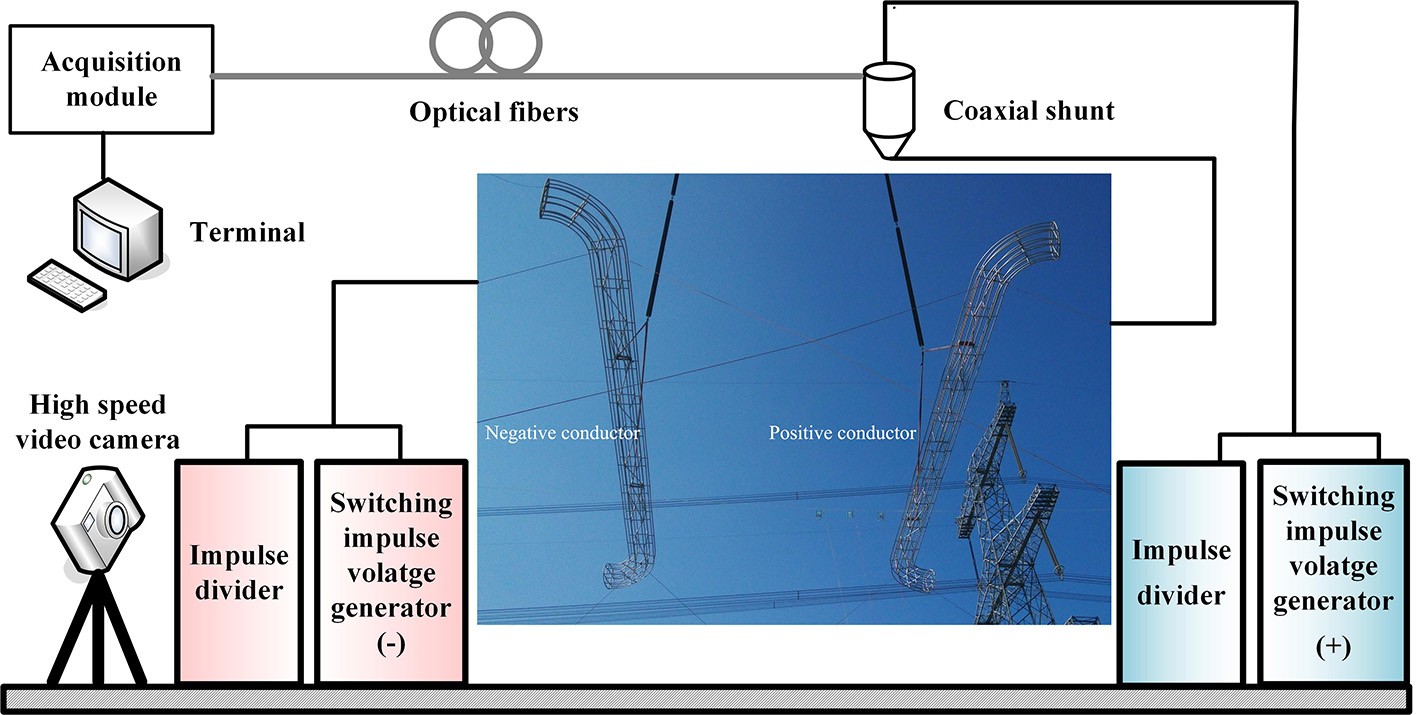
The comprehensive observation platform for long air gap discharge comprises an impulse voltage measurement system, a current measurement system, and a discharge optical morphology imaging system. Impulse voltage measurement is conducted using an impulse voltage divider, while discharge current measurement utilizes a coaxial shunt. The resistance of the coaxial shunt is 0.1 Ω. A CMOS (complementary metal oxide semiconductor) high-speed camera captures time-resolved images of the discharge process. In this experiment, the shooting frame rate of CMOS high-speed camera was set at 76086 fps, with a frame interval of 13.14 μs, an exposure time of 12.36 μs per frame, and an image size of 352×168 pixels. A schematic diagram of the comprehensive observation platform is shown in figure 1.
In the experiment, two eight-bundled conductors were used, with a distance of 400 mm between adjacent sub-conductors and a diameter of 32 mm for the sub-conductors. These two conductors were arranged in parallel with an inter-phase gap of 10 m.
Figure 2 depicts the applied long wave front time impulse voltages (1000/5000 μs). Given that the distance between the conductors and the ground is only 20 m, in order to reduce the probability of breakdown between the conductors and the ground, we simultaneously applied positive impulse voltage to the positive conductor and negative impulse voltage to the negative conductor. The positive impulse voltage has an amplitude of 2010 kV, while the negative impulse voltage is 1366 kV, resulting in a total voltage amplitude of 3376 kV. The negative impulse voltage accounts for 40% of the total voltage amplitude. Throughout the experiment, the ambient temperature ranged between 32 °C and 34 °C, with an absolute humidity level of 20.0–30.1 g/m3. The air pressure at the test site is 99.5–100.5 kPa, approximately equivalent to 1 atm.
The current and voltage waveforms of a typical discharge process are depicted in figure 3 (the letter labels in the figure correspond to the time intervals in figure 4). Positive and negative impulse voltages were initiated at 0 μs. Around 185 μs, the first notable current pulse emerged, signifying the formation of a leader (not the leader resulting in the final breakdown). The interval between two current pulses represents the dark period. Subsequently, multiple significant current pulses occurred, indicating a large number of electrons suddenly injecting into the leader channel, likely associated with the leader re-illumination. Approximately at 545 μs, the current sharply increased and then remained at about 10 A, indicating the final jump stage and subsequent arc-burning stage respectively.
Throughout the discharge process, the current mainly manifested as pulses exceeding 5 A, with no steady current. In contrast, during continuous leader propagation, the current is steady, typically ranging from 0.5 to 2 A [19]. Consequently, the leaders in the experiment mainly propagated discontinuously, which is completely different from the accidental leader re-illumination that occurs during continuous leader propagation in other experiments.
The typical discharge images of a gap breakdown process, which correspond to the current and voltage waveforms in figure 3, are shown in figure 4. In figure 4(a), the weak discharge was observed, corresponding to the initiation of the stable leader that led to the final breakdown. Subsequently, there was an obvious leader re-illumination, and the generation of streamers corresponded to the current pulse in figure 3(b). Then the leader propagated in a re-illumination mode, with each elongation corresponding to the current pulse in figures 3(c) and (d). In figure 4(e), it is evident that the streamers at the front of the leader reached the negative polarity conductor, marking the beginning of the final jump stage. Immediately afterwards, with the breakdown of the inter-phase gap, strong light emission was observed, followed by arc formation. Notably, the leader channel exhibits an intensely luminous and thick appearance, with each elongation segment being considerably straight and long. The characteristics differ significantly from those when the leader propagates continuously in a low humidity environment (4.4–10.0 g/m3). As shown in figure 5, the leader channel in continuous propagation mode appears curved, significantly dimmer and thinner than that in re-illumination mode.
To further investigate the characteristics of leader, figure 6(a) presents the optical image of leader-streamer system in continuous leader propagation mode, contrasted with that in re-illumination mode as shown in figure 6(b). It is evident from the images that, besides the differences of leader, the streamer region in re-illumination mode exhibits a branching shape resembling the “branch type” as described by Chen et al [14]. While during continuous leader propagation, the streamer at the front of the leader appears diffuse, resembling the “diffuse type”.
Figure 7 provides other two images of the leader-streamer system in re-illumination mode. Within the exposure time of each image, two discharge pulses appeared, corresponding to two elongations of the leader. An interesting phenomenon is observed in the images: there are two separated streamer regions in each image, and no streamer is generated between the front and rear of the newly elongated leader. A detailed analysis of the phenomenon will be provided in the following sections.
By analyzing the time-resolved images, multiple sets of one-dimensional elongation lengths of the leaders in the experiment (excluding the final jump stage) were statistically obtained, as depicted in figure 8. Considering the wide variation in the formation time of the stable leaders (ranging from 250 to 750 μs), we designated the initiation time of each leader as time 0 in the figure to more intuitively represent the elongation lengths of the leaders. It is evident that within a frame interval of 13.14 μs, the leaders can achieve an elongation of up to 1 m or even greater, surpassing the elongation lengths observed in continuous leader propagation mode [13].
Figure 9 shows the statistical distribution of the one-dimensional velocities of multiple leaders under the same experimental condition (excluding the final jump stage). It is important to clarify that the velocities represent the average velocities of elongation over an exposure time, which does not signify continuous forward propagation at that speed. A velocity of 0 indicates that the leader is in the dark period and has stopped propagating. In continuous propagation mode of the typical positive leader, the average velocities range from 1.7 to 2.2 cm/μs [13, 20]. However, in re-illumination mode, the distribution of the propagation velocities is highly random, ranging from 3.7 to 18.4 cm/μs. The coefficient of variation of velocities is as high as 36%. The average velocity (9.2 cm/μs) significantly exceeds that of continuous leader propagation (1.5–2.0 cm/μs [20]). Therefore, it is worth investigating whether mathematical models based on continuous leader propagation are still suitable for describing the discontinuous leader propagation.
High atmospheric humidity plays a crucial role in causing the re-illumination phenomenon [14, 21]. When the absolute humidity in the atmosphere is high, free electrons from the leader-streamer region are readily absorbed by electronegative water molecules. This absorption reduces the number of electrons that maintain electrical conductivity and temperature in the leader channel, resulting in a decrease in the net ionization rate [22]. If the ionization rate falls below the threshold required for continuous leader propagation, the current rapidly diminishes, causing the leader to cease propagation. Moreover, when the wave front time of the applied impulse voltage is prolonged, the voltage rise rate decreases. If the voltage increase is insufficient to counteract the shielding effect caused by net space charges, the electric field strength at the leader head diminishes, further reducing ionization activity in the streamer discharge region. Only when the voltage increment surpasses the shielding effect can the leader resume propagation.
In this experiment, the switching impulse voltages of long wave front time (1000 μs/5000 μs) were applied, and the absolute humidity in the environment reached levels as high as 20.0–30.1 g/m3. Under these conditions, the leaders propagated mainly in the re-illumination mode, with almost no significantly continuous propagation occurring, which differs greatly from the conventional experimental phenomena.
The generally accepted theory regarding the streamer-leader transition mechanism is the thermal ionization theory. According to this theory, during the transition from a streamer to a leader, the free electrons produced in the head streamer regions converge at the root of the streamers (stem) and inject the electrode. Under the interaction of electrons and ions, the temperature in the region gradually rises. As the temperature in the transition region reaches a critical temperature of approximately 1500 K, negative ions in the stem detach in large quantities due to the elevated temperature. This process leads to a rapid increase in electron density and electrical conductivity in the stem, facilitating the gradual transition of the stem into an initial leader [23]. Popov proposed that the radius of the stem is 1 cm [24], indicating that the length of each streamer-leader transition is 1 cm. Building upon the theories above, many scholars put forward different mathematical models for the propagation of positive leaders [25]. Gallimberti et al suggested that the positive leader propagation velocity VL is directly proportional to the current IL and inversely proportional to the charge in leader channel per unit axial length qL [23]:
| VL=ILqL. | (1) |
In the equation, the velocity VL is measured in cm/μs, the current IL is measured in amperes, and the charge in leader channel per unit axial length qL is related to factors such as humidity, with a range of 20–50 μC/m. In this paper, the value of qL is taken as 50 μC/m [26].
Andreev et al determined the dependence of the positive leader propagation velocity VL (cm/μs) on the current IL (A) in experiments of 8 m air gap [27]:
| VL=1.88×I0.67L. | (2) |
Gu et al obtained the relationship between the positive leader propagation velocity VL (cm/μs) and the current IL (A) through inversion of multiple discharge observation results [28]:
| VL=(ab+cIdL)(b+IdL)−1×10−4. | (3) |
In the equation, a=189.40;b=35.91;c=5.59×105;d=0.66.
To assess the validity of these models for leader re-illumination, we derived three sets of relationships between leader propagation length and charge by integrating equations (1)–(3) over time. These relationships were used to fit a set of typical experimental data on leader propagation length, as depicted in figure 10. The figure reveals that the mathematical models do not accurately predict the discontinuous leader propagation length, as the actual length exceeds the model predictions significantly. Therefore, these models suitable for continuous leader propagation are inadequate for describing leader re-illumination, suggesting that the mode of streamer-leader transition in re-illumination may be different from that in continuous leader propagation.
It is generally believed that in the continuous development mode, the leader originates from the continuous transition of the stem (about 1 cm [24]). However, this perspective does not explain the optical morphological characteristics of leader in re-illumination mode. When the leader channel continuously transforms from the stem, streamers will be generated from the corresponding leader tip at any moment of continuous leader propagation, indicating that the streamer regions should exhibit macroscopic continuity. In this case, the presence of two separated streamer regions at the front and rear of the leader, as shown in figure 7, would not occur. Additionally, due to the randomness in propagation direction, newly formed leader segments may curve rather than be so straight. In conclusion, the perspective that the leader originates from the continuous transition of the stem (about 1 cm) fails to account for the leader optical characteristics and the leader elongation in re-illumination, indicating different streamer-leader transition process in re-illumination mode compared to continuous leader propagation mode.
In the latest research, it was shown that the length of the stem is not constant [29], indicating the possibility of the region transitioning to the leader being longer. Based on the discharge current waveforms and optical images, we speculate that the newly elongated leader in re-illumination mode does not evolve gradually from the stem (about 1 cm) but rather evolves overall from a thermal channel much longer than stem.
Figures 11(a) and (b) illustrate the streamer-leader transition processes in continuous and discontinuous leader propagation mode, aligning well with the observed experimental phenomena. In continuous leader propagation mode, the stem undergoes continuous heating and development under stable current injection, resulting in the gradual extension of the leader forward. At the head of each newly formed leader, streamer discharge occurs. Consequently, from the optical images captured during the exposure period, we observe streamers generated at every point along the newly elongated leader without interruption.
In discontinuous leader propagation mode, the streamers exhibit a “branch type” appearance with noticeable luminous intensity contrast compared to the more uniform luminous intensity of the “diffuse type” streamers. Chen et al proposed that the differences between the two types of streamers are due to high humidity [14], but they did not investigate the connection between the streamer structure and streamer-leader transition process. Now, we offer our perspective as follows: in the process of streamer-leader transition, the branches with strong luminous intensity inject a relatively large amount of charge, thereby generating significant heating effects, enough to form the initial thermal channel. Due to the short duration of the pulse current generated by streamers, the vibrational temperature of gas molecules within the channel has not reached equilibrium with the translational temperature, with the former surpassing the latter. During the dark period, the temperature of the thermal channel gradually rises due to vibrational-translational relaxation (V-T relaxation), leading to the increase of conductivity. When the applied voltage reaches a sufficient level to initiate the next streamer discharge, the thermal channel, which is much longer than stem, completes the transition to the leader as a whole. This process explains why the elongated segment of the leader appears so straight and long in re-illumination mode. Immediately following the transition, new streamers emerge from the head of the new leader. Therefore, during the discontinuous propagation of the leader, the streamer regions are discontinuous, corresponding to figure 7 showing the two separated streamer regions.
In reference [30], under the slow varying ambient electric field, Huang et al discovered a discharge that emits faint light at the halted leader tip before the intense reillumination by using a high-speed video camera and a synchronized electrical parameter measurement system. They believed that the discharge causing the faint illumination leads to the leader elongation in the pre-reillumination stage, and the leader channel actually restarts and extends forward before the intense re-illumination. This discovery points out that there is leader formation and development in the dark period, which is consistent with our hypothesis that the transformation to the leader takes place in the dark period. However, since we did not observe any clear discharge phenomena during the dark period in our experiments, we are inclined to believe that the leader transformation process during dark period is the overall heating transformation of a thermal channel rather than a gradual progression.
In a high humidity environment, we conducted long spark experiments in a 10 m UHV transmission line gap under switching impulse voltages of long wave front time. Discharge current and voltage waveforms and optical morphological images of the leader-streamer system in re-illumination mode were captured by a comprehensive observation platform. The conclusions are as follows:
(1) Under the conditions of high humidity environment (20.0–30.1 g/m3) and with the application of 3376 kV slowly varying impulse voltages (wave front time of 1000/5000 μs) to a 10 m UHV transmission line gap, positive leaders mainly propagate discontinuously, with almost no significantly continuous propagation occurring. The main reason for this phenomenon is that the electronegative water molecules absorb a large number of free electrons from the leader-streamer region, causing the ionization rate falling below the threshold required for continuous leader propagation. Furthermore, the applied impulse voltages of long wave front time are not conducive to counteract the shielding effect caused by net space charges, thereby exacerbating the discontinuity in leader propagation.
(2) There are significant differences between continuous propagation leaders and discontinuous propagation leaders. In terms of morphology, leaders in re-illumination mode appear intensely luminous with straight elongation segment, and feature “branch type” streamers, while the leaders during continuous propagation appear curved characteristics and “diffuse type” streamers. In terms of development status, the distribution of leader propagation velocities in re-illumination mode is highly random, ranging from 3.7 to 18.4 cm/μs. The coefficient of variation of velocities is as high as 36%, and the average velocity is 9.2 cm/μs, significantly higher than that of continuous propagation mode (1.5–2.0 cm/μs).
(3) Based on the characteristics of straight leader elongation segment accompanied by “branch type” streamers and the phenomenon of two separated streamer regions in re-illumination mode, it is speculated that the newly elongated leader in re-illumination mode does not evolve gradually from the stem (about 1 cm) but rather evolves overall from a thermal channel much longer than stem.
This work was supported by the National Magnetic Confinement Fusion Science Program of China (Nos. 2022YFE03090000 and 2022YFE03060002), National Natural Science Foundation of China (No. 12375214), China National Nuclear Corporation Fundamental Research Program (No. CNNC-JCYJ-202236), and Innovation Program of Southwestern Institute of Physics (No. 202301XWCX006-04). This work was also supported by Youth Science and Technology Innovation Team of Sichuan Province (No. 2022JDTD0003), and US DoE Office of Science (Nos. DE-FG02-95ER54309 and DE-FC02-04ER54698).
| [1] |
Hender T C et al 2007 Nucl. Fusion 47 S128 doi: 10.1088/0029-5515/47/6/S03
|
| [2] |
Troyon F et al 1984 Plasma Phys. Control. Fusion 26 209 doi: 10.1088/0741-3335/26/1A/319
|
| [3] |
Bondeson A and Ward D J 1994 Phys. Rev. Lett. 72 2709 doi: 10.1103/PhysRevLett.72.2709
|
| [4] |
Hu B and Betti R 2004 Phys. Rev. Lett. 93 105002 doi: 10.1103/PhysRevLett.93.105002
|
| [5] |
Liu Y Q et al 2008 Phys. Plasmas 15 112503 doi: 10.1063/1.3008045
|
| [6] |
Chu M S and Okabayashi M 2010 Plasma Phys. Control. Fusion 52 123001 doi: 10.1088/0741-3335/52/12/123001
|
| [7] |
Berkery J W et al 2010 Phys. Plasmas 17 082504 doi: 10.1063/1.3474925
|
| [8] |
He Y L et al 2014 Phys. Rev. Lett. 113 175001 doi: 10.1103/PhysRevLett.113.175001
|
| [9] |
Hao G Z et al 2011 Phys. Rev. Lett. 107 015001 doi: 10.1103/PhysRevLett.107.015001
|
| [10] |
Hao G Z et al 2012 Phys. Plasmas 19 032507 doi: 10.1063/1.3692185
|
| [11] |
Liu C et al 2015 Nucl. Fusion 55 063022 doi: 10.1088/0029-5515/55/6/063022
|
| [12] |
Liu Y Q et al 2000 Phys. Plasmas 7 3681 doi: 10.1063/1.1287744
|
| [13] |
Wang Z R and Guo S C 2011 Nucl. Fusion 51 053004 doi: 10.1088/0029-5515/51/5/053004
|
| [14] |
Li L, Liu Y and Liu Y Q 2012 Phys. Plasmas 19 012502 doi: 10.1063/1.3672512
|
| [15] |
Wang S et al 2021 Plasma Phys. Control. Fusion 63 055019 doi: 10.1088/1361-6587/abf315
|
| [16] |
Xia G L et al 2005 Nucl. Fusion 55 093007
|
| [17] |
Liu Y Q et al 2009 Phys. Plasmas 16 056113 doi: 10.1063/1.3123388
|
| [18] |
Chapman I T et al 2011 Nucl. Fusion 51 073040 doi: 10.1088/0029-5515/51/7/073040
|
| [19] |
Varje J et al 2016 Nucl. Fusion 56 046014 doi: 10.1088/0029-5515/56/4/046014
|
| [20] |
Tani K et al 2016 Plasma Phys. Control. Fusion 58 105005 doi: 10.1088/0741-3335/58/10/105005
|
| [21] |
Sanchis L et al 2021 Nucl. Fusion 61 046006 doi: 10.1088/1741-4326/abdfdd
|
| [22] |
Heidbrink W W 2008 Phys. Plasmas 15 055501 doi: 10.1063/1.2838239
|
| [23] |
Gorelenkov N N, Pinches S D and Toi K 2014 Nucl. Fusion 54 125001 doi: 10.1088/0029-5515/54/12/125001
|
| [24] |
Graves J P et al 2015 Plasma Phys. Control. Fusion 57 014033 doi: 10.1088/0741-3335/57/1/014033
|
| [25] |
Pinches S D et al 2015 Phys. Plasmas 22 021807 doi: 10.1063/1.4908551
|
| [26] |
Liu Y Q et al 2022 Nucl. Fusion 62 066011 doi: 10.1088/1741-4326/ac5b8b
|
| [27] |
Wang X et al 2023 Nucl. Fusion 63 096023 doi: 10.1088/1741-4326/aceb77
|
| [28] |
Li Q and HL-2M Team 2015 Fus. Eng. Des. 96–97 338 doi: 10.1016/j.fusengdes.2015.06.106
|
| [29] |
Liu Y Q et al 2019 Nucl. Fusion 59 126021 doi: 10.1088/1741-4326/ab3f87
|
| [30] |
Xue L et al 2019 Nucl. Fusion 60 016016
|
| [31] |
Duan X R et al 2022 Nucl. Fusion 62 042020 doi: 10.1088/1741-4326/ac3be6
|
| [32] |
Meneghini O and Lao L 2013 Plasma Fusion Res. 8 2403009 doi: 10.1585/pfr.8.2403009
|
| [33] |
Li B et al 2019 Fusion Eng. Des. 147 111229 doi: 10.1016/j.fusengdes.2019.06.002
|
| [34] |
Xu X Y et al 2020 Plasma Sci. Technol. 22 085101 doi: 10.1088/2058-6272/ab8973
|
| [35] |
Jaulmes F et al 2021 Nucl. Fusion 61 046012 doi: 10.1088/1741-4326/abd41b
|
| [36] |
Garofalo A M et al 2000 Nucl. Fusion 40 1491 doi: 10.1088/0029-5515/40/8/307
|
| [37] |
Garofalo A M et al 2001 Nucl. Fusion 41 1171 doi: 10.1088/0029-5515/41/9/305
|
| [38] |
Lütjens H, Bondeson A and Roy A 1992 Comput. Phys. Commun. 69 287 doi: 10.1016/0010-4655(92)90167-W
|
| [1] | Zhiwei LI, Ting LEI, Yu SU, Xiuyuan YAO, Bingxue YANG, Delong LIU, Fangcheng LV, Yujian DING. Dynamic propagation velocity of a positive streamer in a 3 m air gap under lightning impulse voltage[J]. Plasma Science and Technology, 2024, 26(4): 045501. DOI: 10.1088/2058-6272/ad0d51 |
| [2] | Jianghai GENG, Guo LIN, Ping WANG, Yujian DING, Yang DING, Hua YU. Modification of streamer-to-leader transition model based on radial thermal expansion in the sphere-plane gap discharge at high altitude[J]. Plasma Science and Technology, 2024, 26(1): 015501. DOI: 10.1088/2058-6272/ad0c1c |
| [3] | Jianghai GENG, Quansheng WANG, Xiaomin Liu, Ping WANG, Fangcheng LYU, Yujian DING. Dynamic development model for long gap discharge streamer-leader system based on fractal theory[J]. Plasma Science and Technology, 2022, 24(9): 095402. DOI: 10.1088/2058-6272/ac6cd2 |
| [4] | Shuqun WU (吴淑群), Xueyuan LIU (刘雪原), Guowang HUANG (黄国旺), Chang LIU (刘畅), Weijie BIAN (卞伟杰), Chaohai ZHANG (张潮海). Influence of high-voltage pulse parameters on the propagation of a plasma synthetic jet[J]. Plasma Science and Technology, 2019, 21(7): 74007-074007. DOI: 10.1088/2058-6272/ab00b0 |
| [5] | Yang LIU (刘洋), Jiaming SHI (时家明), Li CHENG (程立), Jiachun WANG (汪家春), Zhongcai YUAN (袁忠才), Zongsheng CHEN (陈宗胜). High-power microwave propagation properties in the argon plasma array[J]. Plasma Science and Technology, 2019, 21(1): 15402-015402. DOI: 10.1088/2058-6272/aae369 |
| [6] | Haixin HU (胡海欣), Feng HE (何锋), Ping ZHU (朱平), Jiting OUYANG (欧阳吉庭). Numerical study of the influence of dielectric tube on propagation of atmospheric pressure plasma jet based on coplanar dielectric barrier discharge[J]. Plasma Science and Technology, 2018, 20(5): 54010-054010. DOI: 10.1088/2058-6272/aaaad9 |
| [7] | WANG Maoyan (王茂琰), ZHANG Meng (张猛), LI Guiping (李桂萍), JIANG Baojun (姜宝钧), ZHANG Xiaochuan (张小川), XU Jun (徐军). FDTD Simulation on Terahertz Waves Propagation Through a Dusty Plasma[J]. Plasma Science and Technology, 2016, 18(8): 798-803. DOI: 10.1088/1009-0630/18/8/02 |
| [8] | CHEN She (陈赦), ZENG Rong (曾嵘), ZHUANG Chijie (庄池杰), ZHOU Xuan (周旋), DING Yujian (丁玉剑). Experimental Study on Branch and Diffuse Type of Streamers in Leader Restrike of Long Air Gap Discharge[J]. Plasma Science and Technology, 2016, 18(3): 305-310. DOI: 10.1088/1009-0630/18/3/15 |
| [9] | FU Wenjie (傅文杰), YAN Yang (鄢扬). Analysis of High-Power Microwave Propagation in a Magnetized Plasma Filled Waveguide[J]. Plasma Science and Technology, 2013, 15(10): 974-978. DOI: 10.1088/1009-0630/15/10/03 |
| [10] | LI Chunzao(李春早), LIU Shaobin(刘少斌), BIAN Borui(卞博锐), DAI Zhaoyang(戴钊阳), ZHANG Xueyong(张学勇). Theoretical Analysis on Propagation of Electromagnetic Wave in Preformed Narrow Plasma Channel[J]. Plasma Science and Technology, 2012, 14(8): 702-707. DOI: 10.1088/1009-0630/14/8/04 |
| 1. | Bian, R., Chen, K., Li, G. et al. Analysis of Leader Propagation Velocity Based on Three-Dimensional Reconstruction of Discharge Channel in ±800 kV UHVDC Tower Gaps. Lecture Notes in Electrical Engineering, 2025. DOI:10.1007/978-981-96-1391-5_33 |
| 2. | Liu, L., Zhong, Z., Zhang, H. et al. Research on the Calculation of Initial streamer Length in High-Altitude Sphere-Plate Gap Based on the streamer Injection Development Model. 2024. DOI:10.1145/3702879.3702919 |
| 3. | Zhao, A., Fu, Z., Fu, Y. Simulation Analysis of Interphase Gap Breakdown Characteristics in UHV Transmission Lines with Consideration of Humidity Effects. 2024. DOI:10.1109/ICNEPE64067.2024.10860344 |