| Citation: | Huasheng XIE, Xiang GU, Yumin WANG, Quanyun WANG, Feng WANG, Haozhe KONG, Jiaqi DONG, Yunfeng LIANG, Yueng-Kay Martin PENG, Minsheng LIU, the EHL-2 Team. Preliminary considerations and challenges of proton-boron fusion energy extraction on the EHL-2 spherical torus[J]. Plasma Science and Technology, 2025, 27(2): 024010. DOI: 10.1088/2058-6272/adae43 |
EHL-2 spherical torus (ST) is one of the key steps of p-11B (proton-boron or hydrogen-boron) fusion energy research in ENN. The fusion produced energy is carried mainly by alpha particles of average energy 3 MeV, which ideally can be converted to electricity with high efficiency (> 80%). However, there exist serious difficulties to realize such conversion in a fusion device, due to the high energy density and high voltage required. To comprehensively describe the progress of the EHL-2 physics design, this work presents preliminary considerations of approaches for achieving energy conversion, highlighting critical issues for further investigation. Specifically, we provide an initial simulation of alpha particle extraction in the EHL-2 ST configuration as a starting point for p-11B fusion energy conversion.
The fusion reactions most likely to achieve energy gain include D-T (deuterium–tritium), D-D, D-3He (deuterium–helium-3), and p-11B (proton–boron) [1]. These reactions produce neutrons and charged particles, as shown in the following reactions:
| D+T→n(14.07MeV)+42He(3.52MeV) |
| D+D→n(2.45MeV)+32He(0.82MeV) (50%) |
| D+D→p(3.03MeV)+T(1.01MeV) (50%) |
| D+32He→p(14.68MeV)+42He(3.67MeV) |
| p+115B→342He(8.68MeV) | (1) |
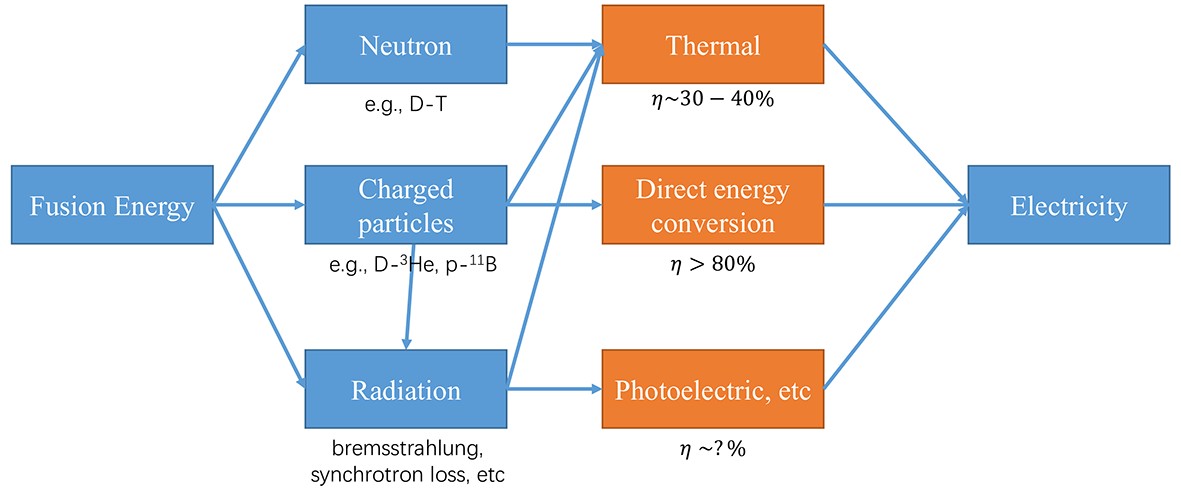
In D-T fusion, the majority of the energy is carried by high-energy neutrons, which can be converted into electricity through conventional thermal conversion methods with an efficiency typically ranging from 30% to 40%. This approach is relatively well-established in fission reactors/plants. For D-3He and p-11B fusion, most of the energy is carried by high-energy charged particles, which could potentially be converted directly into electricity with an efficiency exceeding 80%. This higher efficiency could make p-11B and D-3He fusion, despite being more challenging than D-T due to their low reactivity, more viable by reducing the overall difficulty through improved power generation efficiency. Major approaches for fusion energy conversion to electricity are shown in figure 1.
Direct energy conversion (DEC) in fusion plants means directly converting the kinetic energy of charged particles produced during fusion into electricity, bypassing the need for an intermediate thermal power cycle or other processes. This method can significantly enhance the overall efficiency of fusion power generation compared to traditional approaches that rely on steam turbines, as it avoids the energy losses associated with thermal-to-electrical conversion. However, DEC technology suitable for future fusion plants is still in its infancy and faces numerous unresolved challenges. In spherical torus/tokamak (ST) devices, the magnetic field lines in the plasma confinement region are closed, unlike the open magnetic field line systems used in earlier DEC research, such as magnetic mirrors [2, 3]. This difference introduces additional challenges, particularly in efficiently extracting charged particles in a directed manner. ENN has opted for the p-11B fusion route using ST technology [4], with the aim of employing DEC technology to generate electricity from fusion energy, and plans to test this technology on the EHL-2 device [4, 5].
The challenge of energy conversion in ST p-11B fusion can be divided into four independent issues/problems with two categories of particle extraction (collection) and energy conversion as listed in table 1, which will ultimately need to be integrated, i.e.:
| Categories | No. | Issues to address | Comments |
| Particle extraction (collection) | (1a) | How to align and direct charged particles for efficient extraction and collection. | Could be challenge in closed field line system than in open field line system? |
| (1b) | The characteristics of charged particle losses and extraction within the electromagnetic field structure inherent to the ST. | To maximize the use of the default electromagnetic field structure, including ripple, RMP, ELM, etc. | |
| Energy conversion | (2a) | How to convert the kinetic energy of the collected charged particles. | Detail design of the DEC approach. |
| (2b) | How to convert and collect radiation energy and other forms of energy that cannot be directly converted into electricity. | To improve the overall efficiency. |
(1a) How to align and direct charged particles for efficient extraction.
(1b) The characteristics of charged particle losses and extraction within the electromagnetic field structure inherent to the ST.
(2a) How to recover the energy of the extracted charged particles.
(2b) How to recover radiation energy and other forms of energy that cannot be directly converted into electricity.
The issue (1b) is relevant to issue (1a). For issue (2b) in table 1, the priority is to use high-reflectivity wall materials to minimize energy losses from cyclotron radiation (with frequency mainly at around 50–300 GHz, magnetic field at around 2–8 T), while also considering the application of efficient photoelectric conversion materials to partially recover energy from bremsstrahlung radiation (with energy mainly at around 0.05–1 MeV).
In this paper, section 2 will summarize existing solutions to issue (2a) and discuss the main challenges associated with ST p-11B fusion, which could play a crucial role in selecting a suitable solution. In section 3, we will present some preliminary simulation results related to issue (1b), i.e, the alpha particle energy extraction. Innovative approaches are still required for issue (1a). In section 4, we will provide a summary of the current state and outline future directions. It should be noted that the present work is preliminary and serves to outline the energy conversion aspect of the EHL-2 physics design progress. The primary purpose is to identify critical issues and challenges for future investigations, which are crucial for shaping the overall design.
Although this work focuses primarily on energy extraction, a brief review of proposed approaches for DEC could help in addressing critical issues. In this section, we summarize the proposed solutions to issue (2a) in table 1, and discuss the main challenges associated with ST p-11B fusion. Introduction of DEC can also be found at Wikepedia [6].
Early discussions on fusion energy conversion are well-documented in the works of references [7, 8]. The typical approach to DEC involves decelerating and collecting charged particles. This process can be carried out using various methods: electrostatic conversion with one or more high-voltage electrodes, inverse cyclotron conversion which operates in reverse of a cyclotron accelerator, or high-frequency traveling-wave resonant absorption (e.g., 155 MHz). Post was among the first to seriously study the concept of DEC [2], where he described a highly efficient multi-plate collector. Following Post’s work, extensive theoretical, engineering and economic analyses of DEC were conducted during the 1970s [9, 10], and several experiments are also done later [3, 11]. The primary methods for DEC are summarized in figure 2.
Electrostatic direct energy converters function as linear accelerators operating in reverse. In this system, fast ions enter from the “exit” of the accelerator and are decelerated by retarding electric fields before being collected on high-voltage electrodes, which form the positive terminal of the DEC power source. The Venetian-Blind (VB) [12] direct energy converter concept uses ribbon-like surfaces that allow ions to pass more easily in one direction than in the opposite. As ions move through surfaces with progressively increasing potential, they eventually turn and start to move back, at which point they encounter opaque surfaces and are collected. This method sorts ions by energy, with higher-energy ions being collected on higher-potential electrodes. Electrostatic conversion is generally effective for ion energies less than 1 MeV, as high voltage is still manageable within this range.
Electromagnetic conversion methods [9, 13, 14], such as the magnetic compression-expansion direct energy converter, are conceptually similar to the internal combustion engine, which is only suitable for pulse operation. A thermodynamic efficiency study of the magnetic direct conversion cycle, which converts alpha particle energy into mechanical work, showed an efficiency of 62% [13]. The Traveling-Wave Direct Energy Converter (TWDEC) [15–18], proposed by a joint Japan-USA team, utilizes a gyrotron converter to guide fusion product ions as a beam into a 10 m long microwave cavity within a 10 T magnetic field. In this cavity, 155 MHz microwaves are generated and converted into high-voltage DC output through rectennas. TWDEC is suitable for ions with energy levels greater than 1 MeV, such as the 14.7 MeV protons from D-3He fusion.
The inverse cyclotron converter (ICC) method [19, 20], which employs the reverse process of a cyclotron accelerator, has also been proposed. It is considered to be more compact than TWDEC while maintaining high energy conversion efficiency. Photon direct conversion involves converting the kinetic energy of high-energy particles into photons, which are then used by photovoltaic cells to generate electricity. In a rigorous manner, photon direct conversion is not DEC, since an intermediate process is involved, i.e., kinetic energy to photon energy.
A more detailed comparison of the advantages and disadvantages of these approaches is presented in table 2.
| Conversion scheme | Basic idea | Advantages | Disadvantages |
| Electrostatic conversion [2, 9] | Generating electricity through electrostatic fields created by plasma charge separation. | High energy conversion efficiency (for < 1 MeV). | Electrostatic pressure is prone to breakdown, and the output high-voltage direct current is difficult to convert and transmit. High-energy direct conversion still has drawbacks. |
| Inverse cyclotron converter [19, 20] | Generating electricity via the reverse process of a cyclotron accelerator. | Small size, saves materials and space, can be used for energy > 1 MeV. | May cause significant damage to electrode materials. |
| Electromagnetic conversion [9] | Generating electricity through induced currents from the expansion of plasma fusion. | Plasma generates electricity through electromagnetic induction without direct contact with the walls, reducing material corrosion issues. | Pulsed operation, with significant leakage losses at both ends of the device |
| Traveling-wave resonant absorption [15, 18] | Modulating a longitudinal wave in an ion beam, followed by generating electricity from an induced alternating potential through decelerating electrodes. | No issue with high-voltage breakdown, and the output alternating current energy is easy to transmit. Suitable for high-energy particles > 1 MeV. |
Low conversion efficiency, even lower conversion efficiency for low-energy particles. |
| Photon direct conversion | Converting the kinetic energy of high-energy particles into photons, then using photovoltaic cells to generate electricity. | High energy conversion efficiency | May introduce impurities into the plasma, not conducive to long-term stable high-power operation. |
Mirror and Field-Reversed Configuration (FRC) systems are particularly suitable for the application of direct energy converters, as their plasmas are surrounded by open magnetic field lines. The VB collectors in reference [12] is designed for tokamak, say, via a divertor; however, a detailed technique has not yet been worked out. VB can achieve high energy conversion efficiencies. Reactor studies suggest that the cost of electricity (COE) could be lower for a D-3He FRC than for a D-T tokamak [21].
Note that we have not listed all possible DEC methods here. In the study of D-3He tokamak ARIES-III [22], researchers evaluated fourteen energy conversion options and selected four for further investigation: liquid metal magnetohydrodynamics (MHD), plasma MHD, rectenna conversion, and direct electrodynamic conversion. According to Johansson’s work [21], the most effective energy conversion approach for a D-D or D-3He ST appears to be direct electrodynamic conversion, potentially supplemented by conventional thermal conversion or liquid metal MHD conversion.
In the context of ST, earlier studies, such as those by Peng in 1988 [22, 23], have proposed conceptual designs for DEC systems. The primary concept involves placing a DEC chamber above the core plasma. The scrape-off layer plasma would be diverted into this chamber, where particle drifts would be used to separate ions from electrons. The ions would then be collected on one plate, while the electrons would be collected on another. The estimated efficiency of this system is around 50%, though it remains a conceptual idea that requires further exploration.
The electrostatic approach has already been successfully demonstrated in experiments [3] with open magnetic field line systems, laying a strong foundation for further research. Additionally, MW-level energy recovery has been successfully achieved in neutral beam injection (NBI) systems. There are series energy recovery experiments [24, 25] of 100 keV level positive and negative ion NBI, and designs [26–28] of MeV level for NBI of fusion power plants such as ITER and DEMO. This technique requires the charged particles to be monoenergetic and well-collimated, and also demands the maintenance of a high vacuum to support extremely high voltages. For p-11B fusion in ST, our goal is to achieve simple and efficient energy conversion, which leads us to prioritize electrostatic conversion methods. Therefore, we aim to develop the electrostatic conversion method in EHL-2 as a first step to identify its capabilities and limitations in ST systems, serving as a basis for further studies due to its straightforward nature.
While the concept of DEC may be technically feasible, its viability in practical fusion plants remains uncertain. A more quantitative understanding of the primary challenges is necessary to develop a more objective perspective on its implementation. This issue has been partially explored by Rosenbluth and Hinton [29], who examined factors such as energy density and spatial dimensions. They concluded that efficient direct conversion is unlikely in closed-field line systems and that open geometries, although more conducive to direct conversion, do not provide sufficient confinement for D-3He, let alone p-11B. Additionally, unconverted high-energy particles can damage reactor walls and cause other issues.
To put it simply, the economic viability of fusion power depends on maintaining a sufficiently high plasma density. For p-11B fusion, this density must be on the order of 1×1020 to 1×1021 m−3 [1]. At such densities and assuming temperature to be 100 keV, the Debye length becomes extremely small (~ 1×10−4 m), making charge separation—and thus the construction of energy conversion devices of the necessary small size—impossible. In systems with open magnetic field lines, expanding the ends increases the volume and decreases the density, allowing the Debye length to reach centimeter scales. This makes charge separation for energy conversion feasible. However, in closed systems like ST, achieving low-power energy output is possible, but the size of the equipment and the surface power load [30] required for high-power output may exceed current technological capabilities or may not be economically viable. For example, the size of the DEC expander chamber will probably be of order 75–100 m in radius for mirror reactor [2]. Some rough estimations would be helpful for understanding. Assuming 100 MW p-11B fusion reactor, the alpha particle yield is N=2.2×1020 s−1. If the reactor can confine the alpha particles to τ=10s, the average density of alpha particles is nα=Nτ/V. For a sphere DEC device, volume V=4/3πR3, with radius size R=10m, electron temperature Te=100keV and electron density ne=2nα, we have the Debye length around 3 cm, which is possible to make charge separation. For a cylinder volume DEC device, the length scale R would be even larger. This can be seen as typical size of fusion plant DEC size. For the limitation of potential, it is usually assumed to be smaller than 10 kV/cm, which means that higher energy charged particles require larger DEC system size. There are other constrains for the DEC system design, c.f., [8].
Another significant challenge with p-11B fusion is the fact that the three alpha particles produced by the fusion reaction do not share a single energy level but rather have a distribution of energies (mainly between 1.5 and 4.5 MeV) [31]. This variation makes it impossible to set a fixed voltage for electrostatic conversion, necessitating the use of multi-stage structures to accommodate the range of particle energies. Moreover, in a p-11B fusion reactor, alpha particles would be utilized for both plasma heating and energy conversion, requiring further in-depth analysis to determine the optimal ratio between these two roles. The slowing-down alpha particles used for plasma heating would also partially contribute to energy conversion, adding further complexity to the DEC system.
In EHL-2, p-11B fusion power is around 0.7 kW and alpha yield is around 1.5×1015 s−1 [31]. If 1% of this energy can be used to test the DEC system with conversion efficiency of 70%, a 5 W light bulb can be lighted. Hence, it is feasible to use EHL-2 as a platform to do preliminary study of p-11B fusion DEC. If the predictions can be validated in EHL-2, it would strengthen our confidence in the physics and theoretical models of p-11B fusion, enabling more accurate predictions for future p-11B reactors. Therefore, although the fusion energy in EHL-2 is relatively low, it holds both significant symbolic and scientific value. Thus, we would be interested in the alpha particle extraction characteristic under EHL-2 configuration. As shown in table 1 for issue (1b), to directly output the energy of alpha particles, how to extract them is a key issue that needs to be addressed currently. Based on the current physical design of tokamaks, controlling the extraction of alpha particles could involve the use of active perturbation fields, such as Resonant Magnetic Perturbations (RMPs) [32] or toroidal field ripple [33], or passive perturbation fields like internal kink modes or Edge Localized Modes (ELMs) [34]. The particle extraction and DEC are also somehow similar to the ion energy diagnostic system [35].
Characterization of fast ion loss in EHL-2 spherical torus is studied in reference [36]. Here, we focus on preliminary simulation study of alpha particles loss characteristic in the EHL-2. This work employs PTC (Particles Tracer Code) code to model the particles loss process from the core to the EHL-2 first wall [37]. Three preliminary studies were employed to extract the alpha particles, as illustrated in figure 3.
The first attempt is to apply voltage to the upper right divertor. The second attempt is adding additional toroidal magnetic field. The third attempt is to consider toroidal field ripple, and its spatial distribution is depicted in figure 3.
To analyze alpha particle extraction under different conditions, the first step is to analyze particle loss in equilibrium magnetic field. The equilibrium magnetic field, thermal plasma profiles, and the initial distribution of alpha particles for the EHL-2 steady-state scenario are presented in figure 4. These parameters come from integrated simulations for EHL-2 [4] and are the main physical input for PTC code. In figure 5, panels (a) and (b) depict the spatial distribution of particle losses in the poloidal plane and the corresponding energy distribution, respectively. Panels (c), (d), and (e) illustrate the relationships between particle loss counts, energy loss, and pitch angle with the poloidal angle, respectively. The distributions of pitch angle and average energy indicate that parallel motion and drift effects play a dominant role in the particle loss process. It is observed that the loss distribution is localized in the poloidal plane due to the influence of magnetic drift characteristics, which is highly advantageous for particle collection. However, the broad energy and angular distributions of the lost alpha particles present challenges for efficient energy collection.
As mentioned above, we consider using different additional fields to control the extraction of alpha particles. Our first attempt is to apply different voltages to the upper right divertor. The main results are shown in figure 6. The results show that the impact on the loss of alpha particles is significant only when the voltage reaches the order of megavolts. As the voltage increases, the number of lost particles on the lower right divertor also rises, accompanied by an increase of the mean energy of lost particles. The impact particles’ trajectory is illustrated in figure 7, and it shows a marked effect on the trajectories of particles when the positive and negative potentials (P) reach several megavolts. Given the considerable distance between the divertor and the plasma, coupled with the latter’s inherent electrostatic shielding effect, it seems implausible that alpha particles can be extracted using this approach.
In tokamak plasmas, the toroidal magnetic field is a crucial factor affecting the plasma confinement performance. This study investigates the influence of varying toroidal field strengths on the behavior of alpha particles. We apply an additional toroidal magnetic field in the context of the plasma equilibrium magnetic field.
The simulation results presented in figure 8 indicate that an increase in the strength of the toroidal magnetic field enhances the retention time of alpha particles within the plasma by effectively reducing their radial loss. The simulation results also reveal that while the number of lost particles decreases with increasing toroidal magnetic field strength, the reduction is not substantial, and the average energy loss remains largely unaffected.
In equilibrium conditions, the toroidal magnetic field within the tokamak is generated by the toroidal coils. In an ideal case, the toroidal magnetic field exhibits strict symmetry in the toroidal direction. However, in practice, the axial symmetry of the field is disrupted due to the discrete nature of the toroidal field coils, which can reduce the confinement of alpha particles. This results in a toroidal field that is strong close to the coils and weak in the positions between the coils. This is referred to as a toroidal field ripple. The ripple strength, denoted by the symbol δ, is defined as follows:
| δ=Bmax−BminBmax+Bmin, | (2) |
where Bmax and Bmin are maximum and minimum field magnitudes at the same radial and vertical coordinates. At this point, the toroidal magnetic field strength can be expressed as
| Bφ=Bφ0(1−εcosθ)(1−δ(r,θ)cosnφ), | (3) |
where θ is the poloidal angle and φ is the toroidal angle, respectively, ε=aR is the inverse aspect ratio, and n is the number of toroidal field coils. Figure 3 shows the distribution of ripple of the EHL-2 device. The degree of ripple is the greatest near the outer mid-plane of the plasma, with a value of approximately 3.4×10−2%. The amplitude of the ripple disturbance increases in a natural exponential manner from the core to the boundary.
Scenario without collisions and a source scenario with collisions. In both cases, the alpha particle loss rate is relatively low when toroidal field ripple amplitude is low. The relatively small deviation of the magnetic field lines allows the trajectory of the alpha particles to remain aligned with them, ensuring relatively stable confinement with low loss. While minor scattering phenomena do occur, the overall loss is not significant. As the degree of ripple increases, the magnetic field perturbations cause a shift in the trajectories of the particles, thereby increasing the loss rate of alpha particles in the plasma edge region. Analysis of alpha particles losses in the non-source and no collisional slowing-down cases is shown in table 3. With the toroidal field ripple amplitude factor growing by a factor 1000, the alpha particle prompt loss rate increases gradually from 1.898% to 1.954%. Furthermore, the increase of the delayed loss is more significant.
| Toroidal field ripple | Prompt loss (%) | Delayed loss (%) | Total loss (%) |
| 0 | 1.894 | 0.004 | 1.898 |
| 1 (ripplemax ≈ 4.2×10−5) | 1.894 | 0.004 | 1.898 |
| 10 | 1.897 | 0.005 | 1.902 |
| 100 | 1.901 | 0.023 | 1.924 |
| 1000 | 1.954 | 0.943 | 2.897 |
Considering a source and including collision slowing-down case, figure 9 shows that as the toroidal field ripple amplitude increases by a factor of 100, there is no significant effect on alpha particle loss. While when the ripple increases by a factor of 1000, alpha particles are lost in a steady stream. Collecting these lost alpha particles could provide a reliable source for alpha particle extraction, laying the groundwork for subsequent energy conversion processes. It is thus possible to optimize the magnetic field design of the tokamak to increase the extraction of alpha particles. However, further research is required in order to establish the optimal balance between for the case of a source with a collision.
Table 4 summarizes our main findings on alpha particle loss or extraction under equilibrium magnetic fields and various perturbative conditions. The analysis highlights how factors like divertor voltage, toroidal field strength, and ripple amplitude influence alpha particle loss rate. These insights inform future efforts to optimize magnetic field design for effective alpha particle control and energy conversion. For D-T fusion, alpha particles are primarily used for plasma heating, making the minimization of their loss crucial. In contrast, for p-11B fusion, alpha particles fulfill a dual role: plasma heating and energy conversion, requiring precise control over their loss-to-extraction ratio. EHL-2 serves as an important research platform for studying the optimal balance between alpha particle heating and conversion, and we hope that it will provide valuable insights into this issue. Based on the current study, the next step could focus on optimizing the ripple and divertor structures in EHL-2 to extract both fusion-born and slowing-down alpha particles, as this approach may present fewer engineering challenges. Once extracted, these alpha particles would be directed to the DEC system, where we plan to initially explore the electrostatic conversion method.
| Attempted method | Specific operation | Summary of results |
| Equilibrium magnetic field conditions | Application of equilibrium magnetic field without additional perturbations | Alpha particle loss rate remains low, approximately 1.9%. |
| Apply of divertor voltage | Apply different levels of voltage to the upper right divertor target plate | Significant effect on alpha particle loss as potential increases to the order of megavolts. |
| Increase toroidal magnetic field | Increase in toroidal magnetic field strength | Modifications to the toroidal magnetic field do not exert a notable influence on alpha particle loss or collection. |
| Introduction of toroidal field ripple | Adjust the amplitude of the toroidal field ripple by a factor of 1000. | Under conditions with source terms and collisions, when the ripple amplitude increases to 1000 times, alpha particles are continuously lost, offering a steady source for their potential collection. |
Energy conversion in fusion power plants remains a significant challenge, and advancing new approaches will be essential in the coming years. The primary advantage of DEC approaches lies in their potential for much higher efficiencies than conventional thermal cycles, which typically suffer from heat losses and lower efficiency. However, considerable challenges remain, including particle extraction (collection) and energy conversion as listed in table 1, particularly for the ST devices with closed magnetic field lines. According to a detailed comparison of the advantages and disadvantages of several DEC approaches presented in table 2, ENN initially selected the electrostatic conversion method in EHL-2, designed to achieve observable p-11B fusion reactions, as a first step to identify its capabilities and limitations of DEC in ST systems, serving as a basis for further studies due to its straightforward nature.
In the current work, based on the parameters of EHL-2, a preliminary quantitative analysis of the major challenges has been carried out, and the simulation of alpha particle loss or extraction is presented. Based on the present study, modifying the ripple and divertor structures to extract fusion-born and slowing-down alpha particles in EHL-2 would be our preferred choice for the next step, as this approach may pose fewer engineering challenges. As research progresses, more results will be presented, and specific implementation plans will become clearer over the next few years. The field is open to innovative approaches, which are crucial for identifying and solving these challenges effectively, including those relevant to DEC applications in ITER and DEMO’s MeV-level NBI systems [28, 38]. Similar technology from NBI development would also be beneficial to DEC. DEC is not only vital for p-11B fusion energy research but also for D-D and D-3He fusion, as the majority of their fusion energy is carried by charged particles. Continued research and development in this area are crucial to overcoming current obstacles and realizing the full potential of fusion energy, particularly as neutron-based D-T fusion may face economic challenges due to the limited availability of tritium [4].
This work presents limited quantitative calculations, primarily because a final DEC approach has not yet been selected, and all proposed methods remain in early stages of development. With the growing interest in advanced fusion fuels, research and development in DEC have gained renewed momentum [39].
The authors would like to express their gratitude for the valuable discussions with Nan Chu, Clement Po-Ching Wong, Hulin Huang, Bin Chen, Dong Guo, Kun Wu, Shixin Ren and Junjie Shi.
| [1] |
Xie H S 2023 Introduction to Fusion Ignition Principles (Hefei: University of Science and Technology of China Press) (in Chinese)
|
| [2] |
Post R F 2015 Mirror Systems: Fuel Cycles, Loss Reduction and Energy Recovery (Oxfordshire: British Nuclear Energy Society) p87
|
| [3] |
Barr W L and Moir R W 1983 Nucl. Technol. Fusion 3 98 doi: 10.13182/FST83-A20820
|
| [4] |
Liu M S et al 2024 Phys. Plasmas 31 062507 doi: 10.1063/5.0199112
|
| [5] |
Liang Y F et al 2024 Plasma Sci. Technol. in press (https://10.1088/2058-6272/ad981a
|
| [6] |
Direct energy conversion (last accessed Sep 2, 2024), https://en.wikipedia.org/wiki/Direct_energy_conversion
|
| [7] |
Miley G H 1970 Direct Conversion of Nuclear Radiation Energy (Hinsdale: American Nuclear Society
|
| [8] |
Miley G H 1976 Fusion Energy Conversion (Hinsdale: American Nuclear Society
|
| [9] |
Moir R W 1977 Direct energy conversion in fusion reactors In: Considine D M Energy Technology Handbook( New York: McGraw-Hill
|
| [10] |
Gitomer S J and Krishnan C K 1974 IEEE Trans. Plasma Sci. 2 277 doi: 10.1109/TPS.1974.4316851
|
| [11] |
Barr W L, Moir R W and Hamilton G W 1982 J. Fusion Energy 2 131 doi: 10.1007/BF01054580
|
| [12] |
Moir R W and Barr W L 1973 Nucl. Fusion 13 35 doi: 10.1088/0029-5515/13/1/005
|
| [13] |
Oliphant T A, Ribe F L and Coultas T A 1973 Nucl. Fusion 13 529 doi: 10.1088/0029-5515/13/4/006
|
| [14] |
Sijoy C D and Chaturvedi S 2011 Fusion Eng. Des. 86 174 doi: 10.1016/j.fusengdes.2010.11.008
|
| [15] |
Momota H et al 1992 Fusion Technol. 21 2307 doi: 10.13182/FST92-A29724
|
| [16] |
Momota H et al 1999 Fusion Technol. 35 60 doi: 10.13182/FST99-A11963827
|
| [17] |
Sato K and Katayama H 2001 Fusion Technol. 39 203 doi: 10.13182/FST01-A11963442
|
| [18] |
Takeno H et al 2008 Fusion Eng. Des. 83 1696 doi: 10.1016/j.fusengdes.2008.06.046
|
| [19] |
Monkhorst H J and Rostoker N 2005 Controlled fusion in a field reversed configuration and direct energy conversion University of California University of Florida Research Foundation Inc US6850011B2
|
| [20] |
Rostoker N et al 2006 WO 2006096772-Plasma electric generation system published
|
| [21] |
Johansson M 2003 Direct Conversion of Fusion Energy (Sweden: Royal Institute of Technology
|
| [22] |
Santarius J F et al 1989 Energy conversion options for ARIES-III-a conceptual D-3He tokamak reactor In: Proceedings of the IEEE 13th Symposium on Fusion Engineering Knoxville: IEEE 1989: 1039
|
| [23] |
Peng Y K M 1988 Bull. APS 33 2044
|
| [24] |
Fumelli M, Jequier F and Pamela J 1989 Plasma Phys. Control. Fusion 31 495 doi: 10.1088/0741-3335/31/4/001
|
| [25] |
Paméla J et al 1993 Nucl. Instrum. Methods Phys. Res. Sect. B: Beam Interact. Mater. Atoms 73 296 doi: 10.1016/0168-583X(93)95740-V
|
| [26] |
Moir R W 1994 Fusion Technol. 25 129 doi: 10.13182/FST94-A30262
|
| [27] |
McAdams R et al 2013 AIP Conf. Proc. 1515 559 doi: 10.1063/1.4792828
|
| [28] |
Starnella G 2022 Increasing energy efficiency of neutral beam injection for DEMO: a conceptual design study PhD Thesis Technische Universität, München, Germany
|
| [29] |
Rosenbluth M N and Hinton F L 1994 Plasma Phys. Control. Fusion 36 1255 doi: 10.1088/0741-3335/36/8/003
|
| [30] |
Moir R W, Barr W L and Miley G H 1974 J. Nucl. Mater. 53 86 doi: 10.1016/0022-3115(74)90225-6
|
| [31] |
Li Z et al 2024 Plasma Sci. Technol. in press (https://doi.org/10.1088/2058-6272/ad9da2
|
| [32] |
Xu Y F et al 2020 Nucl. Fusion 60 086013 doi: 10.1088/1741-4326/ab966b
|
| [33] |
Xu Y F et al 2021 Plasma Sci. Technol. 23 095102 doi: 10.1088/2058-6272/ac0717
|
| [34] |
Harrer G F et al 2022 Phys. Rev. Lett. 129 165001 doi: 10.1103/PhysRevLett.129.165001
|
| [35] |
Henkel M et al 2018 Plasma Sci. Technol. 20 054001 doi: 10.1088/2058-6272/aab490
|
| [36] |
Sun T T et al 2024 Plasma Sci. Technol. in press (https://doi.org/10.1088/2058-6272/ad8dfb
|
| [37] |
Wang F et al 2021 Chin. Phys. Lett. 38 055201 doi: 10.1088/0256-307X/38/5/055201
|
| [38] |
Fantz U et al 2024 Nucl. Fusion 64 086063 doi: 10.1088/1741-4326/ad5dcd
|
| [39] |
Rax J M, Kolmes E J and Fisch N J 2024 arXiv: 2410.15485
|
| [1] | Zhiwei LI, Ting LEI, Yu SU, Xiuyuan YAO, Bingxue YANG, Delong LIU, Fangcheng LV, Yujian DING. Dynamic propagation velocity of a positive streamer in a 3 m air gap under lightning impulse voltage[J]. Plasma Science and Technology, 2024, 26(4): 045501. DOI: 10.1088/2058-6272/ad0d51 |
| [2] | Jianghai GENG, Guo LIN, Ping WANG, Yujian DING, Yang DING, Hua YU. Modification of streamer-to-leader transition model based on radial thermal expansion in the sphere-plane gap discharge at high altitude[J]. Plasma Science and Technology, 2024, 26(1): 015501. DOI: 10.1088/2058-6272/ad0c1c |
| [3] | Jianghai GENG, Quansheng WANG, Xiaomin Liu, Ping WANG, Fangcheng LYU, Yujian DING. Dynamic development model for long gap discharge streamer-leader system based on fractal theory[J]. Plasma Science and Technology, 2022, 24(9): 095402. DOI: 10.1088/2058-6272/ac6cd2 |
| [4] | Shuqun WU (吴淑群), Xueyuan LIU (刘雪原), Guowang HUANG (黄国旺), Chang LIU (刘畅), Weijie BIAN (卞伟杰), Chaohai ZHANG (张潮海). Influence of high-voltage pulse parameters on the propagation of a plasma synthetic jet[J]. Plasma Science and Technology, 2019, 21(7): 74007-074007. DOI: 10.1088/2058-6272/ab00b0 |
| [5] | Yang LIU (刘洋), Jiaming SHI (时家明), Li CHENG (程立), Jiachun WANG (汪家春), Zhongcai YUAN (袁忠才), Zongsheng CHEN (陈宗胜). High-power microwave propagation properties in the argon plasma array[J]. Plasma Science and Technology, 2019, 21(1): 15402-015402. DOI: 10.1088/2058-6272/aae369 |
| [6] | Haixin HU (胡海欣), Feng HE (何锋), Ping ZHU (朱平), Jiting OUYANG (欧阳吉庭). Numerical study of the influence of dielectric tube on propagation of atmospheric pressure plasma jet based on coplanar dielectric barrier discharge[J]. Plasma Science and Technology, 2018, 20(5): 54010-054010. DOI: 10.1088/2058-6272/aaaad9 |
| [7] | WANG Maoyan (王茂琰), ZHANG Meng (张猛), LI Guiping (李桂萍), JIANG Baojun (姜宝钧), ZHANG Xiaochuan (张小川), XU Jun (徐军). FDTD Simulation on Terahertz Waves Propagation Through a Dusty Plasma[J]. Plasma Science and Technology, 2016, 18(8): 798-803. DOI: 10.1088/1009-0630/18/8/02 |
| [8] | CHEN She (陈赦), ZENG Rong (曾嵘), ZHUANG Chijie (庄池杰), ZHOU Xuan (周旋), DING Yujian (丁玉剑). Experimental Study on Branch and Diffuse Type of Streamers in Leader Restrike of Long Air Gap Discharge[J]. Plasma Science and Technology, 2016, 18(3): 305-310. DOI: 10.1088/1009-0630/18/3/15 |
| [9] | FU Wenjie (傅文杰), YAN Yang (鄢扬). Analysis of High-Power Microwave Propagation in a Magnetized Plasma Filled Waveguide[J]. Plasma Science and Technology, 2013, 15(10): 974-978. DOI: 10.1088/1009-0630/15/10/03 |
| [10] | LI Chunzao(李春早), LIU Shaobin(刘少斌), BIAN Borui(卞博锐), DAI Zhaoyang(戴钊阳), ZHANG Xueyong(张学勇). Theoretical Analysis on Propagation of Electromagnetic Wave in Preformed Narrow Plasma Channel[J]. Plasma Science and Technology, 2012, 14(8): 702-707. DOI: 10.1088/1009-0630/14/8/04 |
| 1. | Bian, R., Chen, K., Li, G. et al. Analysis of Leader Propagation Velocity Based on Three-Dimensional Reconstruction of Discharge Channel in ±800 kV UHVDC Tower Gaps. Lecture Notes in Electrical Engineering, 2025. DOI:10.1007/978-981-96-1391-5_33 |
| 2. | Liu, L., Zhong, Z., Zhang, H. et al. Research on the Calculation of Initial streamer Length in High-Altitude Sphere-Plate Gap Based on the streamer Injection Development Model. 2024. DOI:10.1145/3702879.3702919 |
| 3. | Zhao, A., Fu, Z., Fu, Y. Simulation Analysis of Interphase Gap Breakdown Characteristics in UHV Transmission Lines with Consideration of Humidity Effects. 2024. DOI:10.1109/ICNEPE64067.2024.10860344 |
| Categories | No. | Issues to address | Comments |
| Particle extraction (collection) | (1a) | How to align and direct charged particles for efficient extraction and collection. | Could be challenge in closed field line system than in open field line system? |
| (1b) | The characteristics of charged particle losses and extraction within the electromagnetic field structure inherent to the ST. | To maximize the use of the default electromagnetic field structure, including ripple, RMP, ELM, etc. | |
| Energy conversion | (2a) | How to convert the kinetic energy of the collected charged particles. | Detail design of the DEC approach. |
| (2b) | How to convert and collect radiation energy and other forms of energy that cannot be directly converted into electricity. | To improve the overall efficiency. |
| Conversion scheme | Basic idea | Advantages | Disadvantages |
| Electrostatic conversion [2, 9] | Generating electricity through electrostatic fields created by plasma charge separation. | High energy conversion efficiency (for < 1 MeV). | Electrostatic pressure is prone to breakdown, and the output high-voltage direct current is difficult to convert and transmit. High-energy direct conversion still has drawbacks. |
| Inverse cyclotron converter [19, 20] | Generating electricity via the reverse process of a cyclotron accelerator. | Small size, saves materials and space, can be used for energy > 1 MeV. | May cause significant damage to electrode materials. |
| Electromagnetic conversion [9] | Generating electricity through induced currents from the expansion of plasma fusion. | Plasma generates electricity through electromagnetic induction without direct contact with the walls, reducing material corrosion issues. | Pulsed operation, with significant leakage losses at both ends of the device |
| Traveling-wave resonant absorption [15, 18] | Modulating a longitudinal wave in an ion beam, followed by generating electricity from an induced alternating potential through decelerating electrodes. | No issue with high-voltage breakdown, and the output alternating current energy is easy to transmit. Suitable for high-energy particles > 1 MeV. |
Low conversion efficiency, even lower conversion efficiency for low-energy particles. |
| Photon direct conversion | Converting the kinetic energy of high-energy particles into photons, then using photovoltaic cells to generate electricity. | High energy conversion efficiency | May introduce impurities into the plasma, not conducive to long-term stable high-power operation. |
| Toroidal field ripple | Prompt loss (%) | Delayed loss (%) | Total loss (%) |
| 0 | 1.894 | 0.004 | 1.898 |
| 1 (ripplemax ≈ 4.2×10−5) | 1.894 | 0.004 | 1.898 |
| 10 | 1.897 | 0.005 | 1.902 |
| 100 | 1.901 | 0.023 | 1.924 |
| 1000 | 1.954 | 0.943 | 2.897 |
| Attempted method | Specific operation | Summary of results |
| Equilibrium magnetic field conditions | Application of equilibrium magnetic field without additional perturbations | Alpha particle loss rate remains low, approximately 1.9%. |
| Apply of divertor voltage | Apply different levels of voltage to the upper right divertor target plate | Significant effect on alpha particle loss as potential increases to the order of megavolts. |
| Increase toroidal magnetic field | Increase in toroidal magnetic field strength | Modifications to the toroidal magnetic field do not exert a notable influence on alpha particle loss or collection. |
| Introduction of toroidal field ripple | Adjust the amplitude of the toroidal field ripple by a factor of 1000. | Under conditions with source terms and collisions, when the ripple amplitude increases to 1000 times, alpha particles are continuously lost, offering a steady source for their potential collection. |
