| Number | Ion source | PNBI (MW) | Ek (keV) | Rtan (m) | ϕ0 |
| A | H+ | 5 | 80 | 0.6–0.8 | 0° |
| B | H+ | 4 | 60 | 0.6–0.8 | 45° |
| C | H+ | 5 | 80 | 0.6–0.8 | 90° |
| D | H− | 3–4 | 200 | 0.6–0.8 | 180° |
| Citation: | Tiantian SUN, Xinchen JIANG, Zhi LI, Xiang GU, Xueyun WANG, Lili DONG, Danke YANG, Pengmin LI, Hanqing WANG, Shuo LIU, Yingying LI, Huasheng XIE, Yuejiang SHI, Yunfeng LIANG, Minsheng LIU, the EHL-2 Team. Characterization of fast ion loss in the EHL-2 spherical torus[J]. Plasma Science and Technology, 2025, 27(2): 024002. DOI: 10.1088/2058-6272/ad8dfb |
This study analyzes fast ion losses in the EHL-2 fusion device, focusing on both beam ions and alpha particles as p-11B fusion reaction products. Using the Monte Carlo orbit-following code TGCO, we evaluate particle confinement under various operational scenarios, including co-injected tangential neutral beam injection at beam energies of 60 keV, 80 keV, and 200 keV. Our simulations estimate the heat load driven by lost beam ions and find it to be within acceptable material limits for a plasma current on the order of mega-amperes. Additionally, we simulate the distribution of fusion products and observe a higher particle loss fraction for alpha particles compared to beam ions. However, due to the relatively low fusion power, these lost alpha particles are unlikely to significantly impact the plasma-facing materials. To assess the impact of the magnetic ripple, we compute the ripple field distribution by modelling the toroidal field (TF) coils as current filaments. The results indicate that the ripple field effect on particle confinement is minimal, primarily due to the large distance of over 1 m between the TF coils and the plasma on the low-field side. The analysis based on the test particle model is a foundational step in ensuring the basic safety aspects of the new device, which is essential for developing a robust design, optimizing performance, and maintaining safe operation.
The EHL-2 is a large spherical tokamak designed to explore proton-boron (p-11B) fusion energy [1, 2]. With objectives that include reaching a central ion temperature of 30 keV and validating the high ion temperature scenario, the EHL-2 is equipped with a powerful neutral beam injection (NBI) system [3, 4]. This system consists of four beamlines: three utilizing positive ion sources, delivering a combined 14 MW of power, and one employing a negative ion source, contributing an additional 3 MW. Under these high-power heating conditions, a significant amount of fusion reactions is expected to occur. Consequently, the plasma will contain a significant population of fast ions, including both the beam ions and the alpha particles produced as fusion products. A critical challenge for EHL-2 is the effective confinement of these fast ions.
Fast ion losses in tokamaks can occur due to various complex mechanisms [5, 6]. One such mechanism is charge exchange, where fast ions transfer electrons to neutral atoms, resulting in their neutralization and subsequent escape from the magnetic confinement. Collisions between fast ions and plasma particles or impurities can scatter the ions, leading to energy dissipation and eventual loss from the confined plasma region. The ripple field, which arises from the discrete nature of the magnetic coils, can cause variations in the toroidal magnetic field strength. These variations can induce drift in fast ions, leading them to escape along trajectories that take them out of the confined plasma [7, 8]. Magnetohydrodynamic (MHD) interactions, including resonances with Alfvén eigenmodes, neoclassical tearing modes, and sawtooth oscillations, represent another significant loss mechanism [9, 10].
Moreover, the presence of a large fraction of fast ions can fundamentally alter plasma behaviour. Fast ions can excite various MHD instabilities, such as Alfvén eigenmodes and fishbone modes [11]. These instabilities can, in turn, enhance fast ion transport and loss of these particles. Additionally, significant fast ion populations can modify the plasma current profile and pressure distribution, potentially affecting the overall plasma equilibrium and stability [12, 13].
The impact of these fast ion losses is significant. Firstly, they reduce the power deposited into the plasma, potentially compromising the heating efficiency required to maintain the high-temperature conditions necessary for fusion. Furthermore, the escaped fast ions can cause localized heat loads on the first wall of the tokamak and other components, increasing the risk of material erosion, melting, and long-term structural damage [14, 15].
This study analyzes fast ion losses for EHL-2 plasmas, focusing on the classical mechanisms that affect beam ions and alpha particles. Section 2 introduces the Monte Carlo orbit-following model used to simulate the trajectories of these ions within the plasma. The analysis of beam ion losses is detailed in section 3, and the behaviour and loss mechanisms of alpha particles are explored in section 4. The impact of the ripple field on fast ion confinement is described in section 5. Finally, the study concludes with a discussion of the results in section 6.
The calculation of fast ion losses involves the modelling of the interaction between fast ions and background plasmas. The Tokamak Guiding Center Orbit (TGCO) code is a simulation tool specifically designed to model the classical behaviour of fast ions within a tokamak plasma environment [16, 17]. The code has been rigorously benchmarked against the well-established NUBEAM code [18], ensuring its accuracy and reliability for simulating fast ion dynamics.
TGCO utilizes a Monte Carlo approach to capture the stochastic nature of fast ion interactions within the plasma. This method involves tracking the guiding center orbits of a large number of individual ions as they traverse the plasma, experiencing processes such as ionization, charge exchange, and collisions with plasma particles. The Monte Carlo method allows TGCO to produce a detailed statistical representation of fast ion behaviour, which is essential for accurately calculating energy deposition and identifying various loss mechanisms.
TGCO takes equilibrium magnetic configuration, plasma density, and temperature profiles as input. Additionally, the effect of boron is also considered in the simulation by introducing boron concentration, defined as fB=nB/(nB+nH), where nB and nH represent the densities of boron and hydrogen, respectively. This inclusion allows the code to account for the impact of boron on particle collisions, energy deposition patterns, and alpha particle yield, thus providing a more comprehensive and accurate simulation of EHL-2 plasmas.
Four beamlines are designed to be installed around EHL-2, labelled A through D, each with distinct injection parameters, as shown in table 1 and figure 1. The toroidal injection angles for beamlines A, B, C, and D are 0°, 45°, 90°, and 180°, respectively. The corresponding beam energies are 80 keV for beams A and C, 60 keV for beam B, and 200 keV for beam D. Beams A, B, and C are powered by positive ion sources, while beam D utilizes a negative ion source. Each beamline in the actual setup comprises four ion sources. For simplicity in this simulation, we represent each beamline with a single ion source that carries the total beam power. This approach reduces the complexity of the simulation while still capturing the essential physics. In the TGCO simulation, each beamline is modelled independently. This means that interactions between the different beams are not considered, focusing solely on the behaviour of each beamline in isolation. To initialize the simulation, we employ 50,000 statistical markers per beamline, each representing a portion of the injected particle population.
| Number | Ion source | PNBI (MW) | Ek (keV) | Rtan (m) | ϕ0 |
| A | H+ | 5 | 80 | 0.6–0.8 | 0° |
| B | H+ | 4 | 60 | 0.6–0.8 | 45° |
| C | H+ | 5 | 80 | 0.6–0.8 | 90° |
| D | H− | 3–4 | 200 | 0.6–0.8 | 180° |
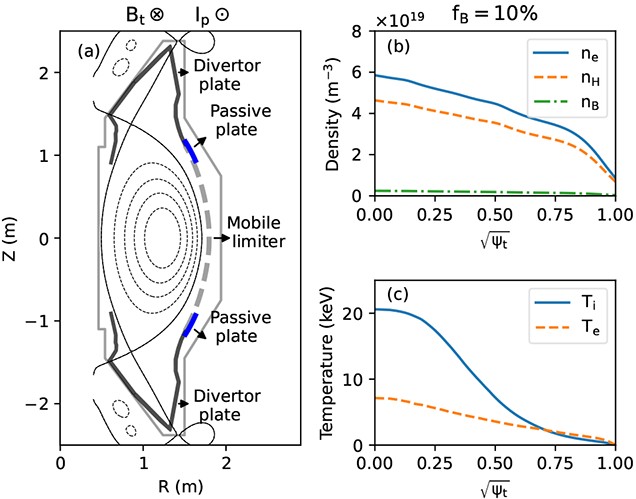
The EHL-2 spherical torus is designed to operate with a plasma current of up to 3 MA and a toroidal magnetic field of up to 3 T. The ion temperature is expected to reach up to 30 keV, with an electron density of 1020 m−3. The device is also flexible, allowing operation under various scenarios to optimize plasma performance and operation conditions [3]. In this section, we analyze beam ion losses under moderate to relatively low plasma conditions in order to identify the potential worst scenarios, such as significant energy loss that could lead to reduced plasma performance. As shown in figure 2, the simulation parameters are as follows: the ion temperature Ti is 20 keV and electron density ne is 6×1019 m−3. The magnetic equilibrium is a double null-divertor configuration with a toroidal field Bt = 2 T and plasma current Ip = 1.5 MA.
The initial energy distribution of injected neutral atoms differs significantly between positive ion sources at 60 keV and 80 keV and negative ion sources at 200 keV. In the case of positive ion sources, the beam exhibits three energy components corresponding to the full energy, half energy, and one-third energy of the injected atoms. In contrast, the negative ion source produces a monoenergetic beam, as shown in figure 3.
High-energy neutral atoms are injected into the plasma at their initial full energy, as well as at half and one-third of the energy. We track the orbits of these particles as they interact with the background plasma through slowing down and pitch angle scattering. As a result of slowing down, the particles gradually lose energy. When the energy of a particle drops below a threshold of several times the ion temperature, it is no longer tracked and is considered to have thermalized. In this simulation, tracking is terminated when the energy falls below 1.5 times the ion temperature (1.5Ti), which corresponds to 30 keV. As beam ions lose energy, they also undergo pitch angle scattering due to collisions with the background plasma. This scattering alters the direction of their motion relative to the magnetic field, leading to a broadening of their distribution in pitch space, where pitch is defined as λ=v||/v.
In the integrated design of the divertor and passive plate (PP) shown in figure 2, a mobile limiter (ML) is strategically placed in front of the PP to mitigate interactions between the plasma and the PP. To evaluate the effectiveness of this configuration, we estimate beam ion loss and wall load under different positions of the ML, with the distance between the last closed flux surface (LCFS) and the ML defined as dR.
When the ML is positioned close to the plasma, at dR=30 mm, the lost beam ions from all beamlines (60 keV, 80 keV, and 200 keV) are primarily deposited onto the ML, as shown in figure 4. This configuration results in a maximum wall load of 100 kW/m2, which occurs on the 80 keV beamline due to its highest injection power of 5 MW. Despite the concentration of lost ions, this level of heat load remains within the tolerable limits for a tungsten-made facing material, which can endure a maximum wall load of up to 10 MW/m2 [20]. This indicates that the ML effectively intercepts the lost ions and protects the PP and other components from excessive thermal stress.
As the ML is moved further away from the plasma, to dR=60 mm, the pattern of lost ion deposition shifts. In this scenario, the 60 keV and 80 keV beam ions are primarily deposited on the top divertor target plate, as shown in figure 5. However, the 200 keV beam ions, with a gyro radius of approximately 50 mm at the LCFS, are still partially intercepted by the ML. This indicates that high-energy ions can still be lost on the ML even as it is moved away from the plasma. The shift in deposition location also reveals a broader distribution of lost particles, particularly in the top region of the device. This is attributed to the magnetic gradient and curvature drift, where the particle drift velocity vD is aligned with the direction of B×∇B, given that the toroidal magnetic field runs anticlockwise when viewed from the top of the device as shown in figure 1.
We examine the relationship between the relative distance dR of the ML from the plasma and particle loss fraction. As shown in figure 6, the beam ion loss fraction increases as the ML moves closer to the plasma, which aligns with expectations. When dR is reduced to 10 mm, the loss fraction remains moderate and within acceptable limits, with approximately 2.2% of 200 keV beam ions, 1.4% of 80 keV beam ions, and 1.2% of 60 keV beam ions being lost. These values indicate that, even at this close proximity, the ML effectively manages beam ion loss, maintaining a moderate level of losses across different beam energies. Similar findings have been observed in studies on EAST [21], where a smaller gap between the limiter and the LCFS led to increased beam ion losses, further confirming the importance of optimizing this distance for effective fast ion confinement.
On the other hand, the choice of injection approach in NBI can significantly affect beam ion behaviour and overall plasma performance. In table 2, we investigate how different NBI injection methods: tangential co-injection, perpendicular co-injection, and tangential counter-injection, affect beam ion losses in a plasma with a beam power of 5 MW and an energy of 80 keV. The simulation results indicate that altering the injection geometry leads to markedly different outcomes in terms of particle confinement, loss fractions, and current drive efficiency.
| Injection type | Rtan (cm) | PNBI (MW) | Ek (keV) | floss (%) | ftrap (%) | Ifast (kA) |
| Perpendicular co-injection | 1 | 5 | 80 | 1.1 | 89.9 | 73 |
| Tangential co-injection | 80 | 5 | 80 | 0.038 | 32.8 | 407 |
| Tangential counter-injection | 80 | 5 | 80 | 3.8 | 36.8 | −287 |
As shown in table 2, when the tangential radius is set to a small value Rtan=1 cm to simulate a perpendicular injection, the fraction of trapped particles drastically increases from 32.8% (for tangential injection) to 89.9%, and the loss fraction rises from 0.038% to 1.1%. Moreover, the current drive efficiency is significantly reduced from 407 kA to 73 kA, demonstrating a substantial decline in the effectiveness of the current drive. In the case of tangential counter-injection, the trapped particle fraction also increases, though less dramatically, to 38.8%, while the total loss fraction rises to 3.8%. The driven current becomes negative at −287 kA, indicating that it opposes the direction of the plasma current.
Both perpendicular and tangential counter-injection approaches lead to increases in the particle loss fraction and trapped fraction, while also reducing current drive efficiency. These changes are undesirable for optimal plasma performance. Consequently, these results highlight the importance of selecting the appropriate injection method to maintain efficient plasma operation and optimize confinement.
Another important factor influencing particle confinement is the plasma current. As shown in figure 7, when the plasma current Ip is reduced from 1.5 MA to 500 kA, the loss fraction increases to 35.25%, 9.87%, and 5.92% for 200 keV, 80 keV, and 60 keV beam ions, respectively. These results highlight the critical role of plasma current in maintaining effective plasma confinement.
Overall, under the current magnetic configuration, particle confinement is well-maintained, particularly in terms of orbit loss. The observed loss fractions are relatively low, suggesting that the ML plays a crucial role in limiting the impact of beam ion loss on the device. However, it is important to note that significant particle losses could occur during MHD events, which can disrupt the stable confinement and potentially lead to higher loss fractions. Therefore, while the system is robust under normal operating conditions, it remains sensitive to plasma instabilities that may require additional considerations in future designs and operational strategies.
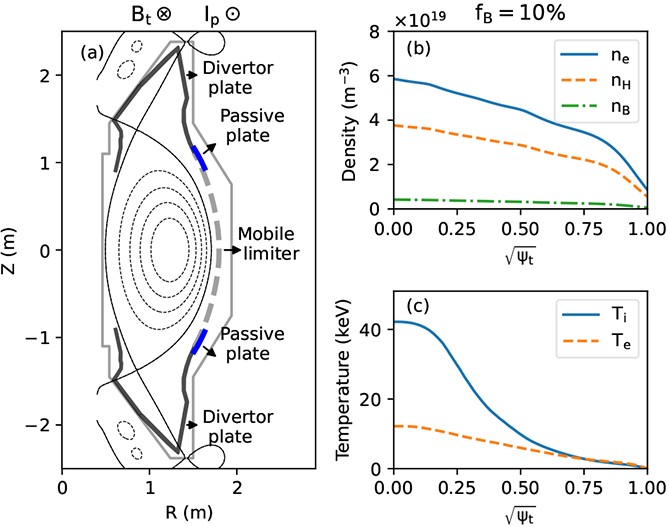
The loss of alpha particles is examined in the high ion temperature scenario which is discussed in reference [3]. The simulation is conducted under typical plasma parameters as shown in figure 8, where the ion temperature Ti is significantly higher than the electron temperature Te, with a ratio Ti/Te>3. The plasma is characterized by a high ion temperature at core Ti0>40 keV and an electron density of 6×1019 m−3. The toroidal magnetic field is set at 3 T, and the plasma current is maintained at 3 MA. These parameters are selected to represent a scenario where the plasma conditions are more extreme than those used in simulations of beam ion losses. Under these conditions, a small amount of p-11B fusion reactions is expected to occur, resulting in the generation and subsequent loss of alpha particles. This investigation aims to provide insights into alpha particle behaviour under such plasma conditions, which are crucial for understanding and optimizing the EHL-2 design.
In Monte Carlo simulations of the p-11B fusion reaction, the initial position and energy distribution of alpha particles are set as follows. The population of alpha particles is closely associated with the fusion reaction rate, R12=nHnB<σv>, which represents the number of fusion reactions occurring per unit volume and time. Here, nH and nB denote the densities of the reactant hydrogen and boron ions, respectively, while the symbol
Two types of fusion rates are illustrated in figure 9. For thermal reactions, both hydrogen and boron ions are assumed to follow Maxwellian distributions. The fusion rate in this case is more localized at ρ<0.2, as it is strongly dependent on the plasma temperature, which peaks near the core of the plasma. Beyond ρ>0.2, the ion temperature drops sharply, leading to a corresponding decrease in the fusion rate. Here, ρ is defined by toroidal flux ρ=√ψt. In the case of beam-target fusion, boron ions are still treated as Maxwellian, while the hydrogen distribution is derived from beam injection simulations, as discussed in the previous section. The fusion rate R12 decreases with increasing ρ, aligning with the distribution of fast ion deposition.
The initial energy distribution of alpha particles in this study is based on the characteristics of the p-11B fusion reaction. Each p-11B fusion event generates three alpha particles, releasing a total of 8.7 MeV of energy as kinetic energy carried by the alpha particles. However, this energy is not distributed uniformly among the alpha particles produced. Experimental results have demonstrated that the energy of the reaction products from p-11B fusion exhibits a non-monotonous distribution [22]. To accurately represent these experimental observations, the initial energy of alpha particles in the simulation is sampled according to these experimentally derived energy spectra [23]. Figure 10 illustrates the initial energy spectrum of the alpha particles used in the simulation. By using this approach, the simulation can effectively capture the non-uniform and complex energy distribution of alpha particles, thereby providing a more realistic representation of the physical phenomena involved in p-11B fusion reactions.
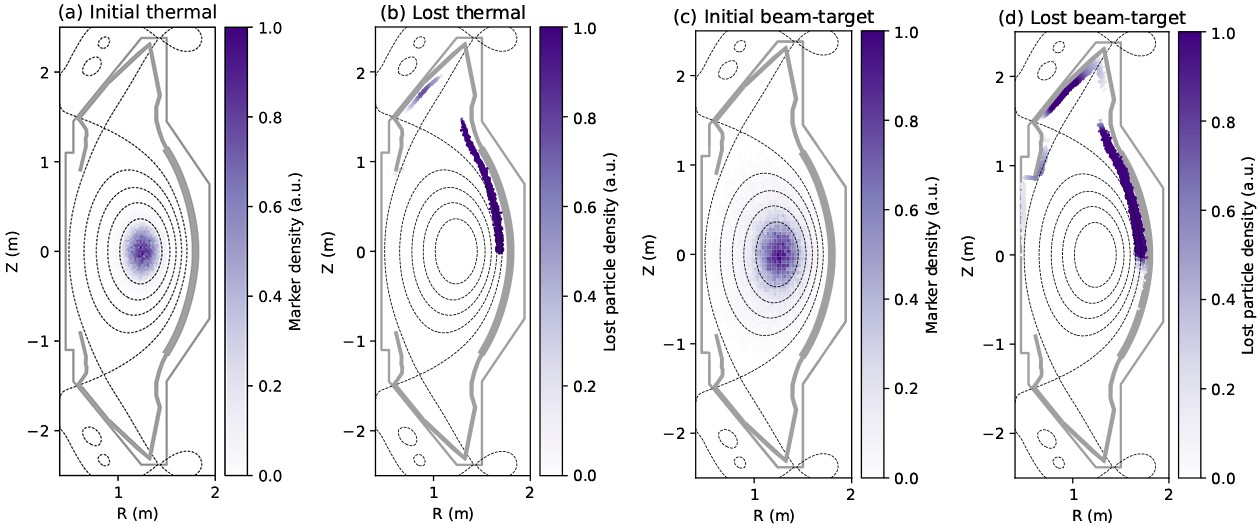
In the high ion temperature scenario provided [3], both thermal fusion and beam-target fusion contribute to the overall fusion power, with respective values of 290 W and 1271 W. However, the loss fractions differ significantly, with the thermal fusion power experiencing a 3.78% loss, while the beam-target fusion power suffers a higher loss fraction of 18.86%. The evaluation of particle loss is conducted with a limiter positioned dR=10 cm in front of the PP. Despite the relatively high particle orbit loss for beam-target fusion, the heat load on plasma-facing components remains within acceptable limits. This is primarily due to the moderate total fusion power output.
As illustrated in figure 11, a portion of losses occurs at the top divertor plates due to magnetic drift effects, and some alpha particles are lost at the outer limiter due to their gyro radius, which exceeds the 10 cm distance between the limiter and the LCFS. Figure 12 illustrates the gyro radius of alpha particles with perpendicular energies ranging from 1 to 6 MeV, calculated using the magnetic configuration shown in figure 8. At the LCFS radius of 1.55 m, the gyro radius spans from 12 to 26 cm, significantly larger than the 10 cm gap. This large gyro radius leads to particle loss as alpha particles approach the LCFS.
This disparity between the gyro radius and the limiter-LCFS distance is the key factor contributing to particle losses. When alpha particles travel near the LCFS, their large gyro radii cause them to interact with the limiter, resulting in their loss from the plasma. This phenomenon underscores the delicate balance required in fusion device design, where the confinement of energetic particles must be weighed against other operational and engineering constraints.
The histogram in figure 13 provides a comparative analysis of thermal and beam-target alpha particle losses. The average loss energy, particle loss counts, and average loss time are plotted as a function of poloidal angle θ. The poloidal angle, ranging from −π to π radians, is calculated relative to the magnetic axis coordinates (R0, Z0) and provides a spatial reference for the particle losses around the tokamak: θ=arctan((Z−Z0)/(R−R0)).
As shown in figure 13, the lost energy for both thermal and beam-target alpha particles generally falls within the 3–4 MeV range, and this energy loss is nearly uniformly distributed along the poloidal angle. However, when considering the particle loss counts, there is a peak at the top divertor plate, labelled as ‘TOP’. Despite this peak, the total particle loss count is higher on the low-field side (LFS), where alpha particles interact more frequently with plasma-facing components, particularly in the beam-target case.
The third subfigure illustrates the average loss time of alpha particles. Two distinct time scales are observed: a fast loss occurring within 10−7 to 10−6 s, primarily in the lower half of the LFS (θ = −1.1 to 1.2 rad) and the upper half of the high-field side (HFS) (θ = 2 to 3.14 rad), and a slower loss happening over 10−4 to 10−3 s. The fast losses correspond to first-orbit losses, which account for less than 1% of total losses. The majority of losses occur during the alpha particle slowing-down process as they collide with background plasma. The lost particle position and energy provide important information for alpha particle measurements and fusion yield evaluation.
A key component of EHL-2’s design is its toroidal field (TF) coils, which are responsible for generating the toroidal magnetic field that confines the plasma within the vacuum vessel. On EHL-2, a set of 16 D-shaped cupper TF coils is symmetrically arranged around the torus, creating a toroidal magnetic field up to 3 T at geometric center R0 = 1.05 m. However, the discrete nature of the TF coils introduces a variation in the magnetic field strength, known as the toroidal field ripple. The ripple amplitude δ(R, Z) is defined as the relative variation in the magnetic field strength between the maximum and minimum points around the torus:
| δ(R, Z)=Bmax−BminBmax+Bmin. | (1) |
To calculate δ(R, Z), we divide each TF coil into multiple current-carrying filaments. These filaments represent discrete sections of the coil, each contributing to the overall magnetic field. The magnetic field produced by each filament is calculated using Biot-Savart’s law. Once the magnetic field contributions from all individual filaments are determined, they are summed to obtain the total magnetic field at various points around the torus. This superposition of fields takes into account the spatial configuration of the filaments, leading to a detailed map of the magnetic field distribution.
In the EHL-2 tokamak, the design and positioning of the TF coils are carefully optimized to minimize the ripple field amplitude. Figure 14 provides a schematic diagram of the poloidal cross-section of the device, showing the relative locations of the plasma, poloidal field (PF) coils, center solenoid coils, and TF coils. The detailed dimension chain indicates that the distance between the plasma and the TF coils exceeds 1 m. A 2D calculation of the ripple field amplitude using 16 TF coils is also shown in the figure. At the LCFS, located at a major radius of RLCFS=1.55 m, the ripple amplitude is calculated to be less than 0.01%.
The impact of the number of TF coils on the ripple amplitude is further explored in figure 15. As the number of TF coils NTF decreases, the ripple amplitude increases. When NTF is reduced to 10, the ripple amplitude rises to approximately 0.35%, which is much higher than the ripple amplitude observed with 16 coils. This increase in ripple field can potentially lead to enhanced fast ion losses, as the magnetic field perturbations become more pronounced. Fast ions are particularly sensitive to ripple fields, as these perturbations can cause them to deviate from their intended orbits, resulting in losses from the plasma.
To quantify the effect of varying ripple amplitude on fast ion losses, calculations are performed for different numbers of TF coils, as presented in table 3. In this analysis, NTF varies between 10 and 16. Despite the increase in ripple amplitude with fewer TF coils, the fast ion losses exhibit only slight variations and remain relatively stable across the range of coil numbers. This indicates that, while a reduction in the number of TF coils leads to higher ripple amplitude, the overall fast ion confinement in EHL-2 remains effective and robust due to the relatively low ripple amplitude across all configurations studied.
| NTF | NBI-60 | NBI-80 | NBI-200 | αthermal | αbeamtarget |
| 10 | 0.0181 | 0.053 | 0.19 | 4.65 | 22.59 |
| 12 | 0.0165 | 0.043 | 0.12 | 4.32 | 20.91 |
| 14 | 0.0109 | 0.042 | 0.085 | 4.01 | 19.06 |
| 16 | 0.0101 | 0.037 | 0.082 | 3.73 | 18.82 |
The ripple amplitude is also influenced by the relative position of the plasma to the TF coils. To illustrate this effect, we calculate the ripple amplitude by varying the major radius of the LCFS from 1.4 to 1.7 m, as shown in figure 16. This range represents the potential locations of the plasma boundary within the tokamak. As RLCFS increases, the plasma edge moves closer to the TF coils, resulting in a corresponding increase in ripple amplitude at the plasma boundary. Despite this increase, the calculation shows that even when RLCFS is set to its extreme outer limit at 1.7 m, the ripple amplitude remains below 0.05%. This indicates that the ripple effect is kept at a low level.
To evaluate the ripple-induced losses under different equilibrium configurations, we use a series of designed magnetic configurations with different LCFS locations RLCFS, as shown in figure 17. In these configurations, RLCFS is varied between 1.5 m and 1.7 m by adjusting the PF coil current distribution while maintaining a plasma current of 3 MA and a toroidal magnetic field of 3 T.
The results of fast ion loss calculations for different equilibria are presented in tables 4 and 5, corresponding to configurations with 16 and 10 TF coils, respectively. The results indicate a slight increase in fast ion losses as RLCFS increases from 1.5 m to 1.7 m. However, these increases in fast ion losses remain comparable to cases without a ripple field, as the ripple amplitude stays below 0.05% across all configurations examined.
| RLCFS (m) | NBI-60 | NBI-80 | NBI-200 | αthermal | αbeamtarget |
| 1.50 | 0.009 | 0.035 | 0.083 | 3.72 | 18.80 |
| 1.55 | 0.0101 | 0.037 | 0.082 | 3.73 | 18.82 |
| 1.60 | 0.01015 | 0.039 | 0.087 | 3.81 | 18.92 |
| 1.65 | 0.0109 | 0.043 | 3.81 | 3.86 | 18.96 |
| 1.70 | 0.0115 | 0.049 | 18.92 | 3.92 | 19.06 |
| RLCFS (m) | NBI-60 | NBI-80 | NBI-200 | αthermal | αbeamtarget |
| 1.50 | 0.0178 | 0.05 | 0.184 | 4.37 | 22.01 |
| 1.55 | 0.0181 | 0.053 | 0.19 | 4.65 | 22.59 |
| 1.60 | 0.0186 | 0.059 | 0.195 | 4.97 | 23.21 |
| 1.65 | 0.0195 | 0.067 | 0.208 | 5.31 | 24.06 |
| 1.70 | 0.0202 | 0.072 | 0.224 | 5.76 | 24.98 |
Despite the variation in plasma boundary location and the number of TF coils, the fast ion losses exhibit only minor differences, suggesting that ripple-induced losses are minimal. The consistently low ripple amplitude, maintained below 0.05%, plays a critical role in preserving fast ion confinement, ensuring that overall plasma performance remains stable across the different equilibrium conditions. On EHL-2, the exceptionally low ripple amplitude is a direct result of the TF coil configuration. The TF coils are positioned farther away from the plasma, located on the outer side of the PF coils. This strategic placement significantly reduces the magnitude of the magnetic field ripple experienced by the plasma, ensuring that the magnetic field is more uniform in the toroidal direction.
In this study, we evaluated the fast ion losses of both beam ions and alpha particles in the EHL-2 tokamak using the Monte Carlo orbit-following code TGCO. The simulation considered four co-injected beamlines with energies of 80 keV, 60 keV, and 200 keV, with tangential radii between 0.6 and 0.8 m. For the beam ion losses, under moderate plasma parameters: magnetic field Bt=2 T, plasma current Ip=1.5 MA, ion temperature Ti=20 keV, and electron density ne=6×1019 m−3, the results show that beam ions are mainly lost at the mid-plane due to their interaction with the outer movable limiter, which is designed to protect the passive plates. Our analysis reveals that the particle loss fraction is directly influenced by the position of the limiter; as the limiter moves closer to the plasma, the loss fraction increases. However, when the limiter is positioned at dR=10 mm from the plasma edge, the loss fraction remains below 2.5%, indicating an overall acceptable loss level. The heat load on plasma-facing components caused by these lost particles is between 40−100 kW/m2, which is within the material tolerance limits. Nevertheless, the sensitivity of beam ion loss to plasma current is significant; a reduction in Ip to 500 kA results in a dramatic increase in the loss fraction for 200 keV beam ions to 32%, highlighting the importance of maintaining optimal plasma current levels to minimize losses.
Alpha particles, generated through both thermal and beam-target fusion reactions, show higher loss fractions due to their large gyro radii at MeV energies. The loss fraction for alpha particles from thermal reactions is 3.78%, with a heat load of 290 W, while beam-target reactions lead to a loss fraction of 18.86% and a heat load of 1271 W. Despite these losses, the total fusion power in the EHL-2 design is relatively low, and the resultant heat load remains within the acceptable limits for plasma-facing materials. The ripple field amplitude generated by the 16 sets of TF coils in the EHL-2 is below 0.01% at the last closed flux surface, indicating that the effect of ripple fields on particle loss is negligible.
While the classical losses of particles are manageable, potential losses induced by MHD effects could be significant. The current study does not consider MHD-induced losses, which remain a critical area for future research. Investigating these effects will provide a more comprehensive understanding of fast ion behaviour and loss mechanisms in the EHL-2 tokamak, which is essential for optimizing device design and operational parameters.
This work was supported by ENN Group and ENN Energy Research Institute. The authors would like to express their gratitude for the contributions of the ENN fusion team and collaborators in supporting this endeavour. We are thankful to Dr. Wei Chen, Dr. Zhengxiong Wang, Dr. Feng Wang, and Dr. Baolong Hao for their valuable discussions on this topic. Special thanks are extended to Dr. Youjun Hu for developing the open-sourced code TGCO and granting permission for its use in this research. The authors also acknowledge the support of Beijing PARATERA Tech Corp., Ltd. for providing HPC resources that have contributed to the research results reported in this paper.
| [1] |
Liu M S et al 2024 Phys. Plasmas 31 062507 doi: 10.1063/5.0199112
|
| [2] |
Liang Y F et al 2024 Plasma Sci. Technol. in press (https://doi.org/10.1088/2058-6272/ad981a
|
| [3] |
Wang X Y et al 2025 Plasma Sci. Technol. in press (https://doi.org/10.1088/2058-6272/ada9c3
|
| [4] |
Jiang X C et al 2025 Plasma Sci. Technol. in press (https://doi.org/10.1088/2058-6272/adae71
|
| [5] |
Fasoli A et al 2007 Nucl. Fusion 47 S264 doi: 10.1088/0029-5515/47/6/S05
|
| [6] |
Heidbrink W W and White R B 2020 Phys. Plasmas 27 030901 doi: 10.1063/1.5136237
|
| [7] |
Tobita K et al 1994 Nucl. Fusion 34 1097 doi: 10.1088/0029-5515/34/8/I04
|
| [8] |
Xu Y F et al 2021 Plasma Sci. Technol. 23 095102 doi: 10.1088/2058-6272/ac0717
|
| [9] |
Fredrickson E D et al 2006 Nucl. Fusion 46 S926 doi: 10.1088/0029-5515/46/10/S09
|
| [10] |
McClements K G and Fredrickson E D 2017 Plasma Phys. Control. Fusion 59 053001 doi: 10.1088/1361-6587/aa626e
|
| [11] |
Heidbrink W W et al 2008 Phys. Plasmas 15 055501 doi: 10.1063/1.2838239
|
| [12] |
Zheng Z et al 2018 Plasma Sci. Technol. 20 065103 doi: 10.1088/2058-6272/aab262
|
| [13] |
Jiang Y et al 2021 Nucl. Fusion 61 116033 doi: 10.1088/1741-4326/ac26a4
|
| [14] |
Sharapov S E et al 2005 Nucl. Fusion 45 1168 doi: 10.1088/0029-5515/45/9/017
|
| [15] |
Fitzgerald M et al 2018 Nucl. Fusion 59 016004 doi: 10.1088/1741-4326/aaea1e
|
| [16] |
Hu Y J et al 2021 Phys. Plasmas 28 122502 doi: 10.1063/5.0069792
|
| [17] |
Hu Y J et al 2023 Phys. Plasmas 30 092507 doi: 10.1063/5.0158503
|
| [18] |
Pankin A et al 2004 Comput. Phys. Commun. 159 157 doi: 10.1016/j.cpc.2003.11.002
|
| [19] |
Gu X et al 2025 Plasma Sci. Technol. in press (https://doi.org/10.1088/2058-6272/adae72
|
| [20] |
Pitts A R et al 2019 Nucl. Mater. Energ. 20 100696 doi: 10.1016/j.nme.2019.100696
|
| [21] |
Li Y L et al 2023 Phys. Plasmas 30 092503 doi: 10.1063/5.0156815
|
| [22] |
Becker H W, Rolfs C and Trautvetter H P 1987 Z. Phys. A: At. Nucl. 327 341 doi: 10.1007/BF01284459
|
| [23] |
Li Z et al 2024 Laser Part. Beams 42 e5 doi: 10.1017/lpb.2024.2
|
| [1] | Zhanhong LIN, Feng WANG, Ming XU, Chaofeng SANG, Chen ZHANG, Zhengxiong WANG. Simulation of charge-exchange induced NBI losses on EAST[J]. Plasma Science and Technology, 2024, 26(9): 095103. DOI: 10.1088/2058-6272/ad56ca |
| [2] | Yingfeng XU, Debing ZHANG, Jiale CHEN, Fangchuan ZHONG. Simulations of energetic alpha particle loss in the presence of toroidal field ripple in the CFETR tokamak[J]. Plasma Science and Technology, 2022, 24(10): 105101. DOI: 10.1088/2058-6272/ac6fb6 |
| [3] | Yingfeng XU (徐颖峰), Youjun HU (胡友俊), Xiaodong ZHANG (张晓东), Xingyuan XU (徐行远), Lei YE (叶磊), Xiaotao XIAO (肖小涛), Zhen ZHENG (郑振). Simulations of NBI fast ion loss in the presence of toroidal field ripple on EAST[J]. Plasma Science and Technology, 2021, 23(9): 95102-095102. DOI: 10.1088/2058-6272/ac0717 |
| [4] | Xingyuan XU (徐行远), Yingfeng XU (徐颖峰), Xiaodong ZHANG (张晓东), Youjun HU (胡友俊), Lei YE (叶磊), Xiaotao XIAO (肖小涛). Simulations of first-orbit losses of neutral beam injection (NBI) fast ions on EAST[J]. Plasma Science and Technology, 2020, 22(8): 85101-085101. DOI: 10.1088/2058-6272/ab8973 |
| [5] | Kunihiro OGAWA, Mitsutaka ISOBE, Takeo NISHITANI, Sadayoshi MURAKAMI, Ryosuke SEKI, Hideo NUGA, Neng PU, Masaki OSAKABE, LHD Experiment Group. Study of first orbit losses of 1 MeV tritons using the Lorentz orbit code in the LHD[J]. Plasma Science and Technology, 2019, 21(2): 25102-025102. DOI: 10.1088/2058-6272/aaeba8 |
| [6] | Zhongtian WANG (王中天), Huidong LI (李会东), Xueke WU (吴雪科). Loss-cone instabilities for compact fusion reactor and field-reversed configuration[J]. Plasma Science and Technology, 2019, 21(2): 25101-025101. DOI: 10.1088/2058-6272/aaead9 |
| [7] | Zhen ZHENG (郑振), Nong XIANG (项农), Jiale CHEN (陈佳乐), Siye DING (丁斯晔), Hongfei DU (杜红飞), Guoqiang LI (李国强), Yifeng WANG (王一丰), Haiqing LIU (刘海庆), Yingying LI (李颖颖), Bo LYU (吕波), Qing ZANG (臧庆). Kinetic equilibrium reconstruction for the NBI-and ICRH-heated H-mode plasma on EAST tokamak[J]. Plasma Science and Technology, 2018, 20(6): 65103-065103. DOI: 10.1088/2058-6272/aab262 |
| [8] | QU Hongpeng (曲洪鹏). Ion-Banana-Orbit-Width Effect on Bootstrap Current for Small Magnetic Islands[J]. Plasma Science and Technology, 2013, 15(9): 852-856. DOI: 10.1088/1009-0630/15/9/03 |
| [9] | WU Guojiang (吴国将), ZHANG Xiaodong (张晓东). Calculations of the Ion Orbit Loss Region at the Edge of EAST[J]. Plasma Science and Technology, 2012, 14(9): 789-793. DOI: 10.1088/1009-0630/14/9/03 |
| [10] | LI Jibo(李吉波), DING Siye(丁斯晔), WU Bin(吴斌), HU Chundong(胡纯栋). Simulations of Neutral Beam Ion Ripple Loss on EAST[J]. Plasma Science and Technology, 2012, 14(1): 78-82. DOI: 10.1088/1009-0630/14/1/17 |
| Number | Ion source | PNBI (MW) | Ek (keV) | Rtan (m) | ϕ0 |
| A | H+ | 5 | 80 | 0.6–0.8 | 0° |
| B | H+ | 4 | 60 | 0.6–0.8 | 45° |
| C | H+ | 5 | 80 | 0.6–0.8 | 90° |
| D | H− | 3–4 | 200 | 0.6–0.8 | 180° |
| Injection type | Rtan (cm) | PNBI (MW) | Ek (keV) | floss (%) | ftrap (%) | Ifast (kA) |
| Perpendicular co-injection | 1 | 5 | 80 | 1.1 | 89.9 | 73 |
| Tangential co-injection | 80 | 5 | 80 | 0.038 | 32.8 | 407 |
| Tangential counter-injection | 80 | 5 | 80 | 3.8 | 36.8 | −287 |
| NTF | NBI-60 | NBI-80 | NBI-200 | αthermal | αbeamtarget |
| 10 | 0.0181 | 0.053 | 0.19 | 4.65 | 22.59 |
| 12 | 0.0165 | 0.043 | 0.12 | 4.32 | 20.91 |
| 14 | 0.0109 | 0.042 | 0.085 | 4.01 | 19.06 |
| 16 | 0.0101 | 0.037 | 0.082 | 3.73 | 18.82 |
| RLCFS (m) | NBI-60 | NBI-80 | NBI-200 | αthermal | αbeamtarget |
| 1.50 | 0.009 | 0.035 | 0.083 | 3.72 | 18.80 |
| 1.55 | 0.0101 | 0.037 | 0.082 | 3.73 | 18.82 |
| 1.60 | 0.01015 | 0.039 | 0.087 | 3.81 | 18.92 |
| 1.65 | 0.0109 | 0.043 | 3.81 | 3.86 | 18.96 |
| 1.70 | 0.0115 | 0.049 | 18.92 | 3.92 | 19.06 |
| RLCFS (m) | NBI-60 | NBI-80 | NBI-200 | αthermal | αbeamtarget |
| 1.50 | 0.0178 | 0.05 | 0.184 | 4.37 | 22.01 |
| 1.55 | 0.0181 | 0.053 | 0.19 | 4.65 | 22.59 |
| 1.60 | 0.0186 | 0.059 | 0.195 | 4.97 | 23.21 |
| 1.65 | 0.0195 | 0.067 | 0.208 | 5.31 | 24.06 |
| 1.70 | 0.0202 | 0.072 | 0.224 | 5.76 | 24.98 |