Effect of parallel resonance on the electron energy distribution function in a 60 MHz capacitively coupled plasma

1.   Introduction

Capacitively coupled plasma (CCP) is used widely in semiconductor manufacturing for processes such as etching, deposition, and sputtering [1–3]. Various frequencies are used in CCP, including 400 kHz, 2 MHz, 13.56 MHz, and 60 MHz. Generally, a low driving frequency is used to provide higher ion bombardment energy, while a higher driving frequency is used to achieve higher electron density. Many studies have used experiments and simulations to investigate the effects of frequency on CCP. For example, the experimental and theoretical results presented by Perret in 2005 [4] illustrated that increasing the driving frequency leads to higher ion fluxes and lower ion energy. Some experimental results [5–9] and simulated results [10–16] indicated that the electron/ion density increases as the driving frequency increases. As a high-frequency CCP, the 60 MHz CCP is typically operated at high RF power to achieve high electron density. In CCP, the plasma resistance between two electrodes is inversely proportional to the electron density [17]. Hence, at a low RF power, the electron density is low and the plasma resistance can be greater than the system resistance causing RF power losses in the impedance matching network and power feed line. Thus, the power transfer efficiency is high at low RF power. However, at high RF power, the electron density is high and the power transfer efficiency is lower than that at low RF power because the plasma resistance decreases with RF power [18]. As a result, the lower power transfer efficiency of the 60 MHz CCP at high RF power becomes a serious issue. Therefore, it is necessary to improve the power transfer efficiency of 60 MHz CCP at high RF power.

Various methods to improve the power transfer efficiency and to increase the electron density in CCP have been studied. In one study, a step-down transformer [19] was connected between the output of the matching network and the power electrode. In the study, a decrease in RF power loss in the matching network was observed, because the step-down transformer decreases the current flowing through the matching network. At the same time, the ferrite core resistance of the transformer becomes a new source of RF power loss. The RF power loss of ferrite core resistance is severe at high frequency because the ferrite core resistance increases with the driving frequency [20, 21]. A higher plasma density has been achieved in the hollow electrodes [22, 23]. This method focuses on the maximum excitation rate caused by the cross-firing of the electron beam, rather than the decrease of RF power loss in the matching network. Consequently, the higher electron density leads to lower plasma resistance and lower power transfer efficiency. An increase in the harmonic currents of the electrode current caused by an impedance control circuit was observed in the experiments [24, 25], and this growth results in an increase in electron density due to the nonlinear electron resonance heating by a resonance between the impedance control unit and the plasma. However, the effect of the harmonic resonant growth on the electron energy distribution function (EEDF) was not investigated. Moreover, the plasma generation was enhanced through a parallel resonance achieved by using a parallel inductor in a 13.56 MHz CCP [26]. An increase of electrode current at the parallel resonance condition was observed, and a parallel resonance circuit model was described in this research; however, it is necessary to study the effect of parallel resonance on the electron heating dynamics.

In this work, a variable vacuum capacitor (VVC) is connected in parallel with a 60 MHz CCP to improve the power transfer efficiency. The CCP consists of a power feed line (usually denoted as an inductor), the electrodes, and the plasma. As the reactance of the CCP measured is positive, the CCP can be regarded as an inductive load. A parallel resonance between the VVC and the inductive load is observed. When the capacitance of the VVC approaches the parallel resonance conditions, the equivalent resistance of the parallel resonance circuit is considerably larger than that without the VVC, and the current flowing through the matching network decreases greatly. Consequently, the power transfer efficiency of the discharge is improved. To investigate the effect of the parallel resonance on the electron heating dynamics, the EEDFs without the VVC and with the VVC are measured. At the parallel resonance conditions, due to the effect of parallel resonance, the electron heating in bulk plasma is strongly enhanced, the shape of EEDF evolves from a bi-Maxwellian to a distribution with the smaller difference between high-energy electrons and low-energy electrons, the rate constants of excitation and ionization reactions increase, and the electron density is higher than that in the case without the VVC.

2.   Experiment setup

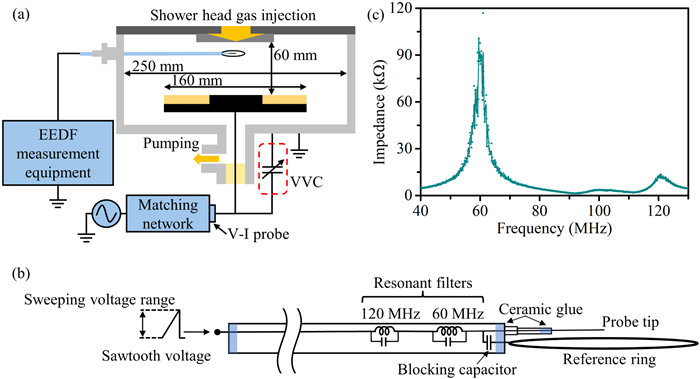
Figure 1(a) shows the experimental schematic diagram. A 60 MHz power generator (MKS, EDGE 6060A) and an automatic matching network (MKS, PF2-TRPL-AG76P-2) are used for RF power supply and automatic impedance matching respectively. A V–I probe is used at the output of the matching network to measure the impedance and the current at that position. The voltage (V), the current (I), and the phase between them (φ) are measured, and the resistance (R) and the reactance (X) are calculated according to the formulas $R=(V/I)\cos \phi$ and $X=(V/I)\sin \phi ,$ respectively. The inner diameter of the cylindrical chamber is 250 mm, and the height from the powered electrode (diameter of 160 mm) to the opposite shower head for gas injection is 60 mm. The top electrode and the powered electrode are electrically floating because of the dielectric film coatings on them, and the sidewall of the chamber is grounded. The CCP consists of a power line, the electrodes, and the plasma. A variable vacuum capacitor (VVC, Comet, CVLI-30BC/15) is connected in parallel with the CCP. The experiment is conducted with argon gas, and pressure is maintained at 30 mTorr by a pumping system.

Figure  1.  The experimental schematic diagram. (a) The diagram of the chamber including the connection of the VVC, (b) the circuit diagram of the single Langmuir probe, and (c) the impedance of the resonant filters used in probe.

DownLoad: Full-Size Img PowerPoint

An RF-compensated single Langmuir probe is placed at the center of the chamber [27]. As shown in figure 1(b), the probe is composed of a tungsten probe tip, which is 0.1 mm in diameter and 10 mm in length with a floating loop reference probe and resonant filters (60 and 120 MHz). The reference ring is 1 mm in diameter and 15 cm in total length, and series connected with a blocking capacitor of 100 pF capacitance. Considering that the sheath length at the probe tip and the reference ring is about 300 μm, the total impedance of the probe tip (including the reference probe) at 60 MHz and 120 MHz is approximately 216 Ω and 108 Ω, respectively. The impedance of the resonant filters in the frequency ranges from 40 to 130 MHz was measured by a network analyzer (Agilent 8735ES) and presented in the figure 1(c). The impedance of the resonant filters is larger than 80 kΩ and 11 kΩ in the frequency range of 59.5–60.5 MHz and 119.5–120.5 MHz, respectively. Since the impedance of resonant filters is much larger than the impedance of the probe tip, the compensation of the RF signal is acceptable. The 10 Hz sawtooth voltage was biased to the probe, and the probe current (I) is obtained by averaging at least 50 times. The second derivative of the probe current ($I\text{'}\text{'}$) relative to probe potential, which is proportional to the electron energy probability function (EEPF) f_p(ε), is obtained from numerical differentiation and smoothing by using the AC superposition method [28, 29]. The EEPF is related to the EEDF f_e(ε) = ε^1/2f_p(ε), and EEDF is obtained from the equation as follows [2]:

where m_e, e, and A are the electron mass, charge, and probe area, respectively. The electron density (n_e) and the average energy (⟨ε_e⟩) be calculated from the EEDF as follows [2]:

where ε_max is the maximum electron energy in the calculation.

3.   Results and discussion

3.1   Impedance and current

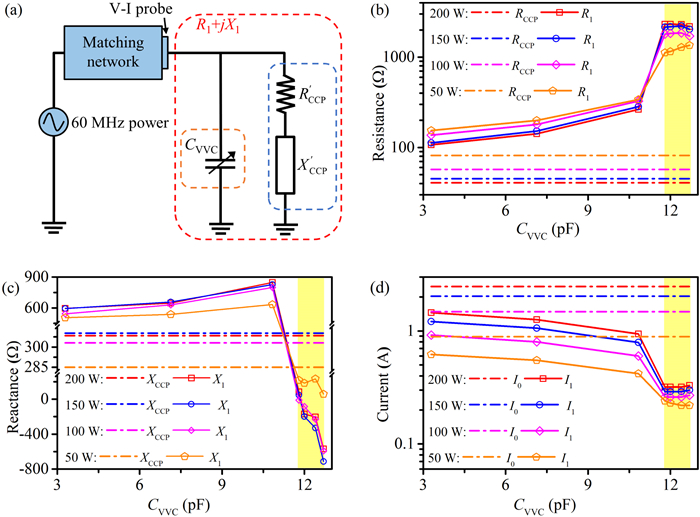
The variations of the resistance without the VVC and the reactance without the VVC with the increasing RF power are presented in figures 2(a) and (b). The results can also be considered as the resistance of the CCP (R_CCP) and the reactance of the CCP (X_CCP). Since the plasma resistance between two electrodes is inversely proportional to the electron density, the higher the RF power supplied, the smaller the R_CCP observed in the experiment. This is consistent with the results of research published in 1991 by Godyak [17]. The decrease in R_CCP means that the power transfer efficiency of this discharge system is lower at higher RF power. X_CCP depends mainly on the inductance of the power feed line and the capacitance of the plasma. At a low driving frequency, such as 13.56 MHz, X_CCP is generally negative. However, at a high driving frequency (60 MHz), since the resonant frequency of the power feed line inductance and the plasma capacitance is lower than 60 MHz, X_CCP can be positive and can act like an inductive load. Because the inductance of the power feed line does not change with the RF power but the capacitance of plasma increases with the RF power, as observed in the previous research [17], X_CCP increases with the RF power.

Figure  2.  Variations in impedance of the CCP without the VVC as a function of RF power: (a) R_CCP versus RF power and (b) X_CCP versus RF power.

DownLoad: Full-Size Img PowerPoint

To improve the reduction of the power transfer efficiency due to the decrease of R_CCP, the VVC is connected in parallel with the CCP, and the equivalent circuit is shown in figure 3(a). As the Q factor of the VVC is very high, the resistance of the VVC is neglected. The resistance of the CCP with the VVC and the reactance of the CCP with the VVC are presented by $R_{\mathrm{CCP}}^{\text{'}}$ and $X_{\mathrm{CCP}}^{\text{'}}.$ The VVC and the inductive load compose a parallel resonance circuit. The resistance with the VVC (R₁) and the reactance with the VVC (X₁) against the varying capacitance of the VVC (C_VVC) are shown in figures 3(b) and (c), respectively. R₁ and X₁ can be regarded as the equivalent resistance and the equivalent reactance of the parallel resonance circuit, respectively. The dash-dotted lines in figures 3(b) and (c) are R_CCP and X_CCP, respectively, at RF powers ranging from 50 to 200 W. R₁ is represented by solid lines in figure 3(b) and is larger than R_CCP. R₁ increases dramatically when C_VVC approaches approximately 12 pF at all the RF power conditions. When the RF power is 50 W, R₁ increases to approximately 1000 Ω, and R_CCP is 80 Ω. At an RF power of 200 W, R₁ grows to more than 2000 Ω, while R_CCP is 40 Ω. A more significant difference between R₁ and R_CCP is observed at the higher RF power. From the change in R₁, it also can be seen that the parallel resonance conditions of this parallel circuit are C_VVC from 11.8 to 12.7 pF, and the resonant region is marked by the light-yellow color in figures 3(b)–(d). At those capacitances, R₁ shows the highest level at each RF power condition. X₁ (solid lines in figure 3(c)) changes from positive (an inductive load) to negative (a capacitive load) with increasing C_VVC. For higher C_VVC, X₁ exceeds -800 Ω, therefore, a perfect impedance matching cannot be achieved, which means less than 1% reflected RF power condition. In figure 3(d), the dash-dotted lines represent the current measured without the VVC (I₀) at the different RF powers, and the current becomes larger as the RF power increases. The solid lines in figure 3(d) represent the current measured with increasing C_VVC (I₁). All these values of I₁ are smaller than I₀ under the same RF power condition, and I₁ falls to the lowest level when C_VVC approaches the parallel resonance conditions. The smaller I₁ means that at the parallel resonance conditions, the RF power loss in the matching network decreases, and the RF power absorbed by the CCP increases. The dramatic increase of R₁, the variation of X₁, and the decrease of I₁ are the typical characteristics of an LC parallel resonance circuit. Hence, this parallel resonance can provide the lower RF power loss at the matching network and optimize the power transfer efficiency of the discharge system.

Figure  3.  The CCP with the VVC and the equivalent resistance, the equivalent reactance, and the current measured at the matching network output. (a) The equivalent circuit of the CCP with the VVC, (b) R_CCP and R₁ versus C_VVC, (c) X_CCP and X₁ against with C_VVC, and (d) I₀ and I₁ versus C_VVC at different RF powers.

DownLoad: Full-Size Img PowerPoint

3.2   Results of EEDF

The EEPFs measured without the VVC at the different RF powers are presented by the black lines in figures 4(a)–(d). Under all the RF power conditions, the EEPFs show bi-Maxwellian distributions, which can be characterized by a low-temperature, low-energy electron group and a high-temperature, high-energy electron group. The low-energy electrons cannot overcome the ambipolar potential to reach the plasma-sheath interface where the stochastic heating in capacitive discharge takes place [2]. Therefore, the low-energy electrons remain in the bulk region and gain energy through ohmic heating; however, the lower electron-neutral collision frequency caused by the Ramsauer minimum effect of argon gas results in weak ohmic heating of the low-energy electron group. The temperature difference between the low-energy electrons and the high-energy electrons is large, and the collision cross section between the electrons is inversely proportional to the fourth power of their velocity difference [2]. Therefore, collisions between low-energy electrons and high-energy electrons are rare, so the low-energy electrons cannot gain energy through this collision. Consequently, the low-energy electron group remains at a low temperature. In contrast, the high-energy electrons can be strongly heated by both ohmic heating and stochastic heating. These are the characteristics of low-pressure argon discharge [27].

Figure  4.  EEPFs measured without the VVC and with C_VVC at parallel resonance conditions, with increasing RF power: (a) 50 W, (b) 100 W, (c) 150 W, and (d) 200 W. The black line is the EEPF without the VVC for each RF power condition.

DownLoad: Full-Size Img PowerPoint

As the electron energy distributions have a bi-Maxwellian distribution, the temperature of the low-energy electron group (T_{e, low}) and the temperature of the high-energy group (T_{e, high}) are calculated by the numerical fitting of the EEPFs. The form of the distribution function used for numerical fitting is the sum of two Maxwellian distribution functions with T_{e, low} and T_{e, high} respectively, as follows:

where A and B are the constants of the low electron temperature Maxwellian distribution and the high electron temperature Maxwellian distribution, respectively. The measured EEPF (f_p(ε)) in the electron energy range from 10 to 14 eV was used to obtain T_{e, high} and the constant B. Because in this energy range the high electron temperature Maxwellian distribution ($f_{\mathrm{h}}(\epsilon )=B\exp \left(-\epsilon /T_{\mathrm{e},\mathrm{high}}\right)$) can be considered to be 1 or 2 orders of magnitude larger than the low electron temperature Maxwellian distribution, therefore, f_p(ε) is almost equal to f_h(ε), and the second reason is that the threshold energy of the excitation reaction in argon discharge is 11.55 eV [2]. The EEPF without f_h(ε) calculated by $f_{\mathrm{p}}(\epsilon )-f_{\mathrm{h}}(\epsilon )$ is regarded as the low temperature Maxwellian distribution, and $f_{\mathrm{p}}(\epsilon )-f_{\mathrm{h}}(\epsilon )$ in the electron energy range from 2 to 5 eV was used to obtain T_{e, low} and the constant A, because of the large signal-to-noise ratio at this energy range. A numerical fitting example of EEPF without the VVC at RF power of 150 W is shown in figure 5(a). The blue dashed line and the magenta dashed line in figure 5 represent the two Maxwellian distributions with T_{e, low} for 0.72 V and T_{e, high} for 3.66 V respectively. Their sum is shown by the black dashed line as the fitting EEPF. The fitting EEPF is consistent with the EEPF measured without the VVC at RF power of 150 W, represented by the red solid line.

Figure  5.  The numerical fitting of the EEPF measured at RF power of 150 W. (a) The case without the VVC and (b) the case with C_VVC of 12.7 pF.

DownLoad: Full-Size Img PowerPoint

T_{e, low} and T_{e, high} calculated by the numerical fitting of the EEPFs with increasing RF power are shown in figure 6. With increasing RF power, both the RF power absorbed by the CCP and the current through the bulk region increase, therefore, the electric field intensity in the bulk plasma, which is proportional to the current density, also increases. In the bulk plasma, the reinforced electric field enhances the ohmic heating and leads to an increase of T_{e, low} with RF power. The decrease of T_{e, high} is due to the enlarged inelastic collision such as excitation and ionization reactions. The increase of T_{e, low} and the decrease of T_{e, high} with increasing RF power indicate that the EEPF evolves to the bi-Maxwellian distribution with a smaller difference between T_{e, low} and T_{e, high} due to the increase of the RF power absorbed by the CCP.

Figure  6.  T_{e, low} and T_{e, high} measured without the VVC as a function of RF power.

DownLoad: Full-Size Img PowerPoint

The EEPFs measured with C_VVC of 11.8 pF, 12.0 pF, 12.4 pF, and 12.7 pF at the different RF powers are represented by the other colored lines in figures 4(a)–(d). The parallel resonance conditions are C_VVC from 11.8 to 12.7 pF, as mentioned in section 3.1; consequently, the effect of the parallel resonance on the EEPF can be observed by the comparison between the EEPFs without the VVC and the EEPFs with the VVC. Figures 4(a)–(d) show that at the parallel resonance conditions all the EEPFs with the VVC evolve to a distribution with the smaller difference between T_{e, low} and T_{e, high}. This transition can also be verified through the changes in T_{e, low} and T_{e, high} at the parallel resonance conditions in figure 7. When C_VVC approaches to the parallel resonance condition, T_{e, low} and T_{e, high} are also calculated by the numerical fitting of the EEPFs, and the example of EEPF at C_VVC of 12.7 pF and RF power of 150 W is shown in figure 5(b). In this case, T_{e, low} and T_{e, high} are 1.21 V and 3.55 V, respectively, the difference between them is smaller than that in the case without the VVC. In figure 7, at all the RF power conditions, the T_{e, low} with the VVC increases compared to T_{e, low} without the VVC. This increase indicates that the electron heating in the bulk plasma is enhanced. Due to the effect of parallel resonance, the power transfer efficiency is optimized, and the RF power absorbed by the CCP is larger than that without the VVC. The larger RF power absorbed by the CCP leads to the enhancement of electron heating in the bulk plasma. This enhancement is similar to the effect of the increase of RF power mentioned before. Because of the larger RF power absorbed by the CCP, the reinforced electrical field in the bulk plasma enhances the ohmic heating and leads to higher T_{e, low}. However, at the parallel resonance conditions, the RF power absorbed by the CCP increases due to the optimized power transfer efficiency caused by the parallel resonance effect. But the enhancement of electron heating caused by the parallel resonance is more intense than the enhancement of electron heating due to the RF power increase without the VVC. T_{e, low} (0.91 V) without VVC at the power of 200 W is even lower than T_{e, low} (1.08 V) with C_VVC of 12.4 pF at the power of 50 W. This means that the higher T_{e, low} shown at the parallel resonance conditions cannot be achieved only by the increase of the RF power without the VVC in this experiment. Due to the parallel resonance effect, the low-energy electrons gain energy by the enhanced ohmic heating in the bulk plasma. Since the plasma electron oscillation frequency is much higher than the driving frequency, the ohmic heating per volume in CCP can be expressed as a function of electrode current density: $P=(1/2)J^2{\sigma }_{\mathrm{dc}}$ [2], where J is the electrode current density and σ_dc is the DC plasma conductivity expressed as (m_eν_m)/(n_ee²) with electron-neutral collision frequency of ν_m. J can be regarded as the electron current in bulk plasma and written as en_eu_e, u_e is the drift velocity of electrons, then the ohmic heating in plasma can be rewritten as: $P=(1/2)J(u_{\mathrm{e}}{m}_{\mathrm{e}}{\nu }_{\mathrm{e}}/e).$ That means that the ohmic heating in bulk plasma can be enhanced as long as the electrode current increases at the parallel resonance condition [26], regardless of the much higher n_e in industrial plasma. Furthermore, collisions between the low-energy electrons and the high-energy electrons at the parallel resonance conditions also occur more frequently than in the case without the VVC. These two factors result in the smaller difference between T_{e, low} and T_{e, high} in all EEPFs at the parallel resonance conditions. Since this evolution of the EEPFs, the population of electrons with energy greater than 4 eV also dramatically increases at the parallel resonance conditions compared to the case without the VVC. Therefore, the population of electrons that can participate in the reactions of the excitation, the ionization, and the generation of radical species significantly increases due to the parallel resonance effect.

Figure  7.  T_{e, low} and T_{e, high} obtained by numerical fitting for the EEPFs without the VVC and with C_VVC at parallel resonance conditions, with increasing RF power: (a) 50 W, (b) 100 W, (c) 150 W, and (d) 200 W.

DownLoad: Full-Size Img PowerPoint

At the different RF powers, the main difference in the parallel resonance effect on the EEPF is the change in n_e. In figure 8, the black dash-dotted line represents n_e measured without the VVC at different RF powers and the error bar of that is presented at the left end point of the black dotted lines, and the n_e measured with C_VVC from 11.8 to 12.7 pF are represented by the dark cyan solid line. In figure 8(a), at RF power of 50 W, all the points below the black dash-dotted line indicate that n_e decreases at the parallel resonance conditions. At the other RF power conditions, the higher the RF power, the more significant the increase of n_e that can be observed. At C_VVC of 12.7 pF, there are increments in n_e of 4%, 18%, and 21% at RF powers of 100 W, 150 W, and 200 W, respectively. The greater increase of n_e at the high RF power is due to the more remarkable improvement in the power transfer efficiency (η) at high RF power. η of the experiment system was measured by the method presented by M A Lieberman in 2005 [2], in which the RF power with discharge (P⁽¹⁾) and the voltage at the output of the matching network (V⁽¹⁾) are measured. Then, RF power of the generator is adjusted without discharge, when the voltage at the output of the matching network is the same as V⁽¹⁾, the RF power is recorded as P⁽⁰⁾. Therefore, η can be expressed as $(P^{(1)}-P^{(0)})/P^{(1)}\times 100.$ In figure 9, at different RF powers, the blue dash-dotted line represents η measured without the VVC, and the pink solid line is η with C_VVC from 11.8 to 12.7 pF. Due to the parallel resonance effect, η with C_VVC of 11.8 pF relative to that without the VVC improves from 76%, 70%, and 68% to 81%, 77%, and 76% at RF powers of 100 W, 150 W, and 200 W, respectively. As mentioned before, at the parallel resonance conditions, the difference between R₁ and R_CCP shows an upward trend concerning the RF power, and the effect of parallel resonance is more intense at the higher RF power. Therefore, the improvement on η also becomes more remarkable as the RF power increases. As the RF power increases, when C_VVC approaches the parallel resonance conditions, the more remarkable improvement of η means that both the RF power absorbed by the CCP and n_e increase more significantly. It can be expected that this parallel resonance effect will be more pronounced when discharging at kilowatt level. However, at RF power of 50 W, n_e decreases at parallel resonance conditions, and to understand the reason for this decrease in n_e the collisional energy loss per electron-ion pair created, ε_c, is calculated as follows [2, 30]:

where ε_iz, ε_ex, and M are ionization energy (15.76 V), excitation energy (11.55 V), and mass of argon atom, respectively. K_iz, K_ex, and K_el are the rate constant of ionization, excitation, and elastic collision reactions, and are calculated as follows [30, 31]:

σ(ε) represents the cross section for each collisional process [32, 33]. The results of ε_c without the VVC and at the parallel resonance conditions are presented in figure 10. ε_c at the RF power of 50 W (figure 10(a)) increases to about 18.3 V at the parallel resonance conditions in contrast to 17.3 V without the VVC. The change in ε_c at the RF power of 200 W (figure 10(d)) due to the parallel resonance is rare. Since the ratio of mass between the electron and the argon atom is too slight, the third term in the calculation of ε_c is less than 0.1 V in all the conditions and the contribution of that to the change in ε_c can be neglected. Therefore, the main change in ε_c is dependent on the ratio of K_ex and K_iz. At the parallel resonance conditions, as the population of electrons with energy greater than 4 eV dramatically increases, both K_ex and K_iz increase. At the RF power of 50 W, compared to the case without the VVC, K_ex/K_iz increases from 0.13 to 0.21 due to the parallel resonance; however, at the RF power of 200 W, K_ex/K_iz changes from 0.21 to 0.22. At the RF power of 50 W, because the improvement of η is not obvious and ε_c increases, n_e decreases at the parallel resonance conditions.

Figure  8.  The change in n_e under parallel resonance conditions at RF power of (a) 50 W, (b) 100 W, (c) 150 W, and (d) 200 W. The black dotted lines represent n_e without the VVC and the error bar of that is presented at the left end point of the black dotted lines.

DownLoad: Full-Size Img PowerPoint

Figure  9.  The power transfer efficiency (η) measured without the VVC and with C_VVC at the parallel resonance conditions. (a) 50 W, (b) 100 W, (c)150 W, and (d) 200 W.

DownLoad: Full-Size Img PowerPoint

Figure  10.  The collisional energy loss at the parallel resonance conditions at RF powers of (a) 50 W, (b) 100 W, (c) 150 W, and (d) 200 W. The black dash dotted lines in figure are the collisional energy loss without the VVC.

DownLoad: Full-Size Img PowerPoint

4.   Conclusion

In this work, parallel resonance between the 60 MHz CCP and the VVC is observed. At parallel resonance conditions, R₁ is two orders of magnitude larger than R_CCP, and I₁ is smaller than I₀. Therefore, the power loss in the matching network decreases, and the power absorbed by the CCP increases. Due to the parallel resonance effect, the electron heating in bulk plasma is strongly enhanced and the higher T_{e, low} that is cannot be observed without the VVC in this experiment is shown, therefore, the EEPF changes from the bi-Maxwellian distribution without the VVC to the distribution with the smaller difference between T_{e, low} and T_{e, high}. The population of electrons with energy greater than 4 eV dramatically increases, consequently, the rate constants of excitation and ionization reactions increase. When C_VVC approaches 12.7 pF, n_e increases by about 4%, 18%, and 21% compared to the values without the VVC at RF powers of 100 W, 150 W, and 200 W, respectively. The increase of n_e is more significant at the higher RF power because the improvement of η is more remarkable at the higher RF power. η improves from 76%, 70%, and 68% to 81%, 77%, and 76% at RF powers of 100 W, 150 W, and 200 W, respectively. At the RF power of 50 W, n_e decreases mainly due to the increase of collision energy loss at the parallel resonance conditions. When much higher RF powers are used, such as kilowatt discharge in industrial plasma chambers, a more pronounced effect of the parallel resonance can be expected.