Effects of power on ion behaviors in radio-frequency magnetron sputtering of indium tin oxide (ITO)

1.   Introduction

Indium tin oxide (ITO) films have diverse applications as transparent conductors in a range of optoelectronic devices, including display panels (such as touch screens, organic light-emitting devices (OLEDs), and liquid crystal displays (LCDs)), solar cells, and even in biomedical and flexible electronics applications. Their exceptional blend of electrical conductivity and optical transparency makes them a versatile choice in these fields [1‒4]. Depending on the specific application requirements and desired film properties, ITO thin films can be fabricated using various techniques, including magnetron sputtering (MS) [5, 6], chemical vapor deposition (CVD) [7], electron beam evaporation (EBE) [8], pulsed laser deposition (PLD) [8] and atomic layer deposition (ALD) [9].

Typically, direct current magnetron sputtering (DCMS) is the preferred method for large-scale industrial production of ITO layers because it offers high uniformity, good adhesion and precise control over the quality of the thin films. Sputtering the ITO target indeed presents challenges, primarily due to the potential for causing significant radiation damage to ITO films or any pre-deposited layers. The impact of high-energy particle bombardment can cause sputtering damages that negatively affect the performance of optoelectronic devices. For example, it can reduce the conversion efficiency of silicon heterojunction (SHJ) solar cells [10, 11] and cause severe degradation in OLEDs [12]. In recent decades, researchers have focused on understanding the behavior and origin of high-energy particles that impact substrates. Many of these studies have used energy-resolved mass spectrometers in the context of DCMS. They have highlighted that the primary source of these high-energy particles consists of sputtered negative ions (such as O⁻, ${\mathrm{O}}_2^-$, InO⁻, etc.). These ions are accelerated by the cathode sheath, carrying an energy level closely matching the target voltage, typically in the range of hundreds of electron volts (eV) [13, 14]. Furthermore, various efforts have been made to mitigate sputtering damages. These include techniques like high-pressure sputtering (HPS) [15] and the implementation of an external biased anode [16].

In contrast, radio frequency magnetron sputtering (RFMS) tends to have a lower deposition rate but offers the advantage of generating less damage to both the target and the films. This is due to the lower self-bias voltages in the presence of better volume ionization within the oscillating field. These characteristics can extend the lifespan of the target and enhance the crystallization in the deposited films [17, 18]. Due to the typically lower target voltages and sputtering rate in RFMS compared to DCMS, it is still reasonable to infer the reduction of the energy and flux of sputtered negative ions. This inference can be supported by a limited number of studies on other transparent conductive films, including ZnO and MgO [19, 20]. In these studies, negative O⁻ and ${\mathrm{O}}_2^-$ ions display significantly different ion energy distribution functions (IEDFs) characterized by multiple peaks, in contrast to the IEDFs observed in DCMS. The distinctive IEDFs are attributed by researchers to the combined influence of the target voltage’s RF oscillation and the plasma potential. It is important to highlight that their simulation results deviate from the measured data, particularly evident in the case of the IEDFs of molecular ${\mathrm{O}}_2^-$ ions. This discrepancy can likely be attributed to imprecise estimates of plasma potential and the omission of the initial energy of emitted particles. Nevertheless, it is worth noting that negative O⁻ and ${\mathrm{O}}_2^-$ ions with energies exceeding 100 eV remain detectable. Additionally, with increasing radio frequency, there is an increase in both energy and flux of backscattered ions. Given these factors, the impact of sputtering damages on the films cannot be disregarded in RFMS.

In this investigation, we utilized RF magnetron sputtering to fabricate ITO films, subjecting them to a range of power conditions. The ion behaviors at the substrate surface during the synthesis of ITO films were systematically analyzed using energy-resolved mass spectrometers and Langmuir probe. Our primary objective is to enhance our comprehension of the ion formation mechanism and the variables influencing their IEDFs and flux. In section 2, we detail the experimental setup and procedures. Section 3 presents an in-depth discussion of the results, and section 4 outlines our conclusions.

2.   Experimental setup

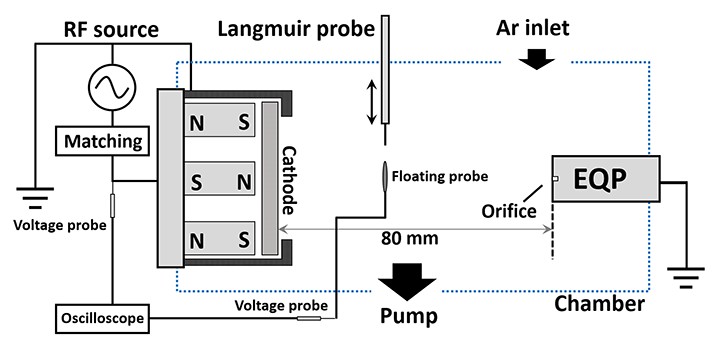
Figure 1 illustrates the RF magnetron sputtering (RFMS) device alongside the diagnostic systems. To the left, the magnetron is equipped with a planar ITO target composed of In₂O₃ (90wt%) and SnO₂ (10wt%), boasting a 90 mm diameter and 6 mm thickness. The magnetron is cased in a grounded stainless steel sheath and is driven by a 13.56 MHz radio frequency power supply (RSG2000S, Rishige). ITO film deposition is realized in a pure argon atmosphere at a constant flow rate of 150 mL min⁻¹ (pressure of 6.0×10⁻¹ Pa), while the discharge power varies from 25 to 100 W. Positioned on the right is the Electrostatic Quadrupole Plasma (EQP) analyzer (EQP 1000, Hiden), with its orifice grounded and aligned along the same axis as the target. The EQP analyzer boasts an independent vacuum system that maintains operational conditions at a meticulously maintained pressure of 5.6×10⁻⁵ Pa. The distance between the orifice and the target remains fixed at 78 mm. It is employed to analyze ion mass spectra and IEDFs for both positive and negative ions. The bulk plasma parameters at an axial distance of 30 mm from the target are determined using a radially adjustable Langmuir probe (Hiden, ESPion) equipped with a wide band compensation electrode. Simultaneously, a floating planar probe is mounted to measure the plasma potential. An oscilloscope (DSO-X 3014A, Agilent) fitted with voltage probes (P5100A, Tektronix) is utilized to record the oscillating floating potential and cathode voltage.

Figure  1.  Illustration depicting the DC magnetron sputtering setup utilized for ITO film deposition including the diagnostic systems.

DownLoad: Full-Size Img PowerPoint

3.   Results and discussions

3.1   Plasma discharge parameters

In figure 2(a), I-V characteristics obtained by the Langmuir probe are plotted and plasma potential ${V}_{\mathrm{P}}$ can be taken from the point where the probe current starts to deviate from exponential growth, that is, where the first derivative of the current, dI/dV is maximum [21]. In figure 2(b), the time-resolved cathode voltage, recorded by the oscilloscope across varying RF powers from 25 to 100 W, is presented. It is evident that the target voltage remains consistently negative throughout operation. Moreover, augmenting the RF power not only amplifies the negative self-bias but also escalates the amplitude. In figure 3(a), the electron density (n_e) and temperature (T_e) at an axial distance of 30 mm from the target are plotted against RF power ranging from 25 to 100 W. An observable trend reveals an increase in n_e from $6.4\times {10}^{15}$ to $1.6\times10^{16}\mathrm{\ m}^{-3}$ with rising RF power, while T_e exhibits a peak of 2.7 eV at 50 W. Figure 3(b) displays the self-bias cathode voltage (V_T0) and the floating potential (V_f) measured by the oscilloscope, along with the plasma potential (V_P) recorded via the Langmuir probe, plotted against RF power ranging from 25 to 100 W. It is evident that increasing RF power not only raises V_T0 but also induces a slight elevation in both V_P and V_f.

Figure  2.  (a) The first derivative of the I-V curves (shown in the inset illustration) measured by Langmuir probe. (b) Time-resolved cathode voltage recorded by oscilloscope with various RF power.

DownLoad: Full-Size Img PowerPoint

Figure  3.  (a) Electron density n_e and temperature T_e at an axial distance of 30 mm from the target with various RF power from 25 to 100 W. (b) The self-bias cathode voltage V_T0, the floating potential V_f and the plasma potential V_P depicted as functions of RF power.

DownLoad: Full-Size Img PowerPoint

Moreover, in figure 4, we present mass spectra recorded at an RF power of 100 W. For positive ions, it is evident that Ar⁺ and In⁺ ions predominate, surpassing the intensity of O⁺ and O²⁺ ions by approximately one to two orders of magnitude. In the context of negative ions, it is noteworthy that O⁻ ions are the most prevalent, while the ${\mathrm{O}}_2^-$ and InO⁻ ions exhibiting roughly one order of magnitude lower abundance. Therefore, a thorough examination of the IEDFs for common ions, such as Ar⁺, In⁺, O⁺, O⁻, ${\mathrm{O}}_2^-$, and InO⁻, has been carried out.

Figure  4.  Mass spectra obtained under an RF power of 100 W for (a) positive ions and (b) negative ions.

DownLoad: Full-Size Img PowerPoint

3.2   Positive ion mass spectra

In figure 5, IEDFs for positively charged Ar⁺, In⁺ and O⁺ ions are graphed across a range of powers from 25 to 100 W. The IEDFs for Ar⁺ and In⁺ ions display a distinct double-peak structures, which are commonly observed in radio-frequency discharges [22‒24]. In these studies, IEDFs are measured using retarding field energy analyzers with scanning biased grids. However, in the case of EQP analysis, a dedicated 45° sector field energy analyzer is employed for ion energy analysis. This distinction has the potential to introduce variations in the IEDFs and warrants careful consideration in the overall comparisons of the results. The ion plasma frequency f_pi plays a pivotal role in plasma physics, governing ion interactions with electromagnetic fields. It is generally represented as: f_pi = ω_pi/2π, where ω_pi = (4πn_iZ²e²/m_i)^1/2, it correlates to ion density (n_i), charge state (Z), and ion mass (m_i). Based on the plasma parameters detailed in reference [23], the f_pi is calculated to be 1.7 MHz for H mode operation and 5.4 MHz for W mode operation. In our investigation, for Ar⁺ ions, the estimated f_pi stands at 3.3 MHz, aligning seamlessly with the range indicated in reference [23]. This substantiates that our findings extend and align with the work in reference [23], where these dual peaks are attributed to plasma potential oscillations, with V_P typically positioned between the peaks in saddle-shaped IEDFs. However, in our works, V_P is closely associated with the primary high-energy peaks. The presence of double-peak structures cannot be explained solely by plasma potential oscillations, as suggested by this misalignment. To explore this further, we express the average ion transit time through the sheath (${\tau }_{\mathrm{i}}$) as a function of the ion acoustic speed (${c}_{\mathrm{s}}$):

Figure  5.  IEDFs for Ar⁺ (a), In⁺ (b), and O⁺ (c) ions in RFMS discharge at different RF power levels. The corresponding V_P measured by the Langmuir probe is indicated by the dashed red lines.

DownLoad: Full-Size Img PowerPoint

Here, ${k}_{\mathrm{B}}$ is the Boltzmann constant, ${T}_{\mathrm{e}}$ is the electron temperature, ${m}_{\mathrm{i}}$ is the ion mass. $D$ is the sheath width which can be estimated as follows:

where ${\varphi }_{\mathrm{s}}$ is the sheath voltage fall, ${\lambda }_{\mathrm{D}}$ is the electron Debye length which can be express as:

Here, $\varepsilon$ is the vacuum permittivity and ${n}_{\mathrm{e}}$ is the electron temperature. Utilizing parameters obtained from the Langmuir probe, including ${n}_{\mathrm{e}}$ of 6.5×10¹⁵ m⁻³, an electron temperature ${{k}_{\mathrm{B}}T}_{\mathrm{e}}$ of 2.7 eV, and setting ${\varphi }_{\mathrm{s}}$​ equal to V_P, ${\tau }_{\mathrm{i}}$ for Ar⁺, In⁺ and O⁺ ions can be estimated to be approximately 232.3, 393.3, and 147.5 ns respectively. These values exceed the period of the applied RF field, which is 73.7 ns at 13.56 MHz. This suggests that ions ionized within the bulk plasma undergo multiple RF field oscillations during transit through the sheath and align with the time-average sheath potential V_P [25]. As a result, IEDFs for Ar⁺, In⁺ and O⁺ ions show distinct peaks at approximately 20 eV, corresponding to V_P. As the power increases from 25 to 100 W, a slight blue-shift towards the high-energy region is noticeable, matching well with the rise in V_P from 20.0 to 22.7 eV.

Extended high-energy tails of Ar⁺ ions’ IEDFs can be attributed to incident Ar⁺ ions that are backscattered, neutralized just in front of the target surface, and subsequently ionized within the bulk plasma. Meanwhile, the asymmetry in the IEDFs of In⁺ and O⁺ ions towards the high-energy region stems from the sputtered In and O atoms retaining their initial energy upon ejection from the target. The factors responsible for the observed low-energy peaks around 5 eV in Ar⁺ ions remain unclear at this point. Resonant charge transfer collisions (${\mathrm{A}\mathrm{r}}^{+}+\mathrm{A}\mathrm{r}\to \mathrm{A}\mathrm{r}+{\mathrm{A}\mathrm{r}}^{+}$) represent one potential mechanism capable of generating these low-energy Ar⁺ ions [26]. However, the occurrence of charge exchange collisions within the Debye sheath is highly improbable due to the extensive mean free path under low neutral pressure. Furthermore, given the operational conditions of the EQP system at a low pressure of 5.6×10⁻⁵ Pa, where collisional mean free paths significantly exceed the size of the EQP, the likelihood of charge exchange and electron impact ionization within the EQP is also slim. To ascertain the true origin of these low-energy Ar⁺ ions, additional comprehensive investigations are warranted. The origin of the low-energy characteristic shoulder around 13 eV for In⁺ ions remains elusive. A similar structure has been observed in the IEDFs of Ti⁺ ions in a hollow cathode discharge, a phenomenon attributed to the dissociation of larger molecular ions [26]. However, in this study, since corresponding larger molecular ions cannot be detected in association with this dissociation process, the low-energy characteristic shoulder may be attributed to in-flight collisions with neutrals.

In figure 6(a), the integrated IEDFs for positive ions are illustrated across a spectrum of RF power. A notable elevation in the flux of positive ions is observed as RF power escalates from 25 to 100 W. This phenomenon can be attributed to the concurrent increase in sputtering rate and electron density, as the majority of these positive ions originate from ionization processes within the bulk plasma. The average kinetic energy of positive ions is depicted in figure 6(b). Each positive ion demonstrates an almost linear increase in average kinetic energy with the ascending RF power. The rise in ion energy is due to the higher plasma potential and initial energy upon ejection from the target.

Figure  6.  (a) The integrated IEDFs and (b) the average kinetic energies of positive ions at different RF powers.

DownLoad: Full-Size Img PowerPoint

3.3   Negative ion mass spectra

In figure 7, IEDFs of O⁻, ${\mathrm{O}}_2^-$, and InO⁻ ions are presented, demonstrating variations with increasing RF power from 25 to 100 W. The spectra for negative ions significantly differ from those for positive ions. Initially, rather than exhibiting single or double sharp peaks, a relatively broad distribution with successive weak peaks is observed. In previous studies [19, 20], this broad distribution has been attributed to the runtime effects arising from oscillating target and plasma potentials. This concept is embraced here, and IEDFs are computed using a straightforward one-dimensional model, relying on the following simplifying assumptions. (1) Target voltage V_T(t) and plasma potential V_P(t) oscillate harmoniously with matching frequency and but varying amplitude and time/phase shift. (2) V_P(t) is spatially uniform with constant amplitude and phase. (3) Sputtered negative ions are consistently emitted from the target at a constant rate. (4) Ions traverse the plasma without collisions. However, the calculated IEDFs of O⁻ ions using this model deviate from the measured data. The maximum attainable energy is significantly lower than the observed value due to the oversight of the initial energy of sputtered O⁻ ions, denoted as ${E}_{0}$. To overcome this limitation, the model is optimized by incorporating ${E}_{0}$, satisfying the well-established sputtered atom energy distribution function developed by Tompson [27]:

Figure  7.  IEDFs for O⁻ (a), ${\mathrm{O}}_2^-$ (b), and InO⁻ (c) ions in RFMS discharge at different RF power levels.

DownLoad: Full-Size Img PowerPoint

Here, $\gamma$ represents the energy transfer coefficient in a direct collision, which depends on the mass of the incoming ions and the target surface atoms. ${E}_{\mathrm{b}}$ denotes the binding energy of the target surface atoms, while $E\mathrm{_i}$ represents the energy of the incident Ar⁺ ions.

Signal-charged negative ions originating from the target undergo three distinct stages on their journey toward the EQP orifice. In the first stage, the ion accelerates from the target surface to the bulk plasma, attaining an energy level equivalent to the cathode sheath voltage fall:

In this context, ${V}_{\mathrm{T}}\left(t\right)={V}_{\mathrm{T}0}+{U}_{\mathrm{T}}\mathrm{s}\mathrm{i}\mathrm{n}\left(\omega t\right)$ represents the instantaneous potential of the target, where ${V}_{\mathrm{T}0}$ is the self-bias potential, ${U}_{\mathrm{T}}$ is the amplitude, ${t}_{1}$ is the transit time across the cathode sheath, and $\omega=2\mathrm{\rm{\pi}}f$ with $f$ being the driving frequency. In the second stage, the ion travels through the bulk plasma with a transit time ${t}_{2}$ which can be estimated using the following equation:

Here, D represents the distance from the target to the EQP probe, $v$ denotes the ion velocity upon entering the bulk plasma, and M represents the ion mass. Upon reaching the entrance of the anode sheath, the momentary plasma potential can be expressed as:

In the third stage, the ion decelerates from the bulk plasma to the grounded EQP orifice, releasing an amount of energy equal to the anode sheath voltage drop ${E}_{\mathrm{D}\mathrm{E}\mathrm{C}}=e{V}_{\mathrm{P}}\left(t+{t}_{1}+{t}_{2}\right)$. Ultimately, it will be detected with an energy of:

The simulation is primarily performed for O⁻, ${\mathrm{O}}_2^-$, and InO⁻ ions at a discharge power of 100 W, the essential parameters considered for the calculation are ${V}_{\mathrm{T}0}$ and ${U}_{\mathrm{T}}$, measured at 107 V and 70 V respectively, using a voltage probe. Moreover, the Langmuir probe yielded ${V}_{\mathrm{P}0}$​ at 22.7 V, while ${U}_{\mathrm{P}}$ detected by the floating probe stands at 20.0 V. The distance from the target to the EQP orifice, D, is fixed at 78 mm. The result is shown together with the experimental IEDF in figure 8.

Figure  8.  IEDFs for O⁻ (a), ${\mathrm{O}}_2^-$ (b), and InO⁻ (c) ions in RFMS discharge at a RF power of 100 W. The data, obtained through EQP measurements (depicted in red), are compared with simulations based on the model (shown in blue).

DownLoad: Full-Size Img PowerPoint

For O⁻ ions, the observed periodic peaks in the measured ion energy distribution are effectively replicated by our simple model by setting the transit time ${t}_{1}$ at 50 ns, particularly in the higher energy range, as shown in figure 8(a). Nonetheless, the sub-peaks in the simulated ion energy distribution appear more irregular when compared to the measured one, which display more consistent and repeatable double-peak periodic structures. At present, no explanation has been given for this mismatch, possibly due to the lack of specific experimental details integrated into the model. For ${\mathrm{O}}_2^-$ ions with lower velocity, the transit time ${t}_{1}$ is set at 70 ns, which closely aligns with the square root of two times the transit time for O⁻ ions. The peaks exhibit densification owing to the delayed response to the oscillating plasma potential, a characteristic feature observed with the heavier ${\mathrm{O}}_2^-$ ions. In the case of InO⁻ ions, a significant blue-shift towards the high-energy region is noticeable in the simulation results. This deviation can be attributed to the omission of collision effects in the simulation. Generally, the high-energy boundary of the simulation distribution is primarily determined by the value of (${E}_{0}+{eV}_{\mathrm{T}0}+{eU}_{\mathrm{T}}+{2eU}_{\mathrm{P}}$). Nonetheless, the mean free path of InO⁻ ions is anticipated to be shorter than that of O⁻ ions. This expectation arises from the larger collision cross section exhibited by InO⁻ ions owing to their greater diameter compared to O⁻ ions. As a result, InO⁻ ions experience a higher frequency of collisions along their trajectory toward the orifice, culminating in a discernible depletion of their energy.

Figure 9(a) shows the integrated IEDFs for negative ions. As RF power increases from 25 to 100 W, there is an increase in the flux of positive ions due to the increasing sputtering rate. The average kinetic energy of positive ions is illustrated in figure 9(b). Each positive ion exhibits an almost linear increase in average kinetic energy with increasing RF power. Notably, the energy of O⁻ ions demonstrates the highest growth rate, as O⁻ ions, being the lightest in mass, exhibit the fastest response to the oscillating plasma potential.

Figure  9.  (a) The integrated IEDFs and (b) the average kinetic energies of positive ions at different RF powers.

DownLoad: Full-Size Img PowerPoint

4.   Summary and conclusion

The study systematically examined the behaviors of positive and negative ions at the substrate position in RF magnetron discharges with an ITO target, using an energy-resolved mass spectrometer. For positive ions, the IEDFs reveal a dominant peak situated near the energy corresponding to the plasma potential. This observation suggests that these ions primarily originate in the bulk plasma and undergo further acceleration by the substrate sheath. With rising RF power from 25 to 100 W, ion energies increase about 3‒5 eV, according to the increase of the plasma potential and initial emergent energy. Simultaneously, the flux of positive ions increases due to the concurrent increase in sputtering rate and electron density. For negative ions, IEDFs exhibit broad distributions with multiple peaks, attributed to the combined radiofrequency oscillation of the target and the plasma potential, as well as the ion transport time. This observation is validated by a simple one-dimensional model that takes into account the initial ion energy. The flux and energy of negative ions exhibit a noteworthy increase with increasing RF power. The heightened flux of positive ions with energy below 100 eV is advantageous for the quality of sensitive ITO films, while the augmented presence of negative ions with energy exceeding 100 eV poses a risk of ion-induced sputtering damages to the ITO layers [11]. To quantitatively assess the impact of sputtering damages on ITO film properties, additional in-depth research through film characterizations is warranted.