Comparison of pulse-modulated and continuous operation modes of a radio-frequency inductive ion source

1.   Introduction

The research on magnetic mirror devices dates back to the 1950s [1]. Soon it was found that magnetohydrodynamic (MHD) instability plagued the plasma and the axial confinement rendered a simply mirror's fusion gain barely able to reach break-even [2]. To combat these deficits, the tandem mirror concept was proposed independently in the former Soviet Union and in the United States [3]. In the plug cells, a positive potential barrier was produced to reflect the escaped ions back to the central cell and hence improved the axial confinement. The instability was curbed by a minimum-B magnetic structure [4–6]. The positive results inspired the construction of a large tandem mirror system, MFTF (Mirror Fusion Test Facility) at the Lawrence Livermore National Laboratory [7, 8], which was then terminated prematurely due to budget cuts to fusion research in the United States.

However, experimental and theoretical studies on mirrors have never stopped, though in a limited scope and capability. Recently, a new axisymmetric tandem mirror concept, namely Kinetic Stabilized Tandem Mirror (KSTM), was proposed by Post [3, 9, 10] and Fowler [11]. It greatly reduced the complexity of mirror configuration by returning to the simply and fully axisymmetric magnetic configuration to avoid neoclassical turbulence which may occur in the minimum-B region [12–14], while the stabilization relied on the good magnetic curvature in the so-called expander chamber which required the thermal pressure on these good-curvature field lines to be high enough to overpower the curvature-induced instability from the bad curvature region along a flux tube. New mirror experiments, for example, WHAM and BEAT, will adopt such axisymmetric magnetic geometry and rely on the pressure-weighted effect to stabilize the plasma. Though there have been many theoretical [15, 16] and experimental studies [17–22] in the past; except for the axisymmetric GDT [21, 22], the magnetic configurations were not purely axisymmetric. Hence, we develop an experiment in the KMAX device, a fully axisymmetric mirror, to study this effect, and the results are reported below.

2.   Experimental setup

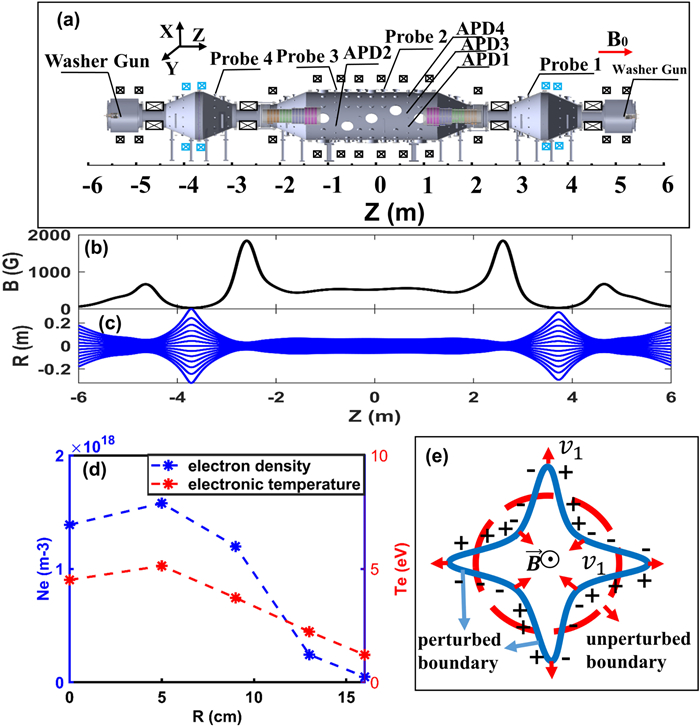
Figure 1(a) illustrates the KMAX device and the diagnostics used in this work. The base pressure inside the device is in the range of 10^-4 Pa and the working pressure for hydrogen is typically (2–5) × 10^-2 Pa. Plasma parameters in the central cell and side cells are $n_{\mathrm{c}}=(0.2–2)\times {10}^{18}{\mathrm{m}}^{-3},$ $n_{\mathrm{s}}=(1–5)\times {10}^{18}{\mathrm{m}}^{-3},$ $T_{\mathrm{ec}}=5–10\mathrm{eV},$ $T_{\mathrm{es}}=5–15\mathrm{eV},$ $T_{\mathrm{ic}}=1–5\mathrm{eV},$ β = 0.1%-0.3% ($\beta$ is the ratio of the plasma pressure to the magnetic pressure), respectively, with subscripts c denoting the central cell and s the side cell. Ion temperatures are measured by a spectrometer located at the midplane. The magnetic field flux densities in the central cell are 550 G and in the magnetic throats, it can be adjusted from 1500 G to 3200 G. In this experiment, we mainly use four radial movable Langmuir probes to measure the ion saturation currents, and the probes are, respectively, located at z=3.23 m, 0.48 m, -0.75 m, -3.23 m, which are labeled as probes #1, #2, #3, and #4 in that order. Four APDs (Avalanche Photo Diode) are used to measure the light emission from the plasma to assist identification of the flute instability. They are distributed at z=0.75 m, -0.75 m, 0.75 m, and 0.75 m, with angles=-24°, -24°, 0°, 72°, respectively, as shown in figure 2(a). The magnetic cusp configuration is formed by applying reverse currents to the light blue magnetic coils, which are also called 'bucking coils', in figure 1(a), and figure 1(b) and (c) respectively show the magnetic flux density and the resultant cusp configuration. Two washer guns installed at the two ends of the device are used to produce the plasmas. The typical radial density and electron temperature profiles at z=0.48 m are shown in figure 1(d). The azimuthal mode number m was deduced from APD 1, 3, and 4 measurements [23]. Finally, the parallel wave number is determined using four axially distributed Langmuir probes and confirmed by APD 1 and 2, see figures 2(a) and (b). The mode number of flute instability is m=1 measured by the azimuthal probe array, which is consistent with the APD measurement result.

Figure  1.  (a) Schematic drawing of the vacuum vessel and selected diagnostics on KMAX, (b) profile of the magnetic flux density on the axis, (c) field lines with cusp configuration, (d) typical plasma density and electronic temperature profiles, (e) an illustration of flute mode.

DownLoad: Full-Size Img PowerPoint

Figure  2.  (a) Fluctuations measured by APDs at different azimuthal and axial positions, (b) fluctuations measured by Langmuir probes at different axial positions.

DownLoad: Full-Size Img PowerPoint

3.   Experimental results

3.1   Identification of the instability

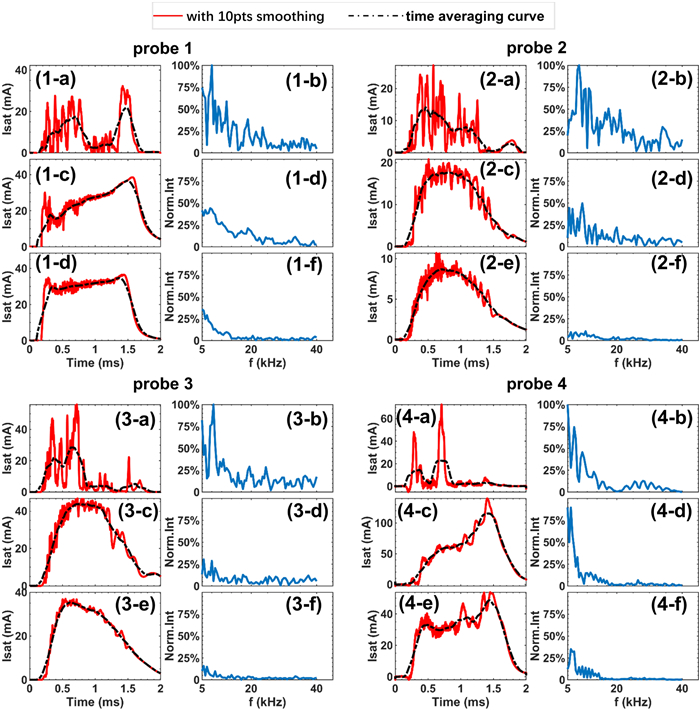
In a previous paper, we have confirmed the m=1 flute instability by the azimuthal probe array in the KMAX device [24]. In this work, we show that it can also be identified by optical measurements. Since m=1 was the main mode in the previous experiment, we use three APDs with an angular distribution capable of distinguishing m up to 7. The same as our previous probe measurements, the phase differences of APD 1, 3 and 4 suggest the mode m=1, and the phase difference in APD 2 and APD 1 is about zero, as shown in figure 2(a). This is consistent with our previous measurement [25, 26] that it is a flute mode with parallel wavelength k_||=0. Caution should be taken to interpret the optical data as it is a line-integrated measurement, however, here the optical data are only used to confirm our previous conclusion that it is a flute with frequency between 5 and 30 kHz.

3.2   Cusp stabilization

Flute instability is also called the magnetic Rayleigh–Taylor instability because the centrifugal force experienced by particles is equivalent to the gravity. The FLR (finite Larmor radius) effect will not be considered here since it plays a negligible role in the stabilization of the m=1 flute mode in the mirror magnetic field unless there is a conducting wall close to the plasma surface [27], which has been confirmed experimentally in GDT [28].

In the following part, we will introduce the experimental results and theoretical analysis of the stabilized plasma in the cusp magnetic field configuration. As mentioned above, we mainly use four axial Langmuir probes to measure plasma density fluctuations to analyze stabilization. The central cell magnetic field is fixed at 530 G, which is the fundamental resonance magnetic field for the KMAX-ICRH [29], and the field line curvature is varied by changing the magnetic field in the side cell or the throat magnetic field.

With a fixed magnetic field in the mirror throat, the coil currents in the side cell can be adjusted to vary the contour of magnetic flux. Figure 3 shows the raw data and their frequency spectra for three cases of no cusp, weak cusped field and strong cusped field measured by probes #1–#4 all at axis. In the case of no cusp, plasma is unstable with very large low frequency perturbations. The perturbations can be mitigated even with a weak cusped field. Such a stabilization effect is more significant in the central cell. Further increasing the cusped field can yield a further suppression of this low frequency perturbations. Hence, the data confirms the effect of cusp on the stabilization of the plasma column. Note, because our plasma has to go through the side cell and mirror throats to enter the central cell, a stronger cusp field can divert more plasmas and result in less plasmas into the central cell.

Figure  3.  Comparison of plasma with cusp off (shot number 48254, top row), weak cusped magnetic field (shot number 48269, middle row) and strong cusped magnetic field (shot number 48289, bottom row). The magnetic fields in the side cell are 100 G, 31 G, -17 G, respectively. The figures in first column of each quadrant are the ion saturation currents collected by probes at $r=0\mathrm{cm},$ with the black dash-dotted lines as the average values, and the figures in the second column are fluctuation in frequency domain.

DownLoad: Full-Size Img PowerPoint

A systematic scan of the fluctuation level as a function of the cusp strength is shown in figure 4. The fluctuation value is given by ${\tilde{I}}_{\mathrm{sat}}=\sqrt{\frac{1}{N}{\displaystyle \sum _{\mathit{i}=1}^N}{\left(\frac{x_{\mathit{i}}-\overline{x}}{\overline{x}}\right)}^2}$, where N is the number of data, $\overline{x}$ is the average value of ion saturation current, shown as black lines in figure 3.

Figure  4.  Measured fluctuations as functions of the magnetic fields in the side cell when (a) the magnetic throat field is 1900 G, and (b) the magnetic throat field is 2800 G. The probes are located at r=0 cm.

DownLoad: Full-Size Img PowerPoint

Figures 4(a) and (b) show how the fluctuations vary with the magnetic fields in the side cell when the magnetic throat fields are 1900 G and 2800 G with probes #1–#4 placed at the central axis. Figure 5 shows the magnetic profiles for different magnetic field strengths at the midplane of the side cell. As the magnetic fields in the side cell are scanned from 100 G to -35 G (a) and from 120 G to -25 G (b), the plasma fluctuations gradually decline. With increasing of cusped field, the fluctuation shows a rapid decline, and the calculated fluctuation value decreases from above 0.70 to ~0.20. Further increasing the cusped field, the fluctuations almost do not change and remain at the level of 0.1–0.2. Note the stronger the cusped field is, the closer to the magnetic axis the null point is. When the distance between the null point and the axis is less than 35 cm, there is a clear stabilizing effect.

Figure  5.  Magnetic profiles for different magnetic field strengths at the midplane of the side cell.

DownLoad: Full-Size Img PowerPoint

3.3   Stability criterion

The pressure-weighted curvature criterion derived by Rosenbluth and Longmire [30] for flute interchange stability is given by

where $\kappa$ is the curvature of the magnetic field lines, r is the distance of the magnetic field line from the axis. $p_{||}$ and $p_{\perp }$ are the parallel and vertical plasma pressures, respectively. For paraxial axisymmetric mirror systems, the stability criterion can be rewritten in a simple form [31]

where a(z) is the plasma radius.

Experimentally, it is difficult to measure the $p_{||}$ and $p_{\perp }.$ Since the pressure value must be positive, then if $I_{\mathrm{M}}={\displaystyle \int a^3}\frac{{\mathrm{d}}^{2}a}{\mathrm{d}{z}^2}\mathrm{d}z\ge 0,$ equation (2) must be satisfied.

In the cusp configuration, the field lines from the central cell may terminate on the wall of the side cell, thus, the plasma column can only be counted from the side cell to the central cell. Therefore, the integration path is chosen from the midplane of the KMAX to the point in the cusp where the curvature radius of the magnetic field line is comparable to the ion Larmor radius so the ideal MHD approximation is satisfied [20].

The integrations for two different amplitudes of the magnetic throat are plotted in figure 6, where the y axis is the magnetic field strength in the side cell. With zero reverse current, the integrated value, I_M, is negative and it becomes positive if the field in the side cell is large enough. With the enhancement of the cusped field, the I_M is larger, or the field line curves are further away from the plasma, making more positive contributions to equation (2), and the plasma shows a trend of stabilization, as evidenced in figure 4.

Figure  6.  The curvature integrations as functions of the magnetic field at the center of the plug cell when the magnetic throat fields are 1900 G, and 2800 G, respectively.

DownLoad: Full-Size Img PowerPoint

Note that in a cusp configuration, see figure 1(c) for reference, the curvature integral in the side cell is usually positive, while the curvature integral in the central cell is usually negative. The plasma in the side cell has a larger radius, acting as an expander plasma. The major difference is that in our case, the medium-sized washer-gun makes the plasma density in the side cell several times that in the central cell [32], suggesting that the integral calculated in this paper is smaller than the actual value if the pressure terms are counted.

In the case of the same magnetic field in the side cell, a more reversed current is required to make the integral I_M positive, see figure 6 for comparison. This is simply due to the fact that the throat magnetic field can also affect the field line curvature, and the I_M decreases with the magnetic mirror ratio increasing. In other words, a larger magnetic mirror ratio can make central cell plasma more unstable.

To study how the mirror ratio affects plasma stability, another experiment was conducted with the side cell magnetic field held at 30 G while varying the magnetic throat magnetic field from 1500 to 3200 G, or the corresponding magnetic mirror ratio (R_m) is from 2.8 to 6. When the magnetic mirror ratio is lower than 4, plasma density fluctuation levels are roughly unchanged; however, when the magnetic mirror ratio is greater than 4, the fluctuations start to grow, as shown in figures 6(a) and (b). Figure 8 is a calculation of I_M, which is consistent with that in figure 7.

Figure  7.  The fluctuation as a function of the magnetic mirror ratio for probes at (a) r=0 cm, (b) r=10 cm. The magnetic field in the side cell is 30 G.

DownLoad: Full-Size Img PowerPoint

Figure  8.  The curvature integration varies with the R_m.

DownLoad: Full-Size Img PowerPoint

Plotted in figure 9 are the radial locations of the null points, which increase almost linearly from r=0.29 m to r=0.47 m with an increasing mirror ratio. Tara tandem mirror [17, 19] has demonstrated that the closer of the magnetic null point to the plasma, the more stable the plasma is, which is consistent with our results. When the null point distance is about 40 cm from the magnetic axis, the plasma has an obvious stabilizing effect.

Figure  9.  Radial position of the null point versus magnetic mirror ratio.

DownLoad: Full-Size Img PowerPoint

4.   Conclusions

We have systematically studied the effect of the cusp field on the stability of the plasma column in a fully axisymmetric tandem mirror for the first time. Specifically, we present experimental evidence to confirm that the stronger the cusped field is, the more stable the plasma is. However, when the magnetic field in the side cell is completely reversed, the plasma density decreases significantly. In addition, the plasma density fluctuation is also related to the mirror ratio R_m. The higher R_m is, the farther the null point is from the magnetic axis, and the more unstable the plasma is. The experimental results are in agreement with the theoretical prediction. In our experiment, when the null point is 35–40 cm away from the magnetic axis, the plasma has good stability and the density is in a suitable range.

With the fully symmetrical configuration becoming the main feature of modern magnetic mirrors, the stabilization by field line curvature effect is worth new investigation. The stabilizing effect by applying cusp configuration in the side cell is global, so potentially one can apply this method without affecting the magnetic field configuration in the central cell. It is critical to the application of radio frequency heating in linear devices.