Efficient combination and enhancement of high-power mid-infrared pulses in plasmas

1.   Introduction

High-power intense mid-infrared pulses are of particular interest in a broad range of scientific fields due to their important role in strong-field physics [1, 2], bright high-harmonic generation [3], filamentation [4], time-resolved imaging of ultrafast molecular dynamics and structures [5], and infrared spectroscopy [6]. Many of these applications would highly benefit from the long carrier wavelength, intense field strength and high pulse energy, because the ponderomotive electron energy and the emitted photon energy are roughly scaled to $I_0{\lambda }_0^2,$ where $I_0$ and ${\lambda }_0$ are, respectively, the peak intensity and the carrier wavelength of the driving optical fields. However, for current ultrafast laser technology, it is still challenging to simultaneously attain these conditions. When the long-wavelength infrared pulses reach relativistically high intensity and/or multi-TW high power, they will open new avenues for investigating strong-field light-matter interactions [7], laser-plasma acceleration [8], and hard x-ray attosecond pulse generation [9]. Over the past decade, significant progress has been made in the development of high-power intense mid-infrared pulses by using various nonlinear optical materials [10–13], but it is difficult to achieve high-energy infrared sources in the spectral range of above 5 μm, especially for extending their intensities into the relativistic regime.

In recent years, plasma-based optical methods have attracted a lot of interest in the generation of high-power high-intensity optical pulses [14–17], since there is no damage limitation in the plasma. This suggests that the plasma-based approach can sustain more intense laser pulses with much higher power and higher intensity than traditional nonlinear crystals. Furthermore, high-power infrared pulses generated by plasma methods can be extended to wavelengths above 10 μm, i.e. to the mid-to-long wavelength infrared frequency range. It is still challenging to achieve these with conventional optical techniques such as chirped mirrors. Several novel concepts for relativistically intense few-cycle infrared light pulses have been recently proposed and/or demonstrated experimentally [18–24], but their spectra are quite broad and their peak powers are typically limited to the sub-TW range. Efforts continue to develop tunable intense long-wavelength infrared sources with relativistic intensity, narrow spectrum and high power in the multi-TW range, which are desired for many cutting-edge applications.

In this paper, we introduce an efficient way of plasma-based optical modulation to solve this intriguing quest. This is attained by merging several driving pulses into a single output pulse, which leads to remarkable increase in the power and intensity of the output pulse. Moreover, the resultant pulse will also undergo strong self-focusing during the interaction. As a consequence, more intense long-wavelength infrared pulses can be obtained while maintaining a narrow spectrum similar to that of the driving pulses. Although this may lead to a broader spectrum of infrared pulses than conventional optical techniques, it enables the light pulse intensity to be expanded to the relativistic regime.

2.   Physical scheme

Figure 1 illustrates the schematic diagram of our scheme. Two driving laser pulses with the same spatial and temporal distributions are incident on a gas target, where an underdense plasma is formed via intense laser field self-ionization. As the intense laser pulse propagates in plasmas, its ponderomotive force expels the plasma electrons out of the pulse driver leaving behind the large massive ions. The expelled electrons are then attracted by the Coulomb field of the ion column to form a plasma wave wake behind the laser pulse. The wave oscillates at the plasma frequency ${\omega }_{\mathrm{p}}={\left(4\pi e^2{n}_{\mathrm{e}}/m_{\mathrm{e}}\right)}^{1/2},$ where $n_{\mathrm{e}}$ is the plasma density, $m_{\mathrm{e}}$ is the electron rest mass, and $e$ is the elementary charge. This gives rise to nonlinear effects such as plasma density modification and the relativistic electron mass correction by the ponderomotive force. The refractive index in the process can be expressed as $\eta ={\left(1-n_{\mathrm{e}}/{\gamma }_{\mathrm{e}}{n}_{\mathrm{c}}\right)}^{1/2},$ where $n_{\mathrm{c}}={\omega }_0^2{m}_{\mathrm{e}}/4\pi e^2$ is the plasma critical density, ω₀ is the laser frequency, and ${\gamma }_{\mathrm{e}}$ is the electron relativistic factor. For a normal Gaussian laser pulse with an intensity profile peaked on the propagation axis, it can lead to the pulse guiding and self-focusing due to $\partial \eta /\partial r<0$ (i.e. a plasma focusing lens). These nonlinear effects become important as the power of the laser pulse propagation in plasmas exceeds the critical power threshold $17\left({\omega }_0^2/{\omega }_{\mathrm{p}}^2\right)\mathrm{GW}$ for relativistic self-focusing [25–27]. Nonlinear coupling interactions of two intense laser pulses copropagating in underdense plasmas have the potential to merge into one pulse beam, and so cause a considerable increase in the light intensity and peak power of the resultant output pulse. In order to describe the mutual interaction of two laser pulses through nonlinear effects in a comoving frame $\xi =x-ct,$ the coupled equations for pulse evolution in plasmas can be given by [28–30]

Figure  1.  Schematic diagram of high-power infrared pulse amplification using a plasma-based optical combiner. In this scheme, the energy of two input infrared pulses can be efficiently combined into a single pulse beam, thus greatly enhancing the light intensity and power of the output pulse.

DownLoad: Full-Size Img PowerPoint

where $\tau ={\omega }_{\mathrm{p}}^{2}t/{\omega }_0,$ ${\mathrm{\nabla }}_{\perp }^2={\partial }^2/\partial y^2+{\partial }^2/\partial z^2$ with the transverse coordinate y and z normalized by $c/{\omega }_{\mathrm{p}},$ ${\gamma }_{\mathrm{e}}\simeq {\left(1+{\left|a_1+a_2\right|}^2\right)}^{1/2},$ and $a_1$ and $a_2$ are, respectively, the normalized vector potentials of the two laser pulses in the slowly varying approximation. Here, $n_0$ is the initial electron density of the unperturbed plasma, and $\frac{n_{\mathrm{e}}}{n_0}=\max \left(0,1+{\mathrm{\nabla }}_{\perp }^2{\gamma }_{\mathrm{e}}\right)$ is the density disturbance associated with the ponderomotive expulsion of plasma density from the high-intensity regions.

3.   Results and discussions

3.1   Efficient combination and enhancement of high-power infrared pulses in underdense plasmas

To demonstrate the proposed scheme, we carry out two-dimensional (2D) particle-in-cell (PIC) simulations with the electromagnetic relativistic code EPOCH [31]. The simulation window has a size of $100{\lambda }_0\left(x\right)\times 180{\lambda }_0\left(y\right)$ with $5000\times 1800$ grid cells and four macroparticles per cell, which moves at the speed of light $c$ along the x-axis. Two driving laser pulses are linearly polarized along the y-direction, which have a same temporal profile of ${\sin }^2(\pi t/2{\tau }_0)$ in the longitudinal direction and the Gaussian spatial distributions of $a_1=a_0\exp \left[-\frac{{(y-{\sigma }_0/2)}^2}{{\sigma }_0^2}\right]$ and $a_2=a_0\exp \left[-\frac{{(y+{\sigma }_0/2)}^2}{{\sigma }_0^2}\right]$ in the transverse direction, with the laser amplitude $a_0=0.5$ (normalized by $E_0=m_{\mathrm{e}}c{\omega }_0/e$), spot size ${\sigma }_0=30{\lambda }_0,$ wavelength ${\lambda }_0=c{T}_0$ and pulse duration of ${\tau }_0=30T_0$ full width at half maximum. Here, we assume the wavelength of the driving pulses ${\lambda }_0=10\mu \mathrm{m}$ (i.e. the CO₂ laser), for example, one has the initial light intensity of $3.4\times {10}^{15}\mathrm{W}{\mathrm{cm}}^{-2}$ and peak power of 4.8 TW for each laser pulse. The plasma target has an initial electron density of $n_0=5\times {10}^{-3}{n}_{\mathrm{c}}$ $\left(\mathrm{where}{n}_{\mathrm{c}}=\frac{{\omega }_0^2{m}_{\mathrm{e}}}{4\pi e^2}\right)$ with a $50{\lambda }_0$ density rising edge, a $50{\lambda }_0$ density falling edge, and a $1500{\lambda }_0$ uniform density part. It should be mentioned that this scheme is also applicable to other optical wavebands, because both the laser and plasma target parameters are normalized to the laser wavelength ${\lambda }_0$ or its frequency ${\omega }_0.$

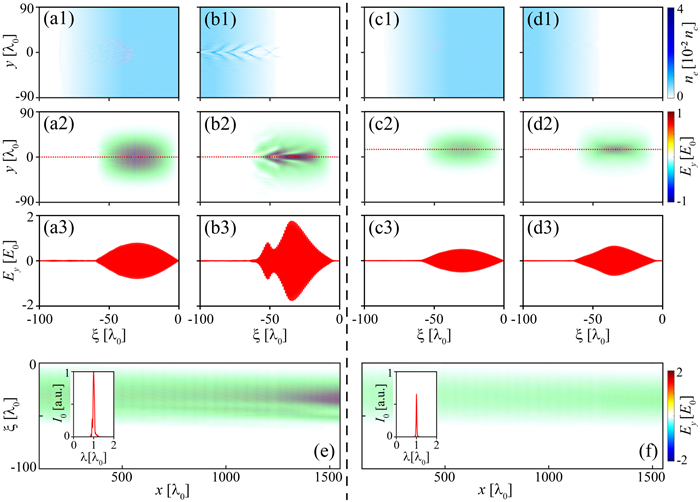
We now investigate the physical processes of plasma-based optical combinations occurring in this scheme. Figure 2 illustrates the evolution of the plasma density, the laser pulse and the transverse electric field. Two driving laser pulses, with $30{\lambda }_0$ transverse distance between their propagation axis, are irradiated into an underdense plasma, where they experience strong interaction and induce nonlinear effects such as plasma density modification and relativistic nonlinearity. In the earlier stage of the interaction process, both the transverse field and the plasma wave are relatively weak, as seen in figure 2(a). As the two pulses propagate in plasmas and undergo self-focusing individually, they appear to attract each other in this process. After an interaction distance of about $1500{\lambda }_0,$ the two driving pulses are merged into a single pulse with high field strength reaching about $1.8E_0$ more than three times higher than that ($0.5E_0$) of the initial laser pulse, as displayed in figure 2(b). In comparison, the field strength can only be amplified to about $0.6E_0$ in the one pulse scheme, as seen in figure 2(d). It should be noted that when two high-power drive pulses are combined into a single pulse with relativistically high intensity, a nonlinear plasma wake is excited, leading to stronger self-focusing and nonlinear effects, which in turn change the profile of the output pulses. Nevertheless, the distinct advantage of this scheme for enhancing high-power infrared pulses is that, unlike ultra-broadband spectrum radiation in previous plasma-based methods [19–24], the resulting pulses have a relatively narrow spectrum with a central wavelength around $1{\lambda }_0$ similar to that of the driving laser pulse, as shown in figure 2(e). The FWHM spectral width of the output pulse increases only from 0.03 to 0.08 compared to the initial input pulse. This makes it possible to obtain relativistically intense infrared pulses while maintaining narrow spectral characteristics.

Figure  2.  Distributions of the electron density, the transverse electric field of the evolved laser pulse and its on-axis electric field are shown at the beginning of the plasma combiner (a) and at the end of the combiner (b) with two driving pulses. The corresponding distributions are shown in ((c) and (d)) with a single driving pulse. Evolution of the on-axis electric field as a function of the propagation distance in the two-pulse case (e) and in the single-pulse case (f). The red dashed lines in ((a2)–(d2)) indicate the positions of the on-axis electric fields. The insets in ((e) and (f)) display the spectral distributions of the output infrared pulses.

DownLoad: Full-Size Img PowerPoint

For comparison, we also investigate the case of one driving laser pulse propagation in plasmas, while all other parameters are the same as those in the two-pulse case presented in figure 2. The findings indicate that one driving pulse can be still self-guided and propagated over a long distance in plasmas owing to its peak power exceeding the critical threshold 3.4 TW, but both the pulse self-focusing and plasma wave excitation are much lower than those in the two-pulse case, as shown in figures 2(c) and (d). This is attributed to the relatively weak nonlinear effects and ponderomotive force induced by one laser pulse. As a consequence, the evolution of the laser pulse propagating in plasmas is quite moderate with only a small increase in light intensity, as shown in figure 2(f), making it unsuitable for achieving high-power relativistically intense infrared pulses.

3.2   Effects of the laser and plasma parameters on the output infrared pulses

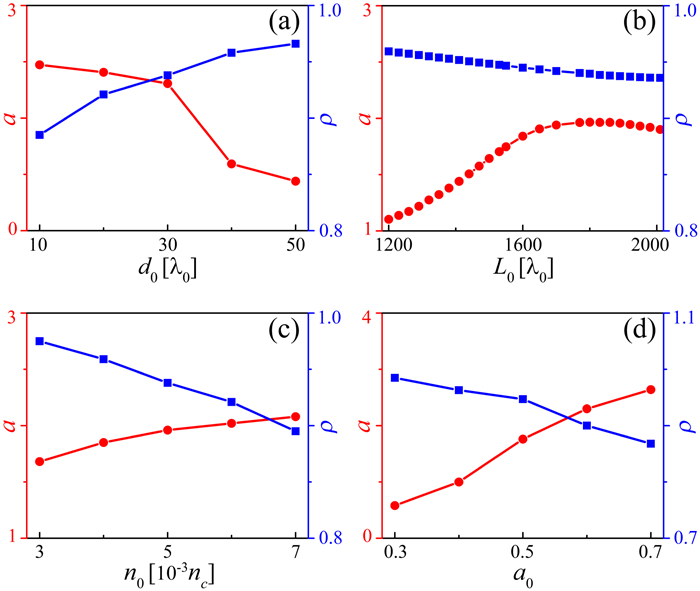
To demonstrate the robustness of the proposed scheme, we consider the effects of the drive laser pulse and plasma target parameters on the combination and enhancement of the infrared pulses in plasmas. We first investigate the effect of the transverse distance ($d_0$) between the two drivers on the pulse amplification in figure 3(a). The results show that the interval distance between two drive pulses should not be too large, otherwise they do not attract and combine well together. For example, when $d_0\ge 40\mu \mathrm{m},$ the intensity of the combined light pulse will be lower than the relativistic intensity. On the other hand, while a smaller pulse spacing is beneficial to achieve more intense combined light pulses, this may prematurely excite stronger nonlinear and relativistic effects, leading to a decrease in the conversion efficiency of the pulse amplification. Therefore, the optimal distance should be slightly less than $30\mu \mathrm{m},$ which is approximately the focal spot size of the driving laser pulse.

Figure  3.  Effects of (a) the transverse distance ($d_0$) between the two driving laser pulses, (b) the length ($L_0$) of the uniform density part, (c) the plasma density ($n_0$), and (d) the driving laser intensity ($a_0$) on the normalized intensity ($a$) and energy efficiency ($\rho$) of the output infrared pulses. Here, $\rho$ represents the ratio of the output infrared pulse energy to the input driving pulse energy.

DownLoad: Full-Size Img PowerPoint

Figure 3(b) illustrates the effect of the longitudinal length of the uniform density part of the plasma combiner on the resultant infrared pulse, where the plasma length ($L_0$) is changed in the range from $1200{\lambda }_0$ to $2010{\lambda }_0,$ while keeping all other parameters unvaried. It is shown that an appropriately long plasma length is beneficial for pulse attraction and combination, because the mutual interaction of optical pulses in plasmas and their nonlinear coupling effects become more strong. This allows the light intensity of the output infrared pulse to increase with the length of the plasma target. Nevertheless, the plasma length should not be too long; otherwise, it will cause significant pulse depletion or attenuation due to strong absorption. The simulation results show that the optimal plasma length is about $L_0=1800{\lambda }_0$ for high-efficiency intense infrared pulse amplification in the considered parameter conditions.

Figure 3(c) presents the effect of the plasma target density on the infrared pulse amplification, which is varied from $3\times {10}^{-3}{n}_{\mathrm{c}}$ to $7\times {10}^{-3}{n}_{\mathrm{c}}.$ Based on the critical power model that is inversely proportional to the plasma density as $17\left({\omega }_0^2/{\omega }_{\mathrm{p}}^2\right)\mathrm{GW}\propto n_{\mathrm{c}}/n_{\mathrm{e}},$ when using a higher density, one can obtain a lower power threshold for self-focusing, so that the mutual interaction and focusing effects of laser pulses and the plasma wave excitation become stronger according to equations (1) and (2). This is demonstrated by our PIC simulations, where one can observe that the intensity of the enhanced infrared pulses increases with the plasma density, as exhibited in figure 3(c). It should be noted that the energy efficiency of the output infrared pulse decreases with increasing plasma density due to the strong interaction between the laser pulse and the plasma resulting in a large amount of pulse energy being absorbed.

The effect of the driving laser intensity on the pulse amplification is illustrated in figure 3(d). Here all other parameters are the same as those presented in the two-pulse case in figure 2, except for the driving laser amplitude $a_0,$ which changes in the range from 0.3 to 0.7. One can see that the normalized amplitude of the output infrared pulse is approximately proportional to that of the driving pulses. This is because the effects of the ponderomotive force, plasma density modification, and relativistic nonlinearity are mainly determined by the driving laser intensity. Interestingly, even using two non-relativistic driving laser pulses with $a_0=0.4,$ it is still possible to obtain a relativistic-intensity output pulse via our scheme. This is due not only to the pulse merging effect, but also to the relativistic self-focusing of the amplified infrared pulse itself in the plasma. More importantly, about 95% of the total energy of the two drive pulses can be effectively combined into a single output pulse. Nevertheless, it is worth noting that the drive laser intensity should not be raised to an arbitrarily high value, e.g., for $a_0>0.7;$ otherwise, a lot of pulse energy will be absorbed and/or transferred to the plasma wave, and strong nonlinear coupling effects may damage the performance of the output pulse. In addition to these effects, electron injection, acceleration, and radiation may occur at high intensities [32, 33], we will not discuss these in detail here, because they are beyond the scope of this paper.

4.   Conclusion

In conclusion, we have proposed and numerically demonstrated a promising and efficient scheme to combine two high-power infrared pulses into one single, more intense pulse, so that the light intensity of the output pulse can enter the relativistic regime. The findings show that the output infrared pulses can be flexibly tuned by varying the driving laser and/or plasma target parameters, where more than 90% of the driving pulse energy can be converted into the output pulses. This scheme provides a robust and practical method for efficient combination and enhancement of high-power long-wavelength infrared pulses in underdense plasmas, enabling a new regime of relativistic intensity and monochromatic spectrum. Such intense infrared pulses may open new possibilities for a variety of applications from fundamental research to materials science and biomedicine.